 QUESTION:
QUESTION:
I have been having
an argument with a 'friend' for 3 years now about how
seadoo thrust works. He says that the water must hit
something in order to move the vessel forward. I believe
that it is basic mass * velocity = force.
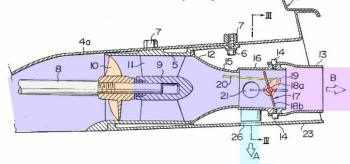
 ANSWER:
ANSWER:
Your friend is wrong.
I would guess
that his opinion stems from that old issue about how a
rocket works: the exiting hot gas must push against the
atmosphere to move the rocket. Therefore rockets do not
work in empty space, right? WRONG! Of course, you are
completely wrong when you say that the force is equal to
the product of the mass and the velocity. Mass (kg)
times velocity (m/s) does not even have the dimensions
of force (kg·m/s
2). The figure shows a
diagram of the jet. The engine is not in the figure but
is spinning the drive shaft 8. Attached to the shaft is
a propeller, referred to as the impeller 10. As it spins
it exerts a force on the water which pushes water out
the nozzle in a stream B. The water pushed out is now
replaced by drawing water in from the outside through a
hole in the bottom of the boat (on the left in the
diagram). Now comes the physics of the whole thing:
Newton's third law says that if the impeller exerts a
force on the water, the water exerts an equal and
opposite force on the impeller. But the impeller is
attached to the boat, so the force on the impeller is a
force on the boat which pushes it to the left. (I have
deleted the second question about the water and the wall
because it makes no sense;
resistance is not
defined.)
 QUESTION:
QUESTION:
Spinning metal shafts power our world as in generators, automobiles, etc. My question is does the very atomic or molecular center of a shaft also spin or does it remain still.
 ANSWER:
ANSWER:
First of all, you cannot apply the concepts of classical mechanics at a microscopic (atomic) level. The notion of the exact point of the axis of rotation being remaining
at rest assumes that the rotating object
has a uniform mass distribution; at the atomic level it
is not uniform at all. Also, in classical mechanics you
can talk about a point which occupies zero volume and
has no rotational degree of freedom and an atom
satisfies neither of those conditions.
 QUESTION:
QUESTION:
Re: speed of gravitational waves. Why is it said to be the same as the speed of light? The speed of light =1/root(eu) where e is the vacuum permittivity and u is the vacuum permeability. e is an electric field parameter and u is a magnetic field parameter, both of which affect photons.
Why would electric and magnetic parameters affect the speed of gravitational waves? It doesn't make any sense.
 ANSWER:
ANSWER:
W
e do not have a theory of quantum gravity, and therefore the graviton, which would play the
same role for gravity as photons play for electromagnetism
("messenger" of the field), is
merely a speculation. However, it is believed that a
graviton would have to be massless and therefore would
travel at the speed of light. Actually, there is a quite
good measurement of the speed of gravity which comes
from the gravity wave measurements at the LIGO/Virgo
gravitational wave detectors. Most events detecting
gravitational waves are from collisions and merging of
two black holes, and therefore we do not detect any
electromagnetic radiation since light cannot escape a
black hole. Rarely, though, gravitational waves from a
collision of two neutron stars is observed. In 2017 such
a collision was observed and 1.7 s later a gamma ray
burst was observed; this implies that the speeds of
light and gravity differ by less than one part in 10
15!
The 1.7 s difference can be attributed to the fact that
photons are affected by interaction with the
interstellar medium (not a perfect vacuum) thus slowing
them ever so slightly.
 FOLLOWUP QUESTION:
FOLLOWUP QUESTION:
The Physicist said: "However, it is believed that a graviton would have to be massless and therefore would travel at the speed of light."
this is what I don't get. why would EM parameters affect the speed of gravity. Here is my suggestion:
Early after the big bang, the four forces are thought to have been united. I suspect that when gravity and EM were united, they shared a parameter that determined the speed of all of their waves. i.e., they were the same, 300,000 km/s.
So speeds of grav waves and EM waves share a common origin and it is not correct to say gave waves are governed by EM.
 ANSWER:
ANSWER:
Since I state on the site that I do not do astronomy/astrophysics/cosmology, I will not comment on your hypothesis about the big bang and unified forces at the time.
However, gravity and electromagnetism are already linked and I will briefly explain how.
The theory of special relativity had its origin purely
in electromagnetism—the speed of light is
independent of the motion of the source or the observer.
And, although Einstein stated it as a postulate, that
fact is already a consequence of the transformation
properties of the waves which emerge from Maxwell's
equations, perhaps the best understood of all field
theories. Having formulated special relativity, Einstein
next turned to extend it to have a broader application,
the theory of general relativity. Of course, general
relativity is the best theory we have of gravity, and
since it is evolved from special relativity which
evolved from electromagnetism, we can say that gravity
has its roots in electromagnetic theory. Gravity must
then obey all the findings of special relativity, in
particular that the speed of anything must not exceed
the speed of light and a massless particle must travel
at the speed of light.
 QUESTION:
QUESTION:
leaving aside for the moment what relativity theopry says, do you know of any experiment that has been performed to show that pure energy can gravitate?
 ANSWER:
ANSWER:
What is "pure energy"? This has no meaning in physics,
energy is energy. And by "gravitate" do you mean responds to a gravitational field? So, I will choose light as
your "pure energy". It is an experimental
fact that light is bent when passing through a gravitational field, so it "gravitates".
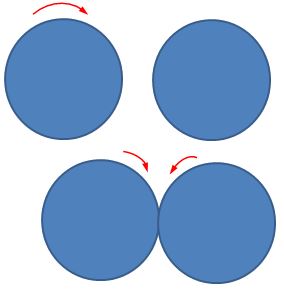
 QUESTION:
QUESTION:
my book says, "friction helps in transferring the motion from one body to another". I am not getting this. can you please explain this.
 ANSWER:
ANSWER:
Here is just one example of many I might think of. There are two wheels side by side; one is rotating with some speed as shown in the upper half of the figure, the other is not rotating. We
now move the rotating wheel so that it eventually touches the unrotating wheel. If there were no friction between the two wheels, the rotating wheel would keep rotating and the unrotating wheel would
stay stationary. But if there is friction, the stationary
wheel would feel a torque due the frictional force from the rotating wheel. This torque would cause the the stationary wheel to speed up; Newton's third law would
tell you that the rotating wheel would feel a frictional force in the opposite direction which would cause it to slow down. Some of the motion of one wheel has been transferred to the other by friction.
If the two wheels were identical, they would eventually
stop slipping would and the wheels would rotate with the
same speeds but in opposite directions.
 QUESTION:
QUESTION:
Is alternating
current the same principle as reverse polarity?
 ANSWER:
ANSWER:
Neither one of these is a "principle" but they are related to each other. Alternating current refers to a current which is changing direction; to change the direction you need to change the polarity of the electric
potential which is driving the current.
 QUESTION:
QUESTION:
William Herschel's
rainbow experiment discovered infrared rays. Looking at
the results of the experiment, we see infrared rays
hotter than visible red light and red hotter than blue
light. Why? The wavelength of infrared is longer than
red light and the wavelength of red is longer than blue.
Additionally astronomers tell us red stars are cooler
than blue ones.
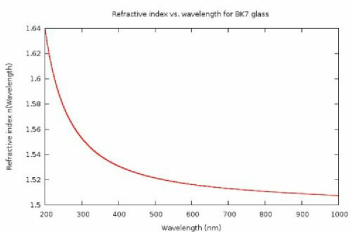
 ANSWER:
ANSWER:
A single photon has an energy proportional to its frequency and therefore inversely proportional to its wavelength. So blue photons have more energy than red photons;
this explains why red stars are cooler than blue stars.
So your question is why Herschel found higher temperatures at the red end of the spectrum than the blue.
The main explanation was, because he used a prism to
create the spectrum of sunlight, and the prism has an
index of refraction which is a function of the
wavelength. That is what causes different colors to bend
by different angles. But it is not a linear function of
wavelength, rather the curve shown in the figure. Note
that the short-wavelength (blue) index of refraction
changes much more rapidly than at long-wavelengths
(red). Therefore the blue portion of the spectrum is
much more spread out than the red and therefore the
thermometer for blue measures a smaller fraction of the
total blue spectrum than the thermometer for read
measures for the red part.
 QUESTION:
QUESTION:
I have seen many years ago a nasa invented patent which i will present to you in a link, which was supposed to propel itself by using double pendulum method. Im asking of you to check it, whether its false or true.
https://youtu.be/6A_cEZCXBIc I hired a specialist in blender to make this animation, based on my memory, because i couldnt find it.
 ANSWER:
ANSWER:
Sorry, I can make no sense of this. What I can tell you is that no isolated object can propel itself. If there are no external forces on an object its linear momentum (mass times velocity) must remain constant.
 QUESTION:
QUESTION:
Say I had an extremely bright flashlight, one impossibly bright for our current technology, and I shone it into space. If I were to then move that light a bit, would I be able to see, in real time, the ray of light bend as it catches up? Or would it be instant? This is specifically referring to the stratosphere

 ANSWER:
ANSWER:
Brightness has nothing to do with it—since
there is air and dust up through the stratosphere there
would be some scattering allowing you to see the beam
for any intensity. The question is whether you could see
a bend. The stratosphere is approximately between 10 km
and 30 km. How long does it take the light to travel 30
km?
t=3x10
4/3x10
8=10
-4 s.
Now, suppose you move the light through
θ=10°=0.175
rad in
T=0.1 s; if the transmission were
instantaneous the beam at
R=30 km would move with a speed
of
V=3x10
4x0.175/0.1=5.25x10
4
m/s so it would go a distance of
D=5.25x10
3
m. The light beam is not (as you state) a straight line
but lags the straight line a bit. All this is shown in
the figure where blue is for straight line travel
(instantaneous) and red for actual (but not to scale as
we shall see!) path. But the actual beam at 30 km will
lag the instantenous beam by
t=10
-4 s which means that the actual distance
traveled in 0.1 s is
D-d=V(T-t)=5.25x10
4(0.1-10
-4)=5.25x(10
3-1)=5.245x10
3.
So (D-d)/D=0.999; so
d is far smaller than
sketched in the figure, only 0.1% of
D. I suggest that it would
be virtually impossible to visually detect any curvature
of the beam.
 QUESTION:
QUESTION:
Why do we always see the same stars in the sky as the ancients did? As if we are ment to be traveling through space... wouldn't we see different stars and certainly wouldn't Polaris at least not be the same centre point?
 ANSWER:
ANSWER:
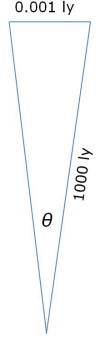
It mostly has to do with the enormous distances to stars from earth. I looked up some data: the speed of a typical star in our galaxy is about 8x10
5 km/hr which is about 10
-3c where
c is the speed of light and the radius of the
Milky Way galaxy is about 5x10
4 ly (light
years). So I will choose as a likely distance to a star
in our sky 1000 ly and a reasonable speed perpendicular
to our line of sight of 10
-3 c. In
one year the star will move 0.001 ly. I
have sketched this in the figure (obviously not drawn to
scale); the angle
θ is 10
-6
radians, about 0.0001°. In 1000 years it will only
have moved about 0.1°, measureable but not even
noticeable. So to see significant changes to
constellations you really need to wait hundreds of
thousands of years. Also of interest is that the earth
has a precession of its axis with a period of about
26,000 years. This does not result in any change of
relative positions of stars but rather moves them
around; in about 12,000 years Vega will be the north star, not
Polaris. Polaris (and everything else) would have moved about 40°
in the sky.
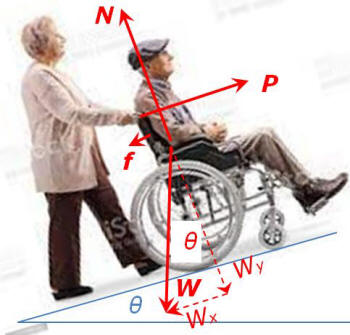
 QUESTION:
QUESTION:
I have a very mundane question. I have a job pushing wheelchairs up driveways and ramps, and also walking wheelchairs down ramps and driveways. So I'm interested in the force needed to do that based on the incline of the ramp or driveway.
Assume that a wheelchair has solid rubber tires that don't deform much. Assume also that the combined weight of the person and wheelchair is 400 pounds. How much force would be needed to push the person and chair
-
On a flat surface with no incline
-
Up an incline of 5 degrees
-
Up an incline of 10 degrees
-
Any other combinations of weight and incline that you might find interesting or instructive.
-
Also, would the force going in the reverse direction (walking the wheelchair down the driveway instead of up) be the same.
 ANSWER:
ANSWER:
I will solve the problem in general, then I can apply that to all your questions. In the diagram I show all the forces on the wheelchair plus occupant:
-
W, the net weight; I also show the components of W along and normal to the incline, respectively;
-
N the normal force which the incline exerts on the chair; this is the force which the incline exerts to keep the chair from falling through it.
-
f, the frictional force impeding the motion; this can be approximated as f=μN where μ is a constant. After searching around I found that
μ can be approximated by a small number of about 0.02.
-
P is the force which the lady (that's you!) pushes to keep the wheelchair moving with a constant speed.
All forces must add up to zero; we achieve this summing separately x and y forces:
-
N-Wy=0=N-Wcosθ or N=Wcosθ, and
-
P-Wx-f=0=P-Wsinθ-Wμcosθ, or P=W(sinθ+μcosθ).
Now I can answer your questions:
-
No incline means θ=0, so P=400x0.02=8 lb.
-
For θ=5, P=400(0.0872+0.0199)=42.8 lb.
-
For θ=10, P=400(0.174+0.0197)=77.5 lb.
-
Note in #5 that going down the incline the friction helps you; going up you have to push against it.
-
For going down the incline, θ is negative, so the equation for P (which is now a pull rather than a push) is
P=W(-sin|θ|+μcos|θ|).
So for θ=5, P=400(-0.0872+0.0199)=-26.9 lb.
 QUESTION:
QUESTION:
I understand why the twin paradox works in theory, but if man had the means to produce all the requisite energy to propel a spaceship at any acceleration for any duration of time , could a twin physically leave earth in a space craft and come back 50 years later noticeably different in age from his/her twin? Or would the amount of acceleration required to obtain the necessary relative speeds be to much for a human to bear?
 ANSWER:
ANSWER:
You are certainly right, the usual discussion of the twin paradox assumes that all accelerations are essentially
instantenous which would make the necessary force infinite. Because we evolved in an environment where the gravitational acceleration is about
g=10 m/s
2 we cannot endure
accelerations much larger than that. The largest a normal healthy person can endure is about 9 times
g and that only for a few seconds before blacking out.
So if you could maintain a constant acceleration of
g would be ideal, but slow; just to get to
c/2,
for example (in the traveling twin's frame) would take
about 6 months. You might be interested in this
web page.
 QUESTION:
QUESTION:
I have to settle a friendly bet. My friend says if gravity was ever instantly nullified for the entire planet Earth, that people and anything not bolted down would instantly begin to fly off into space. I disagree, because there are other forces at work like
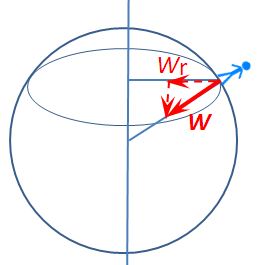 inertia, friction, air pressure, etc that I believe would need to be overcome before everything simply went into freefall. I realize that what he describes would eventually happen as those forces reconcile naturally...but how quickly would that happen?
inertia, friction, air pressure, etc that I believe would need to be overcome before everything simply went into freefall. I realize that what he describes would eventually happen as those forces reconcile naturally...but how quickly would that happen?
 ANSWER:
ANSWER:
Sorry, your friend wins this bet. Everyone on earth is moving in a circle as the earth rotates on its axis.
We are in uniform circular motion which requires a force
toward the axis to keep moving in the circle. This force
is the component of your weight toward the axis of
rotation, shown as
Wr in my figure.
If your weight
W disappears, so does
Wr
and you will continue moving in a straight line in an
easterly direction tangential to the surface. Being
tangential to the surface, you would be rising as you
moved; but the earth would be rotating beneath you so
you would perceive your path as approximately straight
up. Since the air is moving essentially with the
rotating earth, it would not much impede your departure.
Inertia is not a force. Friction from what? You are
essentially moving with the air, so no effects due to
air drag or air pressure would retard your departure.
 QUESTION:
QUESTION:
In Einstein's famous
equation E= MC2, has anyone ever demonstrated the
reversibility of the equation; I.e M= E/C2; the
transmutation of pure energy into newly created matter? I'm a scientist and this simple question has stumped both myself and my peers.
 ANSWER:
ANSWER:
"Pure energy" has no meaning. I can give you a couple of examples of energy being converted into mass. The first is called
pair production. A photon (a quantum of light, electromagnetic energy)
has no mass but has an energy
E=hf where
h is Planck's constant and
f is the frequency of the photon. If the energy
of the photon is large enough, it can (and does) turn
into an electron and its antiparticle the positron, each
with mass
me. A photon will not do
this on its own, but can be triggered to do it by an
intense electric field, for example close to a nucleus
of an atom. Another way that we can turn energy into
mass is to break apart some nucleus. A simple gedanken
is to break a nucleus into two pieces and pull them away
from each other so the two pieces end up very far apart.
Because we know that nuclei exist, those two pieces must
begin our experiment being very attracted to each other;
so to bring them very far apart from each other requires
that we do positive work (that which increases energy)
on them. If you now weigh the two pieces and compare
them with the weight of the original nucleus, you will
find more mass than you started, energy to mass.
 QUESTION:
QUESTION:
I am not a student...Brain storming. Q:in a 100' dia. X 50' high tank with a 10' dia. X 52' high cylinder attached in center,standing straight up (water tight). Fill lg. tank with water. How much pressure is exerted per square inch on inner cylinder at the bottom first few feet?
 ANSWER:
ANSWER:
The pressure in a fluid at depth
d is
P=Pa+ρgd where
Pa is
atmospheric pressure,
g is the acceleration due to gravity, and
ρ is the density of the fluid. This will
also be the pressure on your smaller cylinder at this
depth.
 QUESTION:
QUESTION:
I'm just writing a sci-fi story and am a little pedantic about details. My question is: if my space craft is launching from the Martian surface will my occupants and their unsecured things inside the space craft experience weightlessness like we see on space stations like the ISS at some point?
 ANSWER:
ANSWER:
During the launch they will not experience "weightlessness" because there is a net force upwards to accelerate the craft and all its occupants upwards. As soon as the engines
are turned off, though, they are in free fall and will experience "weightlessness". Be sure to understand that if you define (as I do) weight to be the force of gravity on something, they are not really weightless
but only feel like they are. Once they are far away from Mars, the gravitational force will be small enough that you may say they are approximately truly weightless.
 QUESTION:
QUESTION:
Is force the only thing that can make something move? And secondly, what makes something accelerate? Is it when energy changes form?
 ANSWER:
ANSWER:
Newton's first law says that an object in motion with constant velocity will continue like that until acted upon a force; forces change the motion of an object, but no force is required
to keep it moving. Newton's second law says that forces change the motion of a particle, accelerates it.
Your last sentence does not mean anything to me.
 QUESTION:
QUESTION:
So, kinematics is the about motion, disregarding the forces, like a(t) = dv/dt. What do you call "non-kinematics", when forces are included? Would you call uniform circular motion "kinematics" if what causes the centripetal force is not specified?
 ANSWER:
ANSWER:
Kinematics is the description of motion without any reference to the cause of the motion. If you attribute Newton's second law as being the cause of any particular motion, it is called dynamics.
If you say that an object moves in a circle
R
with constant speed
v and has an accelleration
with constant magnitude
a=v2/
R
which always points toward the center of the circle,
that is kinematics.

 QUESTION:
QUESTION:
My question could be a simple questions, but still I was not able to get a proper explanation for it from the people I asked.
If an ascending balloon in the air is carrying a box and the box is released, why does the box move slighlty upward before going down?
 ANSWER:
ANSWER:
I think you will understand better if I exaggerate the situation a bit.
Instead of a balloon drifting lazily upward, the box is
being carried upward by a rocket ascending with a speed
of 1000 mph. Surely you realize that the box is moving
upward with a speed of 1000 mph. And if you now release
the box, it would not simply immediately be at rest! It
will be going upward with a speed of 1000 mph, but would
begin slowing down immediately because of the downward
force on the weight of the box.
 QUESTION:
QUESTION:
Hi, a question from a home brewer. A constant heat source is applied to a container of water. Is the rate of boil off constant or does it vary as the volume of boiling water decreases?
 ANSWER:
ANSWER:
Once all the water comes to the
boiling point, all the energy being added (heat) goes
into the water*, a certain amount energy will result in
a certain amount water evaporation. So the rate should
not depend on the volume of water.
*I assume that
radiation losses from the container and the surface of
the water are negligible.
 QUESTION:
QUESTION:
It's been proven that time passes faster on the mountain top than on the lowland. Does this speed of the passage of time continue to decrease if it is monitored below the earth's surface toward the earth's core?
 ANSWER:
ANSWER:
The stronger the gravitational field,
the more slowly time passes. If you move from the
surface of the earth toward the center, the
gravitational field decreases and becomes zero at the
center. Therefore the clock inside the volume will run
faster than one on the surface.
 QUESTION:
QUESTION:
How is sound 'carried on the wind' when it itself is a frequency?
 ANSWER:
ANSWER:
Your question does not make sense to
me. I do not see what "…itself is a frequency…"
means and what that might have to do with sound being
"…carried on the wind…" Sound is a wave
which is distubances which propogates through the air,
maybe even one particular frequency but not necessarily.
If there is no wind, you would measure the sound to have
some speed, say vs. If there
happened to be a wind of speed vw
and blowing in the same direction as the sound is
moving, you would measure the speed of the sound to be
vs+vw . So I
guess you would say that the wind is carrying the sound
at a speed faster than the sound would travel with no
wind.
 QUESTION:
QUESTION:
Can elementary particles be created or destroyed.
The impression I am under based on the confusing answers I've found regarding this question is that as far as we can tell, they cannot. Which makes sense, because my understanding of our understanding of the subject at hand is that Elementary particles (Fermions/Bosons) cannot be created from nothing or destroyed into nothing. I get that with annihilation, fusion/fission, vaporization, and plenty more I didn't list or am unaware of basically result in the conversion and recycling of particles, but that doesn't mean, to me at least, they can be destroyed.
 ANSWER:
ANSWER:
Your understanding of the words created or destroyed is not what physicists would say those words mean. You are missing
the fundamental principle here. Any closed system must have its total energy conserved, not conservation of particle kind. If two protons collide with sufficent energy
they may both disappear but not into nothing. The energy
they had (including mass energy, of course) must show up
as some kind of energy so there has been no loss of
total energy. So the collision might result in several
particles which are not protons. So I would say that the
protons have been destroyed and some other kind of
particles have been created. But you would say that the
protons have not been destroyed and the other particles
have not been created. Your definitions is too
restrictive to be useful in physics. But, using your
definitions, no particle can be either created nor
destroyed.
 QUESTION:
QUESTION:
My intuition may be wrong here...
I get the feeling that I've sat in front of electric out door heaters which are really warm, you can feel the heat on your skin, even if there is a breeze. An outdoor LPG heater I guess is warmer on a still day but on a breezy day. What's going on here? Or am I just plain mistaken?
 ANSWER:
ANSWER:
There are three ways we generally
have heat transfer:
-
Conduction
where heat flows through a solid.
-
Convection
where heat is carried by currents in fluids.
-
Radiation
where heat is radiated away from something hot.
In your case, only
#3 is a significant contributor to the heat you feel.
The wind has almost nothing to do with it.
 QUESTION:
QUESTION:
What is the significance of the Dipole moment in electrostatics? No one simply multiplies the distance between charges and the magnitude of the charge without a reason.
 ANSWER:
ANSWER:
What
is the significance of a monopole (a single charge) in
electrostatics? Well they exist in nature and so we
might want to understand what the electric field of a
charge will be; it turns out, as you doubtless know, to
fall off like 1/
r2 where
r
is the distance you are from the charge. But, there are
two kinds of electric charge, positive and negative we
usually label them. And since pairs of equal electric
charges often happen in nature (for example a hydrogen
atom), we might like to know what the field of a dipole
looks like. It turns out that because there is zero net
charge in a dipole, at far distances from the hydrogen
atom you will see no field at all or at least you can
surmise that it falls off faster than 1/
r2. In fact it falls off
approximately like 1/
r3 and it is
not spherically symmetric like a point charge field is.
If you think about it, all molecules and solids are held
together by attractive forces between electrons and
protons. So thinking of molecules requires thinking
about dipoles.
 QUESTION:
QUESTION:
If a boxer was to throw a punch wearing 12 oz gloves and then throw the same punch wearing 16 oz gloves which one would have the most impact force/damage? (...not...homework, just a debate between friends who have no one with the intelligence to settle the debate.)
 ANSWER:
ANSWER:
A little research on my part found that the only differences are the weight of the glove and the amount of padding. The 12 oz gloves are what
are normally used in matches,
the 16 oz are for training and sparring. The reason they are used in sparring is that the extra padding will prevent hurting the other guy
as much when you throw your best punch.
 QUESTION:
QUESTION:
As energy could be
converted to mass, if the einstein's equation is
applied, then the equivalent mass will be produced. Then
the neighbouring planet will gets gravitational
potential energy, so after this proces, we created even
more energy than before? If it's correct, what's the
extra energy from?
 ANSWER:
ANSWER:
I
am assuming that at the beginning of the experiment you
have a planet and some energy density hanging around
somewhere nearby. To make things simple I will assume
that the energy density of the energy hanging around is
spherical and uniform; then I find a way to convert the
energy into mass which is also spherical and uniform. So
now you are saying that the energy arising in the
planet-mass system is different from what was in the
planet-energy system because of the gravitational
interaction between the two masses. But here is what you
are missing: not only mass causes gravity, but any
energy density causes gravity. In my example there is no
difference between the energy contained in the whole
systems because all the fields are exactly what they
were before the energy to mass conversion. Believe me,
in any closed system (interacting with nothing else) the
total energy remains constant.
 QUESTION:
QUESTION:
I am a criminologist so
this is out of my area but I need this information: As I
understand it, entanglement occurs when a particle pair
is divided and then separated Such as a photon. If they
had to be together and they became entangled in would
not the maximum distance they can never be effectively
tested such a test were to be developed would be within
the speed of light distance over a given time.
 ANSWER:
ANSWER:
You have sort of hit on why Einstein called it "spooky action at a distance"! What entanglement is is two particles, two electrons or two photons, for example, which have wave functions which are mixed. (Wave functions essentially
tell you something about the particles.) The most
common experiment is to prepare the system so that the
two electrons have zero net spin. In classical physics
this could only occur if one spins clockwise and the
other counterclockwise, so these would not be
"entangled". But in quantum physics a wave function need
not be "pure" and you could get zero net spin if both
particles were 50-50 clockwise-counterclockwise and we
would say that they are entangled. Some time later, and
it could be as long as you like, you make a measurement
to determine the spin of one of the two and determine
that it is spinning counterclockwise;
instantaneously the wave function of the other
would become pure clockwise. What a measurement does it
to "collapse" the wave function and put the particle
into a "pure" state. And the catch here is the word
instantaneously means just that so any worries about
waiting for the second particle to "get the message"
that her partner has been determined to be in a pure
state are not relevant. In principle you could wait
years to make your measurement and then determine the
immediate result on the other; you would have to do some
very careful timing of the measurement of the far gone
particle but you would find that it happened at the same
time as your measurement.
 QUESTION:
QUESTION:
I have a question regarding light and mirrors. I have been taught that light consists of all colors and that things that appear to be white are only white because they reflect all light. This has me confused because there's another thing that i know of which also reflects all light, mirrors. Why is it then that mirrors create a perfect mirror image whereas a white piece of paper is only the colour white?
 ANSWER:
ANSWER:
Because a mirror
has a very smooth reflective surface and therefor the
law of reflection, that a ray of light reflects at the
same angle relative to the normal with which it came in,
and therefore an image will be formed. A piece of paper
is rough and any ray of light which strikes it will
reflect out at a random angle.
 QUESTION:
QUESTION:
This question comes from my 11 year-old, who thinks about this stuff while falling asleep. Some objects, like dry wood, float because they are less dense than water. Water is heavy. Is there some depth where the weight of the water column above an object would keep it submerged, even though the object is less dense than water. If the less dense object would rise regardless of the depth, why doesn't the weight of all the water above keep it down?
 ANSWER:
ANSWER:
As you go deeper and deeper the pressure in the water gets larger and larger. Suppose that you have a cube of wood. So, the force down on the top of the wood is enormous
so you might think this would certainly hold it down. However, if you go a little bit deeper to the bottom of the cube you find the pressure is a little bit bigger than at the top, so the force up on the wood is a little bit bigger
than the force down at the top.
 QUESTION:
QUESTION:
Witch falls faster a bowling ball or a tennis ball
 ANSWER:
ANSWER:
In a vacuum, both fall the same. In air the force
F due to air drag, which points upward if the
ball is falling, may be approximated as ¼
Av2 where
A is the area presented to the onrushing air and
v
is the speed. The net force on a falling ball is
mg (the weight of the
ball) down plus the drag force, ¼
Av2-mg=ma
so the acceleration is
a=[¼
Av2/
m]
-g.
Putting in the size and mass of the two balls I find
that the drag accelerations are
atennis=0.015
v2
and
abowling=0.0013
v2.
The drag causes more than 10 times the acceleration on
the tennis ball which will therefore fall more slowly
than the bowling ball.
 QUESTION:
QUESTION:
Momentum is always conserved, whenever I hear a
statement that momentum is not conserved, it is usually
because the system being evaluated is not big enough to
account for all the momentum. With that said, I often
hear statements that momentum is not conserved in
General Relativity, but if you account for the momentum
in the field, momentum is conserved. maybe not in the
mechanical mass x velocity aspect, but if you account
for all the momentum, including field momentum it
appears to all be accounted for. So, with all that said,
why do you read / hear statements about how General
Relativity does not conserve momentum? Is it because
they are only viewing the mechanical mass x velocity
momentum? And not taking into accounting for the
momentum in the gravitational field?
 ANSWER:
ANSWER:
Details regarding
general relativity are beyond the scope of this site; I often answer broad questions on that subject, the general principles (principle of relativity, spacetime, equivalence principle,
etc.) Let me tell you
what I do know about linear momentum and then I will point you to a link where you can learn more about your question.
In Newtonian
mechanics, a vector quantity equal to the rest mass
m times the velocity v is
found to be constant for isolated systems; d(mv)/dt=dp/dt=Fext.
The quantity Fext
is the net sum of all forces acting on the system from
the outside; similarly, p is
the vector sum of all linear momenta of all the pieces
of the system. This equation is just a different way of
writing Newton's second law, so you may think of linear
momentum conservation as a clever trick, not some new
physical law.
In classical
electricity and magnetism, you are right: if you want to
see familiar conservation princlples you must consider
the energy, linear momentum, and angular momentum content
of the fields.
In special
relativity, if you define linear momentum as rest mass
times the velocity you find that it is not conserved for
isolated systems. It turns out that you have to redefine
linear momentum as γmv it is conserved
where γ=1/√(1-(v2/c2)).
I have discussed this here many times before and if you
go to the faq page and search for momentum you will find
several links. A more sophysticated way to view all this
is in terms of 4-vectors where the energy-momentum
vector has the (new) linear moment as the space-like
components and energy as the time-like component.
Finally your
question, what about general relativity? From my brief
reading it seems to be a lot like electromagnetism—when a particle is bent by gravity, thereby changing its momentum, the field is also altered. General relativity is a very mathematical
theory and so any discussion of details is likely to be
beyond the purposes of this site. There is a good
readable discussion at this
link.
 QUESTION:
QUESTION:
I am sure you know that if you drop a hammer and a feather in a vacuum from an equal height, they will both hit the ground/floor at the same time.
This I belive is because gravity exerts the same force on both objects but the feather has more air resistance to weight ratio.
I drive trucks for a living so forgive me if this sounds dumb as I have no scientific training.
My question is that once they are on the ground, why is the hammer harder to lift than the feather?
I understand the hammer weighs more, but isn't weight just a consequence of the pull of gravity?
The hammer weighed more when it was falling but was attracted to the earth's centre at the same rate as the feather (in a vacuum)
 ANSWER:
ANSWER:
Gravity does not exert the same force on each, it exerts a force (called its weight) which is proportional to
the mass of each,
w=mg for the feather
and
W=Mg on the hammer where
g is the acceleration due to gravity.
Now, Newton's second law tells us that if an object with
a mass
m (or
M) experiences a force
w
(or
W) it will experience an acceleration
a=w/
m=mg/
m=g.
(or
A=W/M=Mg/M=g). So you see, with
no other forces they will have the same acceleration
which means they would speed up together thence hitting
the ground simultaneously. In words, mass is a measure
of the resistance to being accelerated by some force and
weight is a force which is proportional to mass, then
all masses fall with the same acceleration. In the real
world there is air, and there is therefore air drag
which is approximately proportional the the square of
the speed and points upward, but it does not depend on
the mass. Therefore the larger mass is less affected by
the drag and wins the race as you know it must!
 QUESTION:
QUESTION:
Since all objects that have mass -the earth, a book etc. - cause a distortion of spacetime resulting in gravity, and E=mc2 tells us that mass and energy are the same thing, shouldn't energy cause a distortion of spacetime resulting in gravity?
 ANSWER:
ANSWER:
You are absolutely right! Spacetime is distorted by energy density.
 QUESTION:
QUESTION:
I'm a forensic scientist and feeling a bit out of my
league here. I'm working on research to combat specific
junk science ("grave dowsing"). I've published a blind
study that showed the technique ineffective but one of
the proponents continues to espouse what are clearly
erroneous statements about a piezoelectric property of
bone and oscillation of electromagnetic fields that he
claims cause the divining rods to move. I've only seen
one thing published about this, a really horrible
article published out of the University of Lulea,
Switzerland that had some overt and unforgivable errors
in design. I think just a general understanding of
simple physics make it obvious that even if bone had
piezoelectric properties (which I very much doubt), that
the electromagnetic field produced would be able to
affect metal rods "1/2 mile away" as this instructor is
telling students. I could do a bunch of experiments to
disprove this but I think the answer is already there in
physics and associated mathematics but I don't possess
anything near the understanding of the mathematics to
work the equations. My question, if you can forgive all
the buildup, is whether you think I'm on the right track
looking at the Lorentz force and Maxwell's equations.
Would I be able to use those equations to figure the
amount of charge that would have to be produced by a
bone in order for it to move a metal rod even a meter
away?
 ANSWER:
ANSWER:
Dowsing is a really tricky thing to try
to analyze. There are some very bright people who think
that dowsing for water works. And of course water is not
going to exert a force on a willow branch or coat
hanger. My feeling is that, like the old ouiga board,
that the pushing of the stick is done by the dowser. And
it is altogether likely that he does this unconsciously;
these are often old codgers who have been doing this
most of their lives and have experience. Another
suggestion is that the some of the successes of water
dowsers are because they are often working in areas where a
drilled well anywhere in the vicinity would probably
find water. So, now, what about the grave dowsers? I
have found that bones do indeed have piezoelectric
properties; it plays important roles in bone formation.
It is not clear to me if it has to be a living bone, but
just let us suppose that the weight of the ground above
pushing down on the bone (a person in a coffin would not
have any pressure being applied to his bones) creates a
potential difference. The thing is that the net charge
created is zero, the bone becomes positively charged on
one side and negatively charged on the other; in that
case, it looks like a parallel plate capacitor and
nearly all the electric field would be from positive to
negative, nearly all the field being confined to the
bone. And, because it is dipolar, whatever field exists
outside the bone would fall off very quickly (faster
than the field of net charge which falls off like 1/
r2
with distance
r); the field the dowsers think
they can detect would fall off more like 1/
r3.
Add this to the fact that piezoelectric fields are very
weak in the first place, I can see no possibility that
they could be detected even by the most sensitive of
instruments, let alone a piece of a coat hanger.
Devotees are very serious about this, though, and it is
a losing battle to try to convince them otherwise.
Collect as much data regarding success/failure rates, do
a good statistical analysis, and be happy with that.
Incidentally,
statistical analysis is vital. The classic example is
from several decades ago. For years it was presented as
fact that clusters of cancer occur in communities where
high voltage power lines crossed over, presumably due to
the electric and magnetic fields associated with them.
This was totally debunked by doing proper statistical
analyses.
 QUESTION:
QUESTION:
If the angular and the linear velocity of a wheel at the point of contact to the road is equal to zero.
Where does the velocity or the kinetic energy of the still moving car all go?

 ANSWER:
ANSWER:
It didn't "go" anywhere. There is no rule that anything which has kinetic energy must have all parts of itself moving with the same velocity.
Those parts of the tire in contact
with the road contribute nothing to the kinetic energy of the whole car.
Perhaps it is a bit easier to understand with a WWII
tank. The tread on the ground is at rest; the tread on
top has to be going faster than the tank as a whole; all
the wheels and gears are spinning and different parts
are going up or down or forward or backward or in all
other directions with different speeds. But, if you add
up every single part's contribution to the kinetic
energy of the whole tank, it will stay the same if the
engine provides enough energy to overcome all the
frictional forces present.
 QUESTION:
QUESTION:
Suppose you have two massless containers that are empty. They have no mass so there is no charge nor gravitational attraction between the containers.
If we keep adding protons and neutrons into each container equally, a coulomb attraction from charge and a gravitational attraction due to gravity will develop. Initially, the coulomb force will far outweigh the gravitational force. However, as we continue to add protons and neutrons to the containers, the amount of mass collected will grow to the point where the macro size is large enough to no longer have much coulomb attraction.
However, the gravitational attraction will grow.
Is there a point where the attraction of charge will equal the attraction of gravity. Has this been investigated? And has this been achieved?
 ANSWER:
ANSWER:
I have held back thinking about how to answer this question for some time now. Only this morning did I realize that it is impossible. The reason is that if each
container has a mass
M and a charge
Q, what determines the relative magnitude of the forces between the two containers due to gravity and to Coulomb
forces is only going to depend
on the ratio
M/
Q; but you propose to
add equal amounts of charge and mass to each container
so this ratio would never change!
There is also the
problem of the interaction among the nucleons in the
containers. You are essentially imagining creating super
heavy nuclei and in any nucleus the mass is not equal to
the sum of its parts. It would be impossible to
determine the mass of systems of neutrons and protons in
the numbers far above natuarally occuring nuclei
What I can do is
calculate the ratio for two hypothectical point masses
(M) and point charges (Q) separated by a
distance R such that the attractive
gravitational and repulsive Coulomb forces have equal
magnitudes.
GM2/R2=kQ2/R2
M/Q=√(k/G)=√(9x109/6.67x10-11)=1.16x1010
kg/C.
 QUESTION:
QUESTION:
Let's say there is a container that contains water and an ice cube in floating in it. Some portion of ice is below the water (may be referred as bottom of ice) and some above (may be referred as top of ice). Now volume of only submerged portion of the ice is equal to the volume of the water displaced, isn't it? If so, where did the volume of melted upper portion of the ice that did not displace the water previously go? Should it not be added, hence increasing the total content of water? But that's not the case according to text books.
 ANSWER:
ANSWER:
The reason is that the ice does not have the same density (less than) as the water; that's why if floats. But, if it melts it become water and therefore has a density the same as the water.
The fractional amount by which the density decreases is precisely equal to the amount by
which the volume decreases.
 QUESTION:
QUESTION:
My question has to do with wind chill. I believe that I understand the basic mechanisms by which this works, but my question gets more convoluted. Thinking about how objects which fall into the earth's atmosphere from space will burn up due to the extreme heat of friction with the air, I am wondering if it is possible to say at what velocity would wind change from having a chilling effect to having a heating effect due to friction? My guess is that it would require wind speeds which would far surpass the point of being fatal to a human being, thus negating any specific concerns about wind chill as such,
but who knows?
 ANSWER:
ANSWER:
First of all, you are extrapolating a
qualitative quantity (essentially "how cold do you feel
in a wind") into a region where it was never meant to be
applied. You can find lots of details about wind chill
in the
Wikepedia article, but this article also states "Windchill temperature is defined only for temperatures at or below 10 °C (50 °F) and wind speeds above 4.8 kilometres per hour (3.0 mph)".
The reason is that this effect of wind is to hasten
temperture loss of the body due to the wind, and is most
certainly not applicable to situations where the effect
of wind is to increase, not decrease, temperature. In
the summer time the main reason we feel hotter or cooler
is the humidity. Instances where air drag has a
nonnegligible effect of heating or cooling, these ideas
do not apply. There is, in fact, no way to have a simple
qualitative formula for the effect of speed on heating
because it depends on the shape and size of the object
and on the density of the air through which it is
moving; the SST, for example had a "needle-shaped nose"
to minimize heating at supersonic speeds and would have
burnt up otherwise. The takeaway here is that
qualitative quantities like this may play an important
role in health and safety (
e.g., high winds in
cold weather can result in high probability of
frostbite) and provide information helpful to deciding
how to dress, for example, in different wind conditions.
 QUESTION:
QUESTION:
Hi. My question has to do with gravity. If you took two planets with the same mass, in an area of space without any other immediate gravitational influences, and put them just within each other's gravitation pull but not moving, would they collide, or would they orbit each other? If they would end up orbiting each other, why?
 ANSWER:
ANSWER:
As long as these planets are spherically symmetric (center of mass at the center, density only dependent on distance from the center), their centers will accelerate with equal accelerations
until they collide. They could either stick together and come to rest or bounce off each other and each recoil with equal speeds in opposite directions.
If they were not spherically symmetric, their centers of
mass would certainly not be exterior the the planets, so
their centers of mass would accelerate towards each
other and they would still collide and therefore not
orbit; if they stuck together, they would rotate about
their total center of mass but have no translational
kinetic energy.
 QUESTION:
QUESTION:
If two objects move parallel at the same speed light years away would the a person on one object be able to see the other object? This is assuming a similar starting position, my initial assumption would be no as any light from one object would fail to reach the position of the second object. Or would the two objects be able to observe each other but one would appear "behind" the first? I know its multiple questions but what would be the shift of the object if the second question is in the positive?
 ANSWER:
ANSWER:
Yes, the light from the trailing object would reach the leading object
Y years after it was sent where
Y is the number of light years between the two.
You are not thinking about this problem using the
principles of special relativity (SR). Perhaps the most
important assumption of SR is the principle of
relativity which asserts that the laws of physics are
the same in all inertial frames of reference. An
inertial frame is one which moves with constant velocity
relative to another frame in which you know the laws of
physics. In your case, since both objects are moving
with constant speed in the same direction, they might as
well be at rest.
 QUESTION:
QUESTION:
Is anyone trying to figure out how to tap into the energy that holds atoms together? I don't mean harnessing it to fuel our energy needs. I mean accessing it like we would a hard drive.
It's the one thing that everything in the universe has in common and it's the one thing that connects us all. In it is knowledge beyond comprehension.
 ANSWER:
ANSWER:
So, the question you are asking is very vague. For example, what does "holds atoms together" mean?
-
Does it mean what holds atoms together in a macroscopic piece of matter?
-
Does it mean
what holds the atom itself together?
-
Does it mean what holds the nucleus of an atom together?
And what does "accessing" mean?
What we often think of when something is being held
together is a force; in more advanced physics we usually
express the presence of forces by introducing potential
energy functions. So I am going to answer your question
with a very broad brush; in essence, to "access"
anything at the atomic level you need to ascertain, by
doing experiments and interpreting the data, what the
relevant potential energy functions are (or forces, if
you like). And, guess what! That is what physicists and
chemists have been doing for the past several hundred
years. Condensed matter physicists address #1 above.
Atomic and molecular physicists address #2. Nuclear and
particle physicists address #3. In fact, thinkers from
thousands of years ago, Greek philosophers for example,
have been puzzling over these kinds of questions. So, is
"anyone
trying to figure out" answers to these questions? You
bet! Thousands of men and women for thousands of years!
 QUESTION:
QUESTION:
Can air pressure be different inside the same container? I'm having a debate with my work. WE have a device (packer)that inflates like a long cylindrical ballon inside sewer pipes to install fiberglass patches. They are claiming that if our packer is at the end of the pipe and sticks out at all, that the air pressure inside the pipe, and contained, is less than the air pressure that is outside of the pipe and is bulging out because it is not contained (within the pipe). I say that the pressure is constant throughout the entire thing and there is no change in pressure inside vs outside the pipe because pressure has to be constant inside one container. Who is correct?
 ANSWER:
ANSWER:
So, lets assume that there is some pressure
P inside the balloon and it has some volume
V and that it is wholly inside the pipe. Now it
comes to the end of the pipe and starts expanding
outward since it no longer has the pipe to keep it to
its previous cylindrical shape. If this happens pretty
quickly the temperature will approximately stay the
same. So the product of
P and
V will
remain constant as it expands. The volume will increase
so the pressure will decrease. During the time this
expansion is happening, there will be a different
pressure inside the pipe and outside but that will be
for a very short time, the time it takes for the
slightly lower pressure to equilibrate throughout the
volume. If everything is static and in thermal
equilibrium, the pressure will be the same everywhere.
 QUESTION:
QUESTION:
I have a question pertaining to sound. So, I was
wondering how it is possible for me to hear someone
facing away from me in say, an open field. Because I
know for example, if in a closed space, the sound would
bounce off the wall and come back to me, at least that's
how I think it works. But if I'm able to hear someone
facing away from me in an open field, Is sound more
complicated than a directional wave, is it more spread
out than I think?
 ANSWER:
ANSWER:
Usually in physics we think of wave
sources in some simple geometric form. For example, if
you think of sound as just like a laser for light as a
narrow coherent beam of sound coming out of your mouth,
only someone standing in front of you would be able to
hear you, clearly not the case. If you think of it as a
point source but radiating only forward, anybody in
within a hemisphere in front of you would be able to
hear you. But, as you note, this second simple model
does not fit evidence from the real world, so it must
also be incorrect. But if you think of it as a simple
point source it would radiate in all directions. But
that would say that I hear you just as loud whether in
front of you or behind you; this also is not what we
experience which is your voice is less loud if I am
behind you rather than in front of you. However, we
could account for the smaller amplitude from be due to
the sound source being much more forward in the
head/throat so sound emitted backwards gets attenuated
passing through the head tissues and bones. The fact is
that the source of a human voice is complicated; there
is the larynx containing the vocal cords, the primary
source of sound; but then there are also resonant
cavities*, the mouth, nasal structures, sinuses,
etc.,
which amplify and rebroadcast the sound.
*If you just had a
guitar string simply stretched between two points in a
room, you would be hardly able to hear it. The "box" of
the guitar plays a vital role by resonating and
amplifying the sound from the vibrating string.
 QUESTION:
QUESTION:
I've been thinking about the rising prevalence of coilguns, and an interesting puzzle came to my attention, which it is unfortunately beyond my limited "physics" ability to solve.
Some context:
Rifling in conventional kinetic firearms use grooves in the barrel to change some forward momentum into rotational energy, affording stability to the projectile.
However, I want to try to achieve, with magnetism, an inverse effect - converting rotational energy (at least partially) into forward momentum, without any physical contact with the rotating entity in question.
Assuming no constraints imposed by current technological progress:
What forces, where, and when would be required to achieve such an effect?
Feel free to go into any level of technical detail that you feel is necessary.
 ANSWER:
ANSWER:
You did not mention why this is of
interest to you, so let's assume, since you mention
guns, for purposes of an example, that you would like to
take a rotating bullet-like object and convert
its rotational kinetic energy into translational kinetic
energy. Consider a solid cylinder with mass
m, angular velocity
ω, radius
R, moment of inertia
I=½
mR2.
So, it will have a rotational energy
K=½
Iω2 which we wish to convert 100% into translational kinetic energy
K=½
mv2. If you do
all the requisite algebra you will find that
ω=(√2)(
v/
R).
Suppose
R=1 cm=10
-2 m, a very big
bullet, and
v=1000 m/s, a modest bullet speed;
then I find that
ω=1.4x10
6 rpm.
More than a million rpm means that this would not be a
good way to get energy to launch a projectile.
 QUESTION:
QUESTION:
Baseball commentators often say a hard throwing pitcher is "providing the power". They are meaning the batter doesn't need to swing as hard as he would have to if the pitch was slower. I don't think that can be correct since the faster pitch will have more momentum in opposite direction of the force provided by the bat. What is the correct way of thinking about that?
 ANSWER:
ANSWER:
I believe that the best way to think about this is using impulse. The impulse is the average force
F times the time Δ
t which the ball and bat are in contact,
I=FΔ
t.
Because of Newton's second law,
F=Δ
p/Δ
t,
I=Δ
p where Δ
p is the change of linear momentum. If the incoming pitch has a speed of
v1 and the outgoing ball has
speed
v2, the change in momentum is
m(
v2+
v1)
where
m is the mass of the ball, and therefore
v2=(
I/
m)-
v1.
So you can see that the faster the pitch, the slower the
speed of the hit ball if the batter exerts the same
impulse to the ball. I suspect that the reason the commentators
believe this is that a fast ball right down the middle,
often called a mistake on the pitcher's part, probably
yields the most home runs since it is the easiest for
power hitters to hit; the reason is not that the ball is
going faster, it is because it is going straighter. (All
this assumes that the outgoing and incoming velocities
are in exactly opposite directions to make my example a
one-dimensional problem.)
 QUESTION:
QUESTION:
I want to demonstrate that NaCl solutions conduct electricity to my students and show this qualitatively and quantitatively.
Is it possible to build a complete circuit as follows?
|--9 volt battery---NaCl solution---multimeter---LED/Daylight
bulb---|
|---------------------------------------------<-----------------------------|
 ANSWER:
ANSWER:
Assuming that your multimeter can measure current as well as voltage
this should work; if you are going to measure
conductivity you need the current as a function of the
concentration. Also, I would do the quantitative and qualitative experiments separately because LEDs are not ohmic
and as you change the current you would change the
voltage across the LED and therefore across the
solution; you want to keep the voltage across the
solution constant at 9 V when you are measuring the
conductivity. Also, the resistance of the filament of an
incandescent bulb changes as it heats up, so you should
only use that when you are doing the the qualitative
demonstration. Come to think of it, an incandescent bulb
would probably be better because not all LEDs are
dimmable so it might just turn off when you changed the
solution or not change at all.
 QUESTION:
QUESTION:
Jupiter's moon Io is volcanically active due to the moon being squeezed by the gravity of Jupiter and the interactions with the other moons. Io is hot, where is that energy coming from? There is not supposed to be a 'free lunch' regarding energy, there has to be a cost in energy somewhere else. So what is the supplying the energy in Io. Gravity isn't an energy source, so what is the source of energy for Io?
 ANSWER:
ANSWER:
I usually do not answer questions about
astronomy/astrophysics/cosmology, but will give this one a
try. Why do you say that gravity "…isn't an energy source…"? It is one of the most important sources of energy there is; a ball dropped increases its kinetic energy as it falls
because of the force of gravity doing work on it. In the case of Io, there is a large
tidal force
because of its gravitational interactions with Jupiter
and other moons which are constantly squeezing and
changing its shape. Imagine that you are squeezing and
relaxing a rubber ball; the energy you are putting in
will cause it to heat up. For a full-blown explanation,
see the Wikepedia article on
tidal heating.
 QUESTION:
QUESTION:
Why does certain object falls faster than others?
 ANSWER:
ANSWER:
If there is no air, all objects fall with
the same acceleration. However, if the objects are
falling in air, the air drag force affects some more
than others, and they fall with different accelerations.
 QUESTION:
QUESTION:
Why light speed is a universal constant if gravity can bend space-time?
 ANSWER:
ANSWER:
In everyday life speed and velocity are
synonymous. In physics velocity is a vector (specified
by a magnitude
and a
direction) whereas speed is the magnitude of the
velocity, a scalar. The speed of light is a universal
constant, the velocity need not be. So a beam of light
may be bent by gravity but still maintain a constant
speed. It is like driving on a curvey road at a constant
speed of 50 mph: your speed is a constant but your
velocity is not.

 QUESTION:
QUESTION:
I have a weird question I'd like to ask. I was in the bomber ride, which consists of a long arm with a gondola seating 4 people at each end. The arm revolves forwards for a few times then backwards for a few more times, and the gondola also rotates 360° mid air. The arm is 120 feet high and revolves at a speed of 13 rpm, in which the passenger experiences 3.5 Gs according to Billy Danter's fun fair website. I vomited a little while the gondola was at the very top and rotating, but I cannot remember if we were going forwards or backwards. I apologize for the gross details, but I'm curious to know where my vomit had landed, as it did not splatter on me not on the next seat passenger.
 ANSWER:
ANSWER:
 T
T
he
picture of the ride is shown on the left. It rotates (13
rpm) about an axis in the center. If you want to see a
video of this, click
here. If you are at one of the outer ends of this
thing, you will experience a "fictional" centrifugal
force which is 3.5 times larger than your weight (I
checked and this is correct). If you watch the video you
will see that the gondola itself is also free to rotate
about its own axis, but any centrifugal force due to
that rotation is trivially small compared to the main
centrifugal force and your own weight. The other picture
shows the gondola at one end rotated to some angle and
at the very top as specified by the questioner. The
questioner vomits (I will assume straight out) and the
vomit is labelled
V and has a velocity
in the direction of the yellow arrow. There are two
forces, the orange vectors, on the vomit, its weight
W down and the centrifugal
force 3.5
W up. The net force
on the vomit is therefore about 2.5 times its weight and
so it will appear to someone in the gondola someone in
the gondola to follow a path roughly like the
green-dashed line in the figure. So it is no surprise
that it did not get on anybody.
 QUESTION:
QUESTION:
I have recently gotten into synthesizer and how they affect soundwaves. Can soundwaves really be a Sawtooth and triangle's waves? The sine wave is the only wave that seems possible to me.
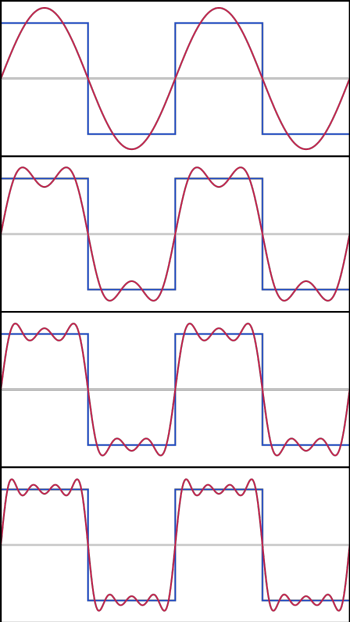
 ANSWER:
ANSWER:
I don't know on what you base your belief that only sine
waves are possible. In virtually all musical instruments
the sounds produced are not pure sine waves; only
electronic instruments are capable of creating simple
sine waves. Imagine two instruments, say a violin and a
piano, both play a middle C; do they sound just the same
to you? Of course not; this qualitative difference is
called timbre. The pitch of a tone is
determined by its periodicity, the frequency with which
the wave repeats its shape when passing; not only sine
waves but any shape you can imagine could repeat itself
over and over. Your brain hears the middle C as the same
note from all instruments but hears differences as well.
But, here is where you have gotten something right when
you think of sine waves as being somehow special.
Physicists and mathemeticians find sinesoidal waves to
be very easy to work because they satisfy a very simple
differential equation and because they form what is
called a complete set of functions. As a result, any
periodic function with frequency f may be described
perfectly by a sum of sine and cosine functions with frequencies
f,
2f, 3f, 4f…
This is called a Fourier series representation
of the wave. The sum is infinite, but normally only a
few members are needed to give a good representation of
the wave. The figure shows Fourier representations of a
square wave for 1, 2, 3, and 4 terms in the series.
 QUESTION:
QUESTION:
What causes one person to float while another person only sinks? Is it the molecular make up of the water, the human, or a combination of both? I am very buoyant, I lay on the surface of water, even with a weight belt is hard for me to stay under water. My GrandMother floats like a large rock, straight to the bottom, she can't even swim with a life vest on. Why is that?
 ANSWER:
ANSWER:
The answer is pretty simple: if the density of the body
(mass/volume) is less than the density of water (997
kg/m3) it will float, if more it will sink.
The body is made up mainly of bone (~1900 kg/m3),
fat (~914 kg/m3), and almost everything
else (~1040 kg/m3).
Approximately 14% of body mass is bone. So, only fat has
a density less than water meaning the more fat you
carry, the more buoyant you are likely to be. It is
difficult to try to get more quantitative because the
lungs occupy a not negligible fraction of the body
volume and air density is ~1 kg/m3. Almost
nobody with air in his/her lungs will sink like a stone.
Your grandmother probably has very low body fat.
 QUESTION:
QUESTION:
Thank you for taking the time to read my question. I am seeking an
explanation to my problem from some time now and I am very glad to have
found your site. I'm an olympic wrestling strength and conditioning couch
and I have my athletes practice an old isometric exercise called pushing the
wall, to develop whole body strength and power. As I have gone thorough this
exercise myself, after a year or so I have discovered that something
interesting is happening and this is what I'm seeking an explanation for.
As I push on the wall ( the push really comes from my back leg and the whole
body but without whole body muscular tension), with the same pressure,
without any other movement ( or an immovable surface) for let's say 30sec -1
min' when I slightly "push off" the wall ( I'm about 96 kg) and I mean
gently, not with force, I find myself been thrown backward for 10-15 meters
or more in some instances, by some kind of force..For the first few meters
the force is very strong that I can't stop the movement myself. The momentum
actually accelerates and it gets stronger as I keep been pushed backwards
and my body keeps going backwards, after a while the momentum comes to a
stop by itself, if I don't make an effort to stop it myself. Even if I put a
slight pressure on the wall and push off very gently, the same effect takes
place.
 REPLY:
REPLY:
I have spent some trying to understanding how this could be. I keep coming
back to your question but to no avail. If you still want me to try to figure
this out, I would appreciate it if you would send me a video you mentioned.

 FOLLOWUP #1:
FOLLOWUP #1:
Thank you so much for getting back to me. I really thought that my inquiry was weird enough for you to not consider it as true. For a while now I considered asking or talking with a physics professor about this and see if there is an explanation for this. You are the only person I thought to ask, and because I think this is a strange phenomena, I thought you might think it is fake and not bother taking it seriously. Because I think is indeed strange ( I call this force: "strange power"), I only think what other people might think about it and not even bother with it, as it seems that it is not a tangible physical thing or force, meaning that it seems that it isn't based on muscular force or physical exertion. As you see in the videos I really don't push hard at all.
 ANSWER:
ANSWER:
Let's look carefully at one of
the videos
you sent. I have inserted one frame showing you right
after you have lost contact with the post; your body is clearly tending
toward a sitting position. Your weight (one of the
forces on you) acts at your center of gravity, clearly
behind your feet. If you don't do something, you will
fall on your ass because your weight exerts an
unbalanced torque about your feet. To keep that from
happening, you push forward with your feet on the floor
and the floor pushes backwards on you; if the floor were
extremely slippery, e.g. like ice, there would
be nothing you could do to avoid going down. But, there
being friction, you now have an unbalanced force on you
which accelerates you backward. This, in itself, does
not keep you from falling but it gives you time to
straighten back up so that your weight no longer exerts
any torque about your feet and you can now stop without
fallin
g. There is no "strange power" force
here, just plain old Newtonian physics.
 FOLLOWUP #2:
FOLLOWUP #2:
Thank you for examining that frame from the video. But, what is that, that is strongly moving my weight and acting on my center of gravity ( as you see in that frame) ?
There is a strong power/force that is causing my position that you see in that frame, my center and body weight gets pushed backwards by that force and it keeps pushing me backwards while I step backwards trying to regain balance and stability. But, there is a strong force that strongly pushes me in that position, and keeps acting on me, when I only slightly push on the wall ( as you see in the videos). What is that force? and where is it coming from?..that is my question. What is that initial force or power that throws me backwards ( which is not the momentum caused by my center of gravity being behind my heals, there is a power that first pushes my weight and/or center of gravity in different positions thus creating a movement afterwards ). Take a look at my starting point. There are almost no external moving parts, that will logically/physically initiate a strong movement of my weight or center of gravity backwards and yet it happens.
And also how can the other things that you see in the other videos be explained.
 ANSWER:
ANSWER:
I will answer your last question first. You sent four
videos, each with 3 or 4 trials. In every trial, just
before you lost contact with your hand, you swung your
hips back so that your legs were angled backward so that
your center of gravity (somewhere inside your chest) was behind your feet. There
are no "…other
things that you see in the other videos…" which
need to be explained beyond this single frame and the
little backward scurrying that follows.
 I
thought that my answer to your first followup question
pretty clearly explained the whole thing without having
to get into the details of the forces and torques I was
talking about. But, it appears from your second question
that you are not familiar with how forces are dealt with
in physics. So I will give a little tutorial on the
important points necessary to understand your situation.
The most important thing to understand are Newton's
three laws:
I
thought that my answer to your first followup question
pretty clearly explained the whole thing without having
to get into the details of the forces and torques I was
talking about. But, it appears from your second question
that you are not familiar with how forces are dealt with
in physics. So I will give a little tutorial on the
important points necessary to understand your situation.
The most important thing to understand are Newton's
three laws:
-
If an object
is at rest or moving in a straight line with
constant speed, the sum of all forces on it
must add to zero.
-
If an object
with mass M has net force F not
equal to zero it will have an acceleration a=F/M
in the direction of the net force.
-
If an object A
experiences a force F from another object
B, object B experiences a force of equal magnitude
but in the opposite direction as F.
As an example, I will look at a case very similar to
yours, a sprinter. The figure shows all the forces
acting him, his weight W which is the force
which the earth exerts vertically down on him, the
normal force N which the ground exerts up on him, and
the frictional force f which the ground exerts
forward on him. Since he is not accelerating in the
vertical direction, only forward, N must be
equal in magnitude to W so that their sum is
zero. The net force at this instant is f, a
forward force which accelerates him in that direction.
Most people will say that it is the muscles in his leg
which propel him forward, but that is wrong; the muscles
in his leg result in the foot pushing backward on the
floor so, because of the third law, the floor pushes
forward on him. (Most people would say that an engine is
what drives a car forward, but it is actually friction
between the tires and ground which causes the car to
accelerate forward. You might be interested in an
earlier
answer.) There is still a subtlety regarding this
situation which is important: suppose this sprinter
encounters a patch of ice so that the friction
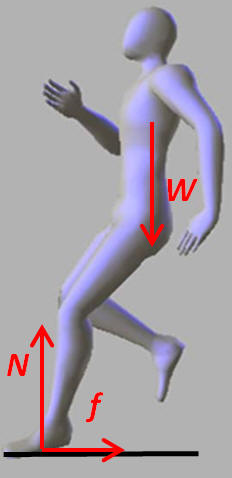 becomes
essentially zero. Now there is a net force on him which
is zero. Does he simply glide across the ice and then
resume running when he gets back to dry land? No,
because his weight will cause his whole body to rotate
about an axis passing through his feet and he will fall
forward until his other foot or his knee hits the
ground. (We say that W exerts a torque equal to W
times the distance horizontally between the axis and the
center of mass.)
becomes
essentially zero. Now there is a net force on him which
is zero. Does he simply glide across the ice and then
resume running when he gets back to dry land? No,
because his weight will cause his whole body to rotate
about an axis passing through his feet and he will fall
forward until his other foot or his knee hits the
ground. (We say that W exerts a torque equal to W
times the distance horizontally between the axis and the
center of mass.)
So now your case. The next figure shows you a short time
after you lost contact with the pole. You had to do
something or else you would rotate because of the torque
due to W and end up sitting on the ground*.
Your brain can detect this situation and lifts
your right leg and pushes on the floor with your left
foot, causing a frictional force ("strange power"?!)
pushing you backward and accelerating
 you.
You will still be starting to rotate but note that your
right foot will hit the floor less separated from the
weight so your tendency to fall will be lessened due to
the decreased torque. When that right food lands on the
floor the left foot lifts and the roles of the two feet
reverses. You keep backpedaling like this until,
finally, your center of gravity is directly above your
feet and you won't fall over.
you.
You will still be starting to rotate but note that your
right foot will hit the floor less separated from the
weight so your tendency to fall will be lessened due to
the decreased torque. When that right food lands on the
floor the left foot lifts and the roles of the two feet
reverses. You keep backpedaling like this until,
finally, your center of gravity is directly above your
feet and you won't fall over.
*In one of your trials you failed to be able to stop the
rotation and did, indeed, land on your ass. Notice your
arms and lifted leg desperately trying to move your
center of gravity farther relative to your right foot
but it did not work! The reason was apparently that you
dropped your hips too far when you launched.
If you just stood straight up with your center of
gravity vertically above your feet, you would not feel
the "strange power" when you lost contact with the pole. I know—I
tried it.
 FOLLOWUP #3:
FOLLOWUP #3:
I do understand what you are saying. Your last sentence was : "If you just stood straight up with your center of gravity vertically above your feet, you would not feel the "strange power" when you lost contact with the pole. I know—I tried it." Well, I tried it too, and again the "strange power" was pushing me off the wall..( strange power...is only a joke :)
The upper body gets pushed by the force first and the feet follow. It looks like the feet are trying to catch up with the momentum of the upper body, but it doesn't feel that way because the force keeps pushing the upper body backwards not because of the momentum of the upper body going backwards. Again, it is the force, a very tangible force, from the slight push off the wall that keeps sending the body backwards and that creates the acceleration. The acceleration of movement doesn't come from trying to stay on my feet or to regain stability after the push off the wall. I know it looks like that but the feeling is not at all like that. This is how it is felt. Also sometimes the force goes down into the feet, making them move backwards, thus moving the whole body backwards, Anyway, maybe it is difficult to understand. It is something that has to be felt, and I have tried to explain it but maybe it is not clear in how I say it. Maybe the only explanation using physics is the one you are giving me. ( I was thinking more in terms of conservation of mechanical energy, potential energy stored in the mechanical bonds between atoms, stored elastic potential energy that changes into kinetic energy when it is released )
Here is a video of it, me standing upright. If you are insisting on the explanation of Newtonian laws at work here, I understand and I thank you for your time.
 ANSWER:
ANSWER:
Here are the first few seconds of one push. Your feet stay put but your body leans. By the time of the fifth picture your body feels like it is falling and your brain perceives you are falling. Your brain interprets that something is pushing you and screams to your body that it has to act fast, keep one foot planted and get the other foot back to try to stop the fall. And so forth as described in my answer.
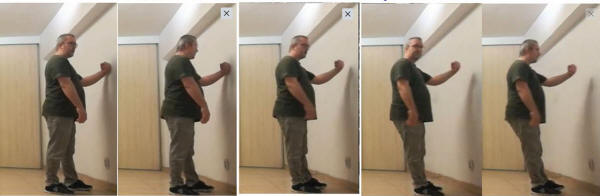
 FINAL
FOLLOWUP:
FINAL
FOLLOWUP:
Yeah, ok. I was sure you would say that. Your opinion makes sense when only watching, yet you seem to reject what I am trying to explain to you,
that what you see is not what is actually happening, ..but you can have your opinion and thank you for that.
It is great that you gave me your point of view, because now I know what other people might say and I know what to expect.
If you would feel what I am talking about you would not have the same opinion. But it is perfectly ok.
Thank you for your answer, thanks for trying.
 MY
COMMENT:
MY
COMMENT:
This is certainly not the first time I have had to end with "we'll just have to agree to disagree)!
 QUESTION:
QUESTION:
I built a spring loaded catapult which sent a practice golf ball a distance of 11.25 feet from a height of 2.66 ft at the horizontal (0 deggres) which I believe gives a velocity of 27.64 ft per sec, since free fall time is 0.407 sec. Then I launched the ball from a 28 deg angle and my assumption is that the initial velocity from any angle would now be 27.64 ft/sec, however the distance only came to 19.25 ft when I predicted it should go 23.8 ft. Working from the distance of 19.25 ft I get an initial velocity of 24.27 ft/sec. How come the initial velocity at another angle is different than the velocity when fired horizontally? I am doing this project in my high school Pre-Cal course of which I am the instructor.
 ANSWER:
ANSWER:
This will be a good lesson for your
students. You are assuming that the usual kinematic
equations for motion in a uniform gravitational field
are exactly correct. But they are only approximations.
In the real world there is air and air drag causes a
force opposite the velocity vector which is
approximately proportional to the square of the speed.
So the mathematics are going to well beyond high school.
Let's just consider the first case to discuss here since
I can make rough calculations to estimate the effect of
drag. I will not go into details, just give you the
results of my scribbling. First you got a velocity 27.64
ft/s by dividing 11.25/0.407; this is only the
horizontal component of the velocity because the ball
would also acquire a downward vertical component of
0.407x32 ft/s=13.02 ft/s. The velocity when it hits the
ground is √(13.02
2+27.64
2)=30.55
ft/s. But that is if you ignore air drag which will take
velocity away as it flies. My rough calculations are
that if the ball has a speed of about 30 ft/s the drag
will exert a force of about 0.013 lb. This will
cause an acceleration of about -4.3 ft/s
2
which would result in a loss of velocity over 0.407 s of
about -1.75 ft/s. (What makes the math difficult is
that, unlike uniform acceleration by gravity, the force
on the ball changes with time.)
The lesson your
students should learn is that it is important to ask
yourself how good a description any "theoretical"
model of a physical situation like projectile motion is.
Your results were pretty good, but you were dealing with
speeds of only about 20 mph; a driven golf ball can have
speeds up to about 200 mph so the drag force they
experience would like 100 times larger than at your
speeds (proportional to v2).
Predictions of the distance of a drive would not be close to how far the ball would actually go.
Another example is a baseball; anyone having played
outfield knows that a long fly ball ends falling almost
straight down, not at the same angle it was launched.
You can demonstrate this by throwing as hard as you can
a crumpled sheet of paper; it will end up falling almost
straight down having lost all its horizontal velocity.

