Ask the Physicist!

QUESTION:
What is the true shape of the Sun?

ANSWER:
I was surprised to learn that the sun is almost a perfect sphere.

QUESTION:
Why do the planets revolve only around the sun? There are stars which are bigger than the sun, and apply more gravitational force, but other planets do not attracts towards the stars. Please tell why?

ANSWER:
I guess you have not been following news articles over the past dozen years! In fact hundreds of exoplanets (planets revolving around stars other than the sun) have been observed.

QUESTION:
I have something I have been wondering about.maybe you can answer. I recently drank a glass bottle of rootbeer and then set the empty bottle on the hood of my car that was slanted slightly (1980's model). Within seconds the empty bottle started to vibrate slightly and then"walked" down the hood of my car. The engine was turned off. I was trying to tell my girlfriend that it was from heat convection, but she disagreed. How can this happen? I did it a second time and the empty bottle did it again. Not my imagination.

ANSWER:
Here is what I think: The bottle probably had condensation (water) which was fairly cool and the hood of your car was warm or even hot. The viscosity of water depends quite sensitively on its temperature. If the water is heated from 25⁰C to 50⁰C, for example, the viscosity decreases from about 890 to 547 μPa s which will result in a much slipperier contact. (Think of a very heavy oil and a very light oil to get an intuitive feeling for how viscosity would affect the frictional force. Or, think of the old expression " as slow as molasses in January ".)

QUESTION:
I don't know if you get this question alot, but a man standing on the ground is subjected to 2 forces. Gravity and normal reaction force. Do they form an action-reaction pair of forces?

ANSWER:
This is one of the most misunderstood aspects of Newton's laws. Let me state Newton's third law for your man in a couple of ways:

The earth exerts a downward force on the man (his weight) and therefore the man exerts an upward force on the earth of the same magnitude as his weight.
The ground exerts an upward force on the man (the normal force) and therefore the man exerts a downward force on the ground of the same magnitude as his weight.

Both of these are correct statements of Newton's third law. Notice that the pairs of forces ("action-reaction") are never on the same object. The two forces you give are both on the same object (the man) and can therefore not be an "action-reaction" pair. So why are they equal and opposite? Because Newton's first law states that if the man is in equilibrium the sum of all forces on him must be zero; therefore the ground must exert an upward force to balance the weight. Your example has nothing to do with Newton's third law.

QUESTION:
Why in the michelson and morley experiment that air does not have any effect on the experiment. To me air would be the current medium that the light is using to propagate and would yield a null result. The main key to this line of thought is that light travels slower through air then in a vacuum. The same as the speed of sound through different medium.

ANSWER:
The Michelson-Morley experiment does not attempt to measure the absolute speed of light. Rather, the idea is to find the difference in speeds between two different directions of travel. That measurement would not depend on the presence of air.

QUESTION:
Does a submarine have to work harder to travel at the same speed in deeper water?

ANSWER:
The drag force in water is approximately proportional to the speed; it also depends on the shape and on the viscosity of the water. As you go deeper into the water, the pressure increases and the temperature decreases. Viscosity only very weakly depends on pressure but increases significantly with temperature. After remaining constant around 20⁰C until a depth of about 200 m, temperature rapidly decreases to about 4⁰C at a depth of about 1000 m; the viscosity, and therefore the drag, nearly doubles at that depth.

QUESTION:
Do permanent magnets have an electric current surrounding them? Permanent magnets seems to have different properties to electromagnets, such that electromagnets can be used for induction and energy transfer if a conductor is placed within their changing magnetic field. So I understand that an electromagnet will have a changing magnetic field, which in turn generates a changing electrical current in a conductor placed within this magnetic field. My question is, do permamant magnets generate this same electric current if a conductor is placed within their magnetic field? I.e. if I had a strong permanent magnet, would I be able to generate a current in a coil if it was brought into the permananet magnet's magnetic field?

ANSWER:
A permanent magnet may be thought of as having "bound" currents. These are not currents you could actually connect an ammeter to and measure. If you are interested, there are, in general, two types of bound current, bound volume current density J_b and bound surface current density K_b. If the magnetization of the material is M, you can write J_b=curlM and K_b=Mxn where n is a unit vector normal to the surface and pointing out of the volume. I do not understand any of your rambling about induction. I will simply say that any changing magnetic field can induce currents and a permanent magnet is a source of a magnetic field; moving that magnet, through a wire ring, for example, will induce a current in the ring.

QUESTION:
Can the equations for Compton Scattering be applied to a photon colliding with a neutron? If no, what equations can be used?

ANSWER:
Yes, you just have to replace the electron mass with the neutron mass. However, the effect for this much larger mass would be very difficult to see because the change in wavelength will be much smaller than for electrons. To have any hope of observing the effect you would need to use extremely high-energy gamma rays.

QUESTION:
If matter above absolute zero emits thermal radiation and thermal radiation is actually electromagnetic radiation what temperature is required for matter to emit visible light?

ANSWER:
Technically, visible light is emitted by all objects because the spectrum of emitted radiation is continuous and includes all wavelengths. However, you can say at what temperature the visible part of the spectrum is more intense than any other emitted radiation, about 5000 K. Interestingly, that is about the temperature of the surface of the sun.

QUESTION:
if an object ( like a bullet ) is fired vertically at constant speed. Where does it land?

ANSWER:
This is a standard intermediate-level classical mechanics problem for accelerated reference frames. All the mathematical background is much too complicated to include here but the answer is that it lands a distance d=4ωv₀³cosλ/(3g²) west of where it was shot; here ω=7.27x10^-5 s^-1 is the angular velocity of the earth, v₀ is the initial velocity of the bullet, λ is the latitude where the gun is located, and g=9.8 m/s² is the acceleration due to gravity. This, of course, neglects any air drag or wind corrections. For example, for λ=45⁰ and v₀=390 m/s (typical 9 mm muzzle speed), d≈60 m. At the poles (λ=90⁰), it goes up and comes back down perfectly vertically.

QUESTION:
what is the cause of buoyancy.? we faced many confusions about then we worked on it and did some experiments and found an another reason which is satisfying all the conditions and aspects...but we wanted to consult about it......

ANSWER:
The reason for buoyancy is simply that the force due to the fluid up on the bottom of something is greater than the force of the fluid down on the top because of the pressure difference.

QUESTION:
Why does stirring a liquid makes it cool faster? Shouldn't the reverse happen, as the kinetic energy imparted to liquid in stirring should also change eventually to heat?

ANSWER:
The liquid in the cup loses energy to its environment (cools) by conduction and radiation from its surfaces. The energy inside the surfaces is transmitted to the surfaces (to continue cooling) by convection. Convection is a relatively slow process for transmitting heat. Stirring more effectively moves hot liquid to the surface. Energy you add by stirring is trivially small compared to the increased cooling rate.

QUESTION:
Suppose light is travelling in a straight line parallel to Y-axis takes time t to reach from y1 to y2 in a frame of reference S. Let there be another frame S' which is travelling parallel to X-axis with velocity v relative to frame S. Then a seen from S' the light ( which was travelling parallel to Y-axis in frame S ) now takes a longer path which is greater than y2-y1 (as now it will be travelling obliquely in frame S' ). Now due to time dilation time taken in frame S' is lower than t as seen by frame S due to time dilation. Then speed of light in frame S' as seen from S will be greater than c as length is higher and time is lower. then how to explain the constancy of speed of light in this case ?

ANSWER:
You are making this too hard! The fact that c is a universal constant is where you should start, not end. When you move with your S' frame with speed v in the positive x direction, the light beam acquires an x-component of c_x=-v. If the speed of the light is to remain constant, this must mean that the y-component must therefore decrease such that c²=c_x²+c_y². So, c_y=c√[1-(v²/c²)] which means that the angle θ which the light makes with the y-axis is θ=tan^-1[β/√(1-β²)] where β=v/c. See an earlier question for an example of this phenomenon.

QUESTION:
If I have two hoses a varying diameters such as a garden hose and a firehose that are vertical and approximately 20 feet long that are filled with the same amount of water and have the same size opening at the bottom, using just gravity, will water flow through each hose in the same amount of time? This question came up during a recent visit with a customer. I sell feeding supplies for neonatal patients. The current product being used is a large bore tube compared to the smaller bore that my company sells. However the opening at the distal tip of the feeding tube is the same size for both. Again, using just gravity, shouldn't the speed at which formula flows through the distal end be the same since it bottlenecks there?

ANSWER:
Your first question (" will water flow through each hose in the same amount of time?") is ambiguous, so let me answer the question by finding how the speed of the delivered formula (labelled V_bottom in the figure) depends on the geometry. The operative physics principal is Bernoulli's equation, P+ ρV²+ρgy=constant where P is pressure, ρ is fluid density, y is the height above some chosen reference level, and g=9.8 m/s² is the acceleration due to gravity. In your case, P is atmospheric pressure both at the top and at the bottom, I will choose y=0 at the bottom so y=h at the top. Therefore Bernoulli's equation becomes V_top²+2gh=V_bottom² or V_bottom=√(2gh+V_top²). There are two ways that V_bottom will be independent of the geometry: (1) if h is held constant by replenishing the formula at the top or (2) if the area of the bore is much larger than the area of the distal tip A>>a. Both of these result in V_top≈0 so V_bottom≈√(2gh). If neither of these is true, it is a much more complicated problem.

QUESTION:
What is the true shape of the earth?

ANSWER:
There is no "true shape" of the earth because the earth is geologically active, constantly changing due to volcanism and plate tectonics. To a very good approximation, though, the earth is a sphere. Closer inspection shows that the earth is slightly oblate, that is, it is slightly pushed out at the equator and scrunched in at the poles; still, it is small, the equatorial diameter being about 42 km larger than the polar diameter. This is referred to mathematically as a negative quadrupole moment, an oblate spheroid of revolution. I remember learning back in the early 60s when satellites were first being launched, that their orbits also indicated an octupole moment of the earth, sort of pear-shaped with the fatter part of the pear in the southern hemisphere. I believe, though that there is still a lot of controversy about such higher moments because similar effects on orbits can be caused by uneven mass distribution of the earth which certainly does occur. You can read more detail in Wikepedia.

QUESTION:
As we know everthing thing is made up of atoms which consist of electrons,neutrons and proton. So we can say blackboard in our classroom is made up of atoms which further consist electron revolving around its nucleus.So if we tap on the blackboard with our hand ,what will happen?Does the electron of the blackboard fell down or electrons from our hand gets transferred into the blackboard or what?

ANSWER:
Basically, the electrons on the surface of your hand and the electrons on the surface of the blackboard repel each other because of the Coulomb force. This repulsive force is the force you feel when you touch the blackboard and the reason your hand does not pass into the wall. Electrons can get transferred between two objects which come in contact, usually by rubbing more than just touching; this is the source of "static electricity shock" when, for example, you walk across a carpet in dry weather.

QUESTION:
I sent you $20 before I even asked the question. This should tell you that I am very interested in the answer. I am way passed school age. This is just for me.

This is a velocity question: The projectile is 25 inches long, 1/2 inch in diameter and weighs 1750 grains. Absolutely the most aerodynamic it can be. It will have a guidance system that will include a very small fin in the front and back. I know this is going to mess up the aerodynamics a bit but I am looking for an answer as close as you can approximate.

How high would this "Arrow" need to be dropped in order to reach terminal velocity and just how fast is terminal velocity? What if the projectile weighed 3500 grains, would this change the velocity or would that only change the kinetic energy on impact? I know barometric pressure, humidity, crosswinds, temperature and the like will affect the speed. Just use an average day...which there aren't any...but something like neutral.

As I read that over, I realized I am asking what the fastest terminal velocity could be of the most aerodynamic object ever in free fall.

ANSWER:
It is ill-advised to make a donation before receiving an answer because if a question makes no sense, I cannot answer it and have no means of returning your money. In your case, there are some misconceptions and you need to understand that the key word in your question is "approximate". All air-drag questions can only have approximate solutions. Your main misconception is that there is no such thing as "the most aerodynamic object ever". A second misconception is that you will ever reach the terminal velocity; the analytical solution to the problem has the terminal velocity reached only after infinite time and I will use 99% of the terminal velocity as having reached full speed. Since I have no information at all about the detailed shape of the object, I will simply use a generic form for the air drag F which can be assumed to be well approximated as a quadratic function of velocity for this case, F=-cv² where c may be approximated as c≈¼A, A being the cross sectional area presented to the wind (the negative sign indicating that F is opposite v). This approximate value of c is valid only if SI units (meters, seconds, kilograms) are used.

Now, the solutions. I will not give full details since they are fairly mathematical. Convert, first, to SI units: L=25"= 0.635 m, R=0.25"=0.00635 m, m₁=1750 grain=0.1134 kg, m₂=3500 grain=0.2268 kg, A=πR²=1.267x10^-4 m², F=(3.167x10^-5 N)v². To calculate the terminal velocity v_t, find when the drag force is equal to the weight mg (g=9.8 m/s²): mg=cv_t², v_t=√(mg/c)=√(4mg/A); I find v_t1≈187 m/s and v_t2≈265 m/s. The height h from which you would have to drop these to achieve this speed with no air drag is given by h=v_t²/(2g), so h₁^{no
drag}=1780 m and h₂^{no drag}=3560 m. Now, if air drag is included in the calculations, it can be shown (see any intermediate-level classical mechanics text) that v/v_t=√[1-exp(-2gh/v_t²)]. Now, setting v/v_t=0.99 and solving for h, I find h₁=6989 m and h₂=14,034 m. I have shown a graph with the velocity relative to the terminal velocity v/v_t as a function of the height h from which it was dropped. Keep in mind that these calculations are approximations as explained above; I have tried to give enough details so that you could vary the parameters if you like, in particular the drag coefficient c and the ratio of v/v_t.

ADDED COMMENTS:
I came across a more detailed discussion of the drag forces for cylinders if you are interested. You will be referring to the picture to the left above for the following discussion. First, I need to discuss the definition of c above more carefully. In more detail, c= ρAC_d where ρ is the density of air which is about 1.2 kg/m³ at standard pressure and temperature and C_d is a number (called the drag coefficient) which depends only on the geometry. Note that for my calculations above the drag coefficient was C_d= A/( x1.2xA)=0.6. The graphs above show that the drag coefficient depends on the ratio of L/d=L/(2R) which has a value of 50 for your projectile. So, if your cylinder has a blunt end, C_d≈0.81 (c≈0.6xAx0.81=0.49 A≈ A) and, for a rounded end, C_d≈0.4 (c≈0.6xAx0.4=0.24 A≈ A). So, the calculation above is likely a good approximation to your " most aerodynamic object " My whole treatment of this problem, though, assumes that c is a constant. However, as you can see above, c depends on the air density and the air density depends on altitude. Surely at values of h around 7 km or 14 km, the air density is much smaller than at sea level and therefore c is much smaller and the terminal velocity is much larger; this makes the problem considerably more difficult requiring numerical solutions, beyond the scope of this web site.

QUESTION:
Why is the direction of magnetic field lines outside the magnet from north to South and not South to North? Is it a convention or has got a logic behind it?

ANSWER:
It is just as arbitrary as whether a electrons are negative or positive or whether an electric field points toward or away from a negative charge. If you chose the different direction from conventional, you would have to use a left hand rule to determine the force on a positive charge moving through a magnetic field.

QUESTION:
so lets say i launch a huge rocket..it leaves the atmosphere and reaches its maximum velocity...and then inside that rocket lets say i had another slightly smaller rocket built...it would travel with the velocity of the larger rocket plus the velocity of the slightly smaller one right?? ..then lets say i keep doing this for a while...can't i reach the speed of light or something like this?

ANSWER:
You can never use velocity addition to exceed the speed of light. See the FAQ page.

QUESTION:
I have a question regarding radiation detector. I understand that we have a scintillator (NaI for example) and a photomultiplier tube. Let's say we have an incident gamma ray that produce a photo-electron (forget about x-rays produced by the electrons reconfiguration) in a NaI crystal. How that photo-electron turns again into a photon so it can strike the photomultiplier.

ANSWER:
If γ-rays are being detected, the photoelectron plows through the NaI crystal, ionizing and exciting atoms as it goes. Ideally it gives up all its energy before escaping the crystal. There are now a very large number of photons in visible or IR or UV the number of which is proportional to the energy of the electron which is equal to the energy of the incident γ-ray. Because of reflective coatings on the surface of the crystal, many of these photons reach the photomultiplier tube as a pulse of photons and strike the photocathode which again cause the electron production via the photoelectric effect; because low-energy photons do not cause photoelectric effect, mainly the UV photons participate here. These electrons are then multiplied by the many dynodes in the tube resulting in a current pulse proportional the energy of the original γ-ray. If charged particles are being detected, the charged particle itself plays the role of the initial photoelectron, ionizing and exciting atoms in the crystal.

QUESTION:
So if two cars were to hit each other head on, what would be the best way to minimize danger? Would you slow to a stop, go faster, or match the other cars speed? My sister says that you would match the speed, because if you stopped you would absorb all of the impact. My dad says you would stop because then the only force being put out would be the other car.

ANSWER:
What hurts you is the force you feel. What determines the force you feel is your acceleration, your rate of change of velocity during the collision. To minimize acceleration, you should minimize the relative velocity between you and the other car which means stopping would be best, but certainly any braking before the collision will help. This assumes that the duration of the actual collision does not depend on the relative speed which is probably roughly true.

QUESTION:
The planets of our solar system orbit the sun in a gravity well resulting from the mass of the Sun (per Einstein). The Sun looses millions of tons of mass each second to fusion. One would expect there to be at least a tiny widening of planetary orbits as the mass of the sun decreases, and the gravity well decreases with it. Yet there seems to be no observable evidence of the planets moving away from the Sun? It seems to me that there should be some observable changes, even it they are very, very tiny. Why is this not true?

ANSWER:
The sun has a mass of about 2x10³⁰ kg. It loses about 6x10⁹ kg/second≈2x10¹⁷ kg/year. So each year the sun loses about 10^-11 % of its mass. This turns out to result in an increase in the earth's orbital radius of about 1.5 cm/year. Accurate measurements of the earth's radius have been made only for about 500 years over which time the earth's orbit would have increased only by about 7.5 m, certainly not detectable.

QUESTION:
Why isn't nitrogen forced upwards by the much heavier oxygen and co2?

ANSWER:
The details are very complicated. I found an article where you can read about it. The key finding of interest to you is in the abstract of this article: "Turbulent mixing keeps the relative concentrations of gases nearly constant in the lowest 100 km. At higher altitudes, molecular diffusion controls the concentrations, with the lighter gases becoming relatively more abundant with increasing altitude."

QUESTION:
I know how a sonic boom works. My question. If a plane is traveling lets say 2 x the speed of sound and people are talking inside the plane, do their voices technically travel forward at 3 x the speed of sound? I know if they were in an open cabin the person in front would not hear the person from behind. So in a closed cabin this effect is negated. I just can't figure out if the sound in the cabin would move forward at The speed of the plane + speed of sound.

ANSWER:
I answer this kind of question so often! In order to specify the speed of something, you must specify what the speed is relative to. The speed relative to the air inside the cabin is the speed of sound, the speed of the sound relative to the ground is three times the speed of sound.

QUESTION:
I m a middle school teacher and I had a bright student show to me the following: If the mean radius of the earth is 3959 miles (taken from space.com) and we assume the earth to be spherical, then we could use the Pythagorean thereom to show the difference between the hypotenuse from the radius over 10 miles to be 66.883 feet. We get after squaring the 3959 and adding 100 for 10 squared, and finding the square root, and finding the difference between the hypotenuse and the radius = 66.883 . The National Geodetic Survey shows only 7.874 inches of variation across the Bonneville Salt Flats. In other words the 10 mile stretch of the Bonneville Speed Track should dip down below the horizon from the start 66+ because of the curvature of the earth, but in fact, does not. I d appreciate any help you can give me to explain to the student why the curvature of the earth does not seem to show itself on the salt flats.

ANSWER:
I am sorry, but I do not understand your calculation. I cannot figure out what you mean by hypotenuse. Let me give you a calculation where I will define clearly what I mean by the amount of variance over 10 miles. Maybe it is not what you want, but at least there is a picture where you can tell me where your hypotenuse is. In my drawing on the left there is an arc of length s (10 miles in your case) which subtends an angle θ from the earth's center. The arc is a distance d above the corresponding chord at its center (the 5 mile mark in your case). The distance along the radius bisecting the angle to the chord is Rcos(θ/2). Noting that Rcos(θ/2)+d=R, you can solve for d=R(1-cos(θ/2)). The angle in radians is θ=s/R=10/3959=0.002526=0.145⁰. It turns out that d=16.67'.

Now I see what you did. The figure on the right adds a tangent. Now (the newly defined) d is given by d=R[(1/cosθ)-1]=66.68' essentially your answer (I think you must have misread your calculator since I get my answer if I do it your way.)

Now, what does it mean? If you had a vertical tower at the end of the track and aimed a transit on the ground horizontally you would see about 67' up on the tower if the earth were a perfectly uniform sphere. But, on a scale as small as 10 miles, there is no reason to expect the terrain to be perfectly spherical, certainly it is not. What if the surface were truly flat? My first calculation shows that the center would only be about 17' lower than the ends, a very small variation over 10 miles (52,800'). I do not know what the 7.874" number means, but it could be a mean variation from truly flat.

QUESTION:
I don t understand how a (passive) megaphone or a tuba can work without violating the law of the conservation of energy. Naively, it would seem that you are getting work for free, since a small sound energy input can aided by an unpowered device somehow yields an amplified noise.

ANSWER:
See an earlier answer.

QUESTION:
I am hoping you can help me solve a question. I am trying to figure out how much a given volume of air expands per degree increase in temperature. (volumetric thermal expansion)I have looked online but I seem to be finding several equations but the formulas seem to have unclear factors and definitions. Here is the exact variables in this problem: If I had 1 cubic inch of air @ 80 degrees Fahrenheit (standard atmospheric pressure and standard air like what we breath)and heated it to 350 degrees Fahrenheit, how much would the volume increase?

ANSWER:
The ideal gas law is what you need, PV/(NT)=constant where P is pressure, V is volume, T is temperature, and N is some measure of the amount of gas. For your case, N and P do not change, so V/T is unchanged as you heat the air, so V₁/T₁=V₂/T₂. But, here is the catch the temperature must be expressed in kelvins, absolute temperature, ⁰F will give a wrong answer. Converting, T₁=80⁰F=300 K and T₂=350⁰F=450 K. Solving, V₂=1.5 in³. These numbers come out so neatly that I now feel that I have been duped into doing your homework. Please do not do that again.

QUESTION:
According to general relativity, our time is relative to our velocity, but is our velocity relative to our galaxy, the universe, or what?

ANSWER:
It is special relativity, not general relativity, in which the time is dependent on the velocity of the clock. I think you misunderstand, though. The time which you call "our time", the rate at which a clock in our frame of reference runs, will be perfectly normal to you. A clock which moves relative to your frame will run more slowly in your frame than it does in its own frame. When you ask about whether time is relative to the "galaxy, the universe, or what?" you are implying that there is one universal clock which is "correct" and this is wrong; there is no universally correct time, time is relative to who is measuring it.

QUESTION:
What is between the electron and proton in the hydrogen atom.

ANSWER:
This question assumes that the electron is in a particular location, presumably some orbit around the nucleus. In fact, the Bohr model of an atom is not correct and a proper solution of the problem has the electron wave function as a solution of the Schr dinger equation. Doing this, it is found that the electron forms a cloud around the nucleus and the electron is everywhere in the atom.

QUESTION:
I am interested in the effects of gravity on objects of the same mass in different configurations. Example: Three long objects of the same mass, for the sake of example, three 6 foot 2x4 boards. If I take two of the boards and lay them parallel, the gravitational attraction between them should be equal at all points. However, if I arrange the three boards in a U shape, does the additional mass connecting the bottom of the two boards at the U cause the attraction to increase by only the amount of the additional mass (the third board), in other words, does the base of the U and the tips of the U retain the same gravitational attraction with regard to each other? Or does the addition of the connecting mass at the base of the U change the attraction of the parallel boards and if so is it in decreasing effect along the parallel boards from the connected base to the tips of the U?

ANSWER:
First of all, it is not really clear what you mean when you say " the gravitational attraction between them should be equal at all points " The force felt by one little piece of mass on one board due to the other board should be the same magnitude as the other board experiences; their directions, however, will not be opposite each other except at the centers. If we approximate your boards as thin sticks (pretty good since 2"<<72" and 4"<<72"), it is fairly easy to calculate the force experienced by a very small piece of mass m in either board due to presence of the other board for your parallel configuration; it is a little messy, so I will not write it here. This force will have both an x-component and a y-component. The graph to the left shows F_x and F_y as well as the magnitude of the force, F=√(F_x²+F_y²) for sticks of mass 1 kg, length 1 m, and separated by 1 m to illustrate; I have chosen x=0 at the left end of the stick and x=1 at the right end. I have normalized the forces to the gravitational constant G. On the right you can see the forces on each stick at the ends and centers (red shows the components). The addition of other masses, like your third board, will not alter the forces between these two sticks but will certainly alter the force felt by any mass on these sticks; I guess the answer to your question is that the force which one board exerts on another is not changed by adding more boards. Still, we are normally interested in the net gravitational force on something, not just the force which one other object of many exerts on it. (Incidentally, note that m will also experience a force due to the rest of its own board.) You might be interested in an earlier answer concerning gravitational fields of cylinders.

QUESTION:
What are leptons?

ANSWER:
In the standard model, the fundamental particles fall into two groups, fermions (spin ) and bosons (spin 1). The fermions are divided into two categories, quarks and leptons. There are six quarks (purple in the figure) (u, d, c, s, t, b), the components of hadrons which include neutrons and protons. All quarks have electric charge 1/3 or 2/3 so they feel the electromagnetic force. They also feel the strong force. They also feel the weak force since we know that β-decay changes a neutron/proton to a proton/neutron via the weak interaction. All are fermions. There are six leptons (green in the figure) (electrons (e), muons (μ), and taus (τ), and three associated neutrinos). All feel the weak force. The neutrinos have no electric charge, so they do not feel the electromagnetic force; the charged leptons, of course, do. For each force there is a field quantum , that particle which communicates the force between particles which feel that force (the pink in figure 4.8 γ, g, Z⁰, W); note that these are all bosons since they have spin 1. For the electromagnetic force, it is the photon. For the strong force, it is the gluon. For the weak force, it is the Z and the W bosons. Note that only the Z has electric charge. The electromagnetic and weak forces have been unified in theory, sometimes collectively called the electroweak force.

QUESTION:
If we have an object x moving "forward" and a person A also moving "forward" can the person A be moving so fast that the object x appears, to him, to be moving backwards? (If I'm correct it can appear to be standing still).

ANSWER:
There are two possible scenarios. Call V_A the speed of A and V_x the speed of x.

A is in front of

A is in front of x

If V_A=V_x, x will appear to be at rest relative to A;
if V_A>V_x, x will appear to move backwards relative to A;
if V_A<V_x, x will appear to move forward relative to A;

A is behind x

If V_A=V_x, x will appear to be at rest relative to A;
if V_A>V_x, x will appear to move backwards relative to A;
if V_A<V_x, x will appear to move forward relative to A;

So, you see, all that matters are the relative speeds, which is ahead does not matter.

QUESTION:
what cause planets to move on their axis

ANSWER:
If something is rotating on an axis, it will continue rotating forever until something exerts a torque to change the rotation. The planets had (just by random accident) angular momentum (spinning) when they formed, so they always will.

QUESTION:
what are electrons,neutrons and protons made up of

ANSWER:
Electrons are believed to be elementary, they have no substructure. Protons and neutrons are made up of quarks.

QUESTION:
What are dark matter and dark energy?

ANSWER:
See the FAQ page.

QUESTION:
Is tension a scalor or a vector qunatity? While considering a rope why do we take into account its magnitude and not direction? Plz clarify.

ANSWER:
Tension is the force which a rope or string exerts. Since it is a force, it is a vector. Probably the reason you are confused is that, unlike most unknown forces, you always know the direction of the tension force: it is always in the same direction as the string and always pulls, never pushes. So, when you solve a problem involving tension, the only unknown thing about a tension force is its magnitude which is a scalar. So it might seem like you are treating tension like a scalar, but you are not.

QUESTION:
This is a classic point of contention for pilots, and has recently come up again. I'd like to know your take on this. It seems more often than not, that the answer is the plane will take off. However, no one from that side of the argument addresses the force of the conveyer in the opposite direction canceling forward force from the engine thrust. I don't see how the aircraft could accelerate from a starting velocity of 0kts. This question has grown men lobbing profanity-laced insults back and forth. The answers are generally not backed by aerodynamics or physics equations. I am graduating from Embry-Riddle in August with a major in Aeronautics. I'm well past my aerodynamics class, so this is certainly not homework for me, but it is most interesting nonetheless. Without further delay, here is the question: Imagine a 747 is sitting on a conveyor belt, as wide and long as a runway. The conveyor belt is designed to exactly match the speed of the wheels, moving in the opposite direction. Can the plane take off?

ANSWER:
My goodness, this is pretty basic physics and should not be a puzzle at a school which has aviation as its main focus. In order for an airplane to fly, lift must be generated. During takeoff, lift is almost entirely the result of air passing over the wings and if the airplane is not moving relative to the air, there is no lift. The engines, if you could point them vertically, could provide lift and cause the airplane to "take off" if their thrust were greater than the weight of the airplane. I looked up the maximum thrust for a 747 and it is only a little greater than ½ the weight. This is no different than an airplane revving up its engines with the brakes locked. It will certainly not be able to take off. Vertical takeoff planes like the Harrier (pictured above) can do this, not conventional ones. A final thought: if you had your 747 on the conveyer belt pointed into a very high wind, it could take off, but since the takeoff speed of a 747 is around 180 mph, there are no such winds even in a hurricane.

QUESTION:
Which main part of the electromagnetic spectrum emitted from the sun, is reflected by mirrors on to a water boiler. For generating renewable energy?

ANSWER:
It depends on two things, the spectrum emitted by the sun and the reflectivity of the mirror itself. The sun has the most energy emitted at wavelengths in the visible region but a significant amount is also in the infrared region. If the reflectivity is fairly flat over this region for the metal you choose as a mirror, the spectrum reflected will be similar to the spectrum of the sun. Both aluminum and silver are at about 90% reflectivity over th range of most of the spectrum and therefore the energy reflected will be very similar to the energy which strikes the mirror. The other thing to consider is that whatever absorbs the reflected radiation should absorb as much of the incident radiation on it as possible, i.e. be "black".

QUESTION:
If im holding a box connected to a non stretching rope to my hand and i start accelerating upwards ( gravity pulls down ) How would i describe a tension in a string if my acceleration is increasing with time ( defined by some function ). This is not homework i swear. I just cant seem to equate all this.

ANSWER:
If the mass of the box is M and its acceleration is a, then T-Mg=Ma (Newton's second law) so T=M(g+a).

QUESTION:
If a circular disk has one half of heavier metal and other one of heavier metal. Would the centre of gravity and centre of mass different? Gravity is uniform. And in uniform gravity , does same mass bodies have their c.g and c.o.m equal?

ANSWER:
The center of mass and center of gravity are coincident if the gravitational field is uniform (the same everywhere across the object). The center of mass of a uniform half disk of radius R is a distance d=4R/(3π) from the center of the straight edge diameter. If the mass density is ρ and the thickness of the disk is t, the mass is M=ρtπR²/2. So you can treat the two halves as two point masses M₁ and M₂separated by a distance 2d. So, the distance from the center of the disk to the center of mass is (dM₁-dM₂)/(M₁+M₂)=[(dρ₁tπR²/2)+(dρ₂tπR²/2)]/[(ρ₁tπR²/2)+(ρ₂tπR²/2)]=d(ρ₁-ρ₂)/(ρ₁+ρ₂); this will always be on the more massive side. For example, if ρ₁=2ρ₂, the center of mass is a distance d/3=4R/(9π)≈0.14R from the center.

QUESTION:
I have seen in your site these questions about guns portrayed in science fiction and you have done calculations for the recoil of these things. So I have a question on how do I calculate the Gs that the recoil causes? Let's use an example. If I have a ship with a mass of three million tons and it has a six hundred meter long mass accelerator gun that fires a twenty kilogram projectile at 2000km/s, how much recoil do I get from that?

ANSWER:
The speed, 2x10⁶ m/s, is very small compared to c=3x10⁸ m/s, so we can use classical mechanics and galilean kinematics. Assuming that the projectile accelerates uniformly, 600= at² and 2x10⁶=at; solving, a=3.3x10⁹ m/s² and t=6x10^-4 s. The force on the projectile during the 6x10^-4 seconds is F=20x3.3x10⁹=6.6x10¹⁰ N. Newton's third law says that the ship (mass 3x10⁶ metric tons=3x10⁹ kg) will experience an equal and opposite force during this time, so a_ship=6.6x10¹⁰/3x10⁹=22 m/s²=2.2g. The speed acquired by the ship is (20/3x10⁹)x(2x10⁶)=1.3 cm/s.

QUESTION:
if black hole has enormous gravitational pull, can it attract light?? if yes, does this prove photon has a dyanamic mass as gravity attracts the mass only??

ANSWER:
Any gravitational field will "attract" a photon, it does not have to be a black hole. For example, a photon passing by a large mass (like the sun or a galaxy) will be deflected. What is special about a black hole is that if a photon gets closer than a critical distance called the Schwartzchild radius, the light cannot escape. However, you are wrong when you say that gravity only attracts mass. A photon has no mass. See the faq page.

QUESTION:
A train is traveling northwards and i am within the train walking southwards, what direction am I traveling?

ANSWER:
I always have to emphasize, you must specify the velocity relative to something else. Your velocity could be either your velocity relative to the train or relative to the ground; if you do not specify which, your question is meaningless. If your speed is smaller than the train's speed, your velocity relative to the ground is north and your velocity relative to the train is south. If your speed is larger than the train's speed, your velocity relative to the ground is south and your velocity relative to the train is south. If your speed is equal to the train's speed, your velocity relative to the ground is zero and your velocity relative to the train is south.

QUESTION:
Is there an equation that tells the period of rotation of pendulum with two masses placed at different distances from point of pivit?

ANSWER:
You must mean period of oscillation, not of rotation. The period of any pendulum can only be approximated for small angle oscillations. Your problem would have M₁ a distance L₁ from the pivot and M₂ a distance L₂ from the pivot. The moment of inertia of the pendulum is I=M₁L₁²+M₂L₂². It is a standard introductory calculation to show that the period T of a pendulum is T=2π√[I/(MgL)] where M is the total mass and L is the distance from the pivot of the center of mass. In your case, M=(M₁+M₂) and L=(M₁L₁+M₂L₂)/(M₁+M₂). Putting it all together, T=2π√[(M₁L₁²+M₂L₂²)/(g(M₁L₁+M₂L₂))].

QUESTION:
How much does density play part in gravitational strength? Like if we had two planets of equal masses but the other is denser and therefore smaller in diameter and the other is less dense but larger in diameter. Would we have equal gravity on these planets?

ANSWER:
What matters on the surface of the planet are the total mass and the radius of the planet; the gravitational field on the surface of the planet is proportional to its mass M and inversely proportional to the square of its radius R: M/R². So, the denser planet in your example will have a larger gravitational field at the surface. But it has nothing to do with the density, it is due to the smaller radius.

QUESTION:
It has occurred to me that the force of gravity is not uniform but rather is a function of the mass upon which it acts -- say two spherical objects of the same diameter, but of differing masses are suspended at the same height (in a vacuum enclosure) and then both are dropped at the same instant, both objects would then strike the ground at the same time.. This would mean that the force of gravity exerted on the sphere of lesser mass would have to be greater than the gravitational force acting upon the sphere of greater mass... Is this accurate??

ANSWER:
How did you come that conclusion? The force on the smaller mass is smaller, and the amount is proportional to the ratio of the two masses. For example, if the mass of one is twice that of the other, the force on it will be twice as great. Newton's second law then shows that the two will have identical accelerations. See the FAQ page.

QUESTION:
Why does light not bend through glass from air if the angle of incidence is at 90degrees to the boundary?

ANSWER:
I can think of several reasons:

Snell's law requires it: n₁sinθ₁=n₂sinθ₂ implies that if θ₁=0 then θ₂=0.

If θ₁≠0, the ray defines a unique plane which is normal to the surface on which the refracted ray would travel. If θ₁=0 and θ₂≠0, how would you know which direction the refracted wave travels?

Huygen's principle would have plane waves striking parallel to the surface generating plane waves also parallel to the surface inside the second medium.

QUESTION:
I am really curious and I would really appreciate if you take some time to answer some questions for me! Where does all this rotation come from? Stars can rotate at incredible speeds, planets rotate, satellites rotate, etc. Why do certain bodies rotate at significantly higher speeds than others? Pulsars can have rotational periods in the milliseconds.

ANSWER:
I state clearly on the site that I do not do astronomy/astrophysics/cosmology. However, I have earlier answered a question about rotations in the solar system, so you can have a look at that. For any individual body, the rotation rate is normally determined by how much smaller or larger it has become over time. For example, some star may have a certain rotation rate in the "prime of its lifetime", slow down when it grows to a red giant when dying, and speed up when it collapses into a very tiny neutron star. Also any interaction with other bodies which causes a torque can change the rate of rotation, the moon being the best known. The moon originally had a much larger rotation rate than its current rate of one rotation every 28 days. The torque exerted on it by the earth resulted in the change of rotation.

QUESTION:
In the proposed photoelectric effect, why can't a single electron absorb 2 or more photons having a frequency lesser than the threshold and gain energy greater than the work function and be ejected?

ANSWER:
It could happen, but the probability is so small that you would never be able to observe it except with extraordinarily intense lasers. If you google "multiphoton photoelectric effect" you will find that it has been observed and studied theoretically.

QUESTION:
Just a question regarding forces acting equal and opposite directions... Let's say an electron is traveling through space with nothing around it for thousands of kilometers and it passes through a magnetic field (or electric field). The source of that field is light years away let's say. The electron will experience a force on it causing its trajectory to change. My question is if the electron is experiencing a force then what is the electron exerting a force back onto? I'm a bit stumped by this.

ANSWER:
The answer is that Newton's third law, applied simplistically, is not likely to work for charged fields moving in electric or magnetic fields. A simple example is given in an older answer. This does not mean that Newton's third law is wrong, it is simply that just by saying two forces are equal and opposite, you have to be more general, particularly when velocity-dependent fields (like the magnetic field) are present. Essentially, Newton's third law is equivalent to momentum conservation; if two isolated objects collide, their momentum would not be conserved if the forces they exerted on each other were not equal and opposite. Thus, in that earlier answer, the total linear momentum of the two interacting charges would not remain constant. But the catch is that these two particles are not the only things around, their fields are also present; it turns out that the fields have linear momentum densities and if you also include this linear momentum in the problem, the total momentum is, indeed, conserved. This sort of gets beyond the "layman" philosophy of this site to pursue this more quantitatively.

QUESTION:
Is it possible to reduce a sound with a sound? To clarify, if sound waves come from point A to p. B, is it possible to reduce that sound pollution by channeling another sound from point B to A? is there any possibility of it with different waves?

ANSWER:
The way you can do this at some point is not to send some other sound back to the source but to cause another sound identical to but out of phase with the first to the same point. This is called interference. This is why some auditoriums have "dead spots" where reflected sound arrives at that point out of phase with the direct sound. It is also the idea behind noise canceling headphones which sample sound coming from the outside and add to it an identical sound but upside down.

QUESTION:
Since mass and energy are interchangeable, does energy have gravity?

ANSWER:
Any region of space which has a nonzero energy density will cause a gravitational field.

QUESTION:
OK, I am having trouble with this speed of light thing. If a train left earth at near the speed of light and turned on it's light, the light would leave the train at the speed of light relative to the train, correct? But doesn't that mean that those photons are traveling away from Earth at waaaay more than the speed of light? If so, could we launch a ship (hypothetically) and get to %90 the speed of light and then launch another smaller ship from that ship and travel %90 of light speed relative to that ship? A ship that would be distancing itself from Earth at waaaay more than the speed of light? This is where our slow speeds in space bother me. Since space is a vacuum (nearly) and rockets only push on themselves from the inside and speed is relative, wouldn't a ship that is coasting 40,000 mph away from the Earth, suddenly fire a rocket and accelerate no matter what? Without wind resistance and with the slight help of solar winds, I would think we could travel a probe much quicker. Might have to build it in space but 8 saturn Vs connected together ought to make a difference. No?

ANSWER:
The problem you are having is that you are thinking in terms or classical physics or intuitively; intuition is based on experience and you have no experience with trains going nearly the speed of light. Whenever you extrapolate what you "know" to be true of trains and light to situations outside your experience, you need to be cautious. You assume that if the train has a speed v relative to the earth and the light has a speed c relative to the train, then surely the speed of that light relative to the earth will be c+v. This is called Galilean velocity addition and is only approximately true when all speeds are very small compared to c. In the theory of relativity the velocity addition expression is u'=(v+u)/[1+(vu/c²)] where v is the speed of one thing relative to the earth, u is the speed of another thing relative to the first thing, and u' is the speed of the other thing relative to the earth. In your first case, v is the speed of your train and u is c, the speed of light, so c'=(v+c)/(1+v/c)=c. In your second case v'=(0.9c+0.9c)/(1+0.81)=0.99c. I urge you to look at the FAQ links, why c is the universal speed limit, and relativistic velocity addition. (For the two velocities moving in the same direction, change v to -v.)

QUESTION:
I understand that a fusion reaction occurs when two light atoms fuse to form a heavier atom, and some of their mass is converted to energy in accordance with mass-energy equivalence. I am struggling to understand exactly what this has to do with the "binding energy" of a nucleus. When two atoms fuse, some of their mass is converted into binding energy to hold the new nucleus together--but if this is so, where does the energy release come from? If the lost mass becomes energy that holds the nucleus together, it seems that all of the energy would still be contained in the atom. Does the binding energy take only some of the converted mass?

ANSWER:
Your error in logic is assuming that it takes energy to " hold the nucleus together ". Indeed, if you have a nucleus it takes energy to pull it apart. As an example, the α-particle (⁴₂He) has a binding energy of about 7 MeV per nucleon; a deuteron (²₁H) has a binding energy of about 1 MeV per nucleon. The α-particle has 2 neutrons and 2 protons and the deuteron has one neutron and one proton, so fusing two deuterons should result in one α-particle. The energy released in such a fusion would be (6 MeV/nucleon)x(4 nucleons)=24 MeV. The rest mass energy of an α-particle is about 3700 MeV, so the fraction of mass turned to energy is about 24/3700=0.0065=0.65%. This released energy shows up as kinetic energy of the α-particles heat, in other words.

QUESTION:
If d= at² and d=vt then at²=vt, which simplifies to: a=2v/t, which is wrong since a=v/t. Where am I and my math going wrong?

ANSWER:
Whenever you write down an equation, it is important to ask the circumstances under which that equation is valid. Your first equation is valid if the object has constant acceleration and begins at rest. The second equation is valid if the object moves with constant velocity (zero acceleration).

QUESTION:
Why charge is not considered as a 'Fundamental' physical quantity? We say that 'mass' originated from energy then from where did 'charge' originate?

ANSWER:
The international system of units have agreed to use electric current as the fundamental quantity because it is easier to do an experiment (the force between two current-carrying wires) as an operational definition of the ampere. Since the ampere is charge per unit time, a coulomb is one ampere second, the amount of charge a one ampere current delivers in one second. I would not say that mass "originated from energy", rather that mass is one form of energy.

QUESTION:
Say I toss a coin into the air. From the moment it leaves my hand, are the cumulative effects of quantum uncertainty in the whole system enough to cause a 50/50 chance of heads/tails? Or is it all, in theory, predictable, provided we knew the initial conditions as defined in classical physics?

ANSWER:
I believe that if you had a very good determination of the initial conditions that this would be deterministic. Corrections for air drag would be necessary which might entail doing computer calculations, but provided the air was still or moved in a known way during the time of the flip, you could reliably predict the outcome (no longer a 50-50 chance) of a particular throw the uncertainty principle would play no role for this macroscopic experiment. A further complication would be the interaction with whatever the coin landed on, but you could start off making that surface sticky and add that complication later.

QUESTION:
I was thinking, If electrons in the same orbital must have opposite spins, because of the Pauli exclusion principle, this means that if you measure one electron and it has spin up, automatically you know the spin of the other electron. This is the definition of quantum entanglement right? Knowing the state of one particle by measuring the other, so, does this mean that the electrons within the same orbital are actually entangled?

ANSWER:
The following is the Wikepedia definition of entanglement: "Quantum entanglement is a physical phenomenon that occurs when pairs or groups of particles are generated or interact in ways such that the quantum state of each particle cannot be described independently instead, a quantum state may be given for the system as a whole." This is custom-made for electrons in an atom. The mathematical way of dealing with the Pauli exclusion principle in a system is called antisymmetrization of the wave function. The result is that you cannot label the electrons, all electrons are also part of all other electrons in the system. The Z electrons in an atom are an excellent example of an entangled group of particles, not just pairwise but with all the other electrons.

QUESTION:
I have a question about the CoM on a rider and a bicycle. The popular narrative or meme is that while riding a bike, with your hands on top of the bars, if you then move your hands to the 'drops' on road bike handlebars, that this lowers the CoM. Personally, I do not believe it because we are talking about the riders shoulders and hands moving downward about 2-3".

ANSWER:

First of all, any drop at all of part of the mass of the object (even 2-3 inches) will result in a drop of the center of mass of the object. However, usually the drop is substantially more than just the distance from the top to the bottom of the handlebars because the cyclist also bends his arms at the elbows as shown in the figures above. Of course there is also the reduced air drag when in the lower position.

QUESTION:
Dropping a 3/4 inch sphere magnet through a 3/4 inch copper tube creates an electrical field, (Lenz's Law) If you suspend some magnets in the copper tube using opposing poles, does this continually create an electrical field?

ANSWER:

Lenz's law applies only if the magnetic field is changing. If you simply have a magnet suspended there there will be no induced electric field. You might as well hang it from a string or hold it up with your finger, if the magnetic field does not change Lenz's law is not applicable.

QUESTION:
A hollow charged conductor has normal electric field on its outer surface , why?

ANSWER:

In an ideal conductor, some of the electrons are free to move. If any electric field at the surface of a conductor had a component parallel to the surface, electrons would accelerate along the surface and you would not have an electrostatic situation.

QUESTION:
I've seen the "battleship in a bathtub" physics illustration, in which we imagine a battleship in a bathtub just barely big enough to hold it (a one foot clearance on the sides and bottom). We are told that indeed the battleship will float with this seemingly minimum amount of water surrounding it. If this is true, how does that coincide with the fact that an object will float only if it displaces more than its own weight in water?

ANSWER:

Just because you cannot see the displaced water does not mean water has not been displaced to make room for the battleship. If the water in the tub were at the same level but the battleship missing, the volume occupied by the battleship would now be filled with water. Now, put the battleship in the tub and the displaced water will spill over the side of the tub.

QUESTION:
I am a state trooper here in NC. I was struck by a commercial full sized bus on December 24 2014. This bus was the size of a (trailways bus) . They pulled the recorder from the bus and determined the speed to be 69.5 mph. After impact my vehicle which was stationary in the road (hit from behind) traveled 180 feet after impact. Could you tell me how many g's that I endured. I was knocked unconscious.

QUERY:
It is impossible to do more than a rough estimate with this information. I do need to know were you in gear or parking brake on so that you skidded rather than rolled?

RESPONSE:
Photo of my 2007 Chevy Tahoe which would have had about an extra 700 pounds of equipment. This was a 2 wheel drive vehicle. Parking brake was off I believe. In reference to my question about how many g's did I experiance in the accident. Struck from behind by commercial bus. My Tahoe traveled 170 feet after impact. Final rest was on rt grassy shoulder of roadway. I was hospitalized for 6 days at Duke Hospital.

ANSWER:

First, be clear that what I can do is an order-of-magnitude calculation, making reasonable approximations. It should not be considered as an accurate calculation, for example something which would be used in a court of law. The bus would have a weight of about 28,000 lb≈12,700 kg and your Tahoe would have a mass of about 6000 lb≈2700 kg. Your car slid d=170 ft=52 m; the coefficient of sliding friction between rubber and dry asphalt I will take to be about μ≈0.65. First estimate the speed v which your car acquired in the collision: change in kinetic energy=work done by friction, mv²=μmgd; solving this I find v≈26 m/s≈58 mph. Now, what is going to matter regarding the acceleration of the car (and you) is the time t which the collision lasted. Assuming that the acceleration was approximately constant over this time, a≈v/t≈26/t. To approximate t, use kinematics of uniform acceleration, s= at²= x(26/t)t²=13t where s is the distance the car moved during the collision which I will estimate from your picture to be the amount by which the car lost length during the collision, maybe about 10% of the approximate length of a Tahoe of about 5 m or about 0.5 m. So, t≈0.5/13≈0.038 s and so a≈26/0.038=421 m/s²≈421/9.8≈43 g. Again, keep in mind that there are lots of uncertainties, for example:

If the road were wet or much of the sliding was done in the grass, μ would have been smaller which would have resulted in the speed after the collision being slower which would have resulted in a smaller acceleration. If μ were only half as large, a≈33 g.

If the distance over the time of the collision were larger, the time would have been larger and the acceleration smaller. If s≈1 m, a≈22 g.

If both 1 and 2 are applied, a≈17 g.

In any case, you suffered quite an acceleration! Note that the folks on the bus experienced only about 2700/12,700=21% the acceleration you did.

QUESTION:
Is there a formula or equation something for vertical mass versus hortizontal mass. I work for a granite company, we have a forklift that's weight limit is 6,000 Lbs that is not a problem holding the slabs vertically however we have to place slabs horizontally on a flat table with suction cups. We bought a 7 foot boom to attach to the forklift. I just want an illustration of the inverse relationship of vertical and horizontal weight supported by physics.

ANSWER:

There is certainly not anything like horizontal and vertical mass. Mass is mass. What is going to matter to you for your problem is where the center of gravity (COG) of your load is and that will determine what the torque which will be exerted on the forklift; this torque will tell you whether you can lift the load without tipping the car. What you want not to happen is what is shown in the left-hand picture. I think that you can intuitively tell that the farther out the load is located, the likelier the forklift is to tip. The picture on the right shows how you need to think about the problem to plan for the car not to tip over the front wheels. What you need to know is where the COG of the car without the load is and where the COG of the load is; in the picture, these locations are where the little pinwheels are. The weight of the load is W_L and its COG a distance D_L from the front axle; the weight of the car is W_C and its COG a distance D_C from the front axle. The torque due to the load is T_L=W_LD_Land the torque due to the car weight is T_C=W_CD_C. As long as T_C is greater than T_L, the forklift will not tip over. Now you can see why things are different for a long slab loaded vertically and horizontally since the COG of a uniform slab is at its geometrical center, D_L, and therefore T_L, is much bigger for horizontal. Many cranes and forklifts have a lot of weight added toward the back to allow the load to have bigger torques; note in the figure that the COG of the car is near the rear implying added weight back there.

QUESTION:
I am trying to code a computer program, and I'm creating balls and having them hit each other with different velocities and masses. The collisions i'm dealing with are totally elastic, so kinetic energy is conserved. My question is, if I know both the velocities and masses of the two objects colliding, how do I figure out the resulting velocities of both of the objects after the collision. Also, neither one of the objects are stationary. I've yet to find an answer to this question on the internet in which both objects are moving when they collide. I look forward to hearing your response.

ANSWER:

The detailed calculation is too involved to put here. However, it sounds like you could easily solve your problem if one of the balls were at rest, so I can give you two suggestions about how to attack your problem.

Transform into the center of mass system about which you can find lots of information on the internet; then, transform back to the lab system after the collision.

Transform into a coordinate system where one of the balls is at rest; then, transform back to the lab system after the collision.

QUESTION:
From what I understand, when an elastic spring is stretched, the energy done to stretch it is stored as elastic potential energy in the spring. Also, there is a limit of proportionality where the spring will no longer go back to its original shape. Therefore, when I stretch a spring and the energy is stored, when I let go, the energy stored is transferred to kinetic energy when it goes back to its original shape. My question is, what happens to the stored energy in a spring when the spring has reached its limit of proportionality? The energy stored isn't transferred to kinetic energy as it doesn't go back to its original shape so where does it go?

ANSWER:

The spring is comprised of atoms which are bound together by molecular forces. You might think of each pair of atoms as being connected by tiny springs. When you stretch the spring, you are pulling pairs of atoms farther apart and each tiny stretch takes work and therefore stores energy. When you release it, each pair of stretched atoms pulls back to its original distance. But there are limits to how far you can stretch a bonded pair of atoms before the bond will be broken. The "lost" energy was used to break bonds between atoms. If you want to get more microscopic, just focus your attention on one pair of iron atoms, with total mass M. You pull them until they break apart; you have done some amound of work, call it W. But, you started with and ended with two atoms at rest, so what happened to the energy you put in? It turns out that if you had a sensitive enough scale you would find that the mass of the two separated atoms was no longer M but was slightly bigger, M+m. And, invoking Einstein's most famous equation, m=W/c². No energy in a closed system is ever lost!

QUESTION:
Firearms have always been a hobby (and sometimes a profession for me). A question I am often asked is why shotguns have such high recoil. (Totally subjective as I have shot many rifles with far more recoil than a shotgun.) However there is some validity in the question. Shotguns usually have more felt recoil (that is the recoil forces perceived by the shooter) than many rifles, even when the kinetic energy of those rifles is higher. Now discounting factors such as the weight of the gun, recoil-suppressing equipment such as muzzle brake, etc I have always attributed this to two factors.
1) Shotguns utilize very fast-burning powders, which means the reaction takes place over a shorter period of time resulting in less time for the body to absorb the recoil forces, thus making it feel sharper and more jarring to the shooter.
2) Shotgun payloads, be they shot or slugs tend to be considerably heavier than rifle projectiles. These heavier projectiles by virtue of being more massive have greater resistance to a change in their motion than do lighter projectiles resulting in heavier recoil per unit of acceleration applied to the projectile.
I am mostly inquiring about Number-2. I was recently challenged on this by an argumentative individual. (One of those who just likes to argue even if he knows nothing about the subject.) So I did not engage, but it occurred to me that I might seek the knowledge of a physicist should it come up in conversation with someone who does merit a discussion. Is my science correct on Number-2?

ANSWER:

Both 1 and 2 play an important role. This is basic Newtonian physics. Newton's second law can be written as F=mΔv/Δt where F is the average force felt by a mass m which changes its speed by an amount Δv over a time interval Δt. So, as your #1 suggests, the shorter the time of the acceleration, the larger the force, everything else being the same. Similarly, the larger the mass, the larger the force, everything else being the same, as per your #2. (Also, of course, the larger the change in speed, the larger the force, everything else being the same.) Clearly, the bigger the force on the bullet, the bigger the force on your shoulder; that should be adequate to demonstrate that your arguments are valid. Quantifying that is tricky because it depends on how you define recoil. To see an exhaustive discussion of recoil, see an earlier answer.

QUESTION:
You may have answered this before, but I could not find it by searching the site. Assume there are two objects in space separated by some relatively large distance. Assume object 1 to have the mass of the sun and object two the mass of a typical 100 meter iron asteroid. Assume the separation distance to be approximately 100 million kilometers. There is currently a fictional force preventing object 2 from accelerating towards object 1 and the relative velocity between the two objects in any other direction is zero. Now the fictional force disappears and object 2(asteroid) starts accelerating towards object 1(sun). I am wondering what the formula would be to determine the final velocity for object 2 as it impacts object 1. I know that object 1 has a gravitational acceleration that depends on the distance from it at 1/r but I am unsure how to put this formula together as both that number and the velocity are changing as r shrinks the velocity continues to climb as it gets closer due to the gravitational acceleration of the sun getting larger

ANSWER:

This is fairly easy using potential energy. The gravitational potential energy for a mass m whose center is a distance r from another mass M is U(r)=-MmG/r where G=6.67x10^-11 N m²/kg². The potential energy has been chosen so that U(0)=0 for r=∞. From the example you give, we can assume that M>>m and therefore M will remain at rest. You also need to know the radii of m and M, call them R_M and R_m. So, the initial energy is E₁=-MmG/r₁and the final energy is E₂=-MmG/r₂+ mv² where r₁=10¹¹ m and r₂=R_M+R_m. You can do all the arithmetic if you like, but I am betting that an excellent approximation would be to take E₁≈0 and R_m<<R_M, so 0≈-MG/R_M+ v² or v≈√(2MG/R_M). If you put in M and R_M of the sun you find v≈6x10⁵ m/s. This is also the escape velocity from the survace of the sun.

QUESTION:
We know that when objects move relative to an inertial frame of reference its length is contracted, given by the formula L = l(1 - (v/c)^2)^(1/2), where 'L' is the new length and 'l' is the original length. MY QUESTION IS,WHETHER THE 'DENSITY' OF THE SUBSTANCE ALSO CHANGES? For example, lets say that, we have a 'cube' of side'l' whose density remains constant throughout our experiment, so initially when this cube is at rest with respect to our inertial frame of reference its mass can be written as m = d*V1, where m=REST mass, d=density and V1=INITIAL volume, i.e V1 = l^3. Now this cube is travelling at a constant speed of 'v'(not accelerating) with respect to our inertial frame of reference, so for us the length of its sides would seem to be contracted given by L = l(1 - (v/c)^2)^(1/2), where 'L' is the new length and 'l' is the original length, therefore its new volume(V2) would be V2 = L*L*L, i.e. V2 = l^3 * (1 - (v/c)^2)^(3/2). We can see that V2 = V1 * (1 - (v/c)^2)^(3/2). Now lets multiply 'd' both sides of this equation(keeping in my that density has not changed). This becomes d*V2 = d*V1 * (1 - (v/c)^2)^(3/2), we can also write this equation in terms of mass 'm', as we know that m = d*V, so it becomes M = m* (1 - (v/c)^2)^(3/2), where 'M' is the relative mass and 'm' is the rest mass. But we know that the ACTUAL RELATION BETWEEN REST MASS AND RELATIVE MASS, is given by M = m * (1 - (v/c)^2)^(1/2). So what mistake have I done? will its density remain constant?

ANSWER:

First of all, define what you mean by density. I will define it, using your notation, as mass divided by volume, d=M/V where M and V are the mass and volume when the speed of the box is v. Since the mass and volume both change with speed, there is no reason to assume that density is constant; but we know that mass increases and volume decreases with speed, so the density will increase with speed. Now, a crucial mistake you made was to assume that that V₂=L³ because length only contracts along the direction of motion. So the volume only shrinks by a factor √[1-(v/c)²]. The mass grows by the same factor, so the density of the moving cube is d=d₀/[1-(v/c)²] where d₀ is the density at rest.

QUESTION:
If a Flea was dropped from the top of the eiffel tower what would happen to it?

ANSWER:

A flea has a very small terminal velocity which means that air drag force up on him will equal his own weight after he has achieved a very small speed. If the air were calm, he would drift slowly to the ground and arrive unhurt. If there were a wind, he might land blocks or miles from the base of the tower. Think of dropping a feather same idea.

QUESTION:
If everyone on Earth were to begin running due east at an appointed time, would the Earth slow in its rotation, even slightly? If so, would that reduction in speed be reversed once everyone ceased running (transferring their momentum back to the globe), or would the day have permanently become longer?

ANSWER:

Let's have a look at the numbers. Suppose that there are about 7 billion people with an average mass of 80 kg. The radius of the earth is about 6.4x10⁶ m. So their moment of inertia is about MR²=7x10⁹x80x(6.4x10⁶)²=6.5x10²⁴ kg m². It would actually be quite a bit less than this because most of the earth's population resides at a distance less than 6.4x10⁶m from the axis of rotation, but let's just suppose everyone went down to the equator for this little game. If we approximate the earth to be a uniform sphere with mass of 6x10²⁴ kg, its moment of inertia would be about 2MR²/5=2x6x10²⁴x(6.4x10⁶)²/5=9.8x10³⁷ kg m². The disparities are enormous and there would be no way you could ever notice any change. Let's look at an extreme case where everyone is running just as fast as the whole earth is moving and in the opposite direction (they would be running west). So, the initial angular momentum is the total moment of inertia 6.5x10²⁴+9.8x10³⁷ kg m² times the initial angular velocity, 1 revolution/day. The final angular momentum would just be moment of inertia of the earth (all the people are no longer rotating) times the new angular velocity, call it ω. Then conserving angular momentum, (6.5x10²⁴+9.8x10³⁷)x1=9.8x10³⁷ω or ω=1+6.5x10²⁴/9.8x10³⁷=(1+6.6x10^-14) revolution/day. This means that the day would be longer by 0.0000000000066%. And, yes, in an idealized world where the people and the earth were the only things in the universe, the earth would speed back up again when everybody took a rest.

QUESTION:
I was watching a video on youtube, when a marshmallow was in a bell jar. The air was being decreased within the jar by a vacuum pump. The marshmallow started to expand in size. In the comments of the youtube video, it says that pascal's principle is being represented, and other people say that it is boyle's principle. I am confused, which one does this video represent?

ANSWER:

Boyle's law is a special case of the ideal gas law, PV=NRT, holding temperature T and amount of gas N constant; so it tells you the relation (inversely proportional) between pressure and volume. Pascal's principle is a special case of Bernoulli's equation, P+ ρv²+ρgy=constant setting the velocity v of the fluid equal to zero; in words, if you change the pressure in one place in a static fluid, the pressure will change by the same amount everywhere else in the fluid. A marshmallow is comprised of many tiny air pockets, each like a little balloon, with the pressure inside equal to atmospheric pressure. And, the pressure outside is also atmospheric. Each little pocket has a constant volume because the pressure outside is the same as the pressure outside. If you lower the pressure outside the pocket, there will be a net pressure outward on the volume which will cause it to expand. All the pockets do this so the whole marshmallow expands. Boyle's law says that if the volume increases, the pressure decreases; when the pressure decreases outside the volume increases and the pressure decreases. This demonstration demonstrates Boyle's law.

QUESTION:
Could an 8x12-inch sheet metal sign mounted on a 5-ft tall metal stanchion set in a 5-gal pail of concrete weighing 75 pounds be knocked over by a 30-mph gust of wind?

ANSWER:

Well, that would depend on things you have not told me, the geometry of the pail and also on the mass of the sign+stanchion. I can estimate the force on the sign due to the wind, call that F. This will result in a torque of 5F ft∙lb. I will work in SI units and then convert back to imperial units; A=96 in²=0.062 m², v= 30 mph=13.4 m/s. The maximum force on the sign would be approximately F≈ Av²= x0.062x13.4²=2.78 N=0.625 lb. So now you can do a little experiment: push on the sign with a force of about 2/3 lb and see if it tips over. I suspect, if the base is broad enough, it will not tip over.

QUESTION:
My question pertains to "time travel". I keep hearing that if you traveled at the speed of light that time would move slower than at normal speed; meaning that one would age less than a person moving at regular speed. How does that work? How does speed affect the physical workings of a biological system? I keep thinking that there is no way, say, in 500 years, someone traveling at light speed would only age a day. Wouldn't the only difference be the distance traveled? How does speed stretch a unit of time?

ANSWER:

Nothing can travel at the speed of light. However, time on any clock which is moving (including biological clocks) runs slower as seen by a nonmoving observer. To understand why, see the FAQ page. But, to understand the light clock you have to understand that the speed of light is the same for all observers, again, see the FAQ page. Finally, look on the FAQ page for a link to a discussion of the twin paradox which essentially addresses what you are interested in; note that to compare the total elapsed times on two clocks one of them must go out and come back to compare. In your case (500 years and 1 day), I estimate that the speed would have to be 99.9999999985% of the speed of light for one twin traveling to a star 250 light years distant and returning. When visiting a site like this one, it is polite to read the instructions before submitting a question (groundrules stipulate no questions with objects with speeds equal to or greater than the speed of light; and you are asked to "peruse the FAQ page before submitting your question".

QUESTION:
The equation Q/t=k*A *T/d is to my knowledge the equation for thermal conduction. In thermal conduction energy some energy is supposed to be lost to the material of the object the thermal heat is conducted through. However; I do not see how that is accounted for in the equation other then in the thermal constant part. While working on a project I got a higher thermal energy value, in joules than initially present on the side it initially came from. This would violate the conservation of energy, I believe, so are there exceptions to this equation, is this equation wrong, or was it a math error? In this project the distance was low in the millimeter range ans the object or late was made of silver, which would account for the high answer.

ANSWER:

I believe that you do not understand the equation you have written. This equation should better be written as ΔQ/Δt=kAtΔT/d where ΔQ/Δt is the rate at which heat goes through an object (J/s) of cross sectional area A and thickness d; ΔT is the temperature difference between the two faces of the object. It is an idealized situation where there is thermal equilibrium established, i.e. the heat entering is equal to the heat exiting. So any energy lost to the object occurs during the time when the heat first begins to flow, before this equation is valid; after equilibrium is achieved, no energy is lost in the conduction process. I would guess that where you lost energy was at the edges; you also have to be careful that the temperature is uniform across the whole area A on both sides, otherwise the equation becomes much more complicated.

QUESTION:
if i put a point sized light source inside a pond then depending upon the refractive index of water the some of the light rays will come out.I have figured it out that a circle will be formed at the pond surface.my question is whether THE LIGHT WILL COME FROM INSIDE THE CIRCLE OR OUTSIDE.(basically i'm asking TIR takes place at angle greater than critical or less than critical)

ANSWER:

Inside.

QUESTION:
Why do materials with static electricity attract substances that are not charged?

ANSWER:

I will assume that the charge on the charged object is positive. If the uncharged object is a conductor where electrons are free to move, electrons will tend to migrate to the surface closest to the charged object leaving a positive charge on the opposite side; since the electrons are closer to the charged object than the positive charge, there will be a net attractive force. If the uncharged object is a conductor where electrons are free to move, electrons will tend to migrate to the surface closest to the charged object leaving a positive charge on the opposite side; since the electrons are closer to the charged object than the positive charge, there will be a net attractive force. If the uncharged object is a nonconductor with electrons not free to move, each atom or molecule in the uncharged object, seeing the electric field from the charged object, will become polarized (see picture above) such that the negative side will be closer to the charged object, again resulting in a net attractive force.

QUESTION:
I have an expandable container with 1 m³ hydrogen and 1/2 m³ oxygen at stp. If I ignite this what would the final volume be if there is no heat loss? The pressure outside the container is 1 atm. I have asked this question on a number of web sites and no one seems to be able to answer this. Most want to answer only if there is heat disapation or ignited in open air. I hope you would answer this. it has plagued me for weeks! Could you show the equations so I can understand how to figure similar problems?

ANSWER:

Well, this turned out to be a real tour de force, particularly since thermodynamics has never been my strong suit! First, let me explain carefully how I did it; I think it matters when and how things happen. I first imagined igniting and letting all the water be formed holding the initial volume fixed and found the new temperature and pressure; next I let the volume expand adibatically (no flow of heat into or out of the volume) until the pressure was 1 atm inside and calculated the new volume and temperature. Data I needed:
Initial temperature T₁=273 K,
Initial volume V₁=1.5 m³,
Initial pressure P₁=10⁵ N/m²,
Specific heats at constant volume and pressure:
C_V(O₂)=0.659x10³ J/(kg∙K)
C_V(H₂)=10.16x10³ J/(kg∙K)
C_V(H₂O)=1.46x10³ J/(kg∙K)
C_P(H₂O)=1.93x10³ J/(kg∙K)
Universal gas constant R=8.3 J/mol∙K
Combustion energy per mole for water 286x10³ J/mol
Taking the molecular weights of H₂, O₂, and H₂O to be 2, 32, and 18, respectively, I find that the container contains 22 moles of O₂, 44 moles of H₂, and 44 moles of H₂O.

The internal energy of a gas may be written U=C_VnRT. I find that U(O₂)=3.285x10⁷ J and U(H₂)=1.013x10⁹ J before the ignition, so the initial internal energy of the system is U₁=1.046x10⁹ J. When ignition is complete, the energy added to the system is Q=44x286x10³=1.258x10⁷ J and so U₂=U₁+Q=1.059x10⁹ J. But, from the internal energy of the water vapor, the temperature may be calculated, T₂=2900 K, and then from the ideal gas law (PV=nRT), P₂=7.06x10⁵ N/m². Finally, the adiabatic expansion. In an adiabatic expansion from P₂,V₂ to P₃,V₃, PV^γ=constant, where γ=C_P/C_V. The work W done by the gas in the expansion is W=P₂V₂^γ(V₃^1-γ-V₂^1-γ)/(1-γ). At this stage we do not know V₃, so we do not know W. But W is also known from the first law of thermodynamics as being equal to the change in internal energy U₃-U₂=W (provided no heat flows in or out). Now, we also know that U₃=C_VnRT₃ and T₃=T₂[(P₃V₃)/(P₂V₂)]. Those equations then lead to the transcendental equation 146V₃-1059=3.747V₃^0.322-4.268 which I solved graphically (see figure to right) to obtain V₃=7.27 m³ and T₃=1991 K.

QUESTION:
Why would Einstein square light in his formula E = mc2, since light will only travel 186,290 miles/second? If 186,290 mi/sec is the peak, doesn't squaring it make the peak; the sum of the speed of light times itself, hugely faster than 186,290?

ANSWER:

I often get this kind of question. Just because some quantity appears in an equation in some form other than just itself, why would that imply that that quantity was no longer that quantity. For example, suppose you want to know the volume V of a cube of side L, V=L³ and L=2 m. Would that mean that as soon as you write the equation that L was now 2³=8 meters long? Of course not, 8 does not even have the dimensions of length, it has the dimensions of length cubed. Just because c appears in Einstein's equation squared does not mean that light now can travel that fast; c² does not even have the dimensions of a speed which would be length/time. In fact the quantity mc would not have the dimensions of energy. And nobody ever said that the number c is the biggest possible number, it is just the biggest possible speed anything can have.

QUESTION:
If a spaceship was to be armed with a large weapon that fire non self-propelled rounds, how would this affect the ship/crew inside? For instance, in Halo, the MAC (Magnetic Accelerator Cannon) fires a massive round in stead of a laser or missile with its own source of acceleration. Does this mean that large enough objects "launched" by a space station or craft could have noticeable/detrimental effects to the ship or crew? For instance, would the weapon move the ship in the opposite direction of firing, injuring non-secured crew members? On a massive scale could this harm crew members or possibly destroy the ship because of the violent "cause and effect" force put upon the craft?

ANSWER:

I have done many such calculations and nearly all are preposterously impossible or would have major effects on the vessel firing them. To see some examples, go to my FAQ page. In fact one answer addresses the Halo MAC.

QUESTION:
I would like to know the measurements of each dimension of a boat if the total weight of the boat were to carry 300 pounds. Also, we plan to make a canoe like shape so if you can help us know the measurements for that shape we would be very thankful.

ANSWER:

This is too vague. Lots of possible dimensions would do the trick. You also need to specify the weight of the boat, not just the load. The important thing is that the buoyant force must be equal to the weight of the boat plus load. The buoyant force is the weight of the water displaced by the floating boat. For example, if the boat itself weighs 100 lb, the boat must have the volume which 400 lb of water would occupy; the density of water is about 62.4 lb/ft³, so the volume of the boat would need to be 400/62.4=6.4 ft³. For example, suppose you model your canoe as a triangular prism as shown to the left where h=b and l=6 ft; its volume is hbl=3h²=6.4, so h=1.5 ft. Of course, this would not be a good design since the surface of the water would be right at the gunnels of the canoe, just about to spill in; you would want to build in a safety factor by making the volume of the canoe quite a bit bigger than 6.4 ft³.

QUESTION:
Can solar radiation (light, heat, microwave, magnetism, wave, or particle) have any affect on the mass of a planetary body?

ANSWER:

Heating due to electromagnetic radiation could result in an unmeasurably tiny increase in mass except that the earth and sun are in thermal equilibrium, the earth radiates the same amount of energy which it absorbs. Particle radiation, protons, electrons, and ions, will increase the mass of the planet but again be very small compared to the planetary mass.

QUESTION:
My young son would like to know how fast something would have to move in order to defy gravity. Thanks! He's 10 and would like to become a physicist.

ANSWER:

The phrase "to defy gravity" does not really have any meaning. If you mean that if something goes fast enough you can make gravity go away, that does not happen. More likely you mean how fast does something have to be going to completely leave the earth and never come back, as in "everything which goes up must come down (except when it doesn't!)" This speed is called the escape velocity. The mathematical expression for escape velocity is v_e=√(2MG/R) where M and R are the mass and the radius of the planet, respectively, and and G is Newton's universal constant of gravitation. This comes out to be about 7 m/s=25,000 mph for the earth. For a more detailed discussion (probably over the head of a 10 year old), see an earlier answer.

QUESTION:
Consider an object of mass m kept on an inclined plane of angle of elevation theta. The object being connected by a thread to a pulley with some moment of inertia I. Question is that: Why is the tension in the string not mgsin(theta)? It turns out to be less than that. I don't get an intuitive sense here.

ANSWER:

Although this question sounds like homework, I will work it out since it is such a classic intro mechanics problem. First of all, I assume there is no friction or else you would not necessarily think that T=mgsinθ. But wait a minute, the tension will only be what you think it is if the mass is in equilibrium which it most certainly not be. The thread is wrapped around a wheel free to rotate, so the mass will be accelerating down the plane and therefore not be in equilibrium. So, the tension in the thread is T, the acceleration of m is a, the radius of the pulley is R, the angular acceleration of the wheel is α=a/R, and the normal force on m is N=mgcosθ. Now, focusing your attention on m, mgsinθ-T=ma, one equation with two unknowns, T and a. Now, focusing your attention on the pulley, τ=TR=Iα=Ia/R, a second equation with two unknowns. Solving, T=mgsinθ/[1+(mR²/I)]; this quantity is always less than mgsinθ, as you note. Note that if I=0, T=0 and if I=∞, T=mgsinθ. Here is how you can understand this intuitively: the forces along the incline on m are the component of the weight mg down the plane mgsinθ and T up the plane. If the block accelerates down the plane, the net force must be down the plane, so T<mgsinθ.

QUESTION:
How do they figure out phases of the moon in the past? in other words, how can they take a date hundreds of years ago and determine what phase the moon was in and specifically when it rose in a particular spot? Short of going month by month back tracking. There are sites that calculate it online but what are they actually doing?

ANSWER:

My usual disclaimer I am not an astronmer and often do not answer astronomy questions. I think I can give at least a semi-quanitative answer to this one, though. The details would be more complicated than I am presenting, but what we know with great accuracy is the moon's orbit around the earth and the earth's orbit around the sun. Both are, of course, elipses and their periods are known: the length of a year is 365.2425 days and the time of one lunar orbit is 27.321661 days. The phase of the moon is determined by the relative positions of the earth, moon, and sun; so all you need to know is the phase of the moon on one particular day and to know exactly where both the earth and the moon are at that time (which I will call the reference point). When you choose a day in the future or past, it is fairly simple to compute how many days that is from the reference point; you have to be careful to correctly apply the rules for leap years, every 4 years except at the century. You can then calculate the whole number of orbits of both the earth and the moon in that time and the fractional time elapsed of the last uncompleted orbit. It will be a little tricky to compute just where some fractional time of an orbit will take you relative to the reference point because, the orbits not being quite circular, the speed varies depending on where you are in the orbit (you go fastest when you are closest to the body you are orbiting); doing this, however, is straightforward, just the Kepler problem. So now you know precisely where the earth and moon are relative to the sun and, from that information, you can calculate the moon phase. There are other details you might need to worry about, for example when the Gregorian calendar was instituted and precession of the lunar orbit (which, not being an astronomer, I do not understand in detail). Also, you cannot go millions of years using this method because the moon's orbit is getting larger with time on this kind of time scale. My final disclaimer this may all be wrong!

QUESTION:
What stops atoms from just all mushing into one big soup. Is each atom surrounded by a magnetic field or some other force that lets it be a singularity?

ANSWER:

First of all, atoms are not singularities because they occupy volume. Now, imagine one atom moving toward another. At large distances neither experiences any appreciable force at all because the net charge of an atom is zero. But as they get close together, the electrons in each atom see the electrons in the other atoms as closer to them than the positive charge of nucleus, so there is a net repulsive force trying to keep them apart. If you push hard enough, the force will become slightly attractive and so molecules or solids may form.

QUESTION:
if I take a uniformly charged non conducting spherical shell then electric field intensity inside the shell is zero (gauss law) but then I put a unit positive charge outside the spherical shell then even as there is no net charge inside the spherical shell so by gauss law electric field must be zero but as shell is non conductor there cant be a new arrangement of charge on shell take place so without a new arrangement how can electric field due to outside charge be neglected?

ANSWER:

No, you cannot just choose a Gaussian surface and say there is no field inside it just because there is no charge inside it. You can only conclude that the net electric flux into the surface and the net flux out are the same. If the charge distribution is spherically symmetric (as it was before the extra charge was added), Gauss's law can be used deduce zero field inside.

QUESTION:
Following a nuclear incident (bomb or power plant accident, etc) we often read that resulting elements and isotopes are found in air, soil or food products both locally and maybe thousands of miles away. My question is, how is it known exactly what element it is that has been found?? How does a scientist/person know the difference between strontium 89 and strontium 90 or potassium 40 or anything else? Is it by chemical analysis of some kind or does each radioactive element have a "signature" emission of some kind? Spectral emission analysis perhaps? I am simply curious how it is that anyone knows after, say...the disaster at Fukushima that someone is able to say for certain the exact radioactive particles have been found here int he USA. I'm sure the report is correct, but I am just curious how the exact element is identified.

ANSWER:

The isotopes associated with nuclear incidents are radioactive. One could detect their presence with a simple Geiger counter. To definitively identify them, one would have to do more careful spectroscopy of the radiation to determine the type and energy of the radiation, but it is not so hard.

QUESTION:
In quantum fluctuations, a particle and its respective antiparticle will very briefly appear, and shortly after annihilate each other, correct? But, for example, if an electron and a positron emerge through quantum fluctuations, and then they annihilate each other, the result will be two photons, correct? So why is it that we don't see a flooding of photons in space, if particles are constatly annihilating each other?

ANSWER:

They appear out of nothing, so they violate energy conservation. But that is ok because the uncertainty principle allows that for a brief time. Then they go back to nothing, no photons appear. So technically, you should not say that they annihilate each other.

QUESTION:
If I could find a way to align all the atoms in a pipe or piece of sheet metal in such a way that all the electric fields and all the magnetic fields in all the atoms were in line and such that the 2 fields were at 90 degree angles to each other, would anything happen and if so what would happen?

ANSWER:

I am afraid that this does not make any sense. First of all, an atom is electrically neutral, so it has essentially zero electric field once you are fairly far away from it. What field it does have points in all directions, so you could not align its field in any particular direction. Magnetic fields are even smaller and also do not point in a particular direction.

QUESTION:
I understand that because of time dilation that we could never see something actually entering a black hole. That is to say, from our earth view, time would appear to stop at or near the event horizon and observed objects would appear to move slower and slower until they seemed to be standing still (to be clear, I certainly understand that the object does not actually halt its motion, and will continue to accelerate and fall into the hole). I understand (layman's level) the basic concept of time dilation, and that it is how the speed of light remains constant for all observers, but the idea of something appearing to halt its motion is pretty mind-blowing...I picture (when it becomes technically possible to actually see one) an event horizon cluttered with incoming matter but that matter never descending (according to our observation) into the black hole. That seems far fetched to me, so I am sure I'm missing something.

ANSWER:

First of all, once an object passes the event horizon, you cannot see it at all. I would say that we really do not know how a clock would behave inside the event horizon because, as shown below, the field is two intense for the usual gravitational time dilation expression to be valid. You might want to look at an earlier answer on gravitational time dilation. The rate at which the clock runs slower in a gravitational field (compared to your clock in a very weak gravitational field) is √[1-(2MG/(Rc²))] where R is the distance from the center of a spherically symmetric mass M. Note that if R=2MG/c² this is zero and the clock stops. That magic R is called the Schwartzchild radius and so if you are watching this clock, you see it stop at this R. From the point of view of someone traveling with the clock, though, time clicks on as usual and you enter inside R. Your fate would be that as you got closer to the black hole (imagine you are falling head first), the gravitational force would be much larger on your head than your feet; this kind of gradient of force is called a tidal force and it would rip you apart. A nice little animation may be see here.

QUESTION:
I have a question regarding charge distribution on different surfaces. We know on sphere charges will be equally distributed but in case of pointed surfaces like triangle this is not the case. I want to ask why more charge is present on pointed edgy surface, is charge density has to do something with it?

ANSWER:

I assume you are talking about ideal conductors. The surface charge on any ideal conductor must be distributed in such a way that the electric field is normal to the surface everywhere. For a nonspherical surface, this cannot be achieved with a uniform charge distribution.

QUESTION:
As the length of a Martian day is approximately 40 mins longer than that of earths, however as the gravitational force exerted is 62% less than that of earth, and gravity has been proven to have a direct influence on spacetime. Would we as humans perceive the Martian day as shorter to that of earths?

ANSWER:

The effect of gravitational time dilation is very small for objects the size of the earth or Mars. The difference between an earth clock and a Mars clock would be almost too small to measure.

QUESTION:
Why does angular velocity act only the in case of circular motion? I mean that if I see a car moving in a straight line(while I am in rest) still the angle subtended by the car at my eye changes (as the car approaches me) since theta is changing with time then there should be angular displacement, and accordingly angular velocity, shouldn't be?

ANSWER:

You are certainly right. You may describe the motion of any particle by specifying angular velocity relative to some axis; you must also express the velocity the object has radially away from (or toward) that axis to fully describe the situation. In the figure to the right, an axis has been chosen and the line from the axis to the car has a length r at this instant; the velocity v of the car has been resolved into radial (v_r) and tangential (v_θ) components. The rate at which r is changing is what v_r is. The rate at which θ is changing is the angular velocity, ω=rv_θ. If the car happened to be moving in a circle around the axis, v_r=0.

QUESTION:
Theoretically speaking, If you had a super powered light bulb Initially located 1 light year away from an observer, but the observer moved at a constant 99% the speed of light away from the light source, how long would the observer observe the light if the light was turned on for a brief moment of like 4 minutes? would the observer observe only 4 minutes of an illuminated bulb or because of the speed of the observer moving away from the light source would it take longer for all of the light to reach be observed thus making it seem like the light would be illuminated for much longer?

ANSWER:

This is an example of the relativistic Doppler shift. If the period of the light waves at the source is T and at the observer is T', the relationship between them is T'=T√[1+β)(1-β)] where β=v/c. For your question, T'=14.1T. It follows, therefore, then that the time it takes for the wave to pass you is 4x14.1=56.4 min. The light has been extremely "red-shifted".

QUESTION:
Is there an anti Higgs boson?

ANSWER:

No. There are two kinds of elementary particles, fermions and bosons, and only fermions have antiparticles. For more detail, see this link.

QUESTION:
I have recently started flying quadcopters (QC) and on a forum I frequent, someone mentioned that by placing the battery of their QC on top of the frame rather than below it they felt that the QC quote "went from Buick to Porsche in responsiveness" Since I found this an interesting assertion I looked at the physics of the situation with my high school physics mentality and came up with this possible explanation. Note it is over 40 years since I left high school by the way, :-) and am just wondering what you think about my hypothesis and if you would care to venture your thoughts on the physics of this situation. My tongue in cheek explanation that accompanied the diagram read as "Correct me if I am wrong, it wouldn't be the first time. The quad pivots around point x and with the battery at the bottom in posn B the distance B is greater than the distance A which is the CG of a battery in posn A. Therefore to overcome the inertia of the posn B battery requires more force than overcoming the inertia of a battery in posn A. This makes the quad more responsive with the battery in posn A. Anyway that is my hypothesis but if I start believing my own BS then let me know"

ANSWER:

This is not quite as simple as I though it was going to be! I will give you the full-blown "physics talk" explanation. First, it will not have any effect on linear acceleration because all that determines that is the total mass of your QC and the force you can get from the propellers. However, moving the center of mass (COM) will have an effect on angular acceleration, the rate at which you can cause it to turn. The appropriate equation to understand this is Newton's second law in rotational form, torque τ equals the moment of inertia about the COM I_cm times the angular acceleration α, τ=I_cmα or α=τ/I_cm. The torque due to each propeller is the force it exerts times the distance from C/L to the propeller shaft. The moment of inertia about the COM of the whole thing is what you need because the whole QC in flight will rotate about its COM. Everything depends on I_cm,and the smaller you can make it, the greater angular acceleration you can get for a given torque. The farther away the battery is from the COM of the QC, the greater I_cm will be. So to have the most responsive handling, you want to find the COM of the QC without its battery and put the COM of the battery right there. I am guessing that battery position A is closer to the COM of the QC without battery than battery position B is. If the mass of the battery is much less than the mass of the QC, the "Porsche responsiveness" is an illusion.

QUERY:
It would be helpful if you could give me the relative masses of the QC without battery and the battery. And also where the COM of the QC without the battery is located. I assume it is on the C/L, but how far from the airframe labeled on your diagram?

REPLY:
The relative mass of the QC is 20 grams without the battery and 28 grams with the battery. The COM of the QC without the battery is located on the C/L, but as to how far from the airframe labeled on my diagram I cannot answer. My whole hypothesis, for want of a better word is based on my assumption that if the left hand propeller is exerting an upward thrust and the right hand propeller a downwards thrust simultaneously, the the QC will rotate around point x.

ANSWER:
Your assumption, as I explained in the first answer, that it rotates about x is not correct; it rotates about the COM wherever that is. It may appear that it is rotating about another point because the overall motion may be described as the sum of translational motion (along a straight line) of the COM and rotational motion around the COM. The mass you state cannot be correct since 20 gm is less than an ounce; but this does not matter because to answer your question all I need are the relative masses of the QC and the battery. As explained above, the battery being a significant amount of the total mass (nearly 1/3), its placement will be important. So the whole key is that the moment of inertia about the COM of the whole vehicle is lowest when the two centers of mass are as close together as possible; this results in the maximum angular acceleration for a given net torque.

QUESTION:
I am at the moment working on a novel. I am trying to create a unified theory of everything within the framework of the said novel without contradicting the actual Laws of Physics (at least in general :)). Which brings me to my question I wasn't able to find the answer to so far: If a being moves an atom (close to another atom to allow them to bind together to create a molecule) what kind of energy transfer happens between the being and the atom if any? Would it make a difference if this happened in space, i.e. in imperfect vacuum, or on Earth?

ANSWER:

Well, I do not know about a "being" moving an atom you or I could cause another atom to move, but you certainly cannot grab onto it with your fingers and make a molecule. So, let's just leave out the "being" part and ask about the physics does it cost energy or release energy if two atoms form a molecule? It is easier to understand in the reverse direction. If you have two atoms bound together in a molecule, to pull them apart you must do positive work which means that you pull on an atom and the direction of your pull is in the same direction as the direction of the motion of the atom. Work is what you do to add energy to something pulling a rock up a hill, for example, increases its potential energy because you are doing positive work on it. Therefore the two atoms have more energy if they are apart than if they are together. What this has to mean is that if you now take the two atoms and bring them back together, they will ultimately attract each other so that you will pull opposite the direction they are moving which is taking energy away from the system (negative work done by you). Also, note that this is common sense; when you burn a fossil fuel, for example, you are combining carbon atoms with oxygen atoms to create carbon dioxide molecules and energy is released in the form of heat.

QUESTION:
Suposing a Man Went To Another Planet That Has a Gravity More Than Earth Hundreds Of Times Wich Means That Time Is Too Slower There Than Earth. If We Say That 1 Hour In That Planet Is 10 Years On Earth And He Stayed 2 Hours There Will He Comeback To Earth Looking 20 Years Younger Than Other People Who Were at The Same Age When He Left ?

ANSWER:

No, not hundreds of times, billions of times more. I calculate that if the planet were the size of earth, its surface gravity would be such that the man's weight would be approximately ten billion times greater than on earth. That man will not be coming back from this planet since his own weight will crush him. If you had some indestructable clock which you could put there and pull back when that clock had run for 2 hours, it would return to you in 20 years as measured by your clocks. Although I have not done the calculation, it is likely that this would, in fact, be a black hole and that it would not be possible to return your clock if it were inside the Schwartzchild radius.

QUESTION:
Would it be acceptable/correct to say that the Sun orbits the Earth, with respect to the Earth? For example, when describing the rising/setting of the sun and the alteration of night/day on Earth (respect to Earth), in that context, could one say that the Sun is orbiting the Earth?

ANSWER:

No, because to an excellent approximation the sun is in an inertial frame (at rest or moving with constant velocity) and the earth is an accelerating frame because of its orbit. There are also innumerable other examples which could not be understood (like the motions of the planets in the sky) by an earth-centric model.

QUESTION:
I'm a writer working on a nonfiction book that concerns climate and landscape, and am seeking help understanding a phenomenon I am hoping to explain to lay audiences in this book. A hot air balloon pilot I interviewed described a near-crash. On a calm morning, this pilot took a family on a short flight over some meadows, not far from a geothermal plant. While preparing to land, the pilot's balloon was drawn uncontrollably towards the geothermal plant, where it was trapped in the column of hot air rising over the plant, like a ping pong ball over a hair drier. I am trying to understand what might have caused the balloon to be drawn towards this rising column of air. The pilot did not accidentally pass over the geothermal plant, but rather the balloon was uncontrollably sucked into the column from the side.

ANSWER:

This is a manifestation, I believe, of the Venturi effect which says, in essence, that the pressure in a fluid decreases as the velocity increases. It is just a special case of the Bernoulli equation, P+ ρv²+ρgy=constant. In cases like this balloon, Bernoulli's equation will not give you precise results because it is valid only for incompressible ideal fluids which air certainly isn't. However, it is excellent for qualitatively understanding many things which happen in moving fluids. Examples of the Venturi effect abound. An airplane wing generates lift because air passes over the top faster than over the bottom resulting in a net upward pressure. A spinning baseball curves because the pressure on one side is greater than the other. A sheet of paper rises when you blow across the top (see picture). Although you do not see this so much any more, if smokers inside a moving car crack the window, the smoke will be drawn out by the lower pressure of the outside air rushing past. In the case of the balloon, the column of hot air is rising whereas the air around it is still, so objects near it will feel a force drawing them into the column.

QUESTION:
why Mercury have less gravity as compared to earth,while its mass is almost same as earth.is it because of lower spin speed

ANSWER:

Sorry, but the mass of Mercury is almost 20 times smaller than earth and its radius is almost 3 times smaller. You can calculate the acceleration due to gravity at the surface, g=MG/R² where G=6.67x10^-11 N∙m²/kg². The data for Mercury (earth) are: mass M=3.3x10²³ kg (6x10²⁴ kg); radius R=2.4x10⁶ m (6.4x10⁶ m); gravitational acceleration at surface g=3.7 m/s² (9.8 m/s²).

QUESTION:
I came across a question in the back of the chapter that asked: "Because a smaller mass results in greater air resistance effects on a ski jumper, all other things being equal a lighter ski jumper flies farther than a heavier one." According to the book the answer is true. My question is why?

ANSWER:

There are two forces on a ski jumper, his weight W and the force of air drag on him F. The weight is always a force which points vertically down and is proportional to the mass m. The air drag always points opposite the skier's velocity and depends only on the velocity magnitude, the properties of the air, and the shape of the skier, but not his mass. Shown in the figure are the force diagrams for the heavy (#1, leftmost) and light (#2) skiers when they are going up. (I have chosen an extreme case, the heavy skier about twice the mass of the light skier, to emphasize the difference. Everything else about the two is identical.) Each force results in an acceleration (shown as the green-dashed vectors) which result in net accelarations shown by the full-drawn green vectors. The lighter skier has a larger drag acceleration because the acceleration is F/m and both have accelerations due to their weights which are equal. But looking at the horizontal and vertical of the net acceleration, both are larger for the light skier he is losing forward speed faster and is accelerating toward the ground faster. Also shown in the figure are the force diagrams for the heavy (#3) and light (#4, rightmost) skier when they are going down. Now, the lighter skier still has a larger horizontal component of acceleration, he is slowing down faster, and his vertical component is perhaps just slightly smaller. I would conclude that your book is wrong, the heavy skier jumps farther. Consider the following experiment to demonstrate that the heavier will have a larger range using two balls of the same size, say a baseball and a baseball-sized nerf ball. Throw them each as hard as you can and projected at about the same angle; which would you expect to go farther? In fact, there is an analytical solution (approximation) for the range R of a projectile: R=[v₀²sin2θ/g)[1-(4v₀sinθ/(3g))(C/m)] where v₀ is the speed at launch, θ is the launch angle, and C is a constant which depends only on the size and shape of the projectile. Note that R gets larger as m gets larger.

QUESTION:
When an object is picking up speed while free-falling in a gravitational field, an accelerometer doesn't register any acceleration because there is none. The objects velocity is changing but it is not accelerating. How do you describe this state in which the velocity of an object is changing but it is not considered to be accelerating? When an object is in free-fall in a gravitational field are there considered to be any forces acting on it? Of course when an object is not in free fall it experiences the force of gravity as it is pressed against the earth. When it is falling however, it feels no force so is it correct to assume that it has no force acting on it while in free-fall .

ANSWER:

You have just encountered what is called the equivalence principle. Since you have stipulated that the object is in free fall, the answer to your second question is that, yes, there is a force on the object, its own weight. The equivalence principle states that if you are inside a box in empty space with acceleration a, there is no experiment you can perform which will tell you that you are not in a box at rest in a gravitational field with gravitational acceleration a. A corollary is that if you are in free fall in a gravitational field, there is no experiment you can perform which will tell you that you are not at rest or moving with constant velocity in empty space. That is why the accelerometer reads zero in your first question, not "because there is none"; the object is actually accelerating but cannot detect it.

There is a story about Albert Einstein, probably apocryphal, but amusing and instructive. As you probably know, his first job was as a clerk in the Swiss Patent Office and he could easily complete all his responsibilities quickly and then spend the rest of the day thinking about physics. One day, while watching a workman paint a building across the square, the workman fell from his ladder. Einstein thought to himself, "there is no experiment which he can do which could distinguish whether he was in free fall in a gravitational field or was in an inertial frame in empty space".

QUESTION:
I heard that when light strikes a target, there is a measurable force that pushes the target a certain amount. So light can exert some "presure", right? My question is, if a light projector is emitting light towards this target, is there any measurable "recoil" on that projector or any force that pushes the projector in the opposite direction the light is traveling?

ANSWER:

There are ways that light pressure may be detected. See earlier answers about a "singing cymbal", light pressure, and Crooke's radiometer. The force for normal light intensities and normal size of target is very small, though, so it is certainly impossible that you could measure the force on a projector due to the light it projects. I will do a very rough estimate of the force felt by the projector to convince you. From the "singing cymbal" answer, you will find an expression for force, F=2Nhf/c where N is the number of photons per second, h=6.6x10^-34 J∙s is Planck's constant, c is the speed of light, and f is the frequency of the light. This equation has a factor of 2 because in that question the photons were being reflected from the target, but in your case they are simply being emitted, so the factor of 2 should be omitted. Also, note that the wavelength of the light is λ=c/f. So, in your case we may write that F=Nh/λ. I showed in a recent answer that an estimate of the intensity of of visible photons from the sun at the earth's surface is about 4.4x10¹⁷photons/s/m²; this should be a reasonable of the intensity of a bright projector. Estimating the lens to have about a 1 cm radius, the area which the light exits is about 3x10^-4 m², so N=4.4x10¹⁷x3x10^-4=1.3x10¹⁴ photons/s, and therefore F=1.3x10¹⁴x6.6x10^-34/550x10^-9=1.6x10^-13 N. I have taken λ=550 nm, yellow, the peak intensity of the sun's visible light.

ADDED THOUGHTS:

It occurs to me that with a force this small, how could the singing cymbal possibly work? I believe that the answer lies in the fact that I have approached this from the perspective of visible light and this is only a very small fraction of the total radiated power. Suppose we reframe the problem by assuming the projector has a color temperature of 5500 K (the same as the sun) and calculating power flux Φ using the Stefan-Boltzman law assuming black body radiation, Φ=σT⁴where σ=5.7x10^-8 W/m²/K⁴; then Φ=5.2x10⁷ W/m². The radiation pressure for this flux is P=Φ/c where c=3x10⁸ m/s, so P=0.17 N/m². Estimating, as above, that the area of the exiting radiation is 3x10^-4 m², the force turns out to be about F=5.2x10^-5 N. I suppose that a carefully designed experiment could detect a force this small. For example, if the projector had a mass of 1 kg and were on a frictionless surface, it would move a distance of about 2 m in a day due to the acceleration caused by such a force.

QUESTION:
I watched a documentary recently about extending human life, and my space loving head went to next stage with the idea and started pondering how that might prove useful for exploring the universe outside our solar system. So to get to the point, the documentary spoke of a theoretical way to extend human life to 3000 years long. So naturally this would mean that traveling to other stars wouldn't be such a horrible ''life eater'' activity and make traveling to other stars more durable. So in my head I took the next step. Travel to other galaxies. The highest velocity on a spacecraft propulsion system I found was anti matter propulsion at 80%C. So let's say our future spacecraft traveling at 80%C to Large Magellanic Cloud is traveling there with its crew. So plugging in relativistic effects, would traveling at 80%C mean that a person who was a baby when the craft left Earth, would still be alive (+and hopefully in a condition to increase the population in the destination) after the trip?

ANSWER:

3000 years sounds pretty crazy to me. And, don't you think it would be pretty boring? Anyhow, we can estimate the time (on the ship) required pretty easily. The gamma factor would be γ=1/√(1-0.8²)=1/0.6 and the distance is about d=1.6x10⁵ ly. So, the length-contracted distance would be d'=d/γ=96,000 ly so the time to get there would be t=d'/v=120,000 years. 3000 years ain't gonna get it!

QUESTION:
I caught a fly in an empty jar and sealed it with lid. Container weighs X. Fly weighs Y. When the fly alights on the bottom, I assume the container now weighs X + Y. If the fly takes flight does the same hold true.

ANSWER:

You forgot about the air in the jar which weighs Z. I have answered this kind of questions many times, usually we have birds in a box. It all boils down to Newton's third law. Assume the jar is on a scale, fly on the bottom. The scale reads X+Y+Z. Now suppose the fly hovers or flies with constant velocity. To do that the air must exert an upward force of Y on the fly; but that means the fly exerts a downward force of Y on the air; but that would have to mean that the air now exerts a downward force of Y+Z on the jar. So, again, the scale reads X+Y+Z. Now suppose the fly has an upward acceleration a. To do that, the air must exert an upward force of Y+(Y/g)a=Y(1+(a/g)) where g is the acceleration due to gravity; it then follows that the scale will read X+Y(1+(a/g))+Z. Similarly, if the fly has a downward acceleration of a, the scale will read X+Y(1-(a/g))+Z. If the fly is in free fall in the jar, the scale will read X+Z. My answer ignores buoyancy of the fly and, when he is in free fall, air drag. If we consider air drag, when the fly in free fall has reached terminal velocity the scale will, again, read X+Y+Z.

QUESTION:
The wake of a model boat traveling at constant speed seems to bend so that the 'older' part (furthest from the boat) appears to be catching up to the boat. I have read that water waves don't accelerate - though they do spread out before they disappear. Is that fact the waves spread causing this effect or something else because they do appear to accelerate?

ANSWER:

It turns out that the wave pattern of a wake in deep water is well understood. It is called a Kelvin wave pattern. The mathematics is somewhat daunting. To the right are a calculated pattern and a figure showing some of the terminology. To the left is a photograph of a boat and its wake. For years I taught that the wake of a boat was just a shock wave because the boat is going faster than the speed of waves in the water (analogy of a shock wave for an airplane going faster than the speed of sound) but apparently that was wrong. The angle of the wave, from the research I did, seems to depend on the depth of the water, not on the speed of the boat. (Once again, Ask the Physicist learns something new!) The "diverging wave crests" which seem to bend forward are what you refer to in your question, I assume.

QUESTION:
Hello, I am a junior in highschool, and I am currently studying about momentum in physics. I am a bit confused with the concept though. I get it is related to newton's laws and it is mostly used for collisions. I also learned this week about impulse and collisions and conservation of momentum. So now my teacher explained that for momentum to be conserved the net force acting on an object must be 0. That's because Ft=deltap (so if f is 0 then change in momentum is 0 so it is conserved) Now we then took about inelastic collisions and that K.E is not conserved because some is changed to hear or other forms of energy. So I've also learned previously that deltaKE=Wnet so if there is change in KE there is work and therefore a force so how then is there a net force acting in inelastic collisions but still momentum is conserved ??

ANSWER:

We often think of putty balls colliding when we think of perfectly inelastic collisions. The balls collide, squish together, and then stick together. Think about squishing a putty ball does it take any work? Of course it does. Can you get that work back? Not a chance. What happened to it? the ball got a little warmer. Or think of a colliding ball as a spring. That makes sense because a rubber ball will compress and then, when you quit pushing on it, go back to its original shape. So, you put some energy into it. If it were an ideal ball-spring and you just let go of it, it would oscillate from squished to stretched back and forth forever. Does it do that? Of course not because it is not an ideal ball-spring and that energy you put into it eventually (actually very quickly) all disappears (becomes a bit of thermal energy in the ball). So, even balls which do not stick together usually have some inelasticity when they collide. But, when balls collide, what is the force which does the squishing work? It is the force which one exerts on the other and the other exerts on the one; Newton's third law tells you that these have to be equal and opposite, so momentum is conserved even if energy is not. Billiard balls are very good (and very stiff) springs and collide almost elastically.

QUESTION:
Charges concentrate on sharp points of an object. Voltage proposionates to charge/radius. In an egg shape object the voltage equal everywhere . But charges are high where the radius is small. How is it possible?

ANSWER:

Electric field at the surface of an ideal conductor is always normal to the surface regardless of the charge distribution. This means that it takes no work to move a charge on the surface. By definition, therefore, the surface is an equipotential.

QUESTION:
When we switch on a flashlight light energy is produced through conversion from heat. Now light being an em wave should leave its source and go away then where does that light energy go on switching off the flashlight?

ANSWER:

The light will either be absorbed by something it strikes (thereby transferring its energy to that something) or it will continue out into space until it does strike something. The energy it carried continues going or is transferred to something else.

QUESTION:
If we heat a metal for a certain period with a certain heat it will radiate EM waves (Light). If we continued to heat it for a long time will the mass of the body gets affected?

ANSWER:

The higher the temperature, the more energy the metal contains. So, technically, a higher temperature means a higher mass. The effect, though, is so small that you would be hard-pressed to measure it.

QUESTION:
There was a paper published recently that hypothesised that when the solar system passes through the galactic disk, dark matter present there might push asteroids around sending them on a collision course with earth and possibly contributing to extinctions. Could geomagnetic reversal also be influenced by passing dark matter? I know there's no correlation between our trip around the milky way and geomagnetic reversals, but I don't see why wandering dark matter might have an impact.

ANSWER:

Inasmuch as nobody has ever directly observed dark matter and we have no idea about its properties beyond that, if it exists, it would interact gravitationally, such a hypothesis is just that, a hypothesis. Regarding geomagnetic reversal, who knows. It seems that gravity would not have a major impact on currents causing magnetic fields.

QUESTION:
If the Higgs Boson is a hundred times heavier than a proton, how are they produced by colliding two protons? Where does the extra mass come from?

ANSWER:

Good question. Relativity tells us that the mass of an object which is m₀ when at rest and is traveling with a speed v becomes m=m₀/√[1-(v/c)²] where c is the speed of light. In the LHC the speed of the protons is 0.999999991c, so their mass is m=m₀/√[1-(0.999999991)²]=7456m₀, plenty of mass available!

QUESTION:
I recently started taking a sociology course, our main focus of study is global inequality and the distribution of wealth. How the current climate of wealthy and poor nations came to be. What's struck me is that it's mostly due to the era of colonialism and the mad dash for resources. Which got me to thinking about how we power our world. Coal, hydroelectric, nuclear, solar, and geothermal. It all boils down to electricity. And I couldn't help but wonder if there isn't anything else? Why is electricity the only energy source we can exploit? The universe is a large and varied place, you'd think that there would be some other power source we could use. So in a nutshell, is electricity all there is? Are we looking into alternatives?

ANSWER:

Well, your question seems a little muddled regarding what "power source" means. What would a power source 200 years ago have meant?

You might have said human muscles and domesticated animals' muscles. But that would not be the source, rather the food you and they ate. But that would not have been the source because the sun provided the energy to make that food.

Or you might have said water (grist mills) and wind (wind mills and sailing ships). But that would not be the source, rather the sun's heating of the atmosphere causes the wind and causes water to evaporate and then fall at high altitudes so that it can fall down and run your mill.

Or you might have said fire which you would used to heat your home or cook your food. But that had its source in the fuel you used, wood or coal or peat. But, those are all from the sun just like the food you eat is.

So, you would conclude that the sun was the "ultimate source" of energy 200 years ago. Now, looking at today, you say that electricity is the power source we use. But that, again is not the ultimate "source". And, it is certainly not the only end of the "energy chains" which power our world. Petro fuels makes most cars, trains, boats, and airplanes do their thing. Muscles still are used to move us around and do lots of work. Also, we are still using wind and hydro power, but those are still ultimately from the sun. But now, these days, we new have sources, some of which are not from the sun.

Geothermal taps the thermal energy deep inside the earth which is not the result of the sun's heating.
Nuclear power utilizes the energy released in the splitting of very heavy atoms. The source of heavy atoms was not the sun but some long dead star.
Solar cells directly convert the sun's light into electrical power.

So, electricity is not the culprit at all. It is a very clean and efficient way to use energy which we reap from the sun and other sources. And I fail to see the link between electricity and wealth distribution. What we should be worrying about is what energy consumption does to the environment. The continued overuse of energy sources which put large amounts of CO₂ into the atmosphere will ultimately mean disaster for all nations regardless of their wealth.

QUESTION:
When light enters into a black hole does the velocity of light become greater than the speed of light in vacuum due to extreme gravity?

ANSWER:

As light falls into a black hole, it gains energy like a mass does, but it does not do that by increasing its velocity. The energy of a photon is hf where h is Planck's constant and f is the frequency of the light. So, the energy of the light increases by the frequency increasing (or equivalently, by the wavelength decreasing).

QUESTION:
When an object that has been constrained to move on a radius, in this case counter-clockwise, is released it (A) flies off tangental to the radius. Does it also have any (B) rotation outwards off its former curve?

ANSWER:

Only if it had that rotation before it broke loose.

QUESTION:
If I observe an object approaching me from 1 light year away at.9 of the speed of light and a second object approaching from the opposite direction at the same speed and from the same distance, then from my point of view they will have travelled a combined distance of 2 light years in .9 of a year. Is it valid in relativity theory for me to apparently observe 2 objects approach each other at greater than the speed of light. I understand that neither of them will see themselves approaching each other at greater than the speed of light.

ANSWER:

As soon as you say "approach each other" you are talking about how "each" sees the "other" "approach". They may seem to you like they are approaching each other with 1.8c, but that is not the same as approaching each other with speed 1.8c.

QUESTION:
I just read that transverse wave can not be produced in air .Then some examples of it are radio and light wave . SO HOW LIGHT WAVES TRAVEL IN THE ATMOSPHERE?

ANSWER:

Because it is not the atmosphere which is supporting the wave, the air is not "waving", rather electric and magneitc fields are waving. If you take the air totally away, the light waves still go through.

QUESTION:
I'd like to know how counting with half-time, the decaying of radioactive atoms, is possible. I mean if it's impossible to know when atoms will decay, how is it possible to know the half-time decay? I understand the principe but not the possibility. (From a high school student)

ANSWER:

Half life is a statistical property you need a large number for it to be meaningful. I think you would agree that some radioactive atoms would be more likely than others to decay. With that idea, it should make sense to your that the rate R at which the number of radioactive atoms in a large sample changes will be proportional to the number in the sample, N. So you can write R=-λN where λ is the proportionality constant (called the decay constant) to make the proportionality an equation; the negative sign is there because it is conventional to make λ be positive and I know that if the number is decreasing, the rate of change of N must be negative (getting smaller). I do not know whether you have had calculus or not yet, but to solve for the unknown N(t) you really need it. I will proceed assuming you know calculus: dN/dt=-λN and so N=N₀e^-λtwhere N₀ is the number of atoms when you started measuring (t=0). The half life is defined to be the time when N=N₀/2, so solving, =e^-λtor ln( )=-0.693=-λTor T=0.693/λ. A graph of N(t) for two values of λ is shown to the right. Here N₀=10,000 and you can see that for λ=1 that t≈0.7 when N=5000.

QUESTION:
Clearly more massive objects create a greater gravitation field, but what about objects that are equally massive yet have more energy? For example, what if a certain object had an outrageous amount of heat, or rotational energy? Would these objects produce an equally strong gravitational field as their equally massive (yet cold and stationary) counterparts?

ANSWER:

It is my understanding that general relativity predicts than any region in space which has energy density creates a gravitational field, it need not be static, cold mass. Since you must add energy to heat something up, it would have more mass but very little more mass.

QUESTION:
I just saw the question in your page about super powered people telekinetically lifting objects and how Newton's laws have something to say about that. So that rose me a question about the thing. Lifting the submarine should require enormous amounts of energy, and this would naturally mean that a super powered individual who uses his or hers powers to lift an object such as a submarine would need to have some borderline magical energy production methods far surpassing anything biology gives to any creature. So if we assume that the sub marine weighs ten thousand tons, how much energy would Magneto need to lift it at the speed of let's say ten meters per second? And how does that relate to energy consumption of a normal human, and would even a nuclear reactor be enough to produce the necessary energy for lifting the submarine?

ANSWER:

It would make more sense for your question to ask about either force, as the original question did, or power, the rate of delivering energy. If the mass is 10⁴ metric tons, the weight would be about F=10⁸ N; that would be the force you would need to exert to lift the submarine. If you were lifting at the rate of v=10 m/s, the power P required would be about P=F∙v=10⁹ W=1 GW. A gigawatt is a typical output for a nuclear power plant.

QUESTION:
I just wanted to ask you about your opinion on string theory outrageous claims, things appearing out of nothing, really string theory. String theory math is all too complicated and with no experimental evidence besides the equations. And the hope of putting all that into a"one inch equation" noble but is it possible? I would love to hear your opinion.

ANSWER:

I think you are overreacting a little bit here! My opinion is that string theory is not a theory because it makes no predictions and is therefore not falsifiable or verifiable. However, it is a hypothesis which many good scientists are struggling to develop. And who can predict that any idea is not "possible"? And the math being "too complicated" does not mean that there is no value in the ideas; Einstein found the math needed for the theory of general relativity was "too complicated" for him and he needed to get help from mathematicians.

QUESTION:
I understand that, with a roughly spherical object, like the earth, the gravitational force tends to act on objects towards the centre of the sphere. What would the direction of the force be with an object shaped like a cylinder? Also would the gravitational pull be greater on each end of the cylinder than at some point in the middle? (In which case I would guess that, in nature, any massive cylinder would collapse to form a sphere?)

ANSWER:

The gravitational field of a cylinder is pretty easy to calculate on its axis and very difficult to calculate elsewhere. At the center of each end of a cylinder of length L and radius R, the field g can be shown to be g_end=[GM/(RL)][(2L/R)+½-½√(R²+L²)/R]. Let me first provide a qualitative argument that the field at the "poles" will be larger than at the "equator". At the equator, the contribution to the field from each piece of mass in one half will have a corresponding piece on the other side and their axial components will cancel out, leaving only the radial components. As long as L>R, there will be much less cancellation for fields at the "poles"; therefore, if the cylinder is not rigid, it will collapse to a sphere axially (from the poles). If L<R, this argument will work in the opposite way and you would expect the collapse to be radially in from the equator. Next, here is an approximate analytical solution for the case L>>R. The end fields can be approximated as g_end≈3GM/(2R²). At the equator, Gauss's law may be used to show that g_equator≈2GM/(LR). So g_end/g_equator≈3L/(2R)>>1. So, your expectation was right, but only if R<L. On the other hand, if R>>L, the field at the pole approaches the field at the center of a uniform disk which is zero by symmetry. So, whatever the field is at the equator, the force tends to collapse the cylinder radially (inward from the equator). The details of the calculations here are given in a separate page where I have also shown a rough sketch of the field for the L>R case.

QUESTION:
The faster you move, the more massive you get, due to E=mc2, right? But do I ACTUALLY get heavier? Does the chemical energy in my cells also have mass? So if I run, therefore spending some chemical energy, and gaining some kinetic energy, do I not weigh the same when standing still and when running? (Assuming of course perfect consumption of energy to move me).

ANSWER:

First of all, it is not due to E=mc², it is due to the redefinition of linear momentum in special relativity. There are lots of links on the faq which address the question of relativistic mass (1, 2, 3). Basically, think of mass as inertial mass, the resistance to acceleration. It is well known that objects cannot exceed the speed of light, no matter how hard you push them. Therefore, inertia must be increase as you go faster. In general relativity, Einstein was able to show that gravitational mass is identical. So you have more mass (and therefore weight) when you are running but by an immeasurably small amount (unless you can run at speeds near the speed of light). All your rambling about chemical energy, kinetic energy, etc. just confuses the issue you are either moving or you're not, don't worry about how you got there.

QUESTION:
Is there a way for water in a static position upwards to create a waterfall effect without the use of any machine or human involvement? Strictly just the water powering itself?

ANSWER:

It is not clear what you mean by "waterfall effect". Ancient Rome had fountains without machines. The trick is to have a reservoir of water higher up than the fountain. And, the water is not "powering itself", it is being powered by gravity.

QUESTION:
Approximately how many photons of sun light are in a square inch over one second? Assuming the sun is directly over head, clear day, standard earth atmosphere at sea level. How many photons in one square inch over one second from a one ~~foot~~ candle source at one foot and would it then be a simple matter of multiplication if I doubled the intensity of the source? The light is coming from a 575 w, 120 v, 3600 kelvin halogen lamp for the second question. I teach the art of lighting design for theater and dance.

COMMENT:

Reading below, you will see that there is an error in the questioner's units since the ft-candle is a measure of intensity, not of power. The source would be characterized by its power, namely a candle or a lumen (which is a fraction of a candle). So I have changed "foot candle" above to just candle.

ANSWER:

I have come to appreciate how convoluted measurement of light is! Furthermore, since the questioner asked for photons/in²/s, one runs into the problem that photons of different color (frequency or wavelength) have different energies. The energy e per photon of a particular wavelength λ is e=hc/λ where c=3x10⁸ m/s is the speed of light and h=6.6x10^-34 J∙s is Planck's constant. The Joule (J) is the SI unit for energy and is most familiar to nonphysicists as the amount of energy delivered per second by a power source of 1 W. So 100 photons of red light delivers much less energy than 100 photons of blue light because blue has a much shorter wavelength. Light intensity is energy/second/area, so equal photons are not equal intensities. Almost any source of light will contain a whole spectrum of light. The spectra of both the sun and the lamp in the second part of the question are well-approximated as black bodies. The curve to the left labelled 5000 K is what the radiation from the sun looks like as a function of wavelength. It peaks at about about λ=555 nm (0.555 μm), yellow. One can find the maximum intensity wavelength (in meters) for any absolute temperature T black-body spectrum using Wien's law, λ_max=2.9x10^-3/T. So the peak for the halogen lamp in the second part of the question is λ_max=2.9x10^-3/3600=810 nm, in the infrared. The problem is that all the "photometric" units (the candle and everything derived from it) are defined in a way which incorporates the sensitivity of the human eye; "radiometric" units are more what a physicist would prefer, the raw data as it were. It is very difficult to unfold one to the other. I will do my best!

To estimate the photon flux I will assume that all the sun's light is at 550 nm. Now, I found that the average intensity of the sun at the distance the earth is from the sun is about I=1367 W/m². Using the expression above, the energy of a yellow photon is e=3.6x10^-19 J/photon. Therefore the flux of photons is Φ=I/e=3.8x10²¹ (photons/s/m²)(1 m²/1550 in²)=2.45x10¹⁸ photons/s/in²). To get a more accurate estimate of the photon flux would involve very complicated mathematics to get the net flux from the continuum of wavelengths, but this calculation certainly gives a reasonable order-of-magnitude estimate.

Another way to approach this is to express the intensity in lux, I=110,000 lux for bright sunlight. Using an online converter to SI units, I=0.16 W/m². Why is this so different from the value of 1367 W/m² above? I believe it is because the lux includes only the visible light which is a very tiny slice of the whole spectrum. So this is probably a much more useful way for the questioner to approach this problem. The photon flux then (maybe we should call it "visible photon flux") is approximated as Φ_visible=I/e=4.4x10¹⁷ (photons/s/m²)(1 m²/1550 in²)=2.9x10¹⁴ photons/s/in².

The second part of the question is trickier because of the units used. The "candle" is a unit measuring power (like a Watt), energy/s. It was historically based on the brightness of a single candle. However, as you get farther away from a source, its brightness gets smaller, so we introduce a quantity usually called intensity or flux which is indicative of energy at some distance passing through some area in one second. The usual unit is the lumen (lm), 1 lm=1 candle∙1 ft²/(4πr²) where r is the distance from the source to where the intensity is measured. Knowing that 4πr² is the area of the sphere with the source at the center, a lumen is seen to be the fraction of the total rate at which energy passes through the 1 ft² at the distance R. [This can be more elegantly expressed by defining a sterradian (sr), the three dimensional analogue of angle called a solid angle, Ω=A/r² (see picture at the right). So you can write 1 lm=1 candle∙Ω/(4π).] So the lumen is also a measure of power but only a well-defined fraction of the total power. The foot-candle is defined as 1 ft-candle=1 lm/ft²; the metric equivalent is the lux, 1 lux=1 lm/m². The ft-candle and lux are both measures of intensity, power/area.

Now to the second part of the question. For a distance of one foot from a 1 candle source, the intensity is 1 ft-candle=10.76 lux. Now, as above, convert this to Watts/m² using the online calculator, I=1.6x10^-6W/m². And so, Φ_visible=I/e=4.4x10¹² (photons/s/m²)(1 m²/1550 in²)=2.9x10⁹ photons/s/in². In this case, I have again used the 555 nm conversion which might not be appropriate for an 810 nm max lamp, but I suspect it may be ok for you since you are interested in visible light.

Regarding your question about doubling intensity, if you double the intensity of the entire spectrum uniformly, then there would be just a doubling of the number of photons. If, for example, you change intensity by increasing the temperature of the lamp, the distrubution would change so that all bets are off.

This may be a long, rambling answer, but I had to learn a lot as I went along. Keep in mind that the photon fluxes are order-of-magnitude, not exact.

QUESTION:
I'm wondering about whether it would be possible for human activity, given current technology, to move a moon such as Europa or, better yet, Ganymede out of Jupiter's orbit and into a solar orbit (down the road, ideally it would be nice to have it impact with another planet with water resources for, you guessed it, terraforming). The parameters of this situation are as follows. Assume cooperative human action which would allow the use of nuclear weapons on the far side of the moon to push it away from Jupiter, but at regular enough intervals to keep it from exploding. Assume also any "tractor" technologies, such as a large, solar-powered spacecraft which uses (some of) the electricity created by the solar panels to create lasers which push it away from this moon. Am I right in thinking that either or both of these techniques this could possibly move an "earth-like" moon into the habitable zone, eventually?

ANSWER:

I am not going to address any of your hypothetical scenarios for delivering the necessary energy because, as I shall show, this is not going to work for any "current technology" you wish to put into play. The energy necessary to remove Ganymede (M_G≈1.5x10²³ kg) from its orbit (R≈10⁹ m) around Jupiter (M_J≈2x10²⁷ kg) is E=GM_JM_G/R≈2x10³¹ J, where G=6.67x10^-11 J∙m/kg² is the universal gravitational constant. Suppose that you wanted to achieve this over a period of T=100 years≈10¹⁰ s. The required power would be P=E/T≈2x10²¹ W=2,000,000,000 TW. The current average power consumption of the entire earth is about 15 TW. (By the way, Ganymede is not really "earth-like" at all since the acceleration due to gravity at its surface is only about 1/5 of earth's.)

QUESTION:
Why kinetic theory claims that free atoms do not have a rotational kinetic energy component while macroscopic spheres obviously have? Is it because a rotational component does not transmit during elastic collisions and hence is not distributed?

ANSWER:

Single atoms do </p>
not have rotational degrees of freedom; the reason is that they are quantum mechanical objects which are spherically symmetric. In that regard, they are not well modeled as rigid spheres. Molecules, however, being not spherically symmetric, do have rotational (and vibrational) degrees of freedom which must be included in kinetic theory of gases to get correctly describe the thermodynamic properties.

QUESTION:
I have heard this so many times "photons see eternity pass in seconds." Photons travel at the speed of light dosen't that mean that time stops for the photon since the photon isn't travelling through time at all so how does a photon see eternity pass at all isn't it frozen in time. Do I have it messed up? Or is it just something people say out of ignorance?

ANSWER:

This is essentially the question I frequently get about what would we experience from the point of view of a photon. Since there is no way a photon can carry a clock or a measuring stick with it, it is safe to say that it does not have a point of view. In other words, trying to imagine what a photon would experience is meaningless.

QUESTION:
Since the big bang have any atoms been added or removed from the universe? If nothing has been added or removed does that mean we may have atoms of people who may have died before?

ANSWER:

Atoms are being created and destroyed continually, mainly in stars. What has remained constant, we believe, is the total amount of energy in the universe and the mass in atoms is just one kind of energy. Regarding your second question, atoms are being continually recycled by biological processes on earth; see an earlier answer.

QUESTION:
Why is Fusion so hard to crack and when can we expect fusion to replace coal and oil?

ANSWER:

Fission is fairly easy because all you have to do is "tickle" a heavy nucleus and it will split in two; this is usually achieved by adding a slow neutron to the nucleus which is easy to do because it has no electrical charge and therefore does not feel any repulsive force from the nucleus. Fusion, however, involves bringing two positively charged light nuclei together. Since they repel each other, they can only get close together if they are going very fast. Another way of saying the same thing is that the fusing target material (generally isotopes of hydrogen) must be very hot. Containing a hot enough gas (plasma, actually, since the high temperature will cause the atoms to be ionized) has turned out to be an extraordinarily difficult engineering problem. There is an old saying among physicists, tongue in cheek, that "fusion is the energy of tomorrow and it always will be!"

QUESTION:
Why Is Galliean relativity wrong?

ANSWER:

Because it makes incorrect assumptions about space and time. For example it assumes that clocks run at the same rate regardless of their motion.

QUESTION:
If you spin a rod each end turns at the same time, now if you spin a laser does the beam change at each end at the same time or is it limited to the speed of light?

ANSWER:
I am not sure what you mean by "at each end" and "turns at the same time". However, just imagine a very long stick and a beam of light. If you shine a laser beam on the moon and move the laser, the spot on the moon will move faster than the speed of light if you are rotating the laser fast enough. If you have a stick which reaches the moon, its end can never exceed the speed of light.

QUESTION:
Is any body able to exert force of magnitude greater than its product of mass and acceleration? For example in case of action and reaction how can small body exert force equal to the action of large body?

ANSWER:
A one pound steak sits on my plate. Its acceleration is zero. It exerts a one pound force down on my plate. The plate, weighing only a half pound, nevertheless exerts a force on the steak of one pound upwards.

QUESTION:
lets speculate that protons fundamental charge and proton's (rest) mass would have slight variation such that for single particle m'/m0 = e'/e0 = L where L is around 1 but not exactly one. -but the charge/mass ratio of proton would be exactly constant: m'/e' = m0/e0 . how easy would it be to show that ordinary would have this variation in physical experiments and that there are some protons that have different mass and charge among the matter? or prove that protons in matter cant have any such mass and charge variation?

ANSWER:
There are too many things which depend on only the mass or only the charge of a proton for this speculation to be possible. For example, an atom containing your irregular protons would not be electrically neutral.

QUESTION:
Is the twin paradox incorrect? How can it be that a person moving relative to another person will experience time slower, because from the moving persons frame of reference, the other person is moving, and therefore should experience time slower as well.

ANSWER:
Do you suppose that is why they call it a paradox? The two are not equivalent because one clearly is the one who must put on his brakes and turn around. You can see a full explanation in an earlier answer.

QUESTION:
Why does the water go down the drain anti-clockwise in northern hemisphere?

ANSWER:
Because of the Coriolis force.

QUESTION:
In case of electron the total energy will be equal to half m v square but it will also be equal to m c square according to Einstein's equation but substituting these we get v of electron more than c(speed of light) about root 2*c what do this mean

ANSWER:
What it means is that you do not understand what mv² and mc² mean. K≈ mv² is the kinetic energy of in classical mechanics of a particle of mass m and speed v; it is only (approximately) true for v<<c. E=mc² is the energy which a mass m has, by virtue of its mass, when it it at rest. The correct kinetic energy of a mass m with speed v is K=mc²[1/√(1-v²/c²)-1]. It is fairly easy to show that, if v²/c²<<1, K≈ mv². Letting β=v/c,

1/√(1-β²)-1=(1-β²)^--1≈1+(- )(-β²)/1!+(- )(- -1)(-β²)²/2!+ -1≈ β²

K≈mc²( β²)= mv².

QUESTION:
how does popcorn pop?

ANSWER:
See this link.

QUESTION:
Built my kid a marshmallow shooter for a science project. Simple design. Main air chamber is 2" pvc with a total length (including bend) of 32". It is then directed into a 3/4" inch pvc pipe with 2 valves. The first valve (1) is the main shut off valve and inch or two from the 2" chamber. Then there is another 21" of 3/4" pvc into the 2nd valve (2). The barrel is 1/2" pvc and 24" long. We can fill the gun with 40 psi with both valves closed. We then open valve 1. Pressure should drop a little, but not much. We then close 1 to preserve pressure and shoot marshmallows with almost 40 psi. They will go 40'. When we fill the tank with 40 psi with valve 1 open and valve 2 closed and shoot it, it'll shoot the marshmallow 100' or more. My question is: why is releasing all the air at once shooting the marshmallows further? It's driving me nuts. Is it the volume of gasses? How can I mathematically solve this mystery?

ANSWER:
It is pretty easy to understand this qualitatively. As the marshmallow moves down the barrel, the volume of the gas behind it increases so the pressure decreases. Your first case (valve 1 closed) there is a pretty big fractional increase in volume so, since PV is constant, a pretty big decrease in the final pressure; in the second case (valve 1 open) there is a much smaller fractional increase in volume so there will be a much smaller decrease in the final pressure. The average force felt by the marshmallow over the length of the barrel will be bigger for the second case.

Now, let's do it analytically. I will ignore the couple of inches between the main chamber and the first valve. The operative principle is that if temperature and amount of gas are unchanged, the product of the pressure and volume is a constant or, equivalently, V_initial/V_final=P_final/P_initial. An important thing to keep in mind is that 40 psi is the gauge pressure, the pressure above atmospheric pressure which is about 15 psi; so P_initial=55 psi. The volume of each section is π(d/2)²L where d is the inner diameter of the pipe (a 3/4" pipe, e.g., specifies the diameter). So V_barrel=4.7 in³, V_primary=100 in³, V_secondary=9.3 in³. In the first case V_initial=V_secondary=9.3 in³ and V_final=V_secondary+V_barrel=14 in³. Therefore V_initial/V_final=0.66=P_final/P_initial and, taking P_initial=55 psi, P_final=36 psi and the corresponding gauge pressure is 21 psi; so the average gauge pressure during firing was (40+21)/2=30 psi. In the second case, V_final=V_secondary+V_barrel+V_primary=114 in³ and V_initial=V_secondary+V_primary=109.2 in³. Going through the same procedure as the first case, P_final=53 psi and the corresponding gauge pressure is 38 psi; so the average gauge pressure during firing was (38+40)/2=39 psi. This means the average force on the marshmallow was 81% greater for the second case; this means that the speed of the marshmallow is almost twice as great, so it is roughly in agreement with your measurements of a distance of 100' compared to 40'. I have not considered air drag during the flight after leaving the gun which will be fairly important for a marshmallow. Also, I have ignored friction between the marshmallow and the barrel; because of this and neglect of air drag, don't expect real good quantitative predictions of range.

ADDED NOTE:
In the first case where you pressurize to 40 psi and then open valve 1, the pressure will drop more than just a little as you expect. V_initial/V_final=100/109.2=0.92, so P_final=55x0.92=51 psi, so the final gauge pressure will be about 36 psi, about a 10% drop.

QUESTION:
There's a weight standing equally on 2 legs, each of which is on a scale. Each scale shows half the weight. If we disappear one of the legs (assume it' stable and won't fall over.), the entire weight should transfer to the remaining leg. Does it transfer instantly, or is there a time lag? If there's a time lag, why? I say there's a time lag (but I can't explain why) and my friend says it's instant.

ANSWER:
Generally, nature does not like discontinuities so you should always assume first that "instantaneous" is not possible. That, indeed, is true for the situation you describe. The two-leg scenario has some problems which complicate things (like the center of mass is not directly over either scale so removing a leg will result in a torque trying to tip the object over). So, I will use a simpler problem, equivalent for your purposes. Imagine that a weight sits on a scale. Now you place a second identical weight atop the first. The information that the second weight has been added gets transmitted at the speed of sound (in the first weight) to the scale. This will still be a pretty short time, but not zero. I have ignored the time it takes the scale to respond.

QUESTION:
Does special relativity theory seriously claim that there is no objective physical world independent of how variously it might be observed, as from frames with relativistic velocities? In other words, do physical objects and the distances between them physically contract? If not, why not clarify that observer-dependent measurements only show *apparent contraction,* not physical "shrinkage?"

ANSWER:
What does it mean to "physically contract"? If you mean is it the same as if we put it in a vise and squeeze it, no, it is not the same. In the vise case, the object gets shorter in its own frame whereas something going past you at some high speed will still be its original length in its frame but shorter in ours. In physics it is important to have objective, not subjective, definitions of what we mean by something like length. Here is what length means in physics: you measure the positions of the ends of the object at the same time and the difference is the length. I think you would agree that this is a perfectly reasonable definition of length. It is not that things appear shorter, it is that things are shorter. You might be interested in an earlier answer.

FOLLOWUP QUESTION:
If a frame of reference approaches Earth at 86% of light speed, special relativity claims it will measure its diameter to be about 4000 miles, half that established by earth science. Please answer my question about *apparent* contraction vs. *physical* contraction. Earth's diameter obviously does not contract to 4000 miles or other variations according to different speeds and directions.

ANSWER:
It is clear to me that you have made up your mind. I cannot imagine that you could have read my answer and have written what you have. Normally, I would not have bothered to answer, but let me try one more thing. The diameter of the earth is about 12.7x10³ m and the 0.86 c is about 2.6x10⁸ m/s. Special relativity says that the diameter, as measured by the moving frame is 12.7x10³√(1-0.86²)=6.5x10³ m. Now, the time which the moving frame observes that it takes for the earth to pass is 2.5x10^-5 s. If the diameter of the earth was 12.7x10³ m, the time would have been 4.9x10^-5. That is how long an observer on the earth observes the moving frame to pass the diameter of the earth. Both length and time depend on your reference frame. Experiments equivalent to this have been done and everything I have written in this paragraph has been experimentally verified. (If you had read my original answer, you would have seen that I did answer your question about apparent vs. physical contraction.)

QUESTION:
In a theoretical universe, only two hydrogen atoms exist. At time=0, they are 100 light years apart from one another. How much time will elapse before they are physically "aware" of each other?

ANSWER:
I guess you mean that they have just "popped into existence". They each have mass, so they can interact gravitationally. It is believed that a gravitational field propagates at the speed of light, so it would be 100 years before they were "aware". A hydrogen atom is electrically neutral and is spherically symmetric, so they will not interact electromagnetically at such a distance.

QUESTION:
Does the Heisenberg uncertainty principal say that getting a precise measurement violates the rules of physics or we just don't have the technology to take the measurement without disturbing the article.

ANSWER:
The laws of physics say that there are some things (like position, momentum, energy) which you cannot know to absolute precision. No technology can defeat this.

QUESTION:
Can momentum of light be increased?

ANSWER:
Yes. For example, a photon falling into a black hole gains energy as it falls. Its energy E is given by E=hf and its momentum p is p=E/c. Therefore, the photon gains momentum by increasing its frequency f.

QUESTION:
2 glasses of water - same volume, same temp, same container characteristics - one placed in 31.5 degree temp and one in 0 degree temp. Does one of them freeze more quickly than the other and if so, why.

ANSWER:
I am assuming you mean the whole volume freezes. Most of the heat lost by the water will occur as conduction through the glass. The rate of heat transfer through some barrier is proportional to the temperature difference across the barrier. Therefore the water in the 0⁰F environment would freeze first.

QUESTION:
Why is it that when my daughter spun the lazy Susan on our table, the salt shaker went flying off?

ANSWER:
An object which is going in a circle requires a force to keep it going in a circle. Imagine that you are spinning a stone attached to a string in a horizontal circle. The force which keeps it going in a circle is the string pulling on it. As you make it go around faster and faster, the string has to pull harder and harder. Eventually the string can no longer pull hard enough and breaks and the stone goes flying away. When the lazy Susan is spinning the salt shaker is moving in a circle and therefore needs a force to keep it going in a circle. The force which keeps it going in a circle is friction between the lazy Susan and the shaker; if the lazy Susan were very slippery, it would not be of much use. As it spins faster and faster, you will need more and more friction but there is a limit to how much friction you can get; think of trying to push a heavy box on the floor you push harder and harder and eventually it will start moving. So, at a high speed the shaker will fly off. Tell your daughter to not spin it so fast! Or maybe she just likes to make it fly off. Good thing it wasn't a glass of milk.

QUESTION:
Me and a friend want to create a kind of motor that could make wheel turn without electricity or fuel ... could you help us ?

ANSWER:
How about a windmill or a water wheel?

QUESTION:
When a gas is pressurized (in favorable conditions) it turns into liquid. Further pressurizing it turns into solid. Now I exert larger pressure on a uniform solid (form all directions). What will happen? Also what will happen if I subject a fundamental particle to very large pressure?

ANSWER:
Very large pressures can change the properties of the material and new phases may occur (like water and ice are two different phases of H₂O). A particularly good example is water itself as shown in the figure to the right; pressures up to about 10,000,000 atmospheres are shown in the graph and numerous phases beyond ice/water/steam are seen at high pressures or very low temperatures. For higher yet pressures, you need to look to stars. If a star is massive enough it will, after going through a supernova stage, collapse under the pressure of its own gravity to where electrons are pushed into the protons and the whole star becomes a neutron star, essentially a gigantic nucleus. If heavy enough, it will continue collapsing into a black hole. What individual particles do depends on the pressure and they essentially will retain their identities or undergo reactions with other particles (as in the neutron star formation) or lose their identities (in a black hole).

QUESTION:
In a book I have found somewhere that "photon theory is failed to explain the phenomenon of interference or diffraction" that means in photon theory there is nothing related to WAVE! And photoelectric effect or Compton effect is based on quantum theory so there should be also nothing related to WAVE!! But in these effects the term "frequency "is used which is related to WAVE!!! I know what is duality and I don't think so it can be answered through duality!!!

ANSWER:
You are pretty handy with an exclamation point!!!!! I do not know what book you found, but you have the whole idea of duality misunderstood. Duality does not mean that a photon is either a particle or a wave, it means that a photon is both a particle and a wave. Whichever you look for you will find. And when you say that "quantum theory [is] nothing related to a WAVE!! ", you are mistaken. Another name for quantum mechanics is wave mechanics and particles are described by wave functions. It is customary to talk about the frequency of a photon since frequency f is synonymous with energy E through the relation E=hf.

QUESTION:
An astronaut rotates in space an observes the universe rotating about them. How does the astronaut know whether the universe is rotating, or if it is their own motion causing that visual spin? Centripetal forces, of course, which will force blood into the fingertips which will surely be detectable in the astronauts frame of reference. Perhaps this suggests that there exists a universal state of rotational rest - detectable by measuring existence or absence of centripetal forces. Is there also a more general universal state of rest? A state that can be also measured and agreed on by any stationary or moving frame of reference? What do we already know about that has a velocity that can be measured and agreed on by all reference frames, even those with different relative velocity? What do we know of that, like zero, has an ultimate limit that cannot be exceeded? Could the speed of light actually be that universal rest frame?

ANSWER:
First of all, it is centrifugal force, not centripetal, which causes blood to be pushed to extremities. But that is not important here. There are two kinds of reference frames, inertial and noninertial. If you are in an inertial frame of reference, Newton's laws of physics are true. A noninertial frame is any frame which accelerates relative to any inertial frame. Because Newton's laws are not valid for your rotating astronaut, blood flows out to the fingertips even though there is nothing pushing it out; that is why you will see centrifugal force referred to as a "fictitious" force. There is no preferred reference frame, no "absolute at rest."

QUESTION:
I am reading a scifi novel and in it gravity is simulated by constant thrust from the engines. If there is no inertia in space would not the spacecraft continue at that speed and therefore the gravity remain constant until the ship used thrusters to slow it down? In the novel, if the thrust is cut off the gravity is reduced. I can't get my head around it?

ANSWER:
When you are in a car which is accelerating you feel as if you are being pushed back against the back of your seat; this is like a horizontal "artificial gravity" and is the effect which causes the artificial gravity when your spacecraft is burning its engines. When the car stops accelerating and moves with constant velocity in a straight line, you no longer feel that you are being pushed back; similarly in the spaceship when you cut the engines. I have no idea what you mean that there is no inertia in space. Inertia means resistant to change in velocity if acted on by a force, so if the engines are not burning you feel weightless; gravity is not "reduced", it disappears. It sounds like you think that you need to push on the spacecraft to keep it going with a constant speed and nothing could be further from the truth. Newton's first law (the law of inertia) says that an object on which no net force acts will move in a straight line with constant speed forever.

QUESTION:
My question is about refracting of light in our atmosphere. Why doesn't the sun look red during the day? The sun's light is strongest in the yellow wavelengths. When the blue light is scattered out by particles in the atmosphere, it seems like the sun should appear red. But the sun still looks yellow during the day, and only looks red during a sunset. Why?

ANSWER:
During the day the light from the sun passes through much less air than at sunset or sunrise. The fraction of the total light coming to you during the day which has scattered is much smaller when the sun is overhead.

QUESTION:
I want to know is there any expression for relativistic force as we have for momentum?

ANSWER:
In classical mechanics, force F only only has meaning in terms of the acceleration a it causes some mass m to have, F=ma. This can be more generally stated as the force is the time rate of change of linear momentum, F=dp/dt. Using the relativistic expression for p, this defines force in special relativity. Usually, though, it is much more useful, both in classical mechanics and relativistic mechanics, to solve problems using the potential energy V(r) associated with a force, V(r)=-∫F∙dr.

QUESTION:
What causes the birth of a black hole?

ANSWER:
When a star has exhausted most of its hydrogen fuel, it begins to collapse under its own gravity. The next thing that happens is that it explodes, a supernova. Then, providing that the star was massive enough, it will continue collapsing until it has essentially collapsed into zero volume, a black hole.

QUESTION:
Have you ever heard of internal conversion in radioactive decay (if not I'm fine with that) because a guy on the internet told me that internal conversion happens when atomic nuclei GAIN energy yet sources I read say that it's another way that excited nuclei LOSE energy without emitting gamma rays, is he correct?

ANSWER:
I would be a pretty poor nuclear physicist if I hadn't heard of internal conversion! It is an alternative to γ-decay of an excited nuclear state. The nucleus certainly does not gain energy since the net result (for the nucleus) is a loss of energy as it makes a transition to a lower-energy state. The energy is carried of by the kinetic energy of an atomic electron which is ejected in the process.

QUESTION:
If a gun is fired in space, how far/long will the bullet travel and how long will it spin? Why? Assumptions: 1) gun s position is fixed relative to the earth; 2) gun s barrel is rifled, thus the spin; 3) bullet travels in unobstructed straight line and it avoids being attracted to another body due to that body s gravity. In one of your earlier answers regarding the relative motion of a gun and bullet in space you say that the bullet would never stop traveling. I suppose that, in my question above, this also means that the bullet would spin forever as well? This confuses me as it would seem to me that motion requires energy, which would eventually be depleted. What am I misunderstanding?

ANSWER:
I will first answer your last question. What you are misunderstanding is Newton's first law which states that an object which experiences no net force will move in a straight line with a constant speed; also, a rigid body which experiences no net torque about its axis of rotation through its center of mass will continue rotating with the same rotational speed about that axis. With these you should understand the answer to my previous answer and also why (your addition) it will spin forever. The only proviso is that there is nowhere in the universe where there is a perfect vacuum, always a few atoms around, and so there is always a tiny amount of friction which would stop your bullet after maybe billions of years. (Also, your supposition that " it avoids being attracted to another body due to that body s gravity" cannot be literally true because gravity is everywhere.

QUESTION:
In nuclear fission and fussion there is no change in mass of reactant and product so from where does the 200 MeV energy come from?

ANSWER:
The questioner included the figure above as an example. The total charge (92) and mass number (236) before and after this fission reaction are the same. However, this does not mean that the total mass is unchanged. If you look up all the atomic masses involved in this fission, you will find that the mass is less after the fission. To understand why, see an earlier answer.

QUESTION:
Where do atoms get their energy?

ANSWER:
What energy are you talking about? Of course, an atom has mass energy, mc²; if you just look at a single atom at rest in its ground state, that is all the energy it has. But here is the interesting part: If you take an atom, pull out all its electrons, protons, and neutrons and weigh them, they will weigh more than the atom had before you pulled it apart. So, if all these pieces are at rest and far apart, you have more energy than the atom had. The reason is that you did work to pull it apart and thereby added energy which showed up as mass energy. So your question should really have been "where did atoms lose their energy?"

QUESTION:
This link is a video of anti gravity and I want to know on how it really works?

ANSWER:
This one is pretty easy to debunk, I believe. The guy and all the apparatus are upside down; the camera taking the video is also upside down.

QUESTION:
If you have 2 plastic balls with the same outer surface but one is filled with water and the other one is empty and you released them, from rest, down the same slope, the ball with the water would accelerate more due to viscous flow right? If that is the case then would a ball that is fairly (but not completely) filled with sand accelerate faster than the empty ball and why? would there be a similar effect?

ANSWER:
There is no clear cut simple answer to this question. I prefer to use energy conservation to look at problems like this. Let's first just consider a hollow sphere and a solid sphere. If we have an object with mass m, radius R, and moment of inertia (about center of mass) I, it is easy to show that the speed of the object when it has rolled down so that its vertical drop is h is given by

v=√[2mgh/(m+(I/R²))].

The moments of inertia are
I_solid=2mR²/5 and I_hollow=2mR²/3.
First note that the mass will cancel out, so
v_solid=√(10gh/7) and v_hollow=√(6gh/5)<v_solid
and so the solid sphere is the winner.

Now, suppose a hollow sphere, mass m, radius R, moment of inertial I=2mR²/3 is filled with a fluid of mass M. (I assume that the spherical shell is very thin compared to the radius of the ball.) There are two extremes of what can happen, either the fluid does not rotate at all as the ball rolls (a superfluid) or else it begins rotating with the ball almost immediately (maybe molasses). In the first case, when the ball reaches the bottom its rotational kinetic energy will be all due to the rotating of the ball, its translational kinetic energy will be due to the both the ball and the fluid, and the potential energy at the beginning will be due to both:

(2mR²/3)(v²/R²)+ (M+m)v²=(M+m)gh.

Solving, I find
v=√[2gh/(M+(5m/3))];
notice that if M=0, v=v_hollow as it should, so any nonzero M will result in losing to the hollow sphere. At the other extreme, the fluid rotating rigidly the whole way, the energy conservation equation will be

(2MR²/5)(v²/R²)+ (2mR²/3)(v²/R²)+ (M+m)v²=(M+m)gh.

Solving, I find
v=√[30(M+m)gh/(24M+25m)].
This can be compared with v_hollow:
v/v_hollow=√[(1+(M/m))/(1+(24M/25m))]
which is always greater than 1 (the denominator in the square root is smaller than the numerator). Again, note that for M=0, v=v_hollow. Therefore the filled ball will always win if the fluid rotates.

Now, to your question. Water will be at neither of the extremes above. It will begin by not rotating, so in a race with a hollow ball, the hollow ball will jump off to a lead. After some elapsed time, all the water will be rotating with the ball containing it, so it will be now be gaining speed faster than the hollow ball and be catching up. Eventually, the water ball will catch up and pass the hollow ball; so, you see, which ball wins will depend on how long the race is. The way you state the sand part of the question is problematical " fairly (but not completely) full " is not very quantitative. Let's just say that it essentially fills the ball but is loosely enough packed so that it can slide over itself. In that case, the sand will act pretty much like a very viscous fluid and will get fully rotating with the ball more quickly than the water did. In both cases (water and sand), trying to do a analytical solution while the fluid is in the transition stage from not rotating to rotating is just about impossible; because of the effects of friction (viscosity, if you like), energy is not conserved during that time.

QUESTION:
What is the direction of the gravity force towards object? Is it radial to center or normal to cross section? Is there any experiment done from space to check whether it is normal to cross section?

ANSWER:
The direction of the force (on a point mass) is in the direction of the gravitational field. If the object is spherically symmetric, it is in a direction which is both radial and normal to the surface. In general, the direction of the field depends on the the mass distribution and might be neither radial nor normal to the surface.

QUESTION:
Why do we multiply 1/2 in kinetic energy equation? Fundamental explanation expected and not by using Newton's equations.

ANSWER:
We do not multiply the in, it appears when kinetic energy is calculated. And it is ridiculous to say that you want to see an explanation." not by using Newton's equations " Everything about classical mechanics is Newton's laws. The derivation is here.

QUESTION:
If you were able to experience a time dilation field and you were to place a living organism half way in to the field, what would be the hypothetical result?

QUERY:
What is a time dilation field?

REPLY:
It's a field where inside it, time is either going by super slow or super fast relative to outside the field. It is used in scifi quite a bit so I'm not sure if it's just science fiction or if it is theoretical

ANSWER:
There are two ways you could achieve this. First, have a very fast-moving spaceship. Inside the time would run much slower than outside. Second, you could have a region of extremely intense gravitational field adjacent to a region of very weak gravitational field; I cannot imagine how you could achieve this. In the first case, how are you going to have your organism half moving and half not? In the second, the strong gravitational gradient across the boundary between weak and strong gravitational fields would rip the organism apart before time could make any difference.

QUESTION:
If you were standing on a flat platform that was free falling from the sky let's say 12000 feet up and right before impact within a certain point, or say within 12 feet, and you jump vertically off the platform, what would happen to you? Would it decrease your speed and your impact or would it just delay you hitting the ground? This question was asked to me by my friend and we both are very curious. We have a few thoughts on the outcome but aren't sure and we really want to know for sure. Hopefully you will be able to answer this for us thank you.

ANSWER:
I answered a similar question in an old answer, jumping in a falling elevator. There is no way that jumping can save you because the amount of upward speed you could give yourself is tiny compared to your downward speed. What would happen depends on the mass of the platform. If the platform had much less mass than you, most of your effort would be to speed up the platform rather than slow you. If it were much heavier than you, you would still only be able to reduce your downward speed by about 3-4 m/s. Of course, if the platform were big enough it would act like a parachute and you would not have to worry about a thing!

QUESTION:
Is this a good way to explain Lenz's law moving magnetic field around a conductor can produce a current. This current is opposing the magnetic fields that created it.

ANSWER:
No, that is not a good way. The induced current tends to have a field which opposes the change in the magnetic field which created it. For example; if the inducing field is increasing, the induced field would be in the opposite direction, and if it were decreasing, the induced field would be in the same direction.

QUESTION:
I am building a customizable "cat highway" of wooden shelves in my living room. The issue I am having is figuring out how much holding force I need, rather than how much weight the actual shelf can support. I know the shelves are plenty strong enough. Now I need to have them fastened strongly enough to the wall. It would be easy, if all I had to consider was the maximum weight a shelf has to take, if all three cats were to lie on it at once. However, I also need to how much weight I have to budget for for impact force - both from a descending and an ascending cat. As there isn't room for multiple cats to jump up or come down at one time, so only the weight of the heaviest cat (3.26587 kg) needs to be used for the calculations. Also, there will not be a height of more than 0.5 m from one shelf to another, nor a distance of more than 0.5 m between one shelf and another. I can't guarantee that they won't skip a shelf, so it might be safer to double the maximum distances just to be safe.

ANSWER:
Normally I answer such questions by "you cannot tell how much force results if an object with some velocity drops onto something". The reason is that the force depends on how quickly the object stops; that is why it hurts more to drop on the floor than to drop on a mattress. In your case, however, we can estimate the time the cat takes to stop because we can estimate the length of its legs which is the distance over which it will stop. You are probably not interested in the details, so I will give you the final answer: assuming constant acceleration during the stopping period, the force F necessary to stop a cat of mass m, falling from a height h, and having legs of length ℓ may be approximated as F=mg(1+(h/ℓ)). E.g., if h≈0.5 m and ℓ≈0.1 m, F≈6mg, six times the weight of the cat.

FOLLOWUP QUESTION:
Thank you for your answer! I thought you might want to know that, unlike a lot of people, I AM actually interested in the details.

ANSWER:
OK, here goes: I will use a coordinate system with increasing y vertically upward and y=0 at the landing shelf. The cat will have acquired some velocity -v when his feet hit the shelf. Assuming he stops after going a distance ℓ and accelerates uniformly, we have the two kinematic equations 0=ℓ-vt+ at²and 0=-v+at. From the second equation t=v/a; so, from the first equation, 0=ℓ-v(v/a)+ a(v/a)²=ℓ- v²/a so a= v²/ℓ. Now, as the cat is landing there are two forces on him, his own weight mg down and the force F of the shelf up, -mg+F and this must be equal, by Newton's second law, to ma, so F=m(g+ v²/ℓ). This, by Newton's third law, is the force which the cat exerts down on the shelf. Finally, the speed if dropped from a height h is v=√(2gh), so F=mg(1+(h/ℓ)).

QUESTION:
In light of the recent deflated football scandal, is there a way to mathematically calculate the change in pressure as the temperature inside the ball changes? Would you assume the volume of the bladder inside the ball to change very little?

ANSWER:
Yes, the ideal gas law, PV/(NT)=constant where P is pressure (not gauge pressure), V is the volume, N is the amount of gas, and T is the absolute temperature. Assuming that V and N remain constant, P/T=constant. Let's do an example. Suppose that the ball is filled to a gauge pressure of 13 psi when the temperature is 70⁰F. The absolute pressure is 13 plus atmospheric pressure 14.7 psi, P₁=13+14.7=27.7 psi. The temperature in kelvins (absolute) is 70⁰F=294 K. Now suppose we cool the football to 10⁰F=261 K. Then, 27.7/294=P₂/261, P₂=24.6 psi, and the resulting gauge pressure is 24.6-14.7=9.9 psi. I guess it is important to fill the ball at the temperature at which it will be played.

ADDED NOTE:
An article in the January 30 New York Times came to essentially the same conclusion as I did here. My answer was posted on January 26. I was astounded to read in that article, though, that initial calculations by physicists had applied the ideal gas law using gauge pressure rather than absolute pressure! Shame on them!

QUESTION:
Imagine the inside of a spacecraft, in orbit, so astronauts experience weightlessness and things float with no friction etc (assume there is no atmosphere in the craft ...). A pencil is floating in mid-air inside the craft. An astronaut pushes (say flicks with a finger) the pencil on one of its very ends. This push will impart linear, rotational, or both types of motion to the previously stationary floating pencil? If the pencil should rotate, about what axis does it rotate and how is angular momentum conserved?

ANSWER:
There will be very small effects due to the fact that this is not true "weightlessness" in that the spacecraft is accelerating in a gravitational field and not of zero size. I take it that this kind of detail is not what you are interested in, that I can simply answer your question for the pencil being in empty space, initially at rest. I will suppose that the pencil has mass m, moment of inertial I about its center of mass which is a distance d from the end where a constant force F is applied, perpendicular to d, for a very short time t. Newton's second law for translational motion says that the impulse equals the change in linear momentum, Ft=mv; after the impulse, the center of mass will move in the direction that F was applied with speed v=Ft/m. Newton's second law for rotational motion says that the angular impulse equals the change in angular momentum, τt=Ftd=Iω; after the impulse, the pencil will rotate around the center of mass with an angular velocity ω=Ftd/I. A relationship between v and ω can be written as ω=mvd/I. You can also write the total energy of the pencil, the sum of translational and rotational kinetic energies, as E= mv²+ Iω².For example, if the pencil is modeled as a uniform stick of length L, I=mL²/12 and d=L/2, so ω=mv(L/2)/(mL²/12)=6v/L and E=3mv²/2; in this case, 2/3 of the kinetic energy is due to the rotation. Also, the speed u of the end of the pencil with respect to its center is u=Lω/2=3v. Regarding angular momentum, Iω, it is conserved after the initial "flick" because there are no torques on the pencil.

QUESTION:
What is the difference between muon and neutrino muon?

ANSWER:
A muon is a lepton, sort of like a heavy electron, and is unstable. It has a mass about 200 times greater than an electron. There is no such thing as a neutrino muon, you must mean muon neutrino. Neutrinos are particles with very small mass and are the product of many particle decays. There are three kinds of neutrinos, the electron neutrino, muon neutrino, and tau neutrino and each has its antiparticle, making six in all.

QUESTION:
If a train is traveling at 60 m/s and a person runs and jumps off the rear of said train (opposite direction of travel) at 3 m/s. Which direction would the person travel? Would they continue to move in the opposite direction of the train, move with the train at relative speed, or drop straight downward?

ANSWER:
Variations on this question are probably the most frequent question I answer. I always say that you always have to specify velocity relative to what. In your question, I take it that the velocity of the train relative to the ground is 60 m/s and the velocity of the person relative to the train is 3 m/s in the opposite direction as the train's velocity. The velocity of the person relative to the ground is 57 m/s in the direction of the train's velocity. An observer on the ground would see the person with a horizontal velocity of 57 m/s regardless of whether he was on the train or had jumped off the back. Once he is off the train he will begin falling and hit the ground with both horizontal and vertical components of his velocity. For example, if he starts at a height of 2 m above the ground, his vertical component when he hits the ground will be about 6.3 m/s so his total speed will be √(57²+6.3²)=57.3 m/s.

QUESTION:
How is Relativity Theory explain the different measure of time? for example: the Sun rotate around itself in 25 days ( depend on the stars observing ) But here on Earth we observe it about 27 days. and some of other lifetime of particles is like this, have a different time in space and in Earth.

ANSWER:
This has nothing to do with relativity theory nor with different rates of time. The reason we observe the sun to rotate with a longer period is that we are in an orbit revolving around the sun and that orbit is the same direction the sun is rotating about its axis. Think of it this way: if the sun rotated on its axis once every 365 days, we would observe it to not rotate at all. The different lifetimes of particles is a different phenomenon and results from their moving at speeds close to the speed of light; that is relativity.

QUESTION:
I am very curious - do different electromagnetic waves/frequencies affect each other in any way? If one photon hits another or if they pass each other, do they affect each other in any way? For instance, if I have a flashlight with a stream of light, and another flashlight with a stream of light shining perpendicularly through the first one, I know that the streams do not seem to affect each other in any way - but do they? In ANY way?

ANSWER:
Indeed, photons interact with each other. However, for all practical purposes, two flashlight beams are not sufficiently intense for there to be an observable rate of interaction. Physicists do study the interaction between two photons, though. One well-known example is the interaction of a high-energy photon with the electric field of a nucleus (and therefore a photon) to create an electron-positron pair.

QUESTION:
What is in a neutron that makes it different from a proton? Is it the stuff that makes up an electron?

ANSWER:
It has nothing to do with electrons. Neutrons and protons, often referred to collectively as nucleons, differ in their internal structure. A proton has two "up" quarks and one "down" quark (leftmost figure) whereas the neutron has two "down" quarks and one "up" quark (rightmost figure). Particles composed of quarks are called hadrons and include also mesons.

QUESTION:
If aluminium and copper pipes are of same length and diameter ... same magnet is dropped through them ...in copper it takes more time to come out of other end, i myself have done this... please answer why is it so?

ANSWER:
Aluminum has an electrical conductivity of about 3.5x10⁸ Ω^-1m^-1 and copper has a value of about 6x10⁸Ω^-1m^-1. Therefore, at any speed, the magnet will induce a larger current in the pipe for the more conductive copper.

QUESTION:
I have seen some questions on your site about the different weaponry in Science Fiction. You have mentioned in many of them that it is ok to use the KE= MV² on them because they are slow in terms of relativity. So my question is at around what velocity should the kinetic energy of an object be calculated with the relativistic kinetic energy calculation? Like I have heard that relativistic effects become noticeable at around 15% lightspeed, so around there? And at what velocity would you say that an object becomes noticeably relativistic as a rule of thumb?

ANSWER:
This depends on how noticeable is noticeable. Usually the gamma factor is used as a guide, γ=1/√[1-(v/c)²]. At v/c=0.15, γ=1.01; a nonrelativistic calculation would only have about a 1% error. The graph to the right shows that relativistic effects do not really start kicking in until around v/c=0.5.

QUESTION:
I was at a training and learned that the Andromeda Galaxy revolves around a black hole. Is that true of all galaxies?

ANSWER:
Astrophysicists believe that nearly all large galaxies have a supermassive black hole at their centers.

QUESTION:
Newton's third law of motion states that, "To every action there is equal but opposite reaction". That means if i throw a ball on a wall it will bounce back but then what happens to mud, if i throw mud on a wall then it does not it bounce back?

ANSWER:
The mud and wall still exert forces on each other during the collision. The force which the wall exerts on the mud causes an acceleration of the mud which has the effect of stopping it as per Newton's second law.

QUESTION:
Is there is any situation which fulfills the first condition of equilibrium but not second condition of equilibrium?

ANSWER:
Of course. Consider a uniform stick of which has opposite forces on its ends, both perpendicular to the stick.

QUESTION:
I understand the bullet leaving the gun at high velocity and bullet dropped from end of barrel at zero horizontal velocity hit the ground at the same time (assuming level ground etc.) but I have a different one. Two bullets leave the same barrel and we do not figure in anything other than level. One has twice as much mass as the other (so it needed more energy to get to the same velocity). So assuming they have the same shape and aerodynamics I think the air friction will act the same and they both hit the ground at the same time. But, others in my group think it doesn't seem right. What is the right answer?

ANSWER:
I do not understand your phrasing " leave the same barrel and we do not figure in anything other than level " I will just assume that the two bullets begin simultaneously at the same height with the same speed and in the same direction. The force of air friction is determined by the shape of the projectile, so each will experience the same force at any particular speed. But the force F on the heavier bullet will result in a smaller acceleration a than for the lighter bullet because of Newton's second law, a=F/m. Therefore the lighter bullet will slow down faster and hit the ground earlier. You can understand this intuitively with an extreme example. Imagine a spherical balloon and a spherical cannonball of the same size both projected horizontally at 100 mph. Which do you think will hit the ground first?

QUESTION:
I've have been researching Einstein's Theory of Relativity and I think I understand it now but I just want to make sure. Below is a basic explanation of what I think Relativity is about. Basically if a truck is going down a road at exactly 50km/h and on the trailer of the truck is a baseball pitcher, catcher and the coach with a speed gun. Provided that the truck continues straight and doesn't hit any bumps, the pitcher would throw a ball at 100km/h and the speed gun would read 100km/h relative to the pitcher, catcher and truck. BUT if there is someone standing on the side of the road with a speed gun, and they point it at the ball, there speed gun will read 150km/h because to the person standing still, the ball that is in the pitchers hand is already moving at 50km/h before he even throws it. Have I understood the theory correctly?

ANSWER:
This is not Einstein's theory of relativity, it is called Galilean relativity. For all intents and purposes, you understand Galilean relativity, but it is only a very good approximation to what really happens. If the speed of the truck and/or baseball were not small compared to the speed of light, Galilean relativity is wrong. See an earlier answer.

QUESTION:
I doing some work with model rockets and I am trying to understand more about the center of mass. Specifically what happens to the center of mass as more weight is added. I understand that the center of mass begins to move nearer to where the weight was added, but as I continue to add more weigh does the movement become larger or smaller? My observation is that it becomes larger, but I've read another document that suggest it should be come smaller. I'm having a difficult time finding other comments on the topic. I would appreciate any help you can provide. I'd like to understand if my observation is incorrect and if so - maybe understand why.

ANSWER:
Suppose the mass of the rocket before you start adding mass is M and that the center of mass of the rocket is a distance L away from where you will adding mass. If you start adding mass m, the center of mass will move away from M and toward m such that it will be a distance d from M. Then the equation for the center of mass is d/L=(m/M)/[1+(m/M)]. The graph to the left is for no mass added (obviously, d=0) up to m=M (d=L/2). To answer your question, note that as m increases, the slope of the curve decreases, that is the rate of change of position of the center of mass decreases. As m gets bigger and bigger, the center of mass approaches d=L, and so clearly it will the rate at which the position changes gets smaller. The graph on the right is plotted up to m=10M so you can see this happen.

QUESTION:
I'm doing an assignment on helicopter flight, and I'm a little confused about the Bernoulli principle. He said that if a pipe is bigger at the beginning and smaller at the end, the fluid traveling through the end of the pipe would have a lower pressure. This seems counter-intuitive. I would have thought that there would be more pressure on the fluid that's "squeezed in together". I don't think I fully understand the concept of pressure.

ANSWER:
Let's first write Bernoulli's equation, P+ ρv²+ρgy=constant. At any point in the fluid P is the pressure, ρ is the density, v is the speed, g is acceleration due to gravity, and y is the distance relative to some chosen reference point (above, y>0, below y<0). Maybe it would help you to accept this if I tell you that Bernoulli's equation is simply conservation of energy. Since To answer your question we can ignore the y part, it is a horizontal pipe, P+ ρv²=constant. You should also understand that this equation is exactly true only under idealized conditions:

An incompressible fluid (water is a very good approximation)

Laminar flow which means flowing smoothly, no turbulence

Irrotational which means there is never any local circulation like whirlpools.

There must be no viscosity or other kind or friction

What goes on around a helicopter blade satisfies none of these conditions! Nevertheless, Bernoulli's equation can be a very useful approximation to understand what is going on even if it is not exactly correct. The most important one for aerodynamics is that which you cite, that when v increases, P increases. I have struggled to come up with a way you could understand this intuitively, but don't seem to find a simple explanation. You should convince yourself that your intuition is wrong by doing some experiments. For example, notice that inside a moving car the smoke from a smoker is drawn through a cracked-open window because of the lower pressure outside where the velocity is higher. Or, do the old blowing on the piece of paper demonstration.

QUESTION:
I wanted to ask that how can we unify general relativity with electromagnetism? I want a mathematical answer which unifies the both fundamental forces of nature.

ANSWER:
Einstein spend the last 30 years of his life trying to achieve this and failed. So, I do not think I am going to be able to answer you!

QUESTION:
I remember from college the common explanation of why massive bodies cannot exceed the speed of light: as objects travel faster, they acquires more mass, and as they acquires more mass, they require more energy to accelerate further. At the limit of the speed of light, it would take infinite energy to accelerate the object. However, gravitational acceleration (in a vacuum) does not depend on mass--the energy required to accelerate an object still scales with its mass, but the gravitational force scales equally.

ANSWER:
Your supposition that gravitational acceleration is independent of mass is incorrect. This is only true at low velocities. To find out how a mass would move in a uniform gravitational field, see an earlier answer.

QUESTION:
I'm working on a story and I'm trying to give a correct scientific explanation I can as justification for the character's powers. My questions are regarding speed and its various effects. Considering a human of mass 60kg can move at the speed of 200 m/s to 300 m/s, and can accelerate and decelerate at the same rate as the acceleration of bullet fired at muzzle velocity (that is almost instantly)

What is the effect of drag at that speed on a human?
How much energy is required to gain this speed with the above mentioned acceleration and deceleration rate and weight moving for 50 meters?
What is the effect of G-force on the human?
How much kinetic energy will the human produce when moving at such speed?
Will the human be clearly visible (or observable) to another normal human, moving at such speed?

ANSWER:
This is really a ridiculously impossible scenario! I will assume that v=250. I will not include all the details of the calculations.

You can estimate drag force as F≈ Av² where A is the cross sectional area. If we take A≈2 m², F≈3x10⁴ N. With this force acting, the power (rate of energy loss) is about P=Fv=8x10⁶ W=8000 kW. This would cause him to burn up, I would guess. I estimate that, if there were not some force to keep him moving, he would lose 90% of his speed in about 4 s.

He has an energy of mv²=2x10⁶ J. The acceleration and distance are irrelevant.

I took an M16 rifle as an example. The muzzle velocity is about 1000 m/s and the barrel length is about 50 cm, so I calculated that the acceleration would be about 10⁶ m/s², about 100,000 g. The maximum which the human body can withstand for short times is about 10 g. The time to accelerate the bullet would be about 10^-3 s.

This makes no sense. The body has kinetic energy but does not produce it. Because of the drag, it loses kinetic energy to heat.

Your speeds are about the same as a commercial airliner, 500-600 mph, and there is certainly no problem seeing them.

QUESTION:
I have recently gained an interest in physics, and have been looking into it over the past year. One of the areas I found interesting was special and general relativity, as I had always known the terms, but had no idea what they involved. As I have put more thought into time dilation, I have come up with a scenario that seems to be a bit of a paradox, and am hoping you can clear some things up for me. The scenario I have thought up is that of a clock on a spaceship that is travelling at a significant percentage of the speed of light. This spaceship is also set up with a camera, recording the clock, that is broadcasting live to another ship that is motionless. I imagine the moving ship to be going in circles around the motionless ship, but I'm not sure what effect, if any, this would have. So my question is, would the broadcast on the motionless ship run at normal speed, or would it run slower? Would people in the motionless ship see the broadcasted clock running slower on their screens, or would it run at the same speed because it's a live feed? To me, it seems that the broadcast would run at the same speed, because the image transferred from the camera on the moving ship to the screen on the motionless ship would travel at the speed of light, causing no significant delay. Also The camera is on the moving ship, so it is motionless relative to the clock, so it seems it would record and broadcast the clock at normal speed. But if time is running slower on the moving ship, how would it be possible for the motionless ship to see the moving clock running at the same speed? Let's say, according to the ship in motion, this task lasted 2.5 years, but it ran for 5 years on the motionless ship. So if the live feed was running the whole time, what happens? The camera was broadcasting the whole time, but that is only 2.5 years for the moving ship and 5 for the motionless one. So the image would have to slow down, wouldn't it? I assume I'm missing something here, so I hope you are able to clear this up for me.

ANSWER:
If the clock moves toward or away, your question involves the question of how time is and how time appears and involves only special relativity. See an earlier answer. If the moving clock is moving in a circular orbit of radius R, it is more complicated and involves both general and special relativity. In that case there will be time dilation due to its speed, v, but also due to its acceleration a=v²/R . To calculate the time dilation due to the acceleration you would use the equivalence principle and say that it would be the same as the gravitational time dilation in a gravitational field with acceleration g=a=v²/R. For the orbit, there would be no difference between how time is and appears to be because the distance from observer to clock is constant. Questions like yours must be considered if GPS systems are to be accurate.

FOLLOWUP QUESTION:
Thanks for answering my question, you helped me to better understand what would be happening to the ship in orbit, so now I see that there would be more at play in that scenario. However, you have not answered my main question. My main concern was what would show on the stationary ship's tv screen. Would it show in slow motion? If so, why does that happen? With my basic understanding it seems like the broadcast would run at full speed, but how could that be possible over a significant amount of time? Is it that the effects from the moving ship's speed and acceleration causes the transmission to slow down? Thanks for answering my question, you helped me to better understand what would be happening to the ship in orbit, so now I see that there would be more at play in that scenario. However, you have not answered my main question. My main concern was what would show on the stationary ship's tv screen. Would it show in slow motion? If so, why does that happen? With my basic understanding it seems like the broadcast would run at full speed, but how could that be possible over a significant amount of time? Is it that the effects from the moving ship's speed and acceleration causes the transmission to slow down?

ANSWER:
Well, you did not read my answer very carefully. If you had read the Q and A about the father and son, it would have told you how sound would be sped up or slowed down and the picture of the clock would behave the same way. I guess I could have added for the circular orbit part that both the special and general time dilations are to slow down, so the video of the clock would be be slower than the local clock. However, to have a significant slowing would require an enormously large orbit to have a large enough speed for special time dilation to be significant and a small enough acceleration that it did not crush you; the reason that the transmitted clock video is slower is simply because the clock being recorded is running slower in the frame of the "stationary" ship. Of course there is the time delay due to the time of transmission in all cases, so if you synchronized all clocks at the start you would see a delay when comparing clocks, so it would be important to look at the rates of the clocks, not their absolute readings.

QUESTION:
I am looking to find out at what speed an object (object 1) was traveling while hitting an other object (object 2) and pushing it straight ahead. Object 1 was traveling straight, rubber wheels on asphalt (no skid marks), had a weight of 4500 lbs, and hit the object 2 with a weight of 3000 lbs at a 90 degree angle (side impact, rubber sliding on dry asphalt). Object 2 was pushed 50 feet. I am looking for the formula to calculate the speed of object 1 at impact. I know there are several factors involved and I do not need a exact number, just an approximate but fairly close value.

QUERY:
I take it that car 2 was at rest initially and that the two remained in contact the whole time (since you said "pushed"). Also, that car 1 did not apply brakes.

REPLY:
Yes that is correct - object 2 was at rest and object 1 did not apply brakes.

ANSWER:
I prefer to work in SI units, so I will transform back to mph in the end. The masses of the cars are about m₁=4500 lb≈2040 kg and m₂=3000 lb≈1360 kg. The coefficient of kinetic friction for rubber on dry asphalt is in the range 0.5-0.8, so I used μ=0.65. The acceleration due to gravity is g=9.8 m/s². The distance pushed is d=50 ft≈15 m. The frictional force F acting on the two cars as they slide is due only to the friction between the wheels of car 2 sliding on the asphalt is F=μm₂g=0.65x1360x9.8=8660 N. The work done by this friction was W=-Fd=-8660x15=-130,000 J. This took away the kinetic energy K the two vehicles had just after the collision, so K= (m₁+m₂)v_2,1²=1700v_2,1²=130,000, so v_2,1=8.7 m/s≈20 mph; this is the speed the two cars had immediately after impact. Finally, use momentum conservation to get the speed of car 1 before the collision: m₁v₁=(m₁+m₂)v_2,1=2040v₁=3400x8.7=29,600 k∙m/s, and so v₁=14.5 m/s≈32 mph.

QUESTION:
A motorcyclist strikes an automoble that turns directly in front of him. The motorcyclist had locked his brakes and was traveling at @ 30 to 35 mph when he struck the front left fender of the automobile and went over the top of his widnshield at approx. 5 feet. It the motorcylist weighs 210 lbs. how far would he have traveled before he struck the ground?

ANSWER:
There is no way to calculate this with the information you have given me. All I can tell you is the absolute maximum distance he would go if launched with a speed of about 30-35 mph at 45⁰ angle to the horizontal, about 25 meters. It would certainly be less than this since he would lose some kinetic energy in the collision and would not be launched with a speed as great as he came in with and he would likely be launched at another angle.

FOLLOWUP QUESTION:
I'm actually thinking that for me to clear a 5 foot windshield directly in front of me from a sitting position about 15 inches behind that windshield and from a sitting position my launch angle was very close if not more than 45 degrees. If that is the case, loss of kinetic energy is the only limiting factor to the distance I traveled before landing on my shoulder on the other side of the car. Is the loss of kinetic energy linear to the distance traveled? In other words if I lost 1/3 of my kinetic energy in the crash would the distance traveled be equal to 1/3 less distance? 25 meters is 82 feet minus 28 feet equals 54.

ANSWER:
OK, here is the full equation if you want to play around with it: R=v²sin(2θ)/g where R is the horizontal range in m, v the speed of launch in m/s, θ is the launch angle, and g=9.8 m/s² is the acceleration due to gravity; this is for the projectile landing at the same level where it was launched from, not really exactly true but of minimal consequence since we are only doing a rough calculation. 30 mph=13.4 m/s, so you can put in any speed as v_m/s=0.45v_mph. For θ=45⁰, R=v²/g. To answer your question, the distance is not proportional to speed, rather to the square of the speed. So, if you lost 1/3 of your speed (30 mph) in the collision your speed in m/s would be v=20x0.45=9 m/s, so R=81/9.8=8.3 m≈27 ft. This is more realistic, I believe, than 25 m which would be predicted for 35 mph.

QUESTION:
Why the magnitude of limiting friction bears a constant ratio to the normal reaction between the two surfaces.

ANSWER:
The thing we learn about frictional forces in an elementary physics course is that they are proportional to the force that presses the surfaces together (usually called the normal force). This is not a law of physics but simply an empirical observation which is a very good approximation for most surfaces under every-day conditions.

QUESTION:
Are there any theories as to why the speed of light is 186,000 mps? That is, why not more, why not less, what dictates this value?

ANSWER:
Maxwell's equations are four equations which contain everything (except photons) that can be known about electromagnetism. They predict waves which propagate with a speed of 1/√(μ₀ε₀) which just happens to be exactly the measured speed of light. The two constants, μ₀ and ε₀, are the permeability and permittivity of free space and essentially quantify the strengths of electric and magnetic forces. You might also want to read earlier related answers linked to on my faq page.

QUESTION:
Most physics texts that I read state that a net torque tends to produce rotation - certainly true in case of a sphere rolling down an incline (friction produces the torque and this results in rotation). However, consider a particle of mass m in the XY reference frame, acted upon by a force F in a direction along the X axis producing an acceleration a = F/m. Let us assume that the mass m is located at (x= 0, y = y0) from the origin. Then there IS a net torque on the mass m (seen about an axis perpendicular to the XY plane passing through the origin) given by (y0) multiplied by F (angle being 90 deg). But mass m undergoes NO rotation! It simply translates in the direction of F. What's confusing for me - is there a definite criterion defining the situation when a net torque would produce rotation and when it would not.

ANSWER:
The example you stated is the simplest to understand because this force is perpendicular to its moment arm and so the torque is τ=Fy₀. First of all, a point mass cannot, by definition, rotate about an axis through itself. If you calculated the torque about an axis ay x=0, y=y₀, you would get zero torque and zero "rotation". I think you are confusing yourself by saying that a torque causes a rotation. In fact, a torque causes an angular acceleration about the axis, so you need to ask whether your applied force causes an angular accleration. Angular accleration a is related to the acceleration a by α=a_t/R where R is the moment arm (y₀ in your example)and a_t is the tangential acceleration, the component of the acceleration a perpendicular to the moment arm. In your notation, the acceleration is all tangential and so a_t=F/m. Therefore, there is an angular acceleration α=F/(my₀) about the z axis at the instant you start your problem. Even if there were no force and the mass were just moving with some velocity v in the x direction right now, it would be considered to be rotating about the z axis with an angular velocity ω=v/y₀. If you exerted some force which kept it moving in a circle of radius y₀ with speed v (that would be a force which had no tangential component, pointed toward the origin, and had a magnitude mv²/y₀), you would surely say that it was rotating around the origin, wouldn't you? In general, whenever the velocity has a component perpendicular to the line drawn to some axis, it has an angular velocity around that axis.

QUESTION:
I purchased a visco elastic (memory foam) mattress topper for my queen sized bed. The advertised dimensions of the topper are 76 inches x 56 inches x 4 inches, and the advertised foam density is 4 pounds per cubic foot. I calculated that the topper should weigh 38 pounds. The delivered topper weighs about 5 pounds. The delivered topper is in a very compressed state constrained by its plastic packaging. The delivered topper has a cylindrical shape with the cylinder being approximately 15 inches tall and 12 inches in diameter. Would the compressed state account for the weight discrepancy? ie if the topper was released from its packaging and allowed to expand to its full size on top of the bed, would the expanded topper weigh 38 pounds. I don't think so. I can't release the topper from its packaging and then return it. I can't put that genie back in the bottle. It is very important to me. The topper was very expensive. Your wisdom would be greatly appreciated.

ANSWER:
The volume of the topper is 76x56x4/12³=9.85 ft³ and would have a weight of 39.4 lb if its density were 4 lb/ft³. The volume of the compressed topper is about π6²x15/12³=0.982 ft³ and since the compressed topper weighs about 5 lb, its density is about 5.09 lb/ft³. Clearly the density number must refer to the material with all air removed from it. That is also the impression I get from looking at advertisements for these products. If you think about it, there is no way that a 4 inch thick foam matress topper for a queen bed whose volume is clearly mainly air would weigh almost 40 lbs. I think that what you got was not falsly advertised.

QUESTION:
From both my high school and university (albeit, introductory at university) physics courses, we've encountered the concept of escape velocity, as the velocity with which an unpowered mass must be accelerated to to escape the gravitational pull of a body. We've also been given an extra piece of information about this, which is that the escape velocity is the speed at which an object will be accelerated to when it hits the surface of a body, falling from an infinitely far away point. So here's my question - it seems then that if I sent an unpowered mass at the escape velocity of a body, then (assuming there were no other gravitational factors, etc), then when it reached the 'infinity far away point' it's velocity would equal zero. So, even though you could never reach that point - is that logic valid? If so, doesn't that mean then that escape velocity is more of a measure of the velocity needed for an unpowered mass to reach x point far away? So then shouldn't it then, if I were to reach a point closer than one infinity far away, why wouldn't I be able to escape with a lesser velocity? I suspect a flaw in my reasoning above, but I don't know what it is - any and all response would be greatly appreciated!!

ANSWER:
I am afraid I do not really get your point. But, escape velocity is a concept somewhat divorced from reality because the universe is not infinitely large and there are other things in it besides the earth and your projectile. It is easy to see how the computation of (ideal) escape velocity can be done. With nothing in the universe but the earth, the total energy of the projectile at the earth's surface is mv(r=R_earth)²-GM_earthm/R_earth where I have chosen potential energy to be zero at r=∞. Anywhere else the energy would be mv(r)²-GM_earthm/r. If we choose r=∞ and use energy conservation, mv(R_earth)²-GM_earthm/R_earth= mv(∞)² and the smallest velocity v(R_earth) could be is called the escape velocity and corresponds to v(∞)=0, so v_escape=√[2GM_earth/R_earth]. If there were indeed nothing else in the universe and the universe were infinitly large, this is the speed you would have to give something for it to never come back. In the real world, interaction with other objects would affect the speed necessary for the object to never come back, so you should not think of escape velocity as that speed because escape velocity is well defined but the speed to escape the real earth is not the same thing.

QUESTION:
If one stood on a typical neutron star and turned on a flashlight pointed horizontally, would the light bend down to the surface much like water coming out of a garden hose here on earth?

ANSWER:
The density of a typical neutron star is about 5x10¹⁷ kg/m³ and the radius is about 12 km, so the mass is about M=5x10¹⁷x4xπ(12x10³)³/3=3.6x10³⁰ kg. To find the acceleration due to gravity, g=GM/R²=6.67x10^-11x3.6x10³⁰/(12x10³)²=1.7x10¹² m/s². So your weight on the surface would be about a trillion times what it is on earth. You would be crushed totally flat. We can estimate how far light would "fall" in such a gravitational field. The time it would take to go 100 m would be about t≈100/(3x10⁸)=(1/3)x10^-6 s. The distance it would fall would be y= gt²≈10 m. On earth the speed of something which dropped 10 m in 100 m would be about 71 m/s≈160 mph, quite a bit faster than a garden hose. But qualitatively, yes the light would fall quite a lot.

QUESTION:
We want to know if a a gun could and was traveling at the speed of light and it was fired would the bullet simply fire as normal or would the bullet refuse to leave the gun? We have tryed to find a definitive answer elsewhere but can't seem to find one

ANSWER:
First of all, it is physically impossible for the gun to move with the speed of light; see my faq page to find out why. But, I think I can get to the crux of your question by having your gun move at 99.9% the speed of light, v=0.999c. Let us suppose the muzzle velocity of the gun were u=0.002c (which is, incidentally, much faster than any real bullet would travel). Now, classical physics would have a bystander see the bullet going with a speed v+u=1.001c, faster than the speed of light. But we know that this is not possible, so classical physics must be wrong. The correct formula for velocity addition in special relativity is (v+u)/[1+(uv/c²)]=1.001c/(1+0.999x0.002)=0.999004c, just slightly faster than the gun. If you were moving along with the gun, you would simply see the bullet go forward with a speed 0.002c. All the above is if the bullet is fired in the direction the gun is traveling. If you fire the gun backwards from its direction of travel, replace u by -u and find that (v-u)/[1-(uv/c²)]=0.998996c, slightly slower than the gun but moving in the same direction. If you were moving along with the gun, you would simply see the bullet go backward with a speed 0.002c.

QUESTION:
I am curious as to how a spoiler on a car somehow provides less wind resistance when driving. One would think that the more surface area against wind would cause more resistance which would slow down the car at a steady RPM. I don't understand how adding another element that increases surface area would somehow decrease resistance. When I think about it, the less surface area against air flow, the less resistance there would be. So my question is, do spoilers on cars really work? If they do, how? I have always been curious about this and have never gotten a straight answer out of somebody that really understands how they work.

ANSWER:
Well, one might say that "nobody really understands how they work"! I have "sort of" answered this question before, but mostly dodged it. Here I will do a little better, I hope! I can give you a good qualitative explanation but in terms of being able to design one by just sitting down with some fundamental equation like Bernoulli's equation, forget it. Fluid dynamics can be deceptively simple or amazingly complex. To do serious aerodynamic design requires extremely powerful computers and trial and error wind tunnel experiments. The things we do to reduce air drag are often counterintuitive compared to our expectations. With that prologue, let me give you a couple of simple examples. Our expectations are that an object should be very smooth and this is often the case, particularly if velocities are not too large. Your expectation that the increasing area presented to the onrushing air causes greater drag is reasonable and you will find many examples here on AskThePhysicist.com where I approximate the drag force as being proportional to the cross sectional area, e.g. F_drag≈ Av². But there are many situations, particularly at high speeds, where this expectation breaks down and the culprit is turbulence in the air. To illustrate how turbulence affects drag and how smooth is sometimes not good, consider a golf ball. Have you ever noticed that a golf ball has dimples? The purpose of these dimples is to reduce air drag. As shown on the left above, a smooth ball at a high velocity has a long turbulent volume behind it; the pressure in this turbulent volume is lower than the pressure on the front of the ball and this contributes to there being a large net drag force. If golf balls were nice and somooth, they would die and fall very much sooner than a dimpled golf ball does. The ball on the right shows the effect of the dimples; the rough surface induces a layer of turbulence which actually makes the ball "slipperier" which causes the flow around the ball to come back together and reduces the volume of turbulence contributing to drag. (The picture on the right, with most of the turbulence erased, would be what the smooth ball would look like at low speeds.) The hairs on a tennis ball serve the same purpose. One other example is the net you sometimes see replacing the tailgate of a pickup to reduce the drag the tailgate causes. This, it turns out, is a complete fraud. With the tailgate closed a bubble of still air forms in the bed of the truck which deflects the air smoothly over the rear of the truck. So, in your case, the purpose of the spoiler, very similar to the golf ball dimples, is to disrupt the smooth flow in such a way that the net effect is less turbulence behind the vehicle. Incidentally, there is something called the Reynolds number which allows you to estimate whether or not turbulence is important.

QUESTION:
What kind of physics or math would explore the relationship of two gears that are engaged and spinning? I am a musician, and envision the bottom gear wheel to be the basic pulse of some music. The top gear being of a different wheel size would represent a polyrhythm. The polyrhythm would complete a full revolution in a longer interval than the smaller wheel, which is the basic pulse. How can I explore this idea mathematically?

ANSWER:
It is just a matter of ratios. You want to compare the rotational frequencies f of two coupled wheels. Look at the gears in the figure. The larger gear has 20 teeth and the smaller gear has 12. So, if the smaller gear rotates once, the larger gear rotates 12/20=3/5 times. So, if the smaller wheel rotates 5 times, the larger rotates 3. The ratio of their frequencies is f₂₀/f₁₂=3/5. In general, if you have one gear with n teeth and one with m teeth, f_n/f_m=m/n. Using gears you are restricted to ratios of integers. If you used two smooth wheels with radii r and R which did not slip on each other, the ratio would be f_r/f_R=R/r.

QUESTION:
Why does the electric field at infinite distance from a uniformly charged disc not equal to that of a point charge as it is zero.

ANSWER:
I do not understand. Any local charge distribution (not, itself, extending to infinity) goes to zero at infinity. What matters is how it goes to zero. At very large r, the field should go to zero like Q/r² where Q is the net charge, and I guarantee that a uniformly charged disc will do this. If the net charge is zero, it will approach zero differently. For example, an electric dipole field will approach zero like 1/r³.

QUESTION:
Can Newton's Laws be logically deduced or experimentally verified? If no, are they mere axioms?

ANSWER:
Your first question is really two questions. Can they be "logically deduced"? The answer is that you cannot get these laws by sitting at your desk thinking about how objects react to forces, you need to actually do experiments to find out; when you do this you find that the acceleration is proportional to the force and inversely proportional to the mass. Can they be "experimentally verified"? Obviously, yes, since that is how you discovered them in the first place. Are they exactly true? Not at very large velocities when the speed is not small compared to the speed of light. Are they always true? No, not if the frame of reference you are in is accelerating. No, they are not axioms.

QUESTION:
How do I calculate the kinetic energy of relativistic objects? I am in the process of doing worldbuilding for a scifi story and I'd need the formula used to calculate relativistic kinetic energy.

ANSWER:
Consider an object with rest mass m₀ and speed v. Its total energy is mc² and its rest mass energy is m₀c² where m=m₀/√(1-(v/c)²). Its kinetic energy is K=mc²-m₀c². It is relatively easy to show that, if v<<c, K≈ m₀v².

QUESTION:
Why is it that we always only see the same side of the moon?

ANSWER:
It is called "tidal locking". You know that the moon causes tides in the ocean; tides are essentially bulges of the ocean on the sides facing and opposite where the moon is. You can read a brief explanation of tidal forces in an an earlier answer. The moon also causes "land tides" causing small deformations of the earth's crust. The earth, of course, also exerts tidal forces on the moon causing tidal bulges on the sides toward us and away from us. If the moon were rotating with a more rapid period than today, as it was sometime in the past, it would be slightly egg shaped because of the tidal forces and the result would be that there would be a torque on the moon tending to slow its rotation down; over time the period slows so that the period of rotation is precisely equal to the period of orbit (about one month) resulting in its keeping the same side toward earth always. You can read much more detail in the Wikepedia article on tidal locking.

QUESTION:
What is radiocarbon?

ANSWER:
I suspect you are asking about radiocarbon dating of organic materials. There are two stable isotopes of carbon, ¹²C and ¹³C. There is one unstable isotope of carbon which exists in nature also, ¹⁴C, sometimes referred to as radiocarbon. Radio carbon is being constantly produced by cosmic rays interacting with ¹⁴N in the atmosphere. ¹⁴C has a half life of about 5730 years, so if cosmic rays suddenly stopped creating it there would be almost none left after a few half lives. But, there is a balance between creation and decay so that the amount of ¹⁴C on earth is about constant at any time. However, suppose you find a fossil which has only about half as much ¹⁴C as all the current living things on earth. Then you can conclude that the fossil is about 5730 years old.

QUESTION:
What is the formula for calculating the velocity of an object (such as a spaceship) at a constant linear acceleration of 1G after X number of hours?

ANSWER:
I believe you will find everything you need in an earlier answer and links therein. The appropriate equation for velocity in m/s is v=(gt)/√[1+(gt/c)²] where t is the time in seconds, g=9.8 m/s², and c=3x10⁸ m/s. (There are 3600 seconds in an hour.) Be sure to note that t is the time as measured on earth, not on the spaceship. A different time will have elapsed on the spaceship.

QUESTION:
Does Heisenberg's uncertainty principle say that quanta (?) are everywhere at once? If I were to say that, would there be anything wrong with this statement?

ANSWER:
Almost never does it say that. A particle whose momentum (speed, essentially) is perfectly known has total uncertainty in position. What that means is that it is equally probably to find the particle anywhere in space. Generally, though, we have some idea where the particle is, for example by confining it in a box, and then you might find anywhere inside that box depending on the quantum state it was in. This does not mean that it was "everywhere [inside the box] at once", it means there is a probability that it might be found anywhere in the box.

QUESTION:
In many fictional works involving superpowered individuals there are people who can lift objects with their minds. Like an example is Magneto lifting a submarine with the magnetic fields he generates in the movie X-Men First Class. From what I remember from high school physics is that because of Newton's third law, all actions produce an equal counteraction. So wouldn't Magneto lifting a submarine with his powers lead to him being heavily attracted by the submarine and flying straight towards it like a bullet and then getting himself completely flattened into a pancake when he crashes with the submarine?

ANSWER:
You are absolutely right, if he exerts a force on the submarine, the submarine exerts an equal and opposite force on him. Now, he is on some kind of aircraft and holding on, so he would have to be superstrong to be able to hold on since the force pulling him is greater than the weight of a submarine. And whatever he is holding on to would have to be strong enough that it could hold more than the weight of the submarine. And the aircraft had better be able to carry the weight of a submarine. But, maybe his power is in his left arm so the force would just rip his arm off. Pretty preposterous, isn't it? Good thinking on your part.

QUESTION:
Imagine a tank full of water on top of a house with a drain pipe down to the ground. The higher the tank, the greater the velocity and force of water coming out of the end of the pipe. But does the tank drain any faster with greater height? You can't pull on water, so the water leaving the tank shouldn't care how high up the tank is, so the answer must be no. On the other hand, it does seem from actual experience that a bucket will be filled faster from the higher tank. I've even measured this! (Imagine this in a vacuum to bypass any air-pressure effects)

ANSWER:
The operative physical principle is Bernoulli's equation, P+ ρv²+ρgy=constant. Here, ρ is the density of the fluid, P is the pressure, v is the speed of the fluid, and y is the elevation relative to some y=0, all at any point in the fluid; g=9.8 m/s² is the acceleration due to gravity. In your case, P is atmospheric pressure both at the top and at the bottom (assuming the tank is not sealed), I will assume y=0 at the bottom and y=h at the top surface, the speeds at the bottom and top are v_bottom and v_top. Putting these into Bernoulli's equation and solving, v_bottom=√[2gh+v_top²]. Now, if the tank is much bigger than the pipe, usually the case, you can approximate v_top≈0 and so v_bottom≈√[2gh]. An interesting fact: this is exactly the speed the water would have if you simply dropped it from a height h. (You do not have to imagine a vacuum since the pressure is easily included and, as here, often the same everywhere. If the tank were pressurized to a pressure greater than atmospheric, the water would drain faster.)

FOLLOWUP QUESTION:
I see the math, but I remain puzzled for this reason: the water flow (in gpm, say) into the pipe (where it connects to the tank) is, obviously, the same as the flow at the bottom end of the pipe. Having constant gpm flow through a constant-diameter pipe necessarily means the water is passing through the entire pipe at the same velocity. So the velocity of the water that s just entered the pipe must be the same as the velocity of the water that s about to exit. But Bernoulli s equation in the form you ve given implies that the water is accelerating as it flows down the pipe. That would require more gpm out than what went in!

ANSWER:
No, I am assuming that the tank has water in it and the point I call y=h in my answer is the top surface of the water in the tank which I subsequently approximate as a much larger area than the pipe. You could, I guess, let y=h be the top of the pipe but then the pressure there would not be atmospheric. Then you, as you suggest, would have to say v_top=v_bottom≡v; the subscript top now means the top of the pipe. So, let's call the depth of the water in the tank to be d and apply Bernoulli's equation to the tank only: P_top+ ρv²+ρgh≈P_A+ρg(d+h) where I have again approximated the top surface of the tank to have zero velocity. Then the pressure at the top of the pipe is P_top≈P_A+ρgd- ρv². Now apply Bernoulli's equation to the pipe: P_A+ρgd- ρv²+ ρv²+ρgh≈P_A+ ρv² or v²≈g(d+h) which you will see is the same answer as above except h now means the bottom of the water in the tank rather than the top of the water in the tank.

ADDED NOTES:
Bernoulli's equation is only applicable for incompressible, nonviscous fluids having laminar (nonturbulent) flow. It is a fairly good approximation for gases with velocities small compared to the speed of sound. It is a statement of conservation of energy.

QUESTION:
Time travel: if you time travel to a specific point in time wouldnt you need to also travel to the space in which the earth was at that specified time in space.

ANSWER:
Right you are, time travel is, of necessity, also space travel. However, you should not say where "the earth was at that specified time" but rather "the earth will be at that specified time" because, at least as best we know, only time travel forward in time is possible.

QUESTION:
In a rotating frame of reference, do the Coriolis force and the centrifugal force act opposite to each other?

ANSWER:
Certainly not. The centrifugal force always points perpendicular to the rotation axis. The Coriolis force always points in the direction of vxω where ω is the angular velocity and v is the velocity of the particle. It is possible for the two to point in opposite directions, but not usual. An example where they do point opposite is shown to the right. You might be interested in a recent answer.

QUESTION:
I was trying to calculate the ideal performance of a bow. I wanted to relate the draw weight of a bow to the speed of the arrow after releasing it. And I couldn't do it. The things I have are arrow weight(300 gramms) and the draw weight peak(that means 27kg at 72cm). The draw weight works like this. At 72cm it's 27kg and at 0cm it's 0kg. It is a linear decrease in kg. 27kg at 72cm, 22.5kg at 60cm, 18.75kg at 50cm and so on. And now I wanted to calculate how fast the arrow will fly after shooting it. That means I would like to know how to calculate the speed of an object with a mass of 300g after 72cm of accelerating with a force of 27kg that linearly decreases over 72cm to 0kg. Could you please tell me the formula I need to use to calculate that? I realy would love the learn the way how I have to do it.

ANSWER:
If the force increases linearly with distance, it is behaving like an ideal spring. The force F necessary to stretch a spring with spring constant k a distance x is F=kx. Now, 27 kg is not a force, it is a mass; but, if you mean that the force is the weight of a 27 kg mass (which you doubtless must), then F=27x9.8=264.6 N. So, since x=0.72 m, the spring constant of your bow is k=264.6/0.72=367.5 N/m. The potential energy of a spring stretched by x is ½kx²=½(367.5)(0.72)²=95.3 J. The kinetic energy of a mass m with a speed v is ½mv²=½(0.3)v²=0.15v². Ideally, all the potential energy of the bow is given to the kinetic energy of the arrow, so v=√(95.3/0.15)=25.2 m/s≈56 mph. If the arrow is not launched horizontally, there would be a small correction for change in gravitational potential energy. I am not sure how good a model this is for a real bow.

FOLLOWUP QUESTION:
The reason why I was asking is because I build bows and wanted to find out how good the efficiency of my bows are. And with your answer I found out that the weight of the arrows I mentioned to you and I was using in my own calculatoins was far too high. In the world of archery the arrows are measured in grains instead of grams but I forgot that and used 300 grams instead of 300 grains. So my calculations of the arrow speed were so low that I thought that I made a mistake in calculating. So I asked you. As the speed you calculated was very low too I started asking myself why and found out what mistake I made.

ANSWER:
300 gr=0.0194 kg, so ½mv²=½(0.0194)v²=0.0097v², so v=√(95.3/0.0097)=99.1 m/s≈125 mph. Incidentally, for easy unit conversion I recommend a free little program called Convert.

FOLLOWUP QUESTION:
I understood your answer and started calculating bows to understand how efficient they work. I was very surprised when I calculated the efficiency of a compound bow. The bow I used to make my calculations is a 2014 PSE Full Throttle from the Pro Series of PSE Archery . The IBO-Bow Speed is measured with a speed chronograph with a bow with 70 pounds draw weight, 30 inches draw length and 350 grain arrow (. The speed measured is 362-370 fps. The astonishing thing I found out that this compound bow has an efficiency of 110-120% compared to a perfect spring. How is this possible? Is it because of the special mechanical function of the cams(wheels) and the cables? Do these cams and cables use a special leverage provided by the cams to gain more power? Here is a link to a pdf of a product review of several compound bows. On page 7 there is a draw force curve. And in the article they are talking about efficiency as well. But I don't realy understand what it means (dynamic efficiency and draw cycle efficiency).

ANSWER:
Actually, I have previously answered a question about a compound bow which you might want to look at to get a brief overview of its advantages. As you can see from the draw force curve which you refer to (see left), the biggest advantage is that you end up with a relatively small force even though you stored a large amount of energy; this allows for conditions for more relaxed aiming and easier steadying of the bow. You still have to put all the energy in which you expect to get out. The important thing to understand is that the energy stored in the bow is the area under the the force vs. pull curve. For a simple spring, the force, kx, is a straight line and so the area under it is the area of a triangle ½∙height∙base where the height is kx and the base is x. The efficiency normally means the ratio of energy out to energy in times 100. As best as I can tell, the draw cycle efficiency is calculated by measuring force vs. distance pulling and comparing with force vs. distance if you then slowly let it back down. It seems to me that dynamical efficiency should compare measured kinetic energies of the launched arrows which is the total energy extracted from the bow, but that does not seem to be the case; I cannot find a clear definition of dynamic efficiency. These differ only because of energy losses, mainly due to friction. Suppose we had a linear bow with the same draw (about 21"=1.75 ft) and which stored the same energy (78.3 ft∙lb); the spring constant would 51.1 lb/ft and the force at maximum draw would be 89.5 lb. I have added the yellow curve to the graph from the paper you referred to which is shown in the figure. Now each arrow from these two bows travels the same distance while rubbing on the bow, so those rubbing frictional forces are the same assuming similar materials and friction between the bow and arrow. But the linear bow acquires a high speed much sooner than the compound bow which means it is going much faster on average even though the two end up at the same speed. But, the air drag is proportional to the square of the velocity so the linear bow will likely lose more energy to air drag during the launch than the compound bow. At least, that is my guess. There can be miriad other reasons why the efficiencies differ due to details like friction in the pulleys, different materials and designs, etc. I must admit that I do not understand what actual and effective letoff are.

QUESTION:
What happens when you compress gas (for example carbondioxide) into a gas cylinder? I am thinking that as pressure increases, so does temperature (because of the increased kinetic energy of the gas molecules). But the 0th law of thermodynamics say that heat energy goes from an object of high temperature to an object of low temperature - so I am thinking that after a while the temperature of the gas will be the same as the room temperature?! Since pressure is constant in the cylinder, isn't the kinetic energy of the molecules? What happens with the gas as energy is transferred to the room? Am i misunderstanding something? I just can't seem to Get my head around this, so I am really hoping you can help med understand!

ANSWER:
When you say " compress gas into a gas cylinder " I assume you mean that you are adding gas to a cylinder with constant volume. All you really need to know is the ideal gas law, PV=NRT where P is pressure, V is volume, N is some measure of how much gas you have, T is the absolute temperature, and R is just a constant of proportionality. You need to make some approximations for a real-world situation. If you add the gas really slowly and/or the cylinder has very thin conductive walls, T will remain constant for the reason you state because the whole system will keep in thermal equilibrium with its environment; this is called an isothermal process and as N increases, P increases. The other extreme is that you take care to insulate the cylinder and/or add the gas very quickly so no heat enters or leaves. In this case, increasing N will increase the ratio P/T; however, you do not have enough information to determine how P and T independently change. If you are using some sort of pump, you will be doing work on the system which will increase its temperature and therefore the pressure has to increase also to keep P/T from decreasing. Another way to add gas to the tank is to take another tank with much more gas in it and connect the two of them together. Since the volume of the two tanks and the total amount of gas in them is constant, the temperature of the whole system will decrease. Again, pressure in your tank must change in such a way that P/T increases and that could mean that P would increase, decrease, or stay the same, depending on initial situations.

FOLLOWUP QUESTION:
Thanks for the quick reply! I am still wondering though, is it possible for a compressed gas in a cylinder to have higher temperature than the room it is stored in over time? I am thinking comparing to coffee in a thermos after some hours will be the same temperature as its surroundings. Is the gas cylinder any different due to pressure inside the tank?

ANSWER:
Eventually the cylinder, room, and gas will all be at the same temperature. If (see above) the temperature right after filling were higher than the room temperature, it would cool down and the pressure would decrease because now both V and N would be constant while the gas cooled. Here is a numerical example: suppose that the room temperature is 20⁰C=293 K and the gas temperature is 40⁰C=333 K and the hot gas has a pressure of 4.0 Atm. Then, P/T=constant=4/333=P_final/293 or P_final=3.5 Atm.

QUESTION:
What is the effect of earth rotation on the human upright posture? Please visit my website. I would like to corroborate my hypothesis from a physics point of view.

ANSWER:
My original response, via email, was "The forces are negligibly small. I am quite certain that they are not a factor in back pain." However, the questioner persisted.

FOLLOWUP QUESTION:
Thank you for responding to my question regarding the effect of rotation on the upright posture. As you stated the effect is indeed subtle but it is there. The point of my work is that the human spine is much more sensitive to these forces than researches realize. Did you solve the question of the effect of rotation on the standing posture and what was the result percentage please. Did you fiqure a formula for this and was it compatible with my rudimentry estimate of 30% percent anterior left torque and spinal differential findings of 8%?

ANSWER:
I cannot resist responding in some detail to this since it is so wrong! What we need to understand is how to do physics in a rotating (accelerating) frame of reference such as the earth. In such a frame, there are real forces and fictitous forces. As the questioner correctly notes, even though we call the fictitious forces fictitious, they can certainly be felt by our bodies if they are large enough. The best known fictitious force is the centrifugal force; this is what we feel trying to throw us off a rotating merry-go-round. A person at rest on the earth's surface experiences a real force called the weight which points toward the the center of the earth and has a magnitude W=mg; a fictitious centrifugal force pointing perpendicular to and away from the axis of rotation and has a magnitude F_cen=mrω² where r is the distance from the axis and ω=7.3x10^-5 radians/s is the angular velocity of the earth's rotation*; and whatever force the floor exerts on the object to maintain equilibrium. If the person is moving along the earth's surface, there is an additional fictitious force called the Coriolis force which I will discuss later. I start with the simplest situation which is someone standing on the equator. The first figure is a view from the north pole; the earth rotates counterclockwise; the man has a mass m=100 kg so his weight (red) is W=980 N; the radius is r=R_earth=6.4x10⁶ m, so the centrifugal force (green and not to scale) is F_cen=100x6.4x10⁶x(7.3x10^-5)²=3.4 N; finally the force from the floor (black) is vertically upward and of magnitude 980-3.4=976.6 N. Note that there is no net torque for a person at the equator and the only effect is that there is approximately a 0.35% reduction in apparent weight. Next let us examine a person at rest but at some latitude, say 45⁰ north as shown in the second figure above; the color coding of the forces is the same as in the first figure. The weight is the same, 980 N; the radius is r=R_earthsin45⁰=4.5x10⁶ and so F_cen=100x4.5x10⁶x(7.3x10^-5)²=2.4 N; F_cen has a radial component 2.4sin45⁰=1.7 N which reduces the apparent weight (radial component of the floor force) by that amount; F_cen has a tangential component 2.4cos45⁰=1.7 N which results in a torque and is also the magnitude of tangential component of the floor force. The torque tries to rotate the person about his feet in a southerly direction (would be northerly in the southern hemisphere) and has a magnitude of 1.7 N∙m, estimating the center of gravity to be 1 m above the feet. To put this in perspective, if the earth were not rotating the person would have to lean at an angle of tan^-1(2.4/980)=0.14⁰ to have a comparable torque about his feet; this angle is far smaller than leaning we do in normal activities. Furthermore, since the direction we happen to be facing is random, the average effect over time of these very tiny torques would be zero.

Finally, there is another (fictitious) force which acts on objects on the rotating earth, the Coriolis force. This is the force responsible for the cyclonic motions of weather patterns. However, the object has to be moving and the direction of the force depends both on location and direction of the velocity. Its maximum possible magnitude is F_Cor=2mωv where v is the speed; its direction is always perpendicular to the velocity vector. The fastest the average person is likely to be moving is the speed of a passenger jet, about 500 mph≈220 m/s, so F_Cor=2x100x7.3x10^-5x220=3.2 N. Again, this force is so small compared to other forces acting that its effect on physiololgy would be essentially zero.

*ω=(1 revolution/day)(2π radians/revolution)(1 day/24 hr)(1 hr/3600 s)=7.3x10^-5 s^-1

QUESTION:
[Gravitational time dilation] From a mechanical point of view I understand how a clock can tick slower if its closer to the gravitational mass. But how does that affect our biology so that we actually age slower?

ANSWER:
Actually, you do not understand how a clock can tick slower because it is not something gravity does to the clock, it is something gravity does to time. All clocks run slower because time itself runs slower as the gravitational field gets stronger; this includes biological clocks. You would never notice it for biological clocks in the earth's gravitational field because the effect is very tiny and biological clocks are not accurate enough to detect the slowing. You could detect it if you were close to a black hole where gravity was much stronger.

QUESTION:
If I were to draw a straight line between the southern most point (isle of scilly) to the Northern most tip (hermaness) of the UK, approx 1000 miles How far under the surface of the earth would the mid point of the line be?

ANSWER:
This is not physics, it is simple geometry. Referring to the picture to the right, 2θ=S/R and the distance you want is R-Rcosθ. For your case, S≈1000 mi, R≈4000 mi, θ≈S/(2R)=0.13=7.16⁰ so R-Rcosθ≈31 mi.

QUESTION:
If I was throwing a ball around with a friend on a torus-shaped space station which was rotating in order to simulate gravity, would I have to lead her? Alternatively: if I "dropped" the ball (i.e. let go of it) from, say, waist-high, would the ball land at my feet, or somewhere else? (Assume that the torus is roughly 50m in diameter, neither accelerating in space nor in terms of its rotation, and that we're using a standard baseball.)

ANSWER:
My first inclination was to say that, assuming that the station was big enough, it would be pretty much like playing catch on earth. The reason for this guess was that I had worked out a similar problem for an earlier answer. The question there was if you were to jump straight up what would happen; this is equivalent to throwing a ball straight up with some speed v. The answer was that for sufficiently large R and small v, in particular R>>v²/g, the time the ball was in the air, the "height" it rose, and the time it took to "return" would all be about the same as if you did the experiment on earth; also, the ball would "land" back where you were. I believe that answers your question about whether a dropped ball would land at your feet it would (approximately). You should be sure you understand that earlier answer. The leftmost figure above reproduces the original figure for the ball thrown straight up. The other two do not have all the vectors labeled, but you can tell by comparison what they are. The components of v are shown by light blue vectors and the red is just to aid me in adding the vectors v and Rω. The second figure is if your partner is ahead of you (ahead meaning in the direction of rotation). The third figure shows how you would throw it if your partner were behind you. It is not at all clear to me where you and your partner would be located when the ball "came back down" to the ring (shown by green dots). Also, trying to do the problem analytically in the nonrotating frame gets very messy. I am just interested, as are you, in your situation thrown baseballs in a 50 m ring rotating such that Rω²=g where ω is the angular velocity in radians per second. So, I am going to do the calculations in the rotating frame (where you and your partner are at rest). This gets sort of tricky since we will have to introduce fictitious forces to describe the motion of the ball; once you throw the ball, there are no real forces on it. Now, maybe you throw the baseball (mass about 0.15 kg) with a speed of 10 m/s≈22 mph. There are two fictitious forces: the centrifugal force F_r=mRω²=mg which points down for you and the Coriolis force F_Cor=2mωv=2mv√(g/R) and points in a direction perpendicular to the vector v as shown in the figure to the left. Now, let's calculate the magnitudes of these forces. F_Cor=2x0.15x10x√(9.8/50)=1.3 N and F_r=0.15x9.8=1.5 N. The Coriolis force is not small compared to the centrifugal force and therefore the ball will not behave at all as it would in a true uniform gravitational field. You would go nuts trying to play catch on this space station! The problem is that R=50 m is not much greater than v²/g=10.2 m. See the added thought below for a discussion of the dropped ball.

ADDED THOUGHT:
When the ball is simply dropped from say h=1 m, the Coriolis force is relatively small because the velocity is small for most of the time of the fall. So, the deflection should be modest. I will try to estimate the amount of deflection. The centrifugal force is mg and is always radially out, choosing +y radially out, a_y=-g+a_y^Cor; I will argue that the ball does not acquire enough speed for the Coriolis force to have a significant radial component, so a_y^Cor≈0, and y≈h- gt², v_y≈-gt. So, the time to fall is approximately t≈√(2h/g)=0.45 s and v_y≈4.4 m/s. The Coriolis acceleration, if the velocity is purely radial, points in the backward direction (to the left in my figure above) and would have a maximum magnitude of about 2v√(g/R)≈3.9 m/s². If the ball drops almost vertically the Coriolis acceleration would be approximately a_x≈2m√[(v_x²+v_y²)(g/R)]≈2mv_y√(g/R)=2mgt√(g/R)=8.68t=dv_x/dt; therefore, integrating, v_x≈4.34t² and x≈1.45t³. So, at t=0.45 s, v_x≈0.88 m/s and x≈0.13 m=13 cm.

QUESTION:
How does a GPS station work? I just learned about them and my teacher was a little unclear so I'm depending on you to hopefully give me a direct answer.

ANSWER:
Suppose that you were on a line and you wanted to know where you were. If there were some point on that line and you knew where you were relative to that point, then you would know where you are. Now suppose that you were on a flat plane. Now you would need two other points the positions of which you knew to figure out where you were relative to these points (this is often called triangulation). Now suppose that you are just somewhere in a three dimensional volume; it would follow that you would need three points whose positions you knew to find out where you were in that space. So, if you want to know where you are if you are at rest, you would need to know the positions three points of reference at rest relative to you and simple geometry would tell you where you were. The GPS system is a bunch of satellites which are zooming around in their orbits and therefore, because both they and possibly you also are moving, you also need to synchronize all the clocks on the satellites with your clock; this synchronization means that you need one more point because you now have four unknowns you need to solve for, your three spacial positions and the data necessary to find the exact time. The GPS receiver in your iphone or whatever receives the data beamed from the four satellites and computes your position to remarkably good accuracy.

QUESTION:
According to Newton's law of gravitation, gravitational force is inversely proportional to the distance between the center of mass of the bodies. So if i place my hands together, the distance between them is very less so the gravitational force should be very high but it is easier to separate them. How?

ANSWER:
First of all, the force is inversely proportional to the square of the distance between the centers of mass. Because gravity is nature's weakest force, it is tiny unless there is a very large amount of mass. Let's estimate that the center of mass of your hands are separated by 1 cm=0.01 m and that the mass of each hand is about 0.25 kg (about lb). The force each hand feels can be approximated as F=Gm₁m₂/r²=6.67x10^-11x0.25x0.25/0.1²=4.2x10^-10 N≈10^-10 lb. As a comparison, the gravitational force on each hand by the earth (whose center is much farther away) is about 2.5 N.

QUESTION:
Is there an atmosphere in moon? If not, why?

ANSWER:
No. Because there is not enough gravity to hold it from escaping into space.

QUESTION:
Elements: Say you have the element hydrogen and it has its electron orbiting at a certain rate of speed and a certain pattern for this entire element. Then you have helium and it has its two orbiting electrons going at a certain rate and pattern, does the two orbit patterns take into consideration each other, in other words does the one electron orbit as if it were alone? Then take the element with three electrons do each electron orbit as if the others weren t there, or do they affect each other? Then consider an element where the electrons are orbiting as if the pattern was one element but didn t have the right number of electrons, what element would it be the correct orbiting one or the right numbered one.

ANSWER:
I should first warn you that if you want to understand the details of atomic structure beyond helium, you will have to give up the Bohr-atom, planetary model of the atom. This is a very primative picture of atoms and, while it opened the door to correct quantum mechanical calculations for atoms where we think of orbitals more like clouds of charge-mass around the nucleus, it is essentially an inaccurate representation. That said, the answer to the meat of your question is that any even approximate model of the atoms will include the repulsive forces among all the electrons as well as their attractive forces to the nucleus.

QUESTION:
How can two metallic objects having same but opposite charges (one loses electrons and one gain electrons) and we know that metals have the ability to lose electrons only?

ANSWER:
Actually, metals can form negative ions. But, that is beside the point. Electrons could be added to a conducting object and they would stay there even if they were in no way bound to atoms. The reason is that in a conductor, if you try to remove an electron from it, a virtual mirror image of the electron is formed which binds it. The energy necessary to remove the electron is referred to as the work function of the metal.

QUESTION:
Is dark matter and dark energy controlled by the brain? Does dark energy and dark matter have a brain?

ANSWER:
Well, my gut tells me to say no and no. However, since neither has ever been directly observed and we have no idea what they are, maybe I should say maybe and maybe!

QUESTION:
My son's friend asks: "why does a rolling object balance better than a stationary one?"

ANSWER:
I assume the friend is thinking about a bicycle which is, as we all know, easier to stay up on if it is moving. The physics of a bicycle is quite complicated, so I am not giving you the whole picture but rather one aspect which will be understandable to a youngster. Any object which is spinning has what is called angular momentum. Shown to the left is a spinning wheel with its angular momentum vector shown. If the wheel were not spinning, this vector would vanish. One of the most important laws in physics is conservation of angluar momentum: if there are no torques on the rotating object, its angular momentum will not change and that includes the direction of the vector. So, if you are riding a bike straight down the street, each wheel has a horizontal angular momentum pointing to the left. If the bike starts to tip over, the angular momentum vector would try to remain horizontal, keeping it from tipping. If you lean hard, there is now a torque on the system and so the angular momentum will change but not resulting in a fall but rather in a turn. You might want to get a toy gyroscope to show the kids how angular momentum works.

QUESTION:
Suppose an object is at rest. Its momentum is zero. According to Heisenberg uncertainty principle, the uncertainty in its position should be infinite, so that the product of the uncertainties of the momentum and position equals a non zero value. But what does this infinite uncertainty in the position mean? I mean the object is there, at rest, so don't we know its position exactly? Or is the uncertainty principle valid only for moving bodies?

ANSWER:
The lesson to be learned here is that you cannot have an object (perfectly) at rest. Infinite uncertainty means you have absolutely no idea where it is. If you make a measurement to determine its position, any place in the universe is equally probable to be where you find it. And since the universe is not infinitely large, you cannot have exactly zero momentum.

QUESTION:
A tall tree cracks and falls. Can the resultant linear acceleration exceed the acceleration due to gravity?

ANSWER:
As the tree falls, it rotates about the point of contact with the ground with some angular acceleration. Therefore, each point along the length of the tree has a different acceleration which is perpendicular to the tree. The falling tree is shown in the figure to the left. The weight mg acts at the center of gravity a distance D from the rotation axis. The tree has height L. An arbritrary distance up the tree is denoted by x. The angle the tree makes with the vertical is θ. The net torque τ on the tree is τ=mgDsinθ=Iα=Ia/x where I is the moment of inertia of the tree about its pivot point, α is the angular acceleration of the tree, and a is the tangential acceleration of the point x. Therefore, a=mgxDsinθ/I. Therefore, if mxDsinθ/I>1, a>g. Consider a simple example modeling the tree as a long thin stick; then I=mL²/3 and D=L/2, so a=3gxsinθ/(2L). Take a particular example, θ=60⁰; then a=1.3gx/L or any point with x>L/1.3 will have a>g.

ADDED NOTE:
The discussion above refers to the tangential acceleration of a point on the tree which is what I thought you were referring to. However, each point at position x also has a centripetal acceleration, call it a_c=v²/x=xω². You can get ω from energy conservation, mgD= Iω²+mgDcosθ and therefore a_c=2mgxD(1-cosθ)/I. So, the total acceleration is √(a²+a_c²); I will let you work that out. Modeling the tree as a uniform stick, I have calculated the total acceleration for several values of x shown in the graph to the right. For x=L/4, all angles have acceleration less than g. For x=L/2, the center of mass, a_total>g for angles greater than about 60⁰. For x=L, a_total>g for angles greater than about 35⁰.

QUESTION:
I'm writing a fantasy novel and I just want to check my science. In it there's a tower which is so tall that it is higher than the troposphere. There is a scene where someone smashes a window. My question is would the air all rush out due to unequal air pressure and when the air pressure settles would everyone feel the effects of explosive decompression or at least find it hard to breathe?

ANSWER:
Presumably, all your windows are sealed such that the inside is at atmospheric pressure. "Higher than the troposphere" would imply the stratosphere which is where commercial jets fly. As you doubtless know, the pressure there is very low. The figure to the right shows that just above the troposphere the pressure is only about 200 millibars, about 1/5 of atmospheric pressure. There is not enough air there to keep you alive. You may recall the crash of the private jet in 1999 which killed golfer Payne Stewart. The plane lost pressure at an altitude of 11.9 km and everyone lost consciousness; it flew on autopilot until it ran out of fuel and crashed. Most certainly the air would be drawn (violently) out of your broken window. Another thing you should think about is that you cannot simply pressurize your entire tower to atmospheric pressure; you have to pressurize in layers of a few hundred meters and isolated from each other. Otherwise you will have the same strong pressure gradient with altitude as the atmosphere has!

QUESTION:
Since the double slit experiment shows that light acts as a wave and is subject to constructive and destructive interference. I was wondering if the what we see in the night sky is really light streaming straight to us or is it the constructive interference of all the light waves out there?

ANSWER:
The bright spots you see as stars are not a result of constructive interference. However, the light you see is not all coming straight at us from the source because if light passes close to a very massive object it is bent; therefore some stars you see are not actually where they appear to be.

QUESTION:
If my friend were to travel toward me near the speed of light, then length contraction would mean he would appear smaller, correct? And from his POV I would appear smaller than him. So if I were to hold up my arm to the side of me and my friend were to run into it, what would this look like? From my POV he has shrunk and would therefore go under the height of my arm untouched. But from his POV, my arm would be near his knee height and he would trip up?

ANSWER:
You have stumbled on an example which argues that length contraction happens only along the direction of relative motion. So you and your friend are each thinner to each other but the same heights. (Regarding your expression "appear smaller", lengths do not necessarily appear shorter but they are shorter. See an earlier answer.)

QUESTION:
If I stick a pea on the out side of a cylinder that is rotating on a fixed axis at a constant speed, what are the forces being applied to the pea? Is the pea under going acceleration? Even at a constant speed due to the angular changes.

ANSWER:
The pea is experiencing what is called uniform circular motion. Its velocity is a vector tangent to the cylinder's surface. Although the magnitude of the velocity (speed) is not changing, the direction of the velocity is constantly changing. Therefore, since acceleration is rate of change of velocity, there is an acceleration. This acceleration, called centripetal acceleration, is a vector, points toward the axis of the cylinder, and has a magnitude v²/R where v is the speed of the surface of the cylinder (and therefore of the pea) and R is the radius. A force must be applied toward the axis of magnitude mv²/R where m is the mass of the pea. This force is provided by whatever adhesive you used to stick the pea there. If the adhesive is not strong enough, the pea will fly off. Of course there is also the pea's weight pointing vertically down and, assuming the axis of rotation is vertical, the adhesive must also exert a force upward to hold the pea from falling due to its weight.

QUESTION:
What would happen if you were to take a satellite that's in orbit, put a giant ball of string on it, and slowly unwind the string letting the free end fall to earth? Would it snap? What if it was made of a material that is extremely strong such as carbon nano tubes?

ANSWER:
Think about it. The ball of string is orbiting with you, has your same speed, and seems to be "weightless" just like you. So you grab onto the loose end and release the ball to fall and nothing happens, it just orbits along with you! Sort of related to your question is the space elevator which might interest you.

QUESTION:
Can you please tell me the difference between dark matter and dark energy.

ANSWER:
There are many instances where astronomical objects behave as if there were more mass present than we can see. The best-known example is the dynamics of galaxies where the speeds of stars far from the centers of the galaxies are much bigger than expected on the basis of the mass that can be seen; see the figure to the left. The conclusion usually drawn is that there is much more mass present than we can see and this hypothesized stuff is called dark matter. Nobody knows what it is nor has it yet been detected directly. A second anomalous feature of the universe is that it has recently been discovered that the expansion of the universe is actually accelerating; the conclusion usually drawn is that there is a repulsive force not heretofore observed which is "winning" a tug-of-war with the attractive force of gravity. This is referred to as dark energy.

QUESTION:
Can you tell me in an everyday comparison how much energy is in 200 Mev?

ANSWER:
200 MeV=200 MeV[1.6x10^-13 J/MeV]=3.2x10^-11 J. Suppose that I doled out 1000 200 MeV packages each second. Then the rate of energy being delivered would be 3.2x10^-8 J/s=3.2x10^-8 W, 32 nanowatts, pretty small! To power a 100 W light bulb, you would have to deliver 3.1x10¹² 200 MeV packages each second, 3.1 trillion. Here is another way to envision it: taking the acceleration due to gravity to be g≈10 m/s² and the potential energy for an object of mass m lifted to a height h to be U=mgh or h=U/(mg), you could lift a 1 gm=10^-3kg mass to a height h=3.2x10^-11/10^-2=32x10^-10 m, about 30 times the size of an atom, with 200 MeV of energy.

QUESTION:
When nucleons come together from large separation, there is a release of energy called binding energy. Where does this energy come from? If it comes from the mass of the nucleons,then how does the total no. of nucleons remain conserved? If it comes from a part of a nucleon(say proton) then after a part of mass of that proton is lost(as energy) shoudn't we call it anything but 'proton' ?

ANSWER:
If you make a ball out of wood instead of lead, you still call it a ball, don't you? The mass of a nucleon in a bound system is simply less than it is if it is not bound. The reason is simple E=mc²; in order to pull a bound nucleon out of a nucleus you must do work, i.e. add energy. Where does that energy go? It goes into the mass. In most respects, nucleons in nuclei retain their identities. We know this from nuclear spectroscopy, the energy levels we observe in nuclei. However, don't forget that nucleons are composed of quarks and, to a small extent, the nucleus can behave as a collection of quarks where nucleons may share and exchange quarks. But this is a very small overall effect.

QUESTION:
I was thinking about the particle colliders we have and how how small are the amounts of mass they accelerate. So how small of a fraction of a gram is being accelerated in the particle colliders around the world? Like is a nanogram a good estimate of how much the masses collided total?

ANSWER:
I will use the LHC as an example. The average beam intensity is about 4x10¹⁸ protons per second and the mass of a proton is about 1.7x10^-27 kg. So the mass per unit time would be about 7x10^-9 kg/s=7x10^-6 g/s=7 μg/s.

QUESTION:
Does the AC current has a DC part?

ANSWER:
It can have if the time-averaged current is not zero.

QUESTION:
How does the quark composition change in beta minus decay/beta plus decay?

ANSWER:
β^- decay essentially changes a neutron into a proton, so the quark composition becomes that of a proton instead of that of a neutron. β⁺ decay essentially changes a proton into a neutron, so the quark composition becomes that of a neutron instead of that of a proton.

QUESTION:
Why does mass not alter the acceleration in a vacuum. If a hammer falls to the earth faster than hammer falls to the moon, then mass does matter. Consider the opposite perspective. Earth falls to the hammer faster than the moon falls to the hammer. Am I wrong in my reasoning? I've asked a few of my physics teachers, but they said that my logic was flawed and you can't consider it that way without explaining why.

ANSWER:
You are completely misunderstanding the constance of acceleration due to gravity, independent of mass. The statement assumes all masses experience the same gravitational field (see FAQ page). Obviously, because of Newton's second law, the acceleration a due to any force F depends on mass m, a=F/m. The "falling" of the moon or earth to the hammer is negligibly small for all practical purposes. However, you could calculate the initial acceleration of each. The force on the earth or moon is F=MmG/R² where M is the mass of the earth (moon) and R is the radius of the earth (moon) (assuming the hammer is close to the surface). So the initial acceleration of each would be a=F/M=mG/R² and therefore a_moon/a_earth=(R_earth/R_moon)². So, the moon actually accelerates toward the hammer faster but it has nothing to do with the mass as you can see, it is because the center of the moon is much closer to the hammer than the center of the earth is. If you were to place the hammer such that it was a distance R_earth from the center of the moon, the earth and moon would have equal accelerations. The earth and moon are responding to the gravitational field of the hammer. So, you are wrong on all your statements and, guess what, your physics teachers are right!

QUESTION:
We know that time is relative to movement, and if we could move at the speed of light time wouldn't pass. We also know that the Earch is moving around the Sun at 108,000 km/h, the Solar System is moving through the Milky Way at 72,000 km/h and the Milky Way is moving through space at 828,000 km/h (wonder who measured that one)... or at least that's what Google says the speeds are. So, my point is, we're not still, at all. So, what would happen if somebody could somehow teleport to outside the galaxy and remain in space, completely still, not affected by all of these movements. How would they perceive time in that case? What would happen if the were to return to Earth after a year passed, both on Earth or from the perspective of the person standing still.

ANSWER:
First of all, you need to appreciate that there is nos such thing as "completely still". All that matters is what your motion is relative to another with which you compare. You should read an earlier answer to appreciate this. I have not checked your numbers, but they are all small compared to the speed of light, about 1,090,000,000 km/hr, so the time dilations among the various frames you refer to, will be very small.

QUESTION:
I have been trying to find an answer to a question for some time now. My question is: Can you impart angular momentum to an atom? Whenever I look for information on this I end up with questions of SPIN which when speaking of atoms as you know means something totally different then what I'm asking. I'm wondering if you could "spin" an atom such that its centrifugal force is strong enough to overcome the nuclear bonds of the atom?

ANSWER:
Spin is a kind of angular momentum and certainly not unrelated to what you are asking. But, let's just confine the answer to what you are probably thinking about orbital angular momentum. Any state of a nucleus has some amount of angular momentum. Just think about a little electron orbit: it certainly has angular momentum. The orbital model is naive, but it gives you the idea that atoms have angular momentum. All even-Z atoms have zero angular momentum in their ground states. There are countless ways you can excite an atom, usually by just shooting something at it, and if you excite it to a state which does not have zero angular momentum, you have imparted angular momentum to it. However, the more angular momentum the atom has, the bigger it is and, as far as I know, it never "flies" apart due to some centrifugal effect; the way to make it fly apart is to give it a lot of energy, not a lot of angular momentum. However, molecules have states which are different from just the orbital motion of the electrons. Imagine a diatomic molecule like O₂, for example. There are states which are rotational states, that is the molecule rotates like a dumbbell. As you give the rotating molecule more and more angular momentum it experiences centrifugal stretching, just like you would expect. If you give it enought angular momentum it will eventually break the molecular bond.

QUESTION:
Is there any practical event in nature which can help to understand the reason for the square of the velocity of em waves(c2) as the constant of equivalce in e=mc2

ANSWER:
Do you mean, like, why isn't it mc or mc³ or m√c? In science, any quanty must be expressed in the appropriate units. The units of energy are Joules (J), and 1 J=1 kg∙m²/s². So, for example, if E=mc, the units would be kg∙m/s which would not be the units of energy. To understand why it is not, say, E= mc², you would need to understand the physics which leads up to the final result.

QUESTION:
How can a nucleus have two options to undergo radioactive decay- either by alpha or beta minus decay. ONe process increases the neutron to proton ratio, while the other decreases it. the nucleus must also become unstable by one of the two things- either increase that ratio, or decrease it. then why the two options?

ANSWER:
What matters is the energetics, whether all the mass energy before the decay is greater than all the mass energy after the decay; that way, there will be some energy left over for the kinetic energy of the products. There is an old saying in physics: "Anything that can happen will happen!"

QUESTION:
I am trying to write a science fiction book, and I've come up with a weapon to end the genocidal war between two species. Would a five meter wide rail gun round, weighing ten tons, traveling at half the speed of light create the kind of horrific damage to a planet I imagine it would? What I foresee from my limited understanding of physics is that there would be at a minimum a crater hundreds or even thousands of miles wide, and deep. The overpressure wave would be tens of thousands of pounds per square inch and wrap around the globe, say a rocky planet with a bit less water than earth. Trillions of tons of material thrown into the atmosphere, and possibly a fracturing of the surface of the planet along any fault lines. I can even imagine that the planet might lose enough momentum to slowly (over tens of thousands of years) degrade enough to fall into the star.

ANSWER:
I will take the mass to be 10 metric tons, 10⁴kg. Your projectile would have a kinetic energy of K=mc²[(1/√(1-.5²))-1]=1.4x10²⁰ J. The most powerful atomic bomb ever detonated had an energy of about 200 TJ=2x10¹⁷ J. Your projectile has the energy of about 700 such bombs. This is certainly a lot of energy, but I somehow doubt that the result of such a projectile would be as drastic as you suggest. On the other hand, this is science fiction and you could probably get away with this without being called crazy! Your second hypothesis about the "degraded" orbit is physically wrong. A planet's orbit is not a delicate balance such that if its momentum were to change a little it would spiral into the sun. A slight change in momentum of a planet will result in a slight change in the shape of its orbit, but the orbit would still be stable. And, although I have not calculated it, the change in momentum as a result of such a collision would be very tiny.

QUESTION:
Why does the area under a velocity time graph represent distance and how can I use it to work out the distance of a non linear graph?

ANSWER:
This is essentially integral calculus. If you know integral calculus then v(t)=dx/dt >x₂-x₁=∫v(t)dt=area under v(t) between t₁ and t₂ and the integral is over the limits t₁ to t₂. If you do not know calculus, try this: In the graph to the left is shown some velocity function v(t). Now, a bunch of little rectangles are drawn and the width of each rectangle I will call Δt and its height is, of course, v(t) at its left edge. But, v(t)=Δx/Δt because that is the definition of velocity. Therefore, the area of that rectangle is (Δx/Δt)Δt=Δx, approximately the distance traveled during that Δt. And, if you add up the areas of all the rectangles, you will get approximately the area under the curve or the distance travelled over the time interval t₁ to t₂. Of course, this is only approximate because you can see that the area of each rectangle is not exactly the area under that segment of the curve. However, if you make Δt vanishingly small (which is what integral calculus does), you will get the area under the curve.

QUESTION:
Is conservation of energy can be violated? Please give me an example?

ANSWER:
Like all laws of physics, conservation of energy is only true under very specific circumstances. The law is stated that the total energy of a system is conserved if there are no external forces doing work on the system. So, if you throw a baseball, its energy is not conserved since it started out at rest, then your hand did work which gave it kinetic energy, and later it is again at rest because other forces did work which took its kinetic energy again. But the total energy of an isolated system will be conserved. Also because of the uncertainty principle, energy conservation may be violated but only for very short times. Approximately, ΔtΔE<ħ where ħ≈10^-34 J∙s. So this means that the energy of a system could increase by 10^-34 J as long as it went back before 1 s had passed.

QUESTION:
I just figured out that there is only one direction of circular rotational motion of planets, opposite direction of motion is simply result of relativity. Is this true?

ANSWER:
What is true is that, with very few exceptions, if you view the solar system from the polar star, nearly all rotations are counterclockwise. I have absolutely no idea what you mean by " is simply result of relativity."

QUESTION:
Is it true that if you have two objects and nothing else you can't tell which object is moving and which object is standing still? Does this mean that we don't know how fast earth is moving? And there is no experiment we can conduct to see if we are moving or not?

ANSWER:
First, you have to understand the difference between inertial and noninertial frames of reference. If Newton's first law is true where you are, you are in an inertial frame of reference. (Newton's first law says that if an object is at rest in your frame the sum of all forces on it is zero.) Any other frame which moves with constant speed in a straight line relative to your frame is also an inertial frame. Noninertial frames are any frames which have an acceleration relative to an inertial frame. The principle of relativity says that the laws of physics are exactly the same in all inertial frames of reference. Since the laws of physics are what will determine the result of any experiment you can do, it makes no sense to refer to an object's being at rest unless with reference to something else. Does this answer your question? Also, we do not know how fast the earth is moving because that is a meaningless question unless we say, for example, how fast is it moving relative to the sun, or how fast is it moving relative to the center of the galaxy, etc.

QUESTION:
Why is a hard ball more likely than soft ball of equal mass and volume to break a glass window if they are thrown at the same speed?

ANSWER:
The average force F which an object exerts during a collision is the change in momentum (Δp=mΔv) divided by the time the collision took Δt. The soft ball has two things going for it. First, because it is "squishy", the collision lasts longer, so Δt_soft>Δt_hard; second, the soft ball is probably less elastic than the hard ball so that it loses more energy in the collision and therefore bounces back with less momentum resulting in Δp_soft<Δp_hard. Therefore Δp_soft/Δt_soft<Δp_hard/Δt_hardor F_soft<F_hard.

QUESTION:
I recently saw the movie Elysium. The most memorable thing was the space station. The station was a completely open system. Would the station's rotation keep its own atmosphere contained? How would a spacecraft land on a rotating ring like this? Would radiation levels just fry everyone and everything on it?

ANSWER:
The rotation causes an artificial gravity. If there were side walls, you could certainly keep an atmosphere in there. For a detailed discussion of how living there would be, see an earlier answer. The way the gravity works is that it is a centrifugal force (a fictitious force) and you want the acceleration at the outer rim to be equal to g=9.8 m/s²; the acceleration at the surface is v²/R where v is the tangential speed at the surface and R is the radius of the ring. So, v=√(g/R). If we take, just as an example, R=1 kg=1000 m, then v≈0.1 m/s; since the circumference is 2πR=6283 m, this means that the ring would rotate about once every 63,000 s=1.7 days. I think you would agree that this speed is very small, so a spacecraft would have no trouble landing on it assuming that the spacecraft had gotten up to the same orbital speed. I do not believe that the radiation level would necessarily "fry" everyone, but it would be a concern for the long term and some kind of shielding would have to be employed.

QUESTION:
If an object had zero mass (technically it could not exist) but for the sake of the question let's assume it can, and could withstand any physical affliction and accelerated at 1 km/h would it accelerate at an infinite speed or travel a certain speed only?

ANSWER:
An "object" with zero mass can exist; it is called a photon. However, you must be thinking of this object classically using Newton's second law, a=F/m and so any force will cause an infinite acceleration. In fact the theory of special relativity requires that any object with zero mass can move only at the speed of light in vacuum. So a massless object at rest cannot exist.

QUESTION:
Why can't UV-B and UV-C rays pass through glass but UV-A rays can?

ANSWER:
I am sure that this will not be a very satisfying answer to you, but that is just the way it is. Every material has a characteristic absorption spectrum where small numbers mean mostly not absorbed and large numbers mean mostly absorbed. What determines the wavelengths which are absorbed and those which are not is determined by the molecular structure of the material, how it interacts with electromagnetic radiation. This is not simple to calculate, even for the simplest assumptions and the simplest materials. The absorption spectra for two different kinds of glass are shown to the left. Since UVA is nominally defined as about 315-400 nm wavelength and UVB and UVC nominally span 100-315 nm, you can see that glass is mainly transparent to UVA and much less so to UVB and UVC.

QUESTION:
I have been attempting to teach myself chaos theory, however I have had trouble understanding it and how it is involved with different levels of quantum physics as well as relativity. I am also having trouble understanding the "three body problem", which seems to occur in many different physical systems. I was hoping that you could help me to understand at least some of Chaos theory and how it connects to both quantum physics and relativity, and what exactly the "three body problem" is.

ANSWER:
I am sorry, but your question is too technical and too unfocused for the purposes of this site. I can tell you something about the 3-body problem, though. If two bodies interact only with each other, for example the earth and the moon, you can write the orbits in simple analytic closed form. However, if there are three interacting bodies there is, in general, no closed-form solution. There are special cases where the 3-body problem can be solved, for example if one of the bodies is held fixed, but not in general. If you google "three body problem" you will find more information. A particularly interesting (and newsworthy) discussion may be read in AAAS Science News. All these special cases are not chaotic because they repeat in a periodic way. More general cases are not periodic and are extraordinarily sensitive to initial conditions.

QUESTION:
according to newton law of motion "a body will move if a net force act on it" then why the earth moves around its axis?

ANSWER:
Whoa! You have Newton's first law all wrong. A body will move with constant velocity if there are no forces on it. For translational motion, this means that the body moves with constant speed in a straight line; if you exert a force on the body, its velocity will change. For rotational motion, this means that the body spins with constant speed in a straight line; if you exert a torque on the body, its spinning speed will change. The earth rotates on its axis with constant speed because there are no torques on it.

QUESTION:
Does Einstein's theory of relativity account for light having properties of a solid? I remember from high school the simple experiment of putting a twirlers in a vacuum, placing it in sunlight and it twirling. The current relativity theory states as an object approaches the speed of light, its mass is increasing exponentially and time is slowing. But if light has properties of a solid, how is that factored into Einstein's equations? Is it true all rays do not have this distinct characteristic of acting on an object as a solid as demonstrated in the simple h.s. experiment?

ANSWER:
I think you must mean that light behaves like a particle, not a solid; indeed Einstein, not in relativity but in explaining the photoelectric effect, showed that electromagnetic radiation was quantized, that is it consists of photons which have energy E=hf where h is Planck's constant and f is the frequency of the light. A photon also has a linear momentum p=hf/c (that does come from the theory of relativity) where c is the speed of light. The fact that photons have momentum means that they can exert a force and this is the usual explanation for Crooke's radiometer which is the experiment I believe you are alluding to in your question. Here is the funny thing, though: radiation pressure has nothing to do with what happens in the Crooke's radiometer. It is not nearly sensitive enough to observe radiation pressure, it is rather a thermal effect as explained in the Wikepedia article I have linked to. Radiation pressure is real, though, and the more sensitive Nichols radiometer can observe it.

QUESTION:
If we have a car driving at a fixed distance (e.g. 100 km), does it cost more energy to reach the destination at 100 km/hr versus 50 km/hr?

ANSWER:
Certainly. The air drag on something moving through air is approximately proportional to the speed squared, so the 100 km/hr car has 4 times the air drag to overcome. Of course, you do not use 4 times as much fuel because there are lots of other energy losses which do not depend on velocity, but you do use a significant amount more fuel.

QUESTION:
These are non-academic, practical questions regarding the physics of balance and weight shifting pertaining to a moveable kitchen island.
Q1: Will an 11 inch countertop overhang alone cause the island to tip?
Q2: If not, what amount of weight (lbs.) can be safely placed on the overhang before tipping?
Q3: Is there a formula I can use to calculate this? Description: I have a moveable kitchen island fabricated with locking casters. I wanted to place a quartz countertop over the island base with an additional 11 inch countertop overhang supported by steel beams extending from the island base. The total length of the countertop is 37 inches (26 inches on the island base plus 11 inches as the overhang).

Proposed dimensions of the island base with its portion of the quartz countertop:
W = 52.5 inches
L = 26 inches
H = 36.0 inches (34.5" base + 1.5" countertop which includes a 5/8 inch plywood sub-counter)
Total Weight of the Island Base with Its 26 Inch Portion of the Countertop = 298 lbs.

Proposed dimensions of the 11 inch overhang:
W = 52.5 inches
L = 11 inches length
H = 1.5 inches (2cm quartz plus 5/8 inch plywood sub-counter)
Total Weight of the 11 Inch Overhang Portion of the Countertop = 50 lbs.

The overhang is a key feature of the kitchen island for our household. The island is intended to serve multiple, mundane purposes in our very compact home: food prep, dining, and working on work projects / homework.

ANSWER:
I will assume that the center of gravity of the island without the overhang is at the geometric center of the base and that the casters are at the corners; also, that the center of gravity of the overhang is at its geometrical center. The red vectors in the figure to the right are pertinent forces for this problem, the 298 lb weight of the island acting at the center of gravity (star), the 50 lb weight of the overhang acting at the center of gravity (5.5" out), the force of the floor on the front casters (N₂), and the force of the floor acting on the rear wheels (N₁). (Ignore the force F for now.) Newton's first law stipulates that the sum of all the forces must be zero, so N₁+N₂=348. Also required for equilibrium is that the sum of torques about any axis must be zero; choosing to sum the torques about the front casters, 26N₁-298x13+50x5.5=0=26N₁-3596 or N₁=138 lb and so N₂=210 lb. Now, let's think about this answer: it tells you that this (unladen) island will not tip over because there is still a lot of weight on the rear wheels. Now, if you start adding weight to the overhang, eventually when you have added enough weight, the force N₁will equal zero when it is just about to tip over. So, add the force F at the outermost edge of the overhang and find F when the island is just about to tip: again summing torques about the front casters, -298x13+50x5.5+11F=0 or F=327 lb. This is the extreme situation you would have to put twice this amount of weight, for example, halfway out the overhang to tip it over. It looks to me that this will be safe for everyday use.

FOLLOWUP QUESTION:
The questioner sent an extremely lengthy recalculation of distances and weights. The only substantive changes were: weight of island without overhang, W=414 lb; weight of overhang w=58 lb; distances of casters from sides d=5.5"; distance of center of gravity from back side D=14".

ANSWER:
The relevant equations to redo the calculations for the unladen island in the original answer are
   N₁+N₂=W+w and
   (26-2d)N₁-(26-D-d)W+(d+5.5)w=0.
I find N₁=137 lb, so the unladen island will not tip. For the second part of the calculation, adding the force F such that N₁=0, the relevant equation is
   (11+d)F-(26-D-d)W+(d+5.5)w=0.

I find F=124 lb at the outer edge to tip the island. Again, a force of 248 lb in the center of the overhang would tip it. These forces are considerably smaller than the original calculation mainly because of the relocation of the casters closer to the center of gravity and, to a lesser extent, the moving forward of the center of gravity. Incidentally, it may seem that the vertical positon of the center of gravity would matter. It does not matter if the island does not tip; if it does begin to tip, it will tip faster if the center of gravity is higher, but not sooner. Since there is no chance that the island will tip sideways, the location of the center of gravity along the long edge is not relevant. Finally, if you are uncomfortable with the amount of weight to tip, I presume that you could add weight inside and near the back-bottom edge as a counter balance. For example, putting 100 lb at the back edge would increase F to 249 lb. (Anything you do which shifts the overall center of gravity closer to the back side will help stabilize against tipping.) You should also be aware that if you move it by pushing forward at the top of the back side, the resulting torque could tip it over, so be careful when moving it. I believe that if it were mine, I would look for a design modification which would move the front casters closer to the front.

ADDED NOTE:
The questioner added some plans, one of which is shown to the left. Note that there is a kick plate recessed by 2" all around. Presumably this plate hides the casters and is very close to the floor. Therefore, as soon as the island would start to tip, these would become the pivot point rather than the caster itself which would make make it less vulnerable to tipping. Essentially, in the calculations above you would reduce d by 2" to d=3.5". This would result in a value of F=207 lb, quite an improvement. You can see why I indicated above that moving the casters in by 5.5" was the main culprit in increasing "tippiness". (I am curious how, if the kick plates hide the casters, you are able to lock them.)

QUESTION:
I'm trying to compare 2 measurements that denote impact. The first measurement is as follows : a glass sheet can withstand a 25mm (diameter) steel ball fired at 80 km's per hour. The second measurement is that another sheet of glass can withstand a 277 gram steel ball dropped from 1 metre in height with a back wind of 60 meters per second. I am trying to bring the second measurement to a measurement comparable to the first measurement.

ANSWER:
The thing which will matter is the linear momentum (p=mv) each ball brings to the glass. The reason is that you are interested in how much force each glass can withstand and the force is the rate of change of momentum. I assume that each ball will spend about the same amount of time during the collision and will exert its force over the same area (very nearly a point for a sphere on a plane), so whichever ball has the most momentum when it hits the glass will indicate the glass with the greatest strength. I took the mass density ρ of steel as 8000 kg/m³. The volume of a sphere is 4πR³/3, so m₁=4ρπR₁³/3=4∙8000∙π∙0.0125³/3=0.0654 kg. Since the speed is given, v₁=80 km/hr=22 m/s, we can immediately write the momentum for #1, p₁=1.44 kg∙m/s. Finding the speed of the second ball is a much more difficult problem. If it were just falling, it would be trivial. But it is being pushed by the downward wind which will make it speed up faster than just falling; so, it is necessary to understand a little about air drag forces. For spheres of normal speeds in air the force of friction is excellently approximated by f=0.22D²u² where D is the diameter and u is the speed of the ball relative to the air. From the density and mass, I find the radius to be 0.0434 m so D=0.0868 m. So, when the ball is first dropped, it has two forces pointing down, its own weight mg=0.277∙9.8=2.71 N and the wind force 0.22∙0.0868²60²=5.97 N; the wind is more than twice the force as the weight. As it falls, it speeds up and so the effect of the wind gets smaller. Newton's second law for v₂, if you care, is now of the form dv₂/dt=g[1+((v_w-v₂)/v_t)²] where v_t=√(mg/(0.22D₂²)) and v_w is the speed of the wind. The solution to this equation is v₂=v_w-v_ttan[tan^-1(v_w/v_t)-(gt/v_t)]. Putting in the numbers for this situation (v_w=60 m/s, v_t=40.5 m/s), the graph for the first 15 s, with and without wind, is shown to the left. The behavior of this graph is interesting. The ball takes about 4 s to reach a speed of 60 m/s; at that point the ball is at rest relative to the air and so there is no air drag and the slope of the curve (which is acceleration) is the same as the curve for no wind at all, as expected. Now, if there were no wind it would take the ball about 0.45 s to fall 1 m and the wind will surely get the ball there in a shorter time; therefore we are really only interested in the first half second of the fall and this is shown to the right. The acceleration over this time is very nearly uniform, about a₂=11/0.4=27.5 m/s²; since this is just an estimate, I will do the calculation assuming uniform acceleration rather than doing the exact calculation to find the velocity after 1 m. I find that the time to reach 1 m is t=√(2/27.5)=0.27 s and so v₂=at=7.4 m/s. Therefore, the momentum for #2 is p₂=m₂v₂=0.277∙7.4=2.1 kg∙m/s. Ball #2 is the winner!

ADDED THOUGHTS:
In retrospect, I could have saved myself some work if I had thought about the fact that in a time less than 0.45 s the acceleration would be essentially constant and simply written 0.277a=2.71+5.97 > a=31.3 m/s² which would give t=0.25 s and v₂=7.9 m/s and p₂=2.2 kg∙m/s. Secondly, I noticed that my solution for v₂ was incorrect for speeds greater than 60 m/s, so I deleted that part of the graph. To satisfy my own curiosity, I solved the problem for speeds greater than 4 s. Because at later times the speed of the ball is greater than the speed of the wind, the air drag force switches to up rather down so the analytical solution to the problem becomes different, v₂=v_w+v_ttanh(g(t-4)/v_t); this corrected calculation is shown in blue. The complete solution is now graphed to the left. At large times, the solution approaches v_t+v_w as expected since v_t is the terminal velocity in still air.

QUESTION:
I have a question about the concept of angular momentum. Suppose a disc is attached to a pole and they're stationary in deep space. If we spin the disc, it's gonna have an angular momentum. Using the right thumb rule to determine the direction of which, does this mean the unit will start moving in said direction? Also, how does the spinning action produce a momentum perpendicular to the force we exerted to cause the spin?

ANSWER:
I am assuming the pole is attached axially, i.e. at the center and perpendicular to the disk. Angular momentum is not a force and therefore it will not cause the system to start moving. Also, it was not the force you exerted which caused it to spin, it was the torque (see left), and the direction of the torque τ is also given by the right hand rule such that the angular momentum L points in the direction as the torque (see right).

QUESTION:
Is it possible to obtain a person's weight, with a certain degree of accuracy, using an accelerometer and having the person walk/jog/run a certain distance or walk down a flight of stairs?

ANSWER:
An accelerometer can be used to determine the mass M of a person, but only if you know the force F being applied on that person at the instant that you measure the acceleration a. This is just Newton's second law, M=F/a. In none of the examples you site do you know the force. If you do find the mass, you can find the weight by multiplying the mass times the acceleration due to gravity.

QUESTION:
An accelerating rocket ship, with an observer at the top (with a clock) and an observer at the bottom (with a clock) will observe the other's clock running at a different rate from than their own. The observer at the top of the rocket will see the "bottom" observer's clock running slow, of course this difference is going to be incredibly small, but we have really good clocks. The observers also have accelerometers. If the top observer views the bottoms observers accelerometer to be the same as his, acceleration same on top as bottom, but the bottom observers clock running slower than his, how would the observers reconcile the fact that there is no relative velocity between the two? The same acceleration, over different amounts of time would result in a relative velocity between the two, but how can there be a relative velocity between the two of them? This can't be a "reading of the clock" error, as in the bottom observer's reference frame, his clock is running fine, it is the top observer's clock that is running fast. Would you have to average the times, and apply this time to the center of mass of the rocket?

ANSWER:
First, let's be sure we understand why the clocks run at different rates. If we apply the equivalence principle, there is no experiment that can be done on this accelerating rocket ship which can distinguish between acceleration a and being at rest in a gravitational field with local gravitational acceleration a. Therefore, it is as if the two were at rest in a uniform gravitational field. Then it is a consequence of general relativity that the clock "higher" in the gravitational field will run faster. Now, you suggest having accelerometers at each end and that they will record the same; that is true because it is no different from being at rest in a uniform field and measuring the field at both ends. Now, if you are at rest in a field, there is no problem because there is no acceleration and therefore no velocity change. So the results are required (by the equivalence principle) to be the same if you are in a zero field with an instantaneous acceleration. I think the problem is that since acceleration is not really a useful quantity in relativity, you should not conclude that equal accelerations in unequal times necessarily implies a relative velocity between two observers in the same frame. In fact, it clearly cannot because both are at rest in the same frame.

FOLLOWUP QUESTION:
One of the stipulations of the Eqivalence Principle, is that that you can not distinguish between an acceleration of a frame at "g" and being in a UNIFORM gravitational field of the same "g". The word Uniform here implies that the acceleration field at point "a" is the same value as the acceleration field at point "b" some distance away, in other words, no gravity gradient. But planets do have gravity gradients ( although the earths is a weak gradient). If I was in a gravity gradient, that was really steep, I would feel this gradient as my feet being pulled at with a different acceleration then my head. How does a steep gravity gradient change the equivalence principle?

ANSWER:
If the field is not uniform, it is just a little harder to compute the relative rates that clocks run in the field. What determines the rates at two points in space is the potential difference between the two points. It does not change the equivalence principle. There is still no experiment you can do to determine whether you are in a uniform field or an accelerated frame. Incidentally, the effect of field gradient is called the tidal force. You may have read somewhere that when you fall into a black hole, you get "all stretched out" as you fall; this is due to the extreme tidal force from one end of your body to the other.

QUESTION:
I'm a young aeronautical student and I'm doing a project on small, basic fixed-wing aircraft. I need to include some explanation of how lift is generated. My teacher has vaguely mentioned the Bernoulli theory once or twice. However after some research, I found that the Bernoulli explanation is outdated. I've found other more accepted theories, only which are too complex for both my understanding and academic level. Could you provide a more accurate but relatively simplified explanation of lift?

ANSWER:
I wouldn't say "the Bernoulli explanation is outdated," it just isn't the whole explanation. Basically, Newton's third law is responsible for much of the lift in flight the air coming off the trailing edge of the wing is deflected down which means the wing exerted a downward force on it which means it exerted an upward force on the wing. Books about flying usually refer to this as "angle of attack." A more complete explanation may be found in an earlier answer. I can also recommend a book, Stick and Rudder by Wolfgang Langewiesche.

QUESTION:
I am a writer involved in the creation of exhibitry for the Telus Spark science museum in Calgary, Alberta. We're building a new gallery about electricity. We have an exhibit about the problem of so-called Vampire Power--the constant sipping of electrical energy by home appliances on standby mode. I'm just a writer, not a physicist, but I have posited that many of our energy conservation problems are not actually problems during the winter months, when all the homes in the city of Calgary are being heated. My reasoning is that all of the electrical energy consumed by everything from coffee makers to the charger for your mobile phone to the TV just end up as waste heat, anyway. And that heat will be that much less heat your furnace (or baseboard heater) has to put out to keep your house warm. So this waste energy is not really wasted at all. Now, I guess that for lightbulbs and televisions and other things emitting light as part of their function, you want to be sure that your curtains are closed so that light energy is not escaping out of the windows and into the streets, where it ends up as heat outdoors, and not in your house where you want it. And of course, in summer, when these same houses are air-conditioned, the waste heat from these appliances is counterproductive, and should be reduced as much as possible. The designers and project administrators are skeptical of my reasoning. They're saying that a TV is a less efficient way (energetically) to produce heat than an electric baseboard heater. But I say it doesn't matter, because all the energy ends up as heat anyway. Even the sound from your TV will bounce off the objects in the room, heating them up. Is there a flaw in my reasoning?

ANSWER:
Let me start out by saying that you are certainly correct, all electrical power eventually ends up as heat. That said, I think that suggesting that the wasted power should not be worried about is misguided for the following reason. The "vampire power" used by the standby mode of many appliances and electronics is really rather low, probably normally a few watts. Such a power source for heating your home would probably only account for a temperature increase of way less than one degree. But, the thermostat connected to your heating system is really not sensitive enough for there to be any difference at all which would result in lower energy consumption by your heating system. In other words, with or without the vampire heat, your heating energy use will be the same. On the other hand, there are thousands of other homes also consuming a few wasted watts of power. If they all stopped there would be kilowatts of power not being used but nobody's heating bill would be changed. This is only the result of my thinking about your question a bit before answering it, I have not seen any similar conclusions.

QUESTION:
Does different surface area with the same mass affect the amount of kinetic friction?

ANSWER:
The simple answer is no, the kinetic friction depends only on the composition of the objects sliding on each other (e.g., wood sliding on concrete) and the normal force pressing them together. However, friction is never simple and can never be represented exactly. The equation we learn in introductory physics courses, f=μN, is only approximately true for many situations, not a law of physics. (By the way, this question is of most interest in regard to static friction rather than kinetic friction. For tires, for example, we want to minimize the change of a car skidding.) For a much more detailed discussion, see an earlier answer.

QUESTION:
If it was possible for every vehicle in the world to point East, and at perfect timing, all accelerate at the same time, surely the torque that is put down on the Earth would affect it's spinning energy?

ANSWER:
Yet another chance to demonstrate what a tiny speck we are in this universe! There are about a billion, 10⁹, vehicles in the world. Suppose the average mass is 1000 kg (about 3000 lb) and the average acceleration is about 5 m/s² (half of g); so the average force per vehicle is about 5000 N and the total force on the earth is therefore 5x10¹² N. Suppose the average vehicle is at about 45⁰ latitude, so the net torque is about τ=5x10¹²R/√2=2.3x10¹⁹ N∙m where R=6.4x10⁶ m is the radius of the earth. The angular acceleration is then given by α=τ/I where I is the moment of inertia of the earth, about 8x10³⁷ kg∙m/s². So, α=2.3x10¹⁹/8x10³⁷=3x10^-19 radians/s²=5x10^-18 revolutions/s²=4x10^-8 revolutions/day². If all your vehicles could maintain this acceleration for a full minute, (certainly not possible), the length of the day would shorten by 5x10^-12 day=4x10^-7 s.

QUESTION:
Can you explain the effect of rifling of the muzzle in rifles ?? I just know that the muzzling is done to impart spin to the bullet when it is fired ?? So, exactly how does the spin imparted to the bullet improves it's aiming accuracy or whatever it does ?

ANSWER:
An object, like the bullet, which is spinning about an axis along its length has angular momentum. The angular momentum vector points along the direction the bullet is flying. An important law in physics is the conservation of angular momentum which says that the angular momentum of an object never changes if there is no torque acting on it. So the angular momentum will continue pointing along the path which means that the bullet will not "tumble". It is the same principle which governs the spiral forward pass in American football. Without spiraling, the tiniest asymetry in the shape of the bullet will cause it to immediately start tumbling when it leaves the barrel.

QUESTION:
Just what is the difference between interference and superposition of light( or any wave, for that matter )...???

ANSWER:
Usually superposition refers to the principle that two waves at the same place and time can be added linearly. Interference usually refers to the observable effects of superposition.

QUESTION:
When I light a torch, why is there a portion in the middle which is dark ?? is it due to diffraction ??

ANSWER:
(For my American readers not familiar with British vernacular, a torch is a flashlight.) No, it is simply imperfections in the optics, in particular that the light bulb is not precisely in the right place (at the focus of the parabolic reflector). Better torches allow for adjustment of the position of the bulb to minimize the dark spot in the center.

QUESTION:
Suppose we have a thick spherical metallic shell with a spherical cavity inside. A charge is placed at any point in the cavity except for the centre of the sphere. Now how do we find the potential of outer surface of the shell? My friend says that no matter where we place the charge inside the cavity, the potential will be the same, as if it is placed at the centre. But he does not have any logical proof to the statement. Is he right? Then what is the proof? If not, how do we go on calculating the potential?

ANSWER:
This question can be answered in a purely conceptual way. Because the electric field in the conductor must be zero and the induced charge on the outer surface must be Q (the same as the point charge), the surface charge distribution must be uniform. In fact, the conductor and the cavity do not even need to be spherical for the field outside to be independent of the position of the charge inside.

QUESTION:
With all the talk of new weapons using kinetic energy as the destructive force instead of traditional explosives; exactly how minimal could a warheads' mass be and what would its velocity need to be to create an "explosive" force of 5,000 lbs of high explosive?

ANSWER:
Funny, I have not heard "all the talk"! I did get one other recent question similar to yours. Anyhow, I guess you want to compare the kinetic energy of a projectile with the same energy as 5000 lb of TNT. 5000 lb is about 2.3 metric tons and the energy content of that amount of TNT is about 10¹⁰ J. Since the kinetic energy of the projectile is mv², there is no clear answer to your question because every mass would have a different velocity to have the requisite amount of energy. The speed of a near-earth satellite is about 8000 m/s and maybe such a satellite would be used to launch your weapon, so let's use v=8000 m/s. Then, 10¹⁰= m(8000)² or m=313 kg=690 lb.

QUESTION:
I'm helping a middle school student with his physics homework. One of the concepts was mass and inertia and he told me that his teacher said they are equal and are synonyms. I was under the impression that they do not mean the same thing, that they are proportional. Could you clarify?

ANSWER:
Here is what the teacher was talking about: There are two kinds of mass, inertial mass and gravitational mass. Inertial mass quantifies how much an object resists changing its motion if a force acts on it. Gravitational mass quantifies how much a gravitational force affects it and how much it gravitationally affects other gravitational masses. It turns out that the two are identical and there is really only one mass. This equivalence is a prediction of the theory of general relativity and is also the reason that all objects have the same gravitational acceleration. Inertia is usually a qualitative term which describes how resistant an object is to being accelerated, which is what inertial mass does quantitatively. However, it is not so unusual to use inertia synonymously with mass or inertial mass.

QUESTION:
I'm curious; and let me know if I am going about this all wrong. A Large wheel about 4ft tall and a small wheel about 1ft tall are both going at 10 meters per second. (Constant acceleration). Will the 1 foot wheel go through more cycles than the 4 foot wheel?

ANSWER:
You must mean constant speed, not constant acceleration, since you specify 10 m/s. I presume this is the speed each wheel is rolling down the road, not the speed of a point on the rim of the wheel. And, why do you mix units (ft/m)? Oh well, the large wheel has a radius R=2 ft=0.61 m and the smaller wheel has R=0.15 m. So the circumferences of the wheels are C=2πR=3.83 m for the big one and 0.94 for the small one. To go 10 m along the road, the big one rotates 10/3.83=2.61 times and the small one rotates 10/0.94=10.6 times. The smaller wheel rotates 4 times faster than the big one. In one second the big wheel rotates 2.61 times, the smaller one 10.6 times.

QUESTION:
My friend and I are having a discussion about centrifugal force and whether or not it exists, is real, and/or if it is present in daily life. Can you expand our knowledge and settle this dispute? We want to know everything about centrifugal force and if you can help us out that would be much appreciated.

ANSWER:
The important concept to understand here is that Newton's laws, which describe motion, are not always true. For example, suppose that you hang a simple pendulum from the roof of a car. When you are standing still or driving down a straight road with constant speed, the pendulum hangs straight down. The forces on the pendulum are its own weight, which points straight down, and the tension in the string which pulls straight up. This pendulum is in equilibrium here in the car and so Newton's first law tells you that the sum of all the forces must add up to zero and so the tension in the string must be equal to the weight of the pendulum bob. Now suppose that you smoothly accelerate; you will find that the pendulum swings toward the rear and hangs at some angle rather than straight down. You will look at that pendulum and say, it is just hanging there at rest in the car and so it must be in "equilibrium". On the other hand, there is no way the (not parallel) forces of the weight and the tension can add up to zero. Newton's first law is a false law in this car! If the first law is true, you are in an inertial frame of reference. If it is false, you are in a noninertial frame of reference. Generally, it is easy to identify a noninertial frame it accelerates relative to an inertial frame. A frame of reference which is rotating is a noninertial frame because an object moving on a curved path has an acceleration even if its speed is constant because the direction of its velocity is always changing. It is shown in any elementary physics text that the magnitude of this acceleration is a=mv²/R, where m is its mass, v is its speed, and R is the radius of the circle it is going around; the direction of the acceleration vector is toward the center of the circle. The acceleration is called the centripetal acceleration from the Latin verb peto which means "I seek". The question is, is there any trick we can pull to force Newton's laws to be true in a noninertial frame. The answer is yes; if you make up a fictious force on any object of mass m which is F_fictitious=-ma in the opposite direction as the acceleration, Newton's laws will work! The centrifugal force is a fictitious force added so that you can apply Newton's laws in rotating systems. (The Latin verb fugo means "I flee".) To see examples of how fictitious forces work, see an earlier question about falling down in a bus which will link you to a question about a car rounding a curve and that will link to a bicycle leaning into a curve.

QUESTION:
Suppose a container is partially filled with a liquid. A small sphere made of a material whose density is less than the liquid is in equilibrium inside the liquid with the help of a thread such that one end of the thread is tied to the sphere and the other end to the bottom of the container. the whole apparatus is kept on a weighing machine. if the thread is cut, then will the reading of the weighing machine change? Since the tension in the thread is an internal force, the reading should not change. However the free body diagram suggests that the reading should change.

ANSWER:
When you refer to " the free body diagram ", you must specify the body. Solving problems like this are most often easiest if you make a clever choice of body. I would choose the body as the container+liquid+ball+thread (taken as weightless, probably); in that case the only downward force is the weight of all three and the only upward force is the scale, so the scale reads the total weight with no reference to whether the thread is connected to the ball or not. You can make this problem difficult by focusing on a different body, maybe the container, but if you draw all your free-body diagrams correctly and apply Newton's third law, you still get the same answer that the the scale reads the total weight. To the left I have shown all the forces on each of the bodies: red is the tension in the string which is also the force the string exerts on the sphere and the on the container; light blue is the force the container and fluid exert on each other; black represents the weight of each; green is the buoyant force which is the force of the fluid on the sphere and the force of the sphere on the fluid; purple is the force the scale exerts on the container.

FOLLOWUP QUESTION:
Suppose the sphere is accelerating upwards inside the fluid, under the influence of the buoyant force. Now its acceleration is dependent upon the buoyant force, while the buoyant force is in turn dependent upon its acceleration. How do we precisely calculate these two quantities, at a particular instant of time?

ANSWER:
First of all, the buoyant force is not dependent on the acceleration. As long as the sphere is fully submerged, the buoyant force (B) is equal the the weight of the displaced fluid. But, you must also include the drag force (D) which the fluid exerts on the sphere and that is not simple to include. But, most fluids have sufficiently large viscosity that the sphere will quickly come to its terminal velocity and move upward with constant speed. When that happens B-D-W=0 where W is the weight of the sphere. If you really want to pursue this farther, the simplest approximation for the drag is that it is proportional to the speed of the sphere, Stokes's law. In that case, a=(B-W-Cv)/m where C is a constant. If you were to neglect drag altogether, which would be a poor approximation for any real fluid, the acceleration would be uniform, a=(B-W)/m.

QUESTION:
I recently saw an experiment on the popular show Mythbusters. The experiment was: a truck moves in one direction at a constant velocity, carrying a canon facing the opposite direction. The canon then fires a football such that it will travel at the same speed at which the truck is going. Successfully performed by the Mythbusters team, the ball dropped straight down to the ground the moment it left the barrel, as theorized, to a remote observer. I wonder, since Earth rotates at about 470metres/s eastward at the equator, if a bullet is fired westward at the same speed but westward, will it drop like the football did? Although I highly doubt this is the case, my calculations so far prove it's theoretically plausible. Am I missing something? Please also elaborate if this is different from the truck-canon experiment.

ANSWER:
In the first example, both the canon and the truck were moving relative to the ground and it was the ground relative to which the final velocity was observed. So, the experiments are not equivalent unless the rifle in the second example were moving east with a speed of 470 m/s. If the rifle was at rest relative to the ground and the observer was not on the ground but looking from space, she would observe the bullet drop straight toward the center of the earth as the earth spun under it.

QUESTION:
Hi, I am a teacher at a high school, my students asked me a question about cracking of sound if we touch a screen of a traditional T.V. (cathode ray tube type).

ANSWER:
The way a CRT works is that a beam of high-energy electrons is fired the screen causing it to emit light. Static charge accumulates on the screen. So the "cracking" is just the same as when you get a mild shock on a dry day when you touch something.

QUESTION:
When an elevator is going down the apparent weight felt by the person is m(g-a) . But if a is greater than g the person will have negative apparent weight. What will the person feel? My physics professor says it is not possible to have a greater than g in practical situations but why is it not possible? What about a hypothetical case?

ANSWER:
Well, it would certainly be possible to have some force pulling down which made the acceleration greater than g. Although your professor is right in that elevators normally do not have such an option, I commend your curiosity regarding "what if?". If a>g, the floor would have to be pulling down on you rather than pushing up your feet would have to be attached to the floor. Alternatively, you would leave the floor and the ceiling would "catch up" with you and exert a downward force on you.

QUESTION:
Since everything is relative in special relativity, it is equally valid to consider the ''Earth'' to be moving toward ''stationary'' particles in the upper atmosphere. In that case, time slows down for Earthbound observers. The particles then decay at their usual half-life pace in their stationary reference frame while only a fraction of these half-time passes for the speeding observers on Earth. Then, just as the speeding astronaut in the Twin Paradox returns to find a much older twin, the speeding Earthbound observers would encounter an extremely old population of cosmic ray particles, which means that they should have long since decayed, and should not have been ''detected''.

ANSWER:
The results are perfectly symmetric regardless of whether you put the earth in a rest frame or the particle in a rest frame. In the earth frame, the lifetime is lengthened by a factor γ=1/√(1-(v/c)²), T'=γT (T is the lifetime for a particle at rest) but in the particle frame, a distance toward the earth is shortened by the factor 1/γ, L'=L/γ (L is the distance for the earth at rest). To check that this makes sense, the earth sees the particle move with speed L/T'=γL'/γT=L'/T and the particle sees the earth move with a speed L'/T=(L/γ)/(T'/γ)=L/T', both the same.

QUESTION:
I was hoping you could answer a question for me concerning gravity. I know that Gravity is associated with a freely falling reference frame. If I was floating in a "cabin" in space, and I ignited a rocket motor the "cabin" (reference frame) would accelerate and I would move inertially until I made contact with the cabin, and then the cabin would accelerate me which I would feel as a g-force (my weight). So the cabin is accelerating in space, which if I used a very distant star I could use this star as a "reference" Now, I'm standing on the earth, the earth is accelerating me upward, this is the g-force (my weight) that I feel. But, what is the earth accelerating relative to? Spacetime itself?

ANSWER:
Your question is a bit muddled, but let me state the equivalence principle for you: there is no experiment you can perform which can distinguish whether you are in a gravitational field with gravitational acceleration a or in a noninertial frame of reference with an acceleration a. I do know what you mean by using a distant star as a reference. I also have no idea what your sentence starting with "Now, I'm standing on earth " means. The earth is not accelerating you upward nor is the earth accelerating. To square your two situations with the equivalence principle, in the cabin it is just like being in a gravitational field but you are not in a gravitational field and on the earth it is just like accelerating but you are not accelerating.

QUESTION:
I was discussing ballistics with someone the other day, and a thought came up about a detail that I am not 100% sure on. With bullets designed to expand, generally speaking materials hardness and velocity determine that expansion. What I am wondering, is does the bullet's energy upon striking the target cause the expansion? Or is the expansion caused by the opposite forces imposed on the bullet by what it is striking? (Or would one say it is a combination thereof?)

ANSWER:
The thing to appreciate is that energy and force are not two separate things. Everything you need to know about collisions is contained in Newton's three laws. The idea of energy often makes problems easier to solve or understand, but the first step in developing the formalism of energy, called the work-energy theorem, is just Newton's second law "in disguise". So, I will discuss the bullet collision both ways:

The bullet hits a wall and stops. What stops it? The force which the wall exerts on the bullet stops it. It begins expanding when it first touches the wall; a point off axis will start moving perpendicular to the direction of the bullet's velocity which has to mean that it is feeling forces from other parts of the bullet since that point is not touching the wall.
The bullet has kinetic energy when it hits the wall. After it stops, that energy is gone. Where did it go? Part of the energy went into doing the work necessary to "squish" the bullet and part was lost to internal friction intrinsic in squishing something soft but not elastic and ends up as thermal energy the squished bullet is hot.

QUESTION:
I am trying to help my son understand velocity, but find myself confused.

Person A drives in a circle. A physics website tells me this represents acceleration, a change in velocity, because his direction is constantly changing even though his speed may not be. Fair enough.
Person B takes a step forward and then a step backward to his original position. A different physics website tells me that this represents zero velocity, no acceleration, because he has not changed position.

But these answers seem contradictory, because person A, driving in a circle, will arrive at his original position at some point. In this respect, he is no different than person B, and could be considered zero velocity. Can't both of these examples be considered changes in velocity? I suppose it depends on the timeframe you use to measure the change in position (?). So the person driving in a continuous circle can be considered to not be accelerating in some cases? This makes no sense.

ANSWER:
The first example refers to the instantaneous velocity and the instantaneous acceleration of person A; instantaneous refers to an instant in time and both acceleration and velocity are continuously changing. The second example refers to average velocity and average acceleration. Average refers to the value of the quantity averaged over some time period and the time period here is the time from when he first stepped forward until he finished stepping back. Suppose that was 10 s and the length of his step was 1 m; then the average velocity is distance traveled divided by the time, 0/10=0 m/s and the average acceleration was the change in velocity divided by the time, 0/10=0 m/s². Person A's average velocity and acceleration over exactly one time around would also be zero.

QUESTION:
I'm having a really hard time understanding something my professor asked me to think about today in my entry level physics class. How can someone ever tell if they are moving if they have no clues of the outside. (sound or sight) If I had a penny and threw it up it would just fall back down, so that would not work. I'm really stumped here!

ANSWER:
This is the heart of a profound physical and philosophical concept. The laws of physics determine the outcome of any experiment you might perform; for example, Newton's three laws of classical mechanics and Maxwell's equations describing electromagnetism are laws of physics. Suppose you have performed experiments to discover these laws in some particular frame of reference and you convince yourself that they are laws which apply and are reproducible for any mechanical or electromagnetic experiment you can think of. Now you decide to redo all your experiments but in a frame of reference which moves with a constant velocity relative to the original frame; you discover a really remarkable thing the laws of physics are identical in this new frame. You can then logically say that there is no experiment you can perform to determine which of these frames is moving and which is at rest. This is called the principle of relativity and frames in which the laws of physics are true are called inertial frames of reference. Any frame which moves with constant velocity relative to one inertial frame is also an inertial frame. Essentially, there is no such thing as absolute rest or absolute velocity. The laws of physics are not true in accelerating (noninertial) frames.

Here is a little more information if you are interested: Newton's laws turn out to be not true for velocities comparable to the speed of light; at high speeds, however, the principle of relativity is still true provided that Newton's laws are corrected (theory of special relativity). Also, it turns out that the principle of relativity is true for all frames, not just inertial frames, provided that you introduce a new principle, the equivalence principle. The equivalence principle states that there is no experiment you can perform which can distinguish whether you are in a gravitational field with gravitational acceleration a or in a noninertial frame of reference with an acceleration a. The generalized principle of relativity and the equivalence principle form the basis of the theory of general relativity which is the modern theory of gravity.

QUESTION:
My question is this, If you are driving in a car (100km/h) and roll down your window a vacuum is created in your car. but what if there is a 100km/h tail wind. does the wind going the same direction cancel out the effect of the vacuum?

ANSWER:
The reason air is drawn out through the open window is Bernoulli's law, which states that when the velocity of a fluid increases the pressure decreases. Therefore, the pressure outside the window (moving) is lower than inside (not moving). This is easy to demonstrate by a smoker inside the car and the smoke being drawn out the window. If there is a tailwind with the same speed as the car, the air outside the car will not be moving with respect to the car and there will therefore be no pressure difference.

QUESTION:
If we see an atom and see all shells and subshells then will I find 3d First or 4s and if we see 4s first then why didn't we name it any other subshell of 3rd shell means why is it 4s? I know that it is chemistry related question but I m confused.

ANSWER:
The letters and numbers mean something. The letters tell you the angular momentum quantum number of electrons in that shell. The letter s tells you that l=0 and 4 tells you that it is the 4th l=0 shell, that there are 1s, 2s, 3s shells with lower energies. The letter p means electrons have l=1, d means l=2, f means l=3, etc. This peculiar labeling of angular momentum quantum numbers is a historical artifact where the words sharp, principal, diffuse, and fine were used to describe spectral lines.

QUESTION:
If two neutrons (just for the sake of ignoring charge) were separated from each other 1 light year, how long would it take for them to "touch" each other based on their gravitational attraction only? They are also in complete isolation from the rest of the universe.

ANSWER:
This is a very strange question. I have answered a nearly identical question before but with much larger masses and smaller distances, but the method is identical so I refer you there. For your masses I calculate about 14x10¹⁸ years, about a billion times the age of the universe. (I would also like to add that I do not believe that this should be taken too seriously because no theory of gravity has been accurately tested for either such large distances or such small masses.)

QUESTION:
I am a fabricator and I currently have a task where I am attempting to create a braking system for downhill rapid propulsion (downhill racing). Although the product exists, it is primitive and not fit for extreme measures, reliabilty, or convenience. There are many variables besides weight, drag coefficient, mass and gravitational acceleration. I would greatly appreciate your professional advice on creating a formula in which I could create, change or gauge different systems for different masses. I have done much research and am increasingly frustrated yet interested. I have come too some conclusions and have run many tests. I am using a polyester blend material for the canopy that is expansive and durable yet retractable, however. My cable system and my rapid cut down on drag are a problem. So my question is if I weigh 160 pounds I am traveling at a speed between 30-60 miles per hour (we will say 45mph) at a down grade of 45% and i would like a slowing to 10 miles per hour between 40-50 feet from deployment. What would my initial area of my canopy be and what would the tensil strength of my cable need to be set at? There are 3 points of contact for the cable system two high and one low.

ANSWER:
First, all air drag calculations are approximate and without extremely complex computer simulations you can only do order-of-magnitude calculations. Since you do not mention any sliding or rolling friction of whatever is going down the incline, I will assume they are negligible. There are, therefore, two forces on the mass, the gravitational force down the incline mgsinθ and the drag of your "canopy" up the incline which I will take as c₂v²; here v is the speed, m the mass, g the acceleration due to gravity, θ the angle relative to the horizontal, and c₂ is a constant determined by the geometry of your canopy. A reasonable approximation for c₂ is c₂≈ A where A is the area presented to the direction of motion of the canopy (only valid in SI units). Since I am a scientist, I will work entirely in SI units here. Newton's second law, which governs the motion of this system, is mdv/dt=-mgsinθ+ Av² or dv/dt=gsinθ[1-(v²/v_t²)] where v_t²=mgsinθ/c₂=4mgsinθ/A; v_t is called the terminal velocity, the speed to which the mass will slow as it goes forward. Solving the differential equation (this is worked out in any intermediate-level classical mechanics book), the following equation is found: v²=v_t²(1-exp(-2gxsinθ/v_t²)+v₀²exp(-2gxsinθ/v_t²) where x is the distance traveled and v₀ was the speed where x=0. That is everything you need since you know everything except A. And you may want to use a fancier value for c₂more tailored to the details of your canopy. As an example, I will use your numbers: m=160 lb=73 kg, v₀=45 mph=20 m/s, v=10 mph=4.5 m/s, x=45 ft=13.7 m, θ=45⁰. Putting these in, I find 20≈(2000/A)(1-exp(-0.094A))+400∙exp(-0.094A) or A(0.05-exp(-0.094∙A))=5(1-exp(-0.094∙A)). Someone more clever than I could probably solve this analytically for A, but I will just solve it numerically by plotting the left and right sides of the equation and finding the intersection (see inset figure on the left). I find that A≈100 m²≈1000 ft²; this would be a square about 30 ft on a side. (Since A is so large, one could have easily solved this by simply neglecting the exponential functions, 0.05A≈5.) Regarding the strength of the cables, since I do not have any details about the design of the canopy, the best I can do is tell you the maximum force the canopy would exert on the mass via the three cables. Since the acceleration is -gsinθ+ Av²/m, the greatest acceleration is when the velocity is greatest, when the braking initiates. So, the force the cables must exert is -mgsinθ+ Av₀²or -mgsinθ+c₂v². For your specific example, this force would be about 9500 N≈2100 lb or roughly 700 lb/cable.

The figure on the right shows the solution I have come up with. Indeed it begins at 45 mph and drops to 10 mph at 45 ft. However, one might just as well say that the speed is also just about 10 mph at 25 ft, just not exactly. That is the problem with analytical solutions sometimes they demand exactness. To me this graph says that you could get away with a significantly smaller canopy and still qualitatively achieve your goal. I think I have done enough here setting stuff up and you could proceed and investigate how much things would change if you changed your speed at 45 ft to be 11 mph, e.g. And, don't forget, these are approximate solutions to be used as a rough guide.

QUESTION:
Say I am a stationary observer and observe a rocket launched from earth to Proxima Centauri which is about 4.35 light years away from us the rocket has a constant acceleration of 1g or 9.8m/s² for half the trip and -1g for the other half. Newtonian kinematics states x_f=x_i+vt+at²/2 yet over the course of time my velocity will become relativistic and the equation no longer applies. What is the correction needed for me, a stationary observer, to solve how long it will take the rocket to reach its destination?

ANSWER:
It turns out that I have already solved this problem; I have found a solution for the distance as a function of time for a constant force F rather than the time as a function of distance, but that can easily be inverted. I showed that x=(mc²/F)(√[1+(Ft/(mc))²]-1); in your case, F=mg, so x=(c²/g)(√[1+(gt/(c))²]-1). Solving for t, t=√[(x/c)²+(2x/g)]. For the first half of the trip, x=2.175 ly and I calculated that g=9.8 m/s²=0.11 ly/yr², so t=6.58 yr. Given the symmetry of the situation, the total time for the trip, as observed by you, is 13.2 yr. Using the result from the earlier answer, v=(gt)/√[1+(gt/c)²], I find that the speed at the midpoint of the trip is 0.89c. The average speed for the whole trip was 4.35 ly/13.2 yr=0.33c.

FOLLOWUP QUESTION:
What is the correction to determine how much time the rocket will have perceived to have passed?

ANSWER:
The mathematics gets a little difficult here but it is a common problem which has been worked out. I will just give you the final result. For more detail, see the blog by John Baez on the relativistic rocket. For a rocket with acceleration g halfway to a distance D in light years and then with deceleration g the rest of the way, the elapsed time T in years on the rocket is T=1.94∙arccosh[(D/1.94)+1)]. So, for D=4.35 ly, T=3.58 yr.

QUESTION:
I'm a climber and I constructed myself an anchor that I fixed to a rock wall. To test it, I hooked to it a 12mm in section steel cable with a length of 2,8m and a concrete block of 30kg to the other tip. I then dropped it from anchor level and it held. I am now wondering what kind of impact force was developed in this test.

ANSWER:
There is no way to know this because what matters is how long it took the block to stop after the cable was straight. One could easily estimate how long it took to fall to the stopping point, about T≈√(2.8x2/9.8)=0.76 s. The speed then would be v≈9.8x0.76=7.4 m/s. The average force would be F≈9.8x30+30x7.4/t=294+222/t where t is the time to to stop. For example, if it took a tenth of a second to stop, the average force felt by the block and therefore by the anchor would be 2494 N≈561 lb. For more detail, see the faq page.

QUESTION:
If you travel a set speed standing in the back of a truck (ex 60mph) and you throw a ball forward at 60mph, does that ball at any point in time double it's speed to 120mph.

ANSWER:
This seems one of the most misunderstood things in physics. Speed has no meaning unless you state what the speed is relative to. In your example, the speed of the baseball relative to you is 60 mph and the speed relative to the road is 120 mph. If there is another truck coming toward your truck with a speed of 60 mph, that truck sees a speed of the baseball of 180 mph. If there is another truck passing you and going 120 mph, he sees the ball with zero speed. The bottom line is that there is no such thing as "absolute speed", it all depends on who measures it. And certainly the ball does not double its own speed.

QUESTION:
Physics says that energy is always conserved in any form. My question is what happens to the energy contained in me if I die? In what form is it transformed?

ANSWER:
Actually, "physics says" that the energy of an isolated system (no external forces doing work on it) is conserved. Or, you could say that the energy of the entire universe is a constant. So, let's talk about what energy there is in your body. Mostly, it is simply the mass of all the atoms in your body, E=mc²and this does not change because as your body decays, all the atoms are indestructible. However, much of the molecular structure of your body changes. I do not know that much detail about microbes and organisms which hasten decay, but essentially they will extract energy from fats and sugars and use it for their own purposes. I assume that heat will also be a result of decay.

QUESTION:
i was wondering if you could tell me why we measure the angles from the normal and not the reflective surface and also could you explain the difference between regular and diffuse reflection.

ANSWER:
No really important reason that I can think of, it is just convention. There are situations in physics where a surface is conveniently denoted as a vector and that direction is normal to the surface, not parallel. Diffuse reflection refers to reflection from a surface which is rough rather than smooth. A nice little app letting you see this kind of reflection may be seen here.

QUESTION:
I was recently at the Map and Government Library of the main library and stumbled upon a stereoscope map reader. When held over two accurately aligned aerial photographs of the hills and valleys of Gordon, Georgia, I can see one image of the trees and hills "popping up" as if they are 3-D. Both lenses are concave, and I was wondering how does the picture appear 3-D?

ANSWER:
First, we need a tutorial on how we see the world in 3-D. Because we have two eyes (binocular vision), each eye sees a slightly different image of the world because of parallax. One of the first things the brain of a newborn baby learns is how to interpret these two images as a 3-D picture. If you had two cameras separated by the distance between your eyes, the pictures would be slightly different. A simple photograph, of course, contains only information from one perspective and therefore lacks the 3-D quality. In the 19^th century cameras were designed with two lenses to form two images. If you then viewed one image with one of your eyes and the other image with your other eye, you could see the photograph as you would if you were looking at the original object, i.e. in 3-D. The viewer is called a stereoscope. The purpose of the lenses is to allow you to view the very close photos with a relaxed eyes.

QUESTION:
We can use the work-energy theorem in any inertial frame of reference. When no external force is applied, and there is no change of height, the change in KE = -(Change in PE(spr)). However, KE change depends upon the frame of reference and the extension of a spring does not depend upon frame's choice! HOW IS THIS POSSIBLE?

ANSWER:
The extension of the spring is the same in both frames, but the work done by the spring is not because the same force acts over a different distance in the moving frame. Forget potential energy and simply write ΔK=W where W is the work done by any conservative external force, W=₀∫^XF(x)dx. I have chosen the starting position as x=0; to keep the algebra simple, I will also choose the starting velocity in this (x) frame to be 0 and the final velocity to be V, so ΔK= MV²=W. Suppose that it takes time t to reach the position X. Now, suppose there is another reference frame (x') which has a speed U in the +x direction and x'=0 at t=0 also. Then x'=x-Ut and the initial velocity is U and the final velocity is U-V in this frame, so ΔK'= M(V-U)²- MU²= MV²-MUV. Finally, calculate the work done in the moving frame: W'=₀∫^X^'F(x')dx'=₀∫^X-UtF(x-Ut)(dx-Udt)=W=₀∫^XF(x)dx-U₀∫^tF(t)dt. The second integral is the impulse which is the change in momentum, MV, so W'=₀∫^XF(x)dx-MUV=W-MUV. Putting it all together, ΔK'=W'= MV²-MUV=W-MUV or MV²=W.

QUESTION:
In the figure to the right, green ring is rotated about horizontal axis, yellow ring is made to rotate about vertical axis and innermost blue ring is made to rotate about horizontal axis and I want to make this system self sustained with the help of induced current and magnetic induction. I am facing difficulty in finding angular momentum. What will be the angular momentum if a ring is made to rotate about the horizontal axis passing through its centre?

ANSWER:
The moment of inertia I of a thin ring of radius R and mass M about a diameter is I= MR². Angular momentum is Iω where ω is angular velocity in radians/second. Good luck making this "self sustained"!

QUESTION:
I have a remarkable image relating to the speed of light. It begins "and God said," and then contains some 30 or 40 detailed equations, then finishes with "and there was light." This is NOT the well known set of 4 Maxwell Equations which has been made into a tee shirt (I wear one proudly!), but a much more sophisticated treatment of the same subject. I am looking for a detailed interpretation of the 30 or so equations, several of which I recognize, but many of which I do not. Can you help or suggest where we might such a treatment? I believe you can find the image I am referring to fairly easily by Googling for light equations or something similar.

ANSWER:
The original and God said T-shirt is infinitely more elegant than this one. This one is trying to be so cool that it comes off as pretentious! Here are some of the things in this mess of stuff which are extraneous to light: expressions for Bessel functions, one-dimensional Schr dinger equation, three-dimensional Schr dinger equation, Coulomb's law (already in Maxwell's equations), LaPlace's equation in spherical-polar coordinates, dependence of mass on velocity (special relativity and irrelevant for massless photons), other stuff from special relativity (already contained in Maxwell's equations), one-dimensional harmonic oscillator energy, deBroglie's hypothesis, etc. The only thing Maxwell's equations do not include that God certainly needed to add was the concept of a photon, E=hf=pc. God would have made his prouncements as concise as possible; see Occam's Razor.

QUESTION:
I am lying on the surface of the earth. I don't have Kinetic energy because I am not in motion. I also cannot have any potential energy because my height raised from the surface of the earth is zero. Does it means I don't have any energy in me?

ANSWER:
But, you have potential energy relative to the bottom of a nearby hole, don't you? And if someone on the moon observed you he would say you were moving, so you would have kinetic energy as seen by him, wouldn't you? Energy is not absolute but "in the eye of the beholder". Also, we know that there is lots of chemical potential energy stored in the fat of your body. Also, we know that there is energy simply because mass is a form of energy, E=mc².

QUESTION:
Suppose i am inside a uniformly moving spaceship and I send a light beam perpendicular to the direction towards the opposite wall. since the spaceship is moving and according to relativity light doesn't feel any sort of "kick", shouldn't the light beam be off the target and thereby letting me know that I am moving?

ANSWER:
If you aim at a spot on the wall you will hit it. You will observe the light beam going straight across the ship. The reason is the principle of relativity which states that there is no experiment that you can perform which can distinguish whether you are at rest or moving with constant velocity. You could also fire a rifle at the opposite wall and hit the target. An observer outside your spaceship, however, will see the light with a component of its speed in the direction you are moving but will still see the speed of the light as being c and the light hit the target. Your experiment would be different, however, if you were accelerating forward; the light would miss the target for the same reason that if you were to aim a rifle directly at a target on earth it would fall some on the way there. You might be interested in the light clock. Your space ship could be thought of as a light clock which ticks once when the light hits the target.

QUESTION:
We did a lab where we put lithium in a flame and saw it emitted red light. We were told this happens because an electron gets so much energy it jumps from one electron shell to the next. Then when it falls back to the lower energy level, it gives off photons of light. We are having trouble understanding what is happening to the electrons. Lithium has two electrons in the first energy level and one in the second. Does an electron in the first shell jump to the second? Or does an electron in the second jump out to the third? And if it is an element with 3 orbitals, does an electron jump from the first to the second, and if it does, does an electron also move from the second to the third? How does the movement of electrons from one shell to the next affect the other electrons in that shell?

ANSWER:
The figure to the left shows the energy-level diagram of lithium. The thing to understand is that the two inner electrons (in the 1s shell) are essentially inert for your experiment, only the outer electron gets excited. So the outer electron (in the 2s shell) looks in and sees the nucleus, charge +3, shielded by two electrons, charge -2, for a net charge of +1. In other words, the lithium spectrum should look a lot like the hydrogen spectrum because the active part of the lithium atom looks pretty much like a hydrogen atom. Note, for comparison, the energy levels for hydrogen shown on the figure. The red line, with a wavelength of about 670 nm, results from the transition from the first excited state (2p) to the ground state (2s).

QUESTION:
When Cavendish calculated the value of universal gravitational constant he used mass of lead balls as reference. But how did he know mass of the lead ball if he doest know the value of G?

ANSWER:
He could simply weigh them because we know W=mg. Since you can also write W=mM_earthG/R_earth², you can identify g=M_earthG/R_earth², and g is easy to measure even if G is not.

FOLLWOUP QUESTION:
How did he build a weighing scale. When weight is determined using a scale it should have been built w.r.t certain standard mass. But with out knowing value of G there is no way they had a standard mass. He could have weighed a 10kg mass as 1kg mass. So, how did he weight exact mass.

ANSWER:
The kilogram was officially defined in 1795 as the mass of 1 liter of water. The Cavendish experiment was performed in 1797-98. Even if the kilogram were not defined, there were other mass definitions which Cavendish could have used. As an example, let me consider the oldest standard weight I could find reference to, the beqa (b) (shown in the figure) defined about 5000 years ago as the mass of 200 grains of barley corn which is about 6.1 grams=6.1x10^-3 kg. So if Cavendish had used the b as his mass standard, he would have found G=6.67x10^-11 kg²/(N∙m²)=6.67x10^-11 [kg∙s²/m³]x[1 b/6.1x10^-3 kg)=4.07x10^-13 b∙s²/m³] (assuming that he used seconds and meters for time and length). It is a different number but means exactly the same thing.

QUESTION:
I have a strange request. I'm a nurse practitioner, I learn, like most people by seeing a picture or being shown the way something works. I don't know if you've heard of Frank-Starling curve related to the heart, it's the idea of volume or pressure on horizontal line and stroke volume or cardiac output (CO) on vertical. The idea is more vol will help, too much will over distend and be less stroke volume or CO. I have the idea of the spring with the weights on it to show this. But I really need something to exhibit this example.

ANSWER:
I hope I am not totally out of my depth here! I will attempt to bring simple physics principles to bear on this question. I had never heard of the Frank-Starling curve and did a little research to educate myself. The curve basically shows, under various conditions, the relationship between how much blood is loaded into the ventricle and how much of that blood is then pumped out. Usually the absissa (x-axis, independent variable) is something called preload, but the basics can be qualitatively understood by plotting volume of blood filling the ventricle. In the figure to the left, focus on the curve labeled B, Normal at rest. I interpret this as the result of the heart acting as an elastic membrane and stretching as blood is added. As the membrane stretches it behaves like a two-dimensional spring and the basic property of a spring is approximated by Hooke's law which essentially says that the force exerted by the spring is proportional to how much it is stretched. Hence, adding more blood stretches the spring more and the more it stretches the harder it pushes on the blood; therefore in a given time more blood will be pumped out because of this greater force (pressure). This explains the rise of these curves. Therefore, your idea of springs and weights is a good one because elasticity is the key here, I think. Maybe even better would be to take a couple of identical balloons and fill one with twice the air as the other; then letting the air flow out for the same times, the fuller one should blow out more air. Also, in times of stress or exercise, I have learned that neurotransmitters are transmitted to the muscle cells in the heart wall which results, via calcium ions, in stiffening the walls more so that they exert even greater force (pressure) on the blood resulting in a curve more like A, Normal during exercise. To demonstrate that, use two different springs with different stiffness or use two different balloons, one much harder to blow up than the other. Drugs can also be used to try to adjust the shape of the curve, e.g. digoxin or calcium channel blockers. Note that in curves C and D a point of diminishing returns is reached actually causing the output to begin dropping with increased input. I guess this could be likened to reaching an elastic limit like when you stretch a spring too far and it will not go back to its unstretched length.

QUESTION:
I've been wondering about the hazards of traveling at high speeds in space for a while and the challenges it would bring for us in terms of spaceship design. My main interest is interstellar travel and how meteors might affect the safety of such an exercise. So if we had a spaceship that would accelerate to let's say 7 percent c and we sent it along with some colonists to Alpha Centauri and the ship while traveling 7 percent c hit a meteor the size of a man's fist, how catastrophic would an impact of this nature be to the spaceship?Let's say the meteor was 10cm across and was comprised of iron.

ANSWER:
There is no way I can even begin to do a calculation here. It would depend on the design of the space ship. Even if I had more details, this would be more of an engineering problem than physics. You can use your imagination, though. The shuttle Columbia was going 545 mph=11 m/s≈0.000004% of c and a piece of foam insulation (much softer than iron) had catastrophic results. You might be interested in another recent answer.

ADDED ANSWER:
I estimate your meteor would have a kinetic energy of about 2x10¹⁷ J, about the same as 2,000 Nagasaki atomic bombs. Curtains for this space ship!

QUESTION:
On a Disk or a Sphere. What is the Centrifugal force on matter when the Disk/Sphere is spinning at half the speed of light?

ANSWER:
I presume you mean the circumference has v=c/2. This is quite well approximated nonrelativistically (γ=0.97), so F≈mv²/R=mc²/(4R)

QUESTION:
I understand you don't answer questions about stars, but im wondering about the nuclear dynamics involved in nuclear fission. In a star with 250 solar masses or more there is something called photofission, where high energy gamma rays cause elements as light as tin to go through nuclear fission. I was wondering, how much energy would be required to cause fission to occur in the element tin?

ANSWER:
Right, this is certainly more nuclear physics than astrophysics, so I can do some rough estimates for you. I can tell you approximately how much energy is released in the symmetric fission Sn¹¹² >2Mn⁵⁶. The binding energies of the tin and manganese are 953.5 MeV and 489.3 MeV respectively. So the energy released in the fission would be 2x489.3-953.5=25.1 MeV. What I cannot tell you is what energy photon would be required to cause the fission. This depends on the structure of the Sn¹¹² and how it interacts with the photon. Fission might be induced by a much lower energy photon than 25 MeV. After all, uranium can fission with no energy whatever added to it (spontaneous fission).

QUESTION:
What is the Cosmological Constant and why it couldn't be described perfectly by Einstein? Was only because of it that Einstein thought the universe was static?

ANSWER:
When Einstein proposed the theory of general relativity, around 1918, it was generally believed that the universe was static. General relativity is the theory of gravity, and if gravity is the main interaction among stars and galaxies, this is not possible; with only gravity, the universe would have to be either expanding and slowing down or compressing and speeding up. Therefore Einstein had to introduce something to balance the universal gravitational force; this something was called the cosmological constant. It was later discovered that the universe is expanding and he later denounced the cosmological constant as " my greatest blunder " Interestingly, several years ago it was discovered that the expansion of the universe is actually speeding up implying some kind of repulsive force, akin to the cosmological constant, often referred to as dark energy; this has reignited interest in Einstein' "greatest (or maybe not) blunder". I do not know what you mean by " couldn't be described perfectly "

QUESTION:
What is the difference between atomic physics , nuclear physics , particle physics and high energy physics ?

ANSWER:
Atomic physics studies the atom, mainly the configurations and properties of the electrons which characterize atomic structure; usually atomic physics also includes molecular physics and chemistry may be thought of as applied atomic and molecular physics. Nuclear physics studies the properties of the atomic nucleus. Particle physics studies elementary particles, constituents of atoms and nuclei. High-energy physics usually refers to physics done using high energy particle accelerators, in the GeV range or above, to study mainly nuclear physics or particle physics; can also refer to cosmic ray physics. There is no clear boundary among these areas of physics and there is often considerable overlap.

QUESTION:
If 2 people are standing in opposite directions but equal distance away from a firework why will one person hear it before the other? I have a very inquisitive child who heard something about this on a TV programme but can't quite remember exactly why and I don't know so please help me!!

ANSWER:
The only thing I can think of is wind. Sound moves with a speed of about 840 mph relative to the air. For example, if there is a wind with speed 30 mph blowing north relative to the ground, then the speed of sound relative to the ground is 870 mph north and 810 mph south. In that case, someone north of the firework would hear it sooner than someone south.

QUESTION:
I was wondering if you could help me explain something that happen whilst heating water using a bunsen burner the other day. I stopped heating (turned the bunsen burner off) at 80 degrees, but the temperature of the water (as read from a thermometer) continued to increase. This only lasted for several minutes, but this is not what I had expected. Given that the heat source was removed, I expected particles to begin to decrease in kinetic energy, and therefore temperature decrease too. Am I correct in thinking particles within water built up momentum during heating, meaning they were still increasing kinetic energy and therefore temperature for a short while after turning the bunsen burner off? This meaning the thermometer increased in temperature too even though bunsen burner was off?

ANSWER:
Your speculation that the kinetic energies of the molecules continued to increase because they were already increasing is wrong energy change does not have "inertia". The instant you stop adding energy (heating) the water, its temperature will stop increasing. Therefore, something must have continued heating the water. If you think about it, the bunsen burner, while the ultimate source of the energy, is not what is heating the water; the bunsen burner is heating the container and the container is heating the water. The container is much hotter than the water when the bunsen burner is turned off and it will continue heating the water until the two are in thermal equilibrium, at the same temperature (ignoring the interaction with the air around them).

QUESTION:
I want to know that as the height increses acceleration due to gravity decreases at diffent height so escape velocity should be different at every value h so will the acceleration due to gravity at height h will be rate of change of escape velocity with respect to time. If no then why beacause acc is the rate of change of velocity with respect to time?

ANSWER:
The escape velocity is defined as the velocity that an object at rest at a particular point in a gravitational field must be given such that it will be at rest when it has gone infinitely far from the source of the field. So, you are right that the escape velocity from earth depends on the altitude at which the object begins. So, it doesn't mean anything to ask what the escape velocity is as a function of time, only what it is as a function of altitude.

QUESTION:
I want to know how to caculate the distance a let say bike would fly if it was projected off a ramp so that I would be able to predict where it will land I have seen the equation but I don't understand it: R=v_o²(sin2θ)/g.

ANSWER:
The equation you found is valid only if the bike comes down at the exact same level that it left the ramp. I refer you to an earlier answer for a more general equation and the explanation. The earlier answer is in English units. If you want to work in SI units, distances should be in meters (m) and speeds should be in m/s, and g=9.8 m/s².

QUESTION:
If a 55,000 pound vehicle slams into a stationary 2,200 pound vehicle at 25 mph, what amount of force is created at impact to occupant of smaller vehicle?

ANSWER:
There is no way to calculate this with this information. Let me do a few calculations to show you what the problems are. If the two vehicles stick together, you can use momentum conservation to get the speed v of the two after the collision: 55,000x25=57,200v, so v=24 mph. I would prefer to do the calculations in SI units, so v=24 mph=10.7 m/s, m=2200 lb=998 kg. The momentum of the car after the collision is p=mv=2.2x10⁶ kg∙m/s. Now, we come to the crux of this calculation (this is from Newton's second law, if you care): the average force which caused the momentum to change is F=p/t=2.2x10⁶/t, where t is the time which the collision lasted and I do not know t. For example, suppose the collision lasted s; then the force was F=4.4x10⁶ N=9.89x10⁵ lb. That is the force the car feels. The occupant will feel a much smaller force. Suppose, for example, that the mass of the occupant is 200 lb=90.7 kg; then the momentum acquired during the collision is 90.7x10.7=970 kg∙m/s. So, if it took s for the occupant to acquire this momentum, the force would be F=1940 N=436 lb. All these calculations would be different if the two vehicles did not move off together after the collision; the car and occupant would be moving faster than 24 mph so the forces would be greater. And, without knowing how long the collision lasted, you cannot know the average force applied.

QUESTION:
I was watching a documentary about quantum mechanics, and after about 4 min of the particle-wave duality I stopped to Google some questions, the 1st I found right away, ie "what would happen if you fired a SINGLE electron. And at least from what i read, the result is still the refraction pattern. So then i got to thinking and this is where my question comes into play. From what i gather observing an object at the quantum scale affects the result, considering our eyes use photons that makes sense but my question is this... if we assume that at the very basic level, both photons and electrons are waves the entire time, could what we see as an observation of the electron be nothing more then an interference pattern created when the wave from the photons leaving our eyes interfere with the waves from the electron, and the point where that interference peaks, is what we "observe" as a particle? And by extension, could all known "particles" simply be varying degrees of interference caused by this effect? Furthermore, if we continue to assume that all particles are in fact waves from the beginning then could the interference of 2 non photons create interference that we observe as other "particles" when our photons interact with the interference pattern caused by those waves?

ANSWER:
It is hard to understand what your question is. But, you state that a single electron will give you a diffraction pattern. If this is what you literally mean, then that is wrong. A single electron will give you a single spot on the screen. The wave-particle duality example here is that if you shoot many electrons but one at a time, you will see the diffraction pattern emerge (see the animation here). With regard to your speculation that "...both photons and electrons are waves the entire time...", this is exactly what wave-particle duality is not. Both are both all the time and you will observe the one which you look for in your experiment. There is also a wonderful video animation (it's in the Brief History section, Animation showing the wave-particle dualitywhich illustrates the double slit for particles, waves, particle-waves, and particle-waves where an observer determines the slit through which slit the particle went through.

QUESTION:
Yesterday, In my exam, I was asked a short question. It was: Does energy of light transfer while changing the medium as velocity differs? Give reason. What might be the correct answer?

ANSWER:
You either remember the question inaccurately or a translation has not been a very good one! I am guessing that the question should be something like: When light passes into a medium where the velocity is different, does the energy of the light change? The answer is no if absorption is negligibly small. If it did change, where would the energy come from or go to? The reason is best understood by thinking of light as photons and the energy of each photon is hf where h is planck's constant and f is the frequency of the light. The frequency of the light in the medium is the same as outside. The wavelength, however, does change because v=fλ where λ is the wavelength and v is the velocity.

QUESTION:
A ball thrown upward has zero velocity at its highest point i.e no acceleration. The resultant of applied force and weight/gravity is also zero. Thus the body is at rest. Is it in equilibrium too? This is not a homework question. I and my friend are too confused.

ANSWER:
Right off the bat, your first sentence is incorrect. Acceleration of something at rest is not necessarily zero. You cannot determine whether an object is accelerating by knowing only its velocity because acceleration is the way the velocity is changing which cannot be known by simply knowing what the velocity is right now. In your example, the ball is at rest right now but was moving upwards just before now and will be moving down just after now. The ball has an acceleration which happens to be 9.8 m/s²; this means that one second before now it was moving upwards with velocity 9.8 m/s and one second after now it will be moving downward with velocity 9.8 m/s. It is not in equilibrium because Newton's second law tells you that any object with a net force on it is not in equilibrium; the only force (ignoring air drag) on the ball is its own weight and this force is the source of its acceleration.

QUESTION:
A space craft travelling at very near light speed sets a course for a distant object. So travelling at near light speed, could a navigation computer make corrections quick enough, and alter the trajectory for objects in its path. Could these calculations be made quick enough to miss the object? Assuming computer calculations happen at the speed of light the craft could be on a collision course with an object before (a:) it saw it and (b:) before it could recalculate an evasive action missing the object. With the added disadvantage that human thought isn't any where near quick enough to react to a blocking object. So how could 'we' travel to distant objects without fear of ploughing into the first path crossing object?

ANSWER:
You raise an interesting and important question here. But it is much worse than you think. For one thing, because of length contraction, an object which an outside observer would see as being, for example, 1 light year away from you would only be 1x√(1-.99²)=0.14 light years away as you observed it if going 99% the speed of light; that gives you a lot less time to maneuver. But there is a much more important barrier to being able to navigate at very high speeds. The upper picture to the left shows the space craft as seen by an outside observer; light from a distant star comes with velocity c making an angle of θ relative to your velocity v. But, if you now are on the space craft, you observe the star at a different location specified by θ' because of the velocity transformation to the new light ray c'. Note that the magnitudes are the same, c'=c, but the directions are different, θ'≠θ. It can be shown that θ'=tan^-1[sinθ√(1-(v/c)²)/(cosθ+(v/c))]. I have plotted this for several values of v to the right. The effect is quite dramatic. For example, at 99.9% the speed of light everything in your forward hemisphere and much from behind you will appear inside a 5⁰ cone in front of you! Just having optics which could give good enough resolution to see anything but an extremely bright light in front of you would be a challenge in itself. But wait, it even gets worse! There will be significant Doppler shifts and much of the light at very high speeds will be shifted out of the visible spectrum. A nice little animated gif below (which I found on Wikepedia) demonstrates this but only up to 89% of c.

QUESTION:
Since gamma rays are basically a form of light with much more energy than ultraviolet rays can they cause fluorescence in the same way as ultraviolet rays (black light)?

ANSWER:
The way fluorescence works is that the photon excites an electron to a highly excited state which then deexcites by cascading down. A gamma ray has so much energy that it the likeliest thing to happen would be to completely knock out an electron, ionize tha atom which would then not result in deexcitation. What then happens is that these energetic electrons interact with atoms in their path, exciting them and then they deexcite by emitting light. This is not the same as fluorescence and is called scintillation. This is one of the main ways gamma rays are detected, by scintillation detectors.

QUESTION:
Does mass affect kinetic energy?

ANSWER:
Of course. Classically the kinetic energy K= mv²where m is mass and v is speed. Relativistically, K=E-m₀c² where c is the speed of light, m₀ is the mass of the object when not moving, the total energy E=√(p²c²+m₀²c⁴), and the linear momentum p=m₀v/√(1-v²/c²). So, you see, mass appears all over the place. The momentum of a massless particle, the photon, is p=E/c; its energy is all kinetic, and K=E=pc since a photon has momentum even though it has no mass.

QUESTION:
Suppose two friends break their arm at the same time and a doctor says it will take both six weeks to heal. On the same day one friend is due to board a rocket that travels 99.999% speed of light. They have an uncanny ability to count in sync, in their heads. They agree to close their eyes and count up to six weeks, second by second. The traveler only has to hit a reverse button once he counts exactly 3 weeks, and he'll return to the exact spot he left his friend. After they have both counted six weeks in their heads, they open their eyes... Is the traveler back with his friend?? The friend who did not travel finds his arm has healed. Has the travelers arm also healed?? The obvious answer is yes to both. The traveler has traveled at the same speed one way as far as the other (counting 3 weeks each way). The traveler has counted the same amount as the non-traveler (3,628,800 seconds) so therefor his arm should be healed. Special relativity would suggest his arm has not healed.. it would suggest a lot less than 6 weeks has passed for him! It suggests that not only time slows down, but the travelers mind & body slow down too...does he count more slowly in his head? Does his breathing slow down also? Wouldn't he die from breathing so slowly??!!

ANSWER:
You have this a bit muddled. Let us first find out how far d' the traveling friend travels in 3 weeks (as measured by him). d'=vt'=0.99999c∙3=2.99997c or d'=2.99997 lw each way (1 lw is a light week, the distance light travels in 1 week). The earth-bound friend, however, sees a much longer distance that his friend has traveled because the friend sees the distance length-contracted by a factor √(1-.99999²); so the distance which the earth-bound friend sees is d=2.99997/√(1-.99999²)=670.8 lw each way; the time someone traveling 0.99999c takes to go this distance out and back is 2x670.8/0.99999≈1342 weeks. The earth-bound friend has been healed for about 1336 weeks, more than 25 years, when his friend returns with a just-healed arm. You still cannot really accept that moving clocks really do run slowly, can you?!

QUESTION:
I am currently doing a research paper on the perfect free-kick, could you find an equation that suits the following variables? The soccer ball is kicked from the origin of a coordinate system with an unknown velocity such that it passes through the points (x,y)=(9.15 m, 2.25 m) and (x,y)=(22.3 m, 2.22 m). How can I find the magnitude and direction of the initial velocity? Just having an equation to help me work with would be very nice.

ANSWER:
The equations of motion for a projectile which has an initial velocity with magnitude v₀ and angle relative to the horizontal θ are x=v_0xt and y=v_0yt- gt² where v_0x=v₀cosθ, v_0y=v₀sinθ, t is the time, and g=9.8 m/s². Solving the x-equation for t, t=x/v_0x; putting t into the y-equation, y=(v_0y/v_0x)x- g(x/v_0x)². Since you have two (x,y) data points, you have two equations with two unknowns, (v_0x,v_0y). The algebra is tedious, but the result is that v_0x=21.0 m/s and v_0y=7.30 m/s; v₀=22.2 m/s, θ=19.2⁰. To check my answer I drew the graph shown to the right (note the different x and y scales); it looks like my solution passes pretty close to the data points.

QUESTION:
Do physicists believe that some quantum events are truly random in the sense that they are unpredictable even if we had a computer that had complete knowledge of the universe? Or is it that they are considered random because there's no way of measuring the events due to a lack of measuring tools?

ANSWER:
Random is not the same as unpredictable. You cannot predict any outcome of anything precisely; you can predict the probability that your measurement will get a particular value that is essentially what quantum mechanics does. Essentially, the reason you cannot predict any outcome precisely is that "complete knowledge of the universe" is impossible by a computer or anything/anybody else. Even complete knowledge on one particle is impossible.

QUESTION:
We have a theoretical minimum temperature, Kelvin's absolute zero. Do we have a theoretical maximum temperature, If so, what is it.

ANSWER:
See an earlier answer.

QUESTION:
Why do I WEIGH the same at the earths pole as I do at the equator ? Given the earths radius 6378km and i'm doing 1600km/h and my MASS is 100kg thats about 3.1Nm/s of force trying to throw me off the ground. YET At the pole i still have 100kg MASS and the earths gravity hasn't changed ...whats the deal ?

ANSWER:
As anyone who follows my answers can tell you, I am very rigid about what weight means. To me, my weight (on earth) is the force which the earth exerts on me. A minority of text-book writers like to define weight to be what a scale reads. There is one main reason why your weight is different at the poles and equator: the earth is not a sphere but is slightly oblate which means that you are closer to the center of the earth if you are at the poles and so you are slightly heavier there. The figure to the left shows equipotential surfaces for an oblate spheroid (much more oblate than earth) and the ratio of the weights at the pole and the equator is 1.52/1.14=1.33. There is an additional reason why a scale reads differently: because of the earth's rotation, there is a centrifugal force at the equator which results in a scale reading less than at the poles. If you take into account that the earth is not really a uniform mass distribution, this can also affect the local value of g. All of these are very small effects on earth. I guess the bottom line to my answer is that you do not necessarily weigh the same anywhere on the surface of the earth as anywhere else. Your mass is always the same, though.

QUESTION:
As when sudden brakes are applied during riding a fast moving bike the back wheel leaves the ground. Why? Also what must be the conditions for the wheel to not leave ground and when bike semicirculy revolves?

ANSWER:
To the right is the "free-body diagram" showing the pertinent forces if the back wheel has not left the ground. The weight mg acts at the center of gravity of the bike+rider and each wheel has a normal force (N) and frictional force (f) from the ground. The bike has an acceleration a in the direction of the frictional forces, so f₁+f₂=ma. The system is in equilibrium in the vertical direction, so N₁+N₂-mg=0. The bike is also in rotational equilibrium so all the torques about any axis must be zero; summing torques about the front axle, Rf₁+Rf₂+DN₂-dmg=0 where R is the radius of the wheel, D is the distance between axles, and d is the horizontal distance between the front axle and the center of gravity. Now, suppose that the rear wheel is just about to leave the ground; then N₂=f₂=0. The three equations then become N₁-mg=0, f₁=ma, and Rf₁-dmg=0. Putting the second equation into the third and solving for the acceleration, a=g(d/R); if you are slowing down any faster than this, your rear wheel will lift off the ground and the bike will no longer be in rotational equilibrium. If a is really big, you will keep rotating until your center of gravity is forward of the front axle; then you will not be able to stop the bike from rotating all the way over and crashing you on the ground. This actually happened to me once when I was mountain biking with my son and I broke a couple of ribs! I do not understand your second question.

QUESTION:
If I shine a light it emits a light particle outwards. Assuming it is travelling in vaccum all the way out and hits no light absorbing medium. The farther it travels it starts losing energy and shifts to the red. Now how long in Earth years will it take for that photon particle to lose "ALL" its energy. How far will it travel in distance (light years) and what happens to that particle at the end of it all.. i.e it just cannot "disappear" or become void or null. Or perhaps it will all be converted to some other form of energy but then what would it lose its energy to given it was hypothetically travelling in a vaccum/void all the way out?

ANSWER:
Let's first consider the particle to be a ball. If you throw it up it will begin immediately losing energy and eventually stop. You can now think of the earth as having acquired the energy the ball had, now in the form of potential energy. Being a ball, it then falls back and extracts all its energy back. However, if you throw the ball hard enough it will keep going forever and the farther away it gets, the less energy it loses, essentially moving with constant speed when it has gotten pretty far away. The same is true of a photon, as you note, except for two important differences: it cannot stop and it has no mass, just pure energy. The red shift you refer to is the gravitational red shift and as the photon becomes redder it is, as you stated, losing energy (also to the mass it is leaving) but not speed. For the earth (and almost every object in the universe) the effect of gravity is insufficient to ever cause the photon to lose all its energy. Only black holes can rob a photon of all its energy; if a photon is inside the event horizon of a black hole, as it tries to escape it gets "redder and redder" until it has finally lost it all and it disappears. The energy it had will now be found in a slightly increased mass of the black hole.

QUESTION:
My husband is employed as a school bus driver. He repeatedly tells the middle and HS students not to stand in the aisle until the bus comes to a complete stop. He tries to explain to them (without knowing the exact physics behind it) that if they're standing while the bus is moving at say 40 mph, and he has to stop short for some reason, they will not be able to stop their bodies from propelling forward. They never seem to "get it". How can he explain this from a physics point of view that they might better "get"?

ANSWER:
Are you kidding? You actually think a bunch of rowdy kids is going to listen to a physics lecture on the bus? If you want a detailed description of the physics of this exact situation, I have given it in an earlier answer. Here is a suggestion which will maybe work: take a tall box with little width, maybe 5 feet tall and 1x1 feet at the base. Have everyone in the bus sit down and have one student stand the box in the center of the aisle when the bus is moving at about 40 mph. Have your husband then stop abruptly (not so quickly that the sitting students might hit their heads) and watch what happens to the box. Better yet, get a mannequin to stand in the aisle. You could also put a little cart with wheels in the aisle which would go zipping to the front of the bus when it stopped. The physical principle is inertia: an object in motion tends to stay in motion unless acted on by some force (like if you were holding on to something).

QUESTION:
Why is a flame hot? Where does its temperature come from?

ANSWER:
You first have to ask what temperature is for a gas: it is the average kinetic energy per molecule. Put into everyday language, the higher the temperature, the faster the average molecule in the gas is moving. The presence of a flame indicates that some kind of chemistry is going on. The simplest thing going on in a typical fire is that carbon (C) is combining with oxygen (O₂) to produce carbon dioxide (CO₂). When any chemical reaction occurs, energy is either released or you must add energy to make it happen. When carbon dioxide forms, energy is released and this energy shows up as the kinetic energy (speeding up) of the molecules in the hot flame.

QUESTION:
Can an object become dead still in space and how would you know?

ANSWER:
There is no such thing as "dead still". Newton's first law or the principle of relativity essentially proclaim that the laws of physics are the same in all inertial frames of reference. There is no experiment you can do which can distinguish which of two frames moving at constant velocity relative to each other is at rest.

QUESTION:
A person is standing on a platform. Below him is another person, not wearing a hard hat. The person on the platform drops a standard sized marble. Approximately how high would the platform have to be in order for a marble to cause trauma/injury?

ANSWER:
This is a little tricky because there is no such thing as a "standard sized marble". I figured an average marble would have a diameter of about 1 cm (R=0.5 cm=0.5x10^-2 m) and a mass of about M=1 g=10^-3 kg. The second tricky part is that I do not know how fast a marble needs to be going in order to penetrate the skull which is how I would judge whether serious injury resulted. I do know that a bullet which has roughly 10 times the mass of a marble will penetrate the skull at a speed of about 60 m/s (about 130 mph). So, I would conclude that a marble would have to be going quite a bit faster to penetrate the skull since it would bring in a lot less momentum than a bullet with the same speed. So the first question I will ask is what will be the speed if I drop it from a height of h=5 miles (about 8000 m). Now, if you were just going to do a simple introductory physics problem you would say "neglecting air friction" the time to fall would be about t=√(2h/g)=√(16,000/10)=40 s and the speed at the ground would be about v=gt=10x40=400 m/s (about 900 mph); I would guess that would do some serious damage! But wait! It would be a really big mistake to say that air friction was negligible for something going 900 mph. The drag on a falling object in air depends on how fast it is going and can be approximated as F≈ Av²(all quantities must be in SI units) where A=πR²≈2x10^-5 m² is the cross sectional area. When the drag is equal to the weight, F=mg≈10^-2 N, the marble will stop accelerating and continue falling with the terminal velocity, v_t=√(4mg/A)≈45 m/s (about 100 mph). So, since 45 m/s is much less than the 60 m/s necessary for a bullet to penetrate the skull, I am guessing that no matter how high you drop the marble from, it will not cause truly serious injury it's going to hurt though!

QUESTION:
Some people believe that because we are about 70% water that the moon and the sun have some force upon us ( gravitational and electromagnetic), similar to the ocean. Scientifically, is there evidence that can support this?

ANSWER:
Water has nothing to do with it. The reason you are aware of the influence of the moon and sun on water is the tides; that is evident because the ocean has a lot of mass and it is easier to pull it up than, for example, to pull up a mountain. Nevertheless, there are forces on you because you have mass and anything with mass will experience a gravitational force from another object which has mass. Suppose that your weight is 150 lb your weight is the force which the earth's gravity exerts on you. The moon exerts a force of about 0.0005 lb and the sun exerts a force of about 0.09 lb on you. Even though the moon is much closer, the sun is much more massive. There is no signfiicant electromagnetic force on us due to the moon and sun.

QUESTION:
I have a question about light. If I am moving toward a source of light, why is it that the measured speed of said light is always constant? I've heard that motion is only relative with particles that have mass, but if I'm going half the speed of light towards a photon that's coming toward me, then why wouldn't I measure the photons speed as half the speed of light?

ANSWER:
I have answered this questions many times. See the links my FAQ page and the links in those links.

QUESTION:
As gas molecule come at rest at absolute zero temperature which is impossible to achieve and also kinetic energy depends on temperature then how is solid ice is formed

ANSWER:
Molecules in a solid are not at rest, they are vibrating like masses on springs. As the gas cools, its molecules slow down and eventually the speed is sufficiently slow that if one molecule collides with another they will bind together. Eventually more and more bind together making the solid.

QUESTION:
Here is the scenario. I have a A volt motor that supplies B watt to work. I connect the motor to a circular disc of R radius that is of M mass and has a density of 1 unit. I want to use the motor to move a conveyor belt of mass N. I will place an object of mass K on the conveyor belt. Is it possible to find the velocity of which the object will be moving at given the voltage? Or perhaps can we find the minimum about of voltage that is needed to move the object. Assume that the efficiency of all the motor as well as the whole thing is 100% and that frictional force and air resistance can be neglected. You can add othervariables in either.

ANSWER:
This is an engineering problem, not physics. However, it sounds to me like there is no answer if you have no frictional losses because the motor will just keep adding energy to the system and there is no loss.

FOLLOWUP QUESTION:
However, it should be able to work since the motor is attached to a conveyor belt, yes it will keep going but it will kep moving the conveyor belt only.

ANSWER:
If the motor is adding energy (B watts would mean B Joules of energy per second are being added) and there are no losses, where is that energy going? It will constantly accelerate the conveyer belt.

FOLLOWUP QUESTION:
Is it possible to find the acceleration of the conveyor belt?

ANSWER:
Yes! Refer to the picture above. Suppose the mass and the conveyor belt move with speed V; then the angular velocity of the disc is ω=V/R. The moment of inertia of the disk is I= MR². The kinetic energy of the belt plus mass is KE₁= (K+N)V² and of the disc is KE₂= Iω²= MV², so the total kinetic energy is KE= ( M+K+N)V². The rate of change of the kinetic energy is equal to the power B, B=( M+K+N)V(dV/dt)=( M+K+N)Va where a is the acceleration. Solving, a=B/[( M+K+N)V]≡C/V. Note that when V=0, a=∞; this just means that the motor cannot deliver power unless it is actually spinning with some rate. But, as soon as it gets moving it will have a very large acceleration which decreases as the speed gets bigger and bigger. So, if you are really interested in such a system, you will have to determine energy losses and probably also have a motor with an adjustable power output. (Also, note that the voltage has nothing to do with it, all you need is the power output.)

QUESTION:
What causes the universe to expand?

ANSWER:
For a long time it was assumed that the expansion was simply the result of inertia achieved in the big bang something that explodes sends its pices flying apart. More recently it has been discovered that the expansion is actually accelerating which implies some repulsive force is pushing out as well; this is generally referred to as dark energy.

QUESTION:
Could you explain why an electron in a stable orbit around a nucleus does not emit electromagnetic waves or photons. It only emits when the electron changes from a higher energy orbit. In a stable orbit the electron still is moving around so why doesn't it create a changing E field and radiate? Thanks, Paul

ANSWER:
The glib answer is simply that that is the way nature works. Here we encounter an example of how we should not extrapolate the behavior of something from a regime with which we have experience (for example, accelerating electrons in a transmitting antenna) to regimes where we have no experience (for example, tiny atoms). At the scale of atoms, the wave-like properties of electrons become important and quantum mechanics must replace Newtonian mechanics; when you solve the problem using the correct mechanics, stable orbits occur naturally. I find the easiest way to understand this qualitatively is to note that in an atom a stable orbit is one in which the wavelength of the electron is just right to form a standing wave in its orbit as shown to the right (de Broglie's picture of the Bohr atom).

QUESTION:
Say I have a simple bar magnet that I hold in my hand vertically. If I move the magnet side to side in a sinusoidal motion am I transmitting electromagnetic waves propagating at the frequency of the movement?

ANSWER:
Yes. The radiation is primarily magnetic dipole radiation.

QUESTION:
I've read that in circular motion things move in a circle because of the centripetal force, and that the direction of acceleration is towards the center for this reason. Now my question is that why don't things actually move towards the center of the circle if there is an unbalanced force directed there and an acceleration in the same direction

ANSWER:
Because "accelerate" is not synonymous with "move". For another example, when a ball is moving vertically up its acceleration is vertically down. The problem is that in everyday life we associate the word accelerate with speeding up but in physics acceleration is the change of the velocity. Velocity is a vector, a quantity which has both magnitude and direction, so there are two ways you can change it change its magnitude or change its direction. If the force happens to be parallel to the velocity, the object speeds up. If the force happens to be antiparallel to the velocity, the object slows down. If the force happens to be perpendicular to the velocity, the object changes its direction without speeding up or slowing down; that is what happens in uniform circular motion. If the force happens to be at any other angle to the velocity, the object changes both magnitude and direction.

QUESTION:
If you have two weights, where one is 100 grams and the other is 200 grams and you use the same spring to create a pendulum with them (one at a time) why isn't the amplitude the double for the heavier weight? (This is the result of an exercise we did, my personal curiosity, and my teacher's unwillingness to explain because he says it's too complicated for our level.)

ANSWER:
I think you must surely mean a mass on a spring oscillating vertically, not a pendulum. That is what I will assume. You have probably not studied energy yet which is why your teacher did not want to get into it. Energy methods are, by far, the easiest way to answer your question which is what I will do. Two things you need to know: potential energy of a spring which is stretched by an amount y is ky² and gravitational potential energy of something a distance y above where y=0 is mgy. Imagine taking a mass m and attaching it to a spring with spring constant k which is unstretched and holding it there for a moment. Where it is right now I will define to be y=0 and so, since the spring is unstretched, the total energy of the system is k∙0²+mg∙0=0; the energy never changes. As it falls it speeds up for a while and then slows down for a while (acquiring and then losing what is called kinetic energy, energy by virtue of motion), finally stopping and going back up. If it has fallen some distance A (for amplitude) before turning around, the energy is now 0= kA²+mg(-A) and so we find two solutions (it is a quadratic equation), A=0 (we already knew it was at rest there) and A=√(2mg/k). So, you see, the amplitude is proportional to the square root of the mass, not the mass. (Incidentally, most folks would call the amplitude of this oscillation to be A. I thought it would be clearer this way.)

QUESTION:
So, objects with energy give off some sort of radiation, some visable some not, and in higher energies and heats, the wavelengths get smaller. Can there be something so hot that the wavelength is smaller than a plank length?

ANSWER:
First of all, there is absolutely no proven significance of the Planck length, it is simply a combination of three fundamental constants, G, c, and ħ, which has the dimension of length, √(ħG/c³)=1.6x10^-35 m. And, you should not think of just fast or hot as being the source of photons (electromagnetic radiation) because it is energy change, not energy itself, which can result in radiation. There is no physical law that I know of which would forbid a photon with wavelength shorter than the Planck length. I should point out, though, that astrophysicists have difficulty coming up with models for how the most energetic cosmic gamma rays observed could have been created and they have wavelengths much longer than the Planck length.

QUESTION:
I came across a particle table one day, and I'm wondering why the amu for an element is constant. From my understanding, a proton is formed by two up quarks and a down quark but there are other particles that have the same charge as the up/down quarks but with different masses(stange,charm,top,bottom). And why can't tau and muon particles replace electrons since they also have the same charge as an electron.

ANSWER:
Regardless of what something is made of, if it is stable it has a definite mass. Muons and taus are not stable particles and so atoms with them replacing electrons are not going to appear in nature. Muonic atoms have been made but survive only fleetingly before the muon decays. I have never heard or a "tauic atom", probably because it has a much shorter lifetime than a muon and cannot therefore be made in copious amounts.

QUESTION:
In looking at the photgraphs from the Rosetta comet mission, I notice what seems to be "loose boulders" on much of the surface. How much gravitational attraction does that comet have? Is it enough to keep the boulders on the surface as it would on Earth, or are the loose parts of the comet simply in a coinciding orbit?

ANSWER:
If they remain in the same place relative to the main body of the comet they are resting on the surface. If they were moving alongside, there would be an attractive force which would bring them together; otherwise, to remain separated, the boulder would have to be orbiting the main body. I once answered a question which surprised me two dice, in empty space and separated by 10 cm, take only about 8 hours to come together.

QUESTION:
How long would it take for a rocket ship travelling at 100 times the speed of sound to reach the nearest star which is at 3.8 light years away?

ANSWER:
Take the speed of sound to be about 300 m/s. Then the speed of the ship would be about v=3x10⁴ m/s. Since the speed of light is about c=3x10⁸ m/s, v/c=10^-4 and so relativistic effects need not be considered. The time would then be about 3.8/10^-4=38,000 years.

QUESTION:
If like in the movie " Armageddon", a giant meteor were to be heading towards the earth, lets say a moon sized meteor moving at well over the speed of sound, assuming that this would wipe out the planet on impact, how much force do we need to completely annhilate it? Or at least render it harmless? Is the force required equal to F=ma , that is an equal and opposite force capable of destroying the planet on its own? Or something far less, capable of destroying the meteor with lesser mass is all thats required?

ANSWER:
To destroy the meteor completely requires a lot of energy. The energy to blast apart a uniform sphere of mass M and radius R is U=3GM²/(5R). For the moon, U=2.5x10³¹ J. Suppose that to just blow it to small pieces rather than total dust took a billion times less energy; then the energy would be on the order of 10²² J. The energy of the Nagasaki bomb was about 10¹⁴ J, so you would need about 10,000,000 of them. Clearly, it is hopeless to obliterate a moon-sized object. Better to catch it early and deflect it by a small angle which would be adequate to avoid collision. It would still take a good amount of energy for an object as massive as the moon, though.

QUESTION:
If I am at a point in space where very, very, very little gravitational pull exists, what happens to the time clock on my spaceship as opposed to clocks on earth?

ANSWER:
A clock in a gravitational field runs slower.

FOLLOWUP QUESTION:
What I am asking, if you were at a point in space, where the gravitational forces were almost 0, would your clock, in your space craft be running extremely fast as observed from earth? I was thinking about a spot in the universe were the gravitational effect would be almost opposite of a black hole. Like the trampoline example you used on the web site. I set 5 or 6 bowling balls on the trampoline so each makes a dent. I then use a marble and find a spot to set it where it is not affected by the bowling balls. Now I know my ship is moving always towards some mass but there has to be a spot where gravitational effects all almost 0. Maybe that spot in space would be inaccessible to my ship, kind of an anti event horizon where I would not have enough power to get too. a spot where Light can not even enter. I Know that my question is flawed, but I can't help to think about a black hole as a gravity pit, and that makes me think of a spot in space where there is a gravitational mountain (Anti-Black Hole, with an anti-Event horizon). In this spot time runs so fast as not to exist....

ANSWER:
First of all, all your thrashing around with black holes etc. trying to find a spot with small gravitational field is totally unnecessary; all that is required is that you need to be far away from any mass and nearly the whole universe satisfies that criterion. We generically call such places intergalactic space. So, choose some place, maybe halfway between here and the Andromeda galaxy, our nearest galactic neighbor. For all intents and purposes the field there is zero and a clock would run at some rate. Now, take that clock and put it on the surface of a sphere of radius R and mass M. The gravitational time dilation formula you need to compute how much slower the clock would tick at its new location is √[1-(2MG/(Rc²))] where G=6.67x10^-11 N∙m²/kg² is the universal constant of gravitation and c=3x10⁸ m/s is the speed of light. Putting in the mass and radius for the earth, I find that the space clock runs about 7x10^-8% faster than the earth clock, certainly not "extremely fast"! The bottom line here is that, except very close to a black hole, gravitational time dilation is a very small effect. An interesting fact, though, is that corrections for this effect must made in GPS devices because they require extremely accurate time measurements to get accurate distance measurements.

QUESTION:
My physics lecturer tried his best to answer my question on what exactly a spin in electron is. But I couldn't understand. Besides he himself looked quite confused. So could you kindly help me out? What is generally a spin of an electron? And how would I explain it to a layman?

ANSWER:
Take the earth as an analogy. Earth has two kinds of angular momentumit revolves around the sun and it rotates on its axis. Angular momentum refers to rotational motion of something. Its motion around the sun is called orbital angular momentum and its spinning on its axis is called intrinsic angular momentum. Now think about an electron in an orbit around a nucleus in an atom. It clearly has orbital angular momentum because of its orbit. But it also has intrinsic angular momentum, that is, it behaves in many ways as if it were spinning on its axis; this is usually referred to simply as spin. This is a fine analogy, but it should be emphasized that spin is not really such a simple classical concept. For example, if you were able to exert a big enough torque on the earth you could stop its spinning or make it spin faster. The spin of an electron is a constant property and cannot be changed. You can change the direction (clockwise or counterclockwise) but not the magnitude of spin. Also, if you try to model the electron as a little spinning sphere to find out how fast it is spinning, you get absurd, unphysical answers. So, you can only push the electron/earth analogy so far. Finally, I should note that in relativistic quantum mechanics (Dirac equation) the existence of electron spin is predicted to be exactly what it is measured to be.

QUESTION:
I intend to heat a sealed tank to 280 degrees fahrenheit to cook lumber. I would like to be able to keep the pressure at a contsant 40-50 psi inside the tank. I need to know how much the pressure will increase inside the tank as I add the heat. I don't want the tank to blow up on me while it's being heated. I plan on using heating elements inside the tank. If needed I will also add pressure to the tank to get to 40-50 psi.

ANSWER:
The operative principle here is the ideal gas law, PV=NRT where P is absolute pressure, V is volume, N is the amount of gas, T is absolute temperature, and R is a constant. The pressure numbers you gave are, I assume, gauge pressure, the amount above atmospheric pressure which is about P₁=15 psi, so your target absolute pressure range is P₂=55-65 psi. The temperatures must be converted to kelvins (K), absolute temperature: I will take room temperature T₁=70⁰F=294 K and T₂=280⁰F=411 K. This is all we need to know to find the final pressure assuming V, N, and R do not change: P=P₁(T₂/T₁)=15(411/294)=21 psi. This is well below your target pressure. Probably the easiest thing to do is to increase the pressure before you heat it by pumping in room-temperature air. Suppose you want a final pressure of about 60 psi (45 psi gauge pressure), then the room-temperature pressure you should start with should be P₁=60(T₁/T₂)=P₁=60(294/411)=42.9 psi=27.9 psi gauge pressure.

All this assumes that your tank is strong enough to take these pressures and I cannot guarantee that. I strongly suggest that you be certain you have a pressure guage on the tank as well as a pressure relief valve which will keep the pressure from getting too high.

QUESTION:
What frequencies of light or radiation will petroleum jelly absorb. What is happening at the nuclear level when petroleum jelly adsorbs light from a black light and turns it into visible light.

ANSWER:
Your first question is far too broad to answer here. If it really matters to you, you can get a lot of information here. The second question is easier to answer with a qualitative answer. First, nothing goes on at the "nuclear level", it all happens at the atomic or molecular level. Ultraviolet light has enough energy to excite the electrons in the molecules to a very high excited state; then, rather than dropping back down to the ground state, they cascade down through many levels emitting many much less energetic (longer wavelength) photons which happen to be in the visible range. The picture to the right is a smiley face made of petroleum jelly and illuminated by a black (ultraviolet) light. This phenomenon is called fluorescence.

QUESTION:
How fast would a more massive planet than Earth, say twice as massive, with the same diameter have to spin to produce an Earth-like sense of gravity, due to centripetal force, at its equator? Might there exist a massive Earth-like planet, environmentally speaking, that we could still live on because its spin negated its gravitational pull enough to produce an Earth-like sense of gravity that wouldn't crush us?

ANSWER:
Let's just do it in general, the pseudo-earth being N times more massive than our earth. The apparent weight at the equator would be Nmg-F_c=mg where F_c=mv²/R=mRω²is the centrifugal force. Solving, ω=√[(N-1)g/R] where ω is the angular velocity in radians per second. For example, if N=2, ω=√[g/R]=√[9.8/6.4x10⁶]=1.24x10^-3 s^-1=2π/T where T is the period. So, T=5.08x10³ s=1.41 hours.

QUESTION:
Consider two identical coins placed one over the other. Now the force of gravitation acting between them should be infinte as gravitational force varies inversely to the distance between their centres which is very very small so force of gravitation between them should be infinite and we couldn't be able to separate the two bodies. But it is not true we easily separate them. So basically what is mistake/error that I have made?

ANSWER:
First of all, very small does not mean zero. The force will certainly not be infinite. Let's do an approximate calculation for two US pennies, mass of 2.5 g=2.5x10^-3 kg, thickness of 1.52 mm=1.52x10^-3 m. So their centers of mass are separated by 1.52x10^-3 m. I will treat them as point masses at their centers since an exact calculation would be pretty complicated and I just want to demonstrate that the force will certainly not be infinite. The force of gravitational attraction is F=(2.5x10^-3)²(6.67x10^-11)/(1.52x10^-3)²=1.8x10^-10 N. The weight of each penny is 2.5x10^-3x9.8=2.5x10^-2 N.

QUESTION:
My question is one of curiosity regarding the LHC. If I am correct, when you accelerate an object to relatively high speeds then the mass of the object increases. As far as I know, at the LHC they are accelerating lead nuclei near the speed of light. My question is what is the mass/energy of these nuclei when they reach top speed? Mostly curious on something I can compare it to on a classical scale. I understand that nuclei are very small and don't have much mass but with that amount of energy put into them I would imagine that the impact is tremendous for their size.

ANSWER:
I have previously worked this out for a relativistic proton at 99.99999999% the speed of light. A lead nucleus would with this speed would have about 200 times the mass of a proton, so its mass would be about 2.4x10^-20 kg; a baseball has a mass of about 0.15 kg. Since the mass of the lead nucleus at rest is about 3.4x10^-25 kg, its energy is nearly all kinetic, K≈mc²=0.002 J; a 100 mph fast ball has a kinetic energy of about mv²≈150 J. As in the earlier answer, the mass has increased by a factor of about 70,000 but is still very tiny.

QUESTION:
I know that when electrons get excited they basically move further from the nucleus, but what happens when the nucleus itself goes into an excited state?

ANSWER:
Nuclear structure is more difficult to understand because, unlike an atom, the main force felt by the nucleons (protons and neutrons) is not from some central point but from their nearest neighbors. It was therefore somewhat surprising to find that many aspects of nuclear structure may be understood by considering the nucleons as moving in an average central potential and in well-defined orbits, similar to how electrons in an atom move; this is called the shell model of the nucleus. So one way to excite a nucleus is to simply raise some particular nucleon to a higher orbit as happens in atoms. These are called single-particle states or shell-model states. However, nuclei may also be viewed as fluid drops which behave collectively, that is, all the nucleons move together. Most nuclei are spherical and can be excited simply by causing them to vibrate imagine a liquid drop which is sloshing around in a rhythmic way. The picture to the left shows the most common shape vibration of nuclei, the quadrupole vibration; the original nucleus (dot-dash line) oscillates between football-shaped (American or rugby) and doorknob-shaped, passing through the spherical shape as it vibrates between the two. Some nuclei are not spherical, usually football shaped, and these nuclei may be excited by being made to rotate.

QUESTION:
When a string acting as a standing wave is plucked/oscillated at its fundamental frequency (like that of a guitar), is there a relationship between the amplitude of the string itself and the amplitude of the sound it makes. If so what exactly is it? I know that the string will be a transverse wave while sound is a longitudinal wave, so im not sure how exactly to convert the amplitudes if there is a way to do so.

ANSWER:
Take a guitar string and connect it between two rigid points (two granite walls, for example) and pluck it. You will hardly be able to hear it at all. A guitar is a complex instrument and it is really the vibration of the guitar itself and the air inside it that is mainly what you hear the string excites the guitar and it acts like an amplifier. The response of the guitar to the vibrating string depends on the quality of the guitar or else there would be no reason to have some guitars much more expensive than others. So there is no simple relationship which you seek between the amplitude of the string and the amplitude of the of the resulting sound. I would think, up to a point, you could roughly estimate that the two were proportional. However, you also have to factor in that a guitar string does not vibrate with only the fundamental frequency but also includes all the overtones and the guitar will respond differently to the different overtones.

QUESTION:
Could an object theoretically achieve light speed if it were falling far enough for long enough? Since speed increases the closer a falling object gets to it's periapis, could an object with an apoapsis incredibly high and an incredibly low periapsis fall for long enough that it would reach speeds close or surpasing the speed of light?

ANSWER:
No object could ever reach the speed of light. See my faq page for why not. No matter how long a particle falls through a gravitational field, it can never reach the speed of light. An earlier answer works out the speed as a function of time for an object falling through a uniform field. Classically, if you have two point masses they will accelerate toward each other with ever increasing acceleration such that when they are coincident their speeds will be infinite. But when either of their speeds become comparable to c they are no longer classical and their speeds will approach c instead. Also, there is no such thing as a true point mass.

QUESTION:
From a spaceship that moves away from you very quickly (say 0.33c), a "photon" is emitted when the spacecraft clock marks the time t0 and a length measurement performed on the ship tells your distance is 1 light year. How long does the photon to reach you? with times measured by you and by the ship?

ANSWER:
The distance from you to the ship, as measured by you, at the time the photon is emitted is 1/√(1-.33²)=1.06 ly and so the time it takes to reach you is 1.06 yr. An observer on the ship says it takes 1 yr.

QUESTION:
Suggest that we have constructed a model of the solar system by decreasing the sizes of all orbits by n = 0.005. We have kept the average density of the planets and that of the Sun the same as in the original solar system. How will the periods of rotations of planets change in our model?

ANSWER:
Is this homework? Are the sizes of the planets also scaled by n?

QUESTION:
No this is not homework-it was in a past paper and I was curious as to how to answer it! And the question does not say, although I assume so!

ANSWER:
The period T of an elliptical orbit with semimajor axis a around a body of mass M which is much bigger than the mass of the orbiting body is given by T=2π√[a³/(GM)] where G is the universal gravitational constant 6.67x10^-11 N∙m²/kg². So the answer to the question depends on how the masses are scaled. If all the masses stay the same, the new periods T' are simply scaled by √0.005³=3.54x10^-4. If the densities remain the same and the sizes of the sun and planets themselves are also scaled down by 0.005, then the masses will scale by the factor M'=0.005³M because the mass is the density (unchanged) times the volume (proportional to the cube of the radii). Therefore the periods would remain unchanged, T'=2π√[(0.005∙a)³/(G∙0.005³∙M)]=T.

QUESTION:
I measure 179cm in height and exert 194lbf on a scale. If I were to gain 61cm in height and keep the exact same body proportions in relation to the height I have now, how much force would I exert on the scale? (This is not homework. I'm an author. I did some math myself, and came up with a factor of about 2.4 for the weight increase, but I wanted a professional opinion.)

ANSWER:
If all dimensions of an object increase by a factor N, the volume increases by a factor N³. In your case N=(179+61)/179=1.34, so the volume increases by a factor of 1.34³=2.41. Assuming that the density of all body parts remains constant, the weight would then be 468 lb. Looks like you nailed it!

QUESTION:
How we can describe formation of a stationary wave in open organ pipe? How is the wave reflected at the open end?

ANSWER:
It is a property of all waves that, when the medium in which the wave is traveling changes its physical properties, reflection can happen. The properties of the air certainly changes at the open end it is contained inside a tube inside and not constrained outside.

QUESTION:
Why is uranium the most common element used in nuclear fission?

ANSWER:
It is the only naturally occuring fissile nucleus.

QUESTION:
Which scale in temperature is larger? For example, in terms of distance we have meter and kilometer 1 km=1000 m, so km is larger than meter. In terms of temperature,we have celcius and kelvin 273k=0 C, 373k=100 C. By similar concept i may say celcius is larger than kelvin. But I'm told by my teacher that the conversion formula suggests kelvin is a larger scale. So which is a larger scale?

ANSWER:
Your confusion is that you do not define carefully what you mean by "which scale is larger?" For example, you say that, for scales of distance, a kilometer is larger than a meter which is certainly true; but in any given length there are more meters than kilometers so you could say that the meter gives a bigger number than the kilometer does. I prefer the first definition whereby you compare the sizes of the units. For temperature, 1 K is exactly equal to 1⁰C, so both you and your teacher are wrong the scales are the same. In terms of magnitude, though, the temperature of something measured in Kelvins is always a larger number than if measured in degrees Celcius.

QUESTION:
When I was young my father had a glass of water from our well that never froze at -40 degrees Farenheit? What else was in the water that would make this happen?

ANSWER:
Did your father have a still in the well house? Sounds like grain alcohol to me, freezing point -173⁰F.

QUESTION:
We know in mechanics that F=ma. But then in electric fields force on a charge is given by Coulomb's law. Both are forces. What bothers me is that can they be shown to be the same in a way. Like is there a way to compare them? I'm just confused about this.

ANSWER:
F=ma, Newton's second law, is telling you what happens if you exert some force F on some mass m; you should maybe think of it as a=F/m. Coulomb's law tells you what the force is between two point charges, F=kq₁q₂/r². You could now examine the special case of charge q₁ which happened to have a mass m₁ and be a distance r from charge q₂: it would have an acceleration a=kq₁q₂/(r²m₁).

QUESTION:
Two people are on a high speed, constant velocity train on opposite ends. One person standing on the platform. The two people aim a laser beam at the center of the train at the same time in their reference frame. In the center of the train, there is a special gun that will shoot and kill whoever's laser beam reaches it first; but if the two beams reach it at the same time it will be disarmed. From the person on the platform's standpoint, one of them dies due to the relativity of simultaneity. From the two people on the train's standpoint, they both live. Later, the two people get off their high speed train and meet with the person on the platform. Is this not a paradox? The platform person clearly saw one of them die.

ANSWER:
The only thing that matters is what the "special gun" sees; it is in the same inertial frame as the shooters, so it does not fire because the two pulses arrive simultaneously in that frame. The guy on the side sees the pulses not arriving simultaneously but the gun not firing.

CORRECTION:
It has been pointed out to me by John Disciullo that the above answer is wrong. Here is his answer:

ANSWER:
I think the person on the side will see the lasers arriving simultaneously at the gun and disarming it. What will not be simultaneous to the observer on the side is both people shooting. He will see the person at the back of the train shooting first and the person at the front of the train shooting slightly afterwards. The laser shot from the back of the train, even though fired first, arrives at the same time as the one shot from the front since the special gun is moving away from the back laser pulse and towards the front one.

QUESTION:
All the electrons in an atom keep moving (vibrating) year by year from where they get this much of energy? And why the energy does not finishes like our energy finishes after some time?

ANSWER:
When you say " energy finishes after some time " I suppose you mean like you have to fill up your gas tank in order to keep your car moving. The only reason you have to do that is because friction is constantly taking energy away from your car so energy is being lost. But electrons in atoms do not experience friction and, once they are given energy in the first place, the energy will never go away. Imagine a mass hanging on a spring and subject to no friction; once you start it vibrating (give it some energy), it will never stop.

QUESTION:
I am inside a train in which all the sides/windows are sealed i.e., I cannot see anything from inside. If the train moves in a friction-less rail, i.e., without jerking, how can I prove that there is motion taking place or there is displacement?

ANSWER:
There is no such thing as absolute velocity, so there is no way you can determine if you are moving with a constant velocity. The principle of relativity says that the laws of physics are the same in all inertial frames of reference so there is no experiment you could devise telling you what your speed is. If your train were on earth, then you would not be in an inertial frame because the earth is curved and rotating and very careful measurements could detect this, but I believe you meant that the train is moving in a perfectly straight line and no earth motion effects.

QUESTION:
Is higher frequency really more energy? It is thought that higher frequency has more energy. However, the higher the frequency the smaller the wavelength. With that said, though the higher frequency is delivering more energy to a a given location, it is giving less total energy in a given area. A lower frequency is giving less energy to a given location, but more energy in an area. So the TOTAL energy of the wave would seem to be equal. Greater area(wavelength), lower frequency. Higher frequency, lower area(wavelength).

ANSWER:
I am afraid your thinking is muddled. Electromagnetic waves of a given frequency are a collection of particles called photons; the smallest amount of electromagnetic wave you may have is a single photon which will correspond to a particular frequency. The energy E of a single photon is proportional to its frequency f, E=hf where the proportionality constant h is Planck's constant. So if 1000 red photons hit a wall and 1000 blue photons hit a wall, the blue photons will bring the most energy.

QUESTION:
Is it possible to derive the formula for Kinetic Energy without using work? Or are they linked by definition?

ANSWER:
Well, I can tell you that you never have to utter the phrase "work is defined as " The work-energy theorem is merely the integral form of Newton's second law. For simplicity, I will do this simple derivation in one dimension. F=ma=m(dv/dt)=m(dv/dt)(dx/dx)=m(dv/dx)(dx/dt)=mvdv/dx. Rearrange: Fdx=mvdv. Integrate: ∫Fdx=m∫vdv= mv₂²- mv₁² where the integral on the left is from x₁ to x₂ and is usually called the work W. Generalizing to three dimensions, ∫F∙dr= mv₂²- mv₁² where the integral on the left is from r₁ to r₂.

QUESTION:
How can we know the true mass of an object in space with no gravity? What's holding us back from moving at light speed without restrictions of gravity? From what I understand we should be able to reach light speed with a burst of energy that should be almost instantaneous.

ANSWER:
I will take "true mass" to mean rest mass. The mass m is a measure of the inertia, the resistance to acceleration when pushed on by a force F. The relation is m=F/a where a is the acceleration of m resulting from F (as measured for velocities small compared to the speed of light); this is Newton's second law. So if you push on the object with a known force and measure the acceleration, you will have the "true" mass. Gravity has nothing to do with how fast something can go. For a more detailed description correct at higher velocities, see an earlier answer.

QUESTION: (This question refers to an earlier answer.)
With respect to your ANSWER above, I have read elsewhere that one of the observations from particle accelerator experiments is that the mass of a particle increases the more it is accelerated AND that there is a concomitant release of energy (I recall reading release vs expenditure or other terminology, but the ol memory could be faulty, too). I read in your ANSWER above about inertia being what increases versus size/"amount of stuff" as a given mass accelerates. Per my outside reading I am attempting to conceive the source of the energy released, not in mathematical terms, but with creative logic, if there is such a thing. Maybe not. Math might be the only way to explain the phenomenon. My attempt with English in question form: Is the measurable release of energy due to the fact that so much more energy is required to continue accelerating the particle? Is the source of the "released energy" I read about actually the instrument itself, the particle accelerator? For some reason, prior to reading your ANSWER, I conceived that as mass/inertia increases [as acceleration takes place] there was some inherent energy in the particle itself that with the added force of acceleration, inevitably had to be expressed / released. Creative huh? What IS the source of that energy "released" as the particle accelerates and its inertia increases? Or am I so way off you re having a cleansing, releasing, hearty laugh? And, can this concept be explained in language that an extremely uneducated but by no means unintelligent person can understand? Are you that good? I m giving you lots of credit, much deserved for your dedication to askthephysicist. If answering requires math, my reading said answer is not likely to be helpful so save yourself the time and move on with your day.

ANSWER:
I have never encountered reference to such an "energy release" by relativistic particles as they were accelerating. While the particle is accelerating, the force accelerating it does work which increases the energy of the particle. The only math I will use here is E=mc² which I hope you can work with this simply says that any particle has an inherent energy due to is its mass m which is the mass (inertia) times the speed of light c squared. If a particle is at rest, it has energy because it has mass. If a particle is moving, the classical view is that it also has what we call kinetic energy, energy by virtue of its motion. There are two ways you can look at it. One is that we observe that the mass m increases as the particle speeds up, eventually approaching infinite mass as the speed approaches the speed of light; in this view, kinetic energy is simply folded into the quantity mc². The other way is to say that the particle has an inherent mass energy m₀c² where m₀ means the value that m has if the particle is at rest; in this view we interpret the energy of the particle to be rest mass energy plus the kinetic energy K it has by virtue of its motion, m₀c²+K. These are just two ways of looking at the same thing; therefore, if the particle retains its identity, if it now interacts with its environment it can release the energy it previously gained by accelerating and if it ends up at rest it will have released K Joules of energy. Any energy this particle "releases" can only come from energy which it previously acquired.

ADDED THOUGHT:
An accelerated particle does radiate electromagnetic energy (light, x-rays, etc.) called bremsstrahlung; this is how an antenna works. So, when a particle is being accelerated, it does "release" a bit of energy in this way. The rate of this radiation is usually quite small compared to the rate at which energy is being added and is often ignored when considering accelerators. That which you "read elsewhere" might have been referring to bremsstrahlung.

QUESTION:
How come when I suck on an empty glass bottle hard enough my lips get pulled into the opening of the bottle?

ANSWER:
When you remove air from the bottle, the pressure inside the bottle is lowered compared to normal atmospheric pressure. It is the atmospheric pressure on the outside which pushes your lips into the bottle.

QUESTION:
I hope this question is stupid and is a very quick answer. If i have an object submerged in a tank of water, say a solid cylinder, obviously the pressure at the top of the cylinder will be less than the pressure at the bottom of the cylinder, due to the difference in depth between the two points (P=pgh). What I would like to know is how this difference in pressure exerts a force on the cylinder, and where? Is the force acting on the bottom of the cylinder, trying to push it to the surface? I just can't wrap my head around this at all.

ANSWER:
Sorry to disappoint you, but your question is not stupid. You have discovered Archimedes' principle. Since, as you note, the pressure at the bottom of the object (pushing up) is greater than the pressure at the top of the object (pushing down), there is a net push up. This force is called the buoyant force. It may be shown that the buoyant force is equal to the weight of the water displaced by the object. The buoyant force determines whether an object floats (buoyant force greater than weight) or sinks (buoyant force smaller than weight) and why swimming is sort of like flying.

QUESTION:
If a single seed can produce a huge tree then how can we say that the mass of the universe is constant?

ANSWER:
We can't say that the mass of the universe is constant; the energy of the universe is constant. Every day, for example, the sun's mass decreases because mass (hydrogen) is being turned into energy plus different smaller mass (helium). In your example, the mass of the tree was not created from nothing. The tree took water, carbon dioxide, and nutrients from the soil and using energy from sunlight it built itself. All the mass and energy are accounted for.

QUESTION:
If you have a space station with a 95 meter diameter gravity deck at one g what would the gravity be if you put another deck 9 meters above it?

ANSWER:
So the radius of your primary deck is 47.5 m and your secondary deck has a radius of 56.5 m. The pertinent equation is ω=√(a/R) where a is the acceleration, R is the radius, and ω is the angular velocity. For your primary deck ω=√(9.8/47.5)=0.45 s^-1. The secondary deck has the same ω, so 0.45=√(a/56.5) or a=11.7 m/s² which is about 19% larger than g=9.8 m/s2. I should point out that since a person has a size on the order of 2 m, this will not be a very comfortable environment since the gravity at your head would probably be noticably different than at your feet.

QUESTION:
I came across your site while looking for the answer of a physics problem I would like to program in a Smartphone app. A toy car is dragged from position A to B giving it an initial velocity (v=d/t). At point B the car is released where it travels in a straight line until the frictional force of the ground stops the toy car completely (position C). I would like to find a formula that relates the distance from B to C with the initial velocity provided from A to B.

ANSWER:
Of course, you have to know the frictional force f and the mass m of the car in general. Assuming that the force is constant all along the path B to C, the distance s can be written s= mv²/f. In most cases f is proportional to m so that you do not need to know the mass. If you are on level ground, f=μmg where μ is called the coefficient of friction and g=9.8 m/s² is the acceleration due to gravity (mg is the weight of the car). The coefficient just is a parameter which is small if there is little friction. So, finally you have s=v²/(2μg). If the car is on a slope making an angle θ with the horizontal, s=v²/(2μg∙cosθ).

QUESTION:
Okay I have wondered this question for many years, and more over have wondered how to ask it! So I think a scenario is best; when an object is propelled forward or in any direction very rapidly, let's say for this case a bullet out of a gun, does it advance through every speed in between it's current speed, zero, and it's maximum velocity or does it simply "jump" from zero to it's maximum velocity? And also the same for when it hits its target, let's say a thick steel plate does it go from its current velocity straight to zero, or is there some sort of slowing down in which it goes through every speed in between?

ANSWER:
Fundamental to classical physics is Newton's second law. That which changes the speed v of something is a force F, a push or a pull. Further, the bigger the rate of change of speed (acceleration a), the bigger the force double the force and you double the acceleration. This is often written as F=ma where m is the mass of the object. Suppose that you shoot a bullet from a gun. The bullet starts with a speed v=0 and ends with speed v and this happens in some time t and so the acceleration can be written as a=v/t. If, as you suggest, the bullet "jumps" instantaneously to v, then t=0. But for t=0 the acceleration would be infinitely large which would imply that you had to push on it with an infinite force. The same reasoning can be applied to stopping the bullet. You know that the force propelling or stopping a bullet in the real world is finite. It is a pretty good rule-of-thumb for everyday occurances that there are no infinities or discontinuities (instantaneous changes) in the universe.

QUESTION:
I am a member of US Coast Guard Forces. I am trying to describe to people how hard it is to find them so wear a life-jacket so we have more time. I am looking for an 'apparent size' formula, i.e., supposing someone's head is 1 ft high, what is its apparent size to me, on a boat 200 ft away, looking for that person (who hopefully still has his head above water!) 200 ft is ~ 1/10th of a nautical mile and is a standard separation distance for search and rescue patterns to run at.

ANSWER:
The way astronomers determine relative sizes of objects in telescopes is the angle subtended by the image of the object. The angle, θ, in radians, is given by θ=s/R where s is the size of the object and R is the distance away. So if someone's head is 200 ft away, it will be smaller by a factor of 20 than if it were 10 ft away. But you are actually looking at the area of the head, and that is proportional to the square of its size, so that area would appear to be 400 times smaller than if it were 10 ft away. Another way to put it is that at 200 ft a 1 ft diameter head would look like a 12/20=0.6 inch ball at 10 ft, maybe like a grape 10 ft away.

QUESTION:
Please help a work related dispute. Can the weight of a patient in a wheelchair be calculated using f=Ma? Even if it's a guesstimate? I thought, if m= F/a Where a = V₂-V₁ / t Where V₂ is the velocity of the chair (and porter?) and V₁ is coming to a stop. Would F be the mass of the porter multiplied by the common acceleration of chair and porter? I'm guessing if I clock the speed of the porter I can calculate a.

ANSWER:
I guess that "porter" means the guy pushing the chair. You have it all wrong, I am afraid. But, it is worth talking about for a bit. There are three masses involved here, M_patient, M_chair, and M_porter. There is an acceleration a which we can agree could be roughly measured by measuring times and distances. Suppose we first look at all three and call the sum of their masses M. Then M=F_all/a where F_all is the force which is causing the collective mass to stop. If you neglect the friction which would eventually stop the wheelchair with no porter, F_all is the frictional force between the porter's feet and the floor; you do not know that force. Suppose you focus your attention on the patient. M_patient=F_patient/a where F_patient is the force responsible for stopping the patient. This would be the frictional force the seat of the chair exerts on the patient's butt; you do not know that force. Suppose you focus your attention on the porter. M_porter=F_porter/a where F_porter is the force responsible for stopping the porter. If we call the force with which the chair pulls on the porter F_c-p, then F_porter=F_all-F_c-p; you do not know either of these forces. I could go on and focus on the chair alone next, but you can see that you do not know any of the forces which are responsible for stopping any or all of the masses, so you cannot infer the mass of any of them. Just knowing the acceleration, you cannot infer the mass.

QUESTION:
Since radio waves are electromagnetic waves, do they attract Iron?

ANSWER:
The magnetic field in a typical radio wave is extremely weak compared to a simple bar magnet and its effect on a piece of iron would be very hard to observe. Also, since the fields are rapidly oscillating, the average field over a period would be zero.

QUESTION:
I am writing a couple of SF novels that take place in significant part on a beanstalk station, i.e. a space elevator station located in geosynchronous orbit, and my question concerns the design. In my stories, the station is about a half mile wide with a shape similar to a hockey puck. The center third of the puck is attached to the elevator ribbon (both up to the counterweight and down to the planet of course) and fixed, therefore essentially in freefall. My question concerns the outer section, which in my story spins in order to provide some g-force: is this a viable model? Some of my concerns are that the spin will create a gyroscopic effect as the station orbits around the Earth that will twist it off the ribbon or perhaps exert other forces on the hub that I'm not accounting for. One possible solution I thought of would be to split the outer section into two rings rotating in opposite directions to cancel out those effects. Assuming some clever engineer works out the minor details of moving between the hub and the rotating section(s) is this design plausible? I like the science in my science fiction to be accurate and reasonable.

ANSWER:
I believe you are right to worry about the rotation causing problems. If you just forget about the elevator and have a rotating "puck station" in orbit, its axis will always point in the same direction in space (conservation of angular momentum); so, if it were in a geosychronous orbit, from earth it would appear to do a 360⁰ flip every day. I think that having a counter-rotating ring would be a very good idea, the ring having the same angular momentum (moment of inertia times angular velocity) as the main station. If the station has a radius of about a quarter of a mile, about 400 m, the angular velocity ω needed to have an earth-like artificial gravity (g=9.8 m/s²) at the edge would be ω=√(g/R)=0.16 radians/s=0.025 revolutions/s≈1.5 rpm.

QUESTION:
In the Meissner effect; when the falling bar magnet's descent is arrested due to the magnetic impermeability of the supercooled lead dish toward which it is falling, what happens to its inertia? Does the field around the bar magnet deform and absorb it, or does something else occur?

ANSWER:
It is essentially the same as if the magnet were attached to an unstretched spring (no superconductor) and dropped; as the magnet falls, gravitational potential energy decreases and spring potential energy increases but faster. The magnet will speed up until eventually you reach a point (equilibrium point) where the force from the spring (up) is the same as the weight of the magnet (down) and then it slows down, eventually going back up and so on. Because there are drag forces (air drag and the damping in the spring) it settles down to the equilibrium point. In the Meissner effect, the magnetic field of the magnet plays the role of the spring in my simple analogy. Because the superconductor excludes the field, the field deforms as the magnet falls and pushes back up harder on the magnet as it gets closer to the superconductor which means that the energy of the field is increasing. You were essentially right in your speculation that " the field around the bar magnet deform[s] and absorb[s] " the kinetic energy.

QUESTION:
Regarding a atomic blast. What are what appears to be thin streamers on the outside of the mushroom cloud? They are some distance away from the cloud and go from the ground to the cloud itself.

ANSWER:
I assume you mean like shown to the left. These are the contrails of rockets shot off just before the blast; their purpose is to detect the shock wave from the explosion.

QUESTION:
Is it true that even an electron has its north and south poles; if it is, then how?

ANSWER:
A simple bar magnet (shown blue) has a magnetic field shaped as shown by the right-most figure to the right. What is fundamental is the shape of the field, not the N and S poles of the bar. This is called a magnetic dipole field. To the left of this is the magnetic field caused by a current loop. Notice that this little current loop has a very similar field shape, so you could identify its N and S poles. An electron has an angular momentum, that is it is spinning. A spinning ball of electric charge is like a stack of tiny current loops, so it will also have a dipole-shaped field. Therefore you could say that an electron has a north and south pole.

QUESTION:
What makes neutron radiation so dangerous?

ANSWER:
Mainly, it is because they can cause an atomic nucleus to become radioactive. If a neutron is absorbed by a nucleus of an atom in your body, it will most likely become a radioactive nucleus which will decay and the decay products (beta or gamma rays) will be ionizing radiation which will do damage to the molecules in your body.

QUESTION:
Hypothetically, what would be the energy (in joules) required to maintain a specific car (described below) at 50 mph on a flat concrete surface for 1 mile. No, it's not homework. I'm a 20 year old college dropout. It's a design I started in college and wanted to finish. The specifics of the car are as follows:

Total weight: 205 lbs
rolling resistance: μ=0.0025
drag coefficient: c_d=0.14
Frontal Surface area: A=13.295 ft²

ANSWER:
First you need to find the rolling friction which is easy because on level ground the force is the coefficient of friction times the weight: F_r=0.0025x205=0.51 lb. The drag friction is a bit harder because it depends on the speed v=50 mph=73.3 ft/s and the mass density of the air, ρ≈0.002377 slugs/ft³ (I hate working in English units!): F_d= ρv²c_dA= x0.002377x73.3²x0.14x13.295=11.89 lb. So the net force is 12.40 lb which is, of course, the force you must exert over the distance of a mile; therefore the energy required is the work done which is W=12.40x5280 ft∙lb=65,449 ft∙lb=88,737 J. Just to check that this is reasonable, you could calculate the power, energy per unit time. The time to go one mile is about 72 s and so the power delivered to the car is about 88,737/72 J/s=1232 W=1.65 hp. That seems on the low side to me, but that is how it comes out with your numbers.

To double check, I redid the calculation in SI units: First you need to find the rolling friction which is easy because on level ground the force is the coefficient of friction times the weight: F_r=0.0025x93x9.8=2.28 N. The drag friction is a bit harder because it depends on the speed v=50 mph=22.4 m/s and the mass density of the air, ρ≈1.225 kg/m³: F_d= ρv²c_dA= x1.225x22.4²x0.14x1.235=53.14 N. So the net force is 55.42 N which is, of course, the force you must exert over the distance of a mile; therefore the energy required is the work done which is W=55.42x1609 J=89,171 J. Just to check that this is reasonable, you could calculate the power, energy per unit time. The time to go one mile is about 72 s and so the power delivered to the car is about 89,171/72 J/s=1238 W=1.66 hp which is about the same answer as above.

QUESTION:
Hello, we are having a debate over which syringe and needle are less painful for injections. Assuming the exact same amount and viscosity of the material (whether normal saline or a viscous medication), if a 3cc syringe is attached to the same 30gauge needle as a 1cc syringe, which is less painful to receive the injection? The 3cc syringe is appx the same length of the 1cc syringe, with a wider barrel. Some feel with Poisoille law, the velocity of med pushed is slower with a 1cc syringe, so the med injected slower in 1cc is less painful than a 3cc. Some feel the 3cc is less painful because the pressure of pushing is dispersed over a larger diameter, so less pressure is less pain. Others feel, that even though the 3cc is wider than 1cc syringe, all is neutralized because the 30g needle attached is the same. We are assuming the pain of the injection is related to the push of the medication, but cant decide if velocity of flow or pressure of push is the main factor. We know if med is pushed quickly, it is more painful. But we can't quantify the difference between 1cc and 3cc syringes, other than anecdotes... Can you help with a physics explanation?

ANSWER:
I am not a physician, so I cannot speak to the question of pain. I have considered a very similar question before and concluded that Poiseuille's law is irrelevant here. The only difference between the two is that the 3 cc syringe, having a larger barrel than the 1 cc syringe, will result in a smaller pressure pushing out the medication if both are pushed with the same force; with identical needles, therefore, the 1 cc syringe will result is a faster fluid flow. So, if you know that faster is more painful, the 3 cc syringe should be used.

QUESTION:
Let's say you're playing baseball and your standing in the field by a base and someone threw the ball to you so that you could get the person running to the base you're by out. So you look and you see the ball coming towards you. Is it possible that while you're starring, concentrating on the ball that time or at least the ball looks like its slowing down and if so is it just your brain playing tricks on you?

ANSWER:
In fact, the ball is slowing down the whole time it is coming toward you because of air drag. However, I do not believe that you would be able to tell that. What is certainly true is that time does not slow down as you wait for the ball to arrive. I suppose that it is possible that your concentration on catching the ball results in it looking like the ball is slowing down, but that is not really physics.

QUESTION:
hi, me and my friend are having a debate on wind turbines or anything that travels in a circular motion. one of us say that the very tip of the turbine blade is traveling faster than the inner part that is close to the axis. we both agree that it has the same rpm but what part travels at a faster mph?

ANSWER:
The velocity is proportional to the radius of rotation, so a point twice as far from the axis as some other point is traveling twice as fast.

QUESTION:
I need a very brief explanation for Kindergarteners. (I just want to use the right words; they can learn the details of what they mean later.) In an amusement park ride in which long swings are spun around a central pole, why do the swings rise up as the pole spins faster? (I assume it's the same reason that a skirt rises up when the person wearing it twirls.) Is it something to do with centrifugal force? Centripetal force? Nothing I saw on the web about these forces seems to explain why the objects rise, only why they move to the inside or outside of the orbit.

ANSWER:
For Kindergarteners, use of centrifugal force is probably best. I guess the merry-go-rounds we had on playgrounds when I was a kid have been deemed unsafe, but get your students to appreciate that the faster they are spun on something akin to this, the harder it is to hang on. This is because the centrifugal force which tries to push them off gets bigger as the merry-go-round spins faster. Then you can just take a pendulum and demonstrate that the harder you pull, the higher it rises. The little diagram to the right shows the effect of the the centrifugal force F which will lift the pendulum bob higher as it pulls harder.

QUESTION:
I have a question that been thats been unanswered from quite some time now. I even tried asking that to a person in NASA houston and he couldn't even understand. Hope you can help. We know that space dust and debris keep on falling on earth. And I have read that its many tons a day. Considering mass of earth is increasing every day for millions of years now, how does it affect its revolution, speed and axis. Or does it even affect that.

ANSWER:
One estimate is that the earth gains 40,000 metric tons (4x10⁷ kg) per year. So, in a million years that would be a gain of 4x10¹³ kg. The mass of the earth is 6x10²⁴ kg. The moment of inertia will be proportional to the mass times the square of the radius, so, assuming the change in radius is negligible, the fractional change in moment of inertia over a million years would be approximately 4x10¹³/6x10²⁴≈10^-9=10^-7 %. Since angular momentum (moment of inertia times angular velocity) is conserved, this would mean that the angular velocity would decrease by about 10^-7 %, an increase in the length of a day of about (24 hr)(3600 s/hr)x10^-9≈10^-3 seconds in a million years!

QUESTION:
At the LHC protons travel at .999999991 c, or about 3 metres per second slower than the speed of light. I the the proton is also spinning at above 3 metres per second in the direction of travel wouldnt at least part of the proton for a brief period of time exceed the speed of light. I'm guessing the answer is no because all answers involving exceeding the speed of light are no, but i'm having trouble understanding why.

ANSWER:
Many elementary particles, for example the proton in your question, have "spin" which is intrinsic angular momentum. However, you are attempting to model this angular momentum classically, that is by thinking of the proton as a little spinning ball. However, a proton is not a classical object and it is incorrect to model it as such. Any attempt to classically find the speed of the "surface" using reasonable numbers for the radius and mass distribution will result in nonsensical results.

QUESTION:
While looking towards the northwest after dusk on 7-31-14 in Honolulu, we noticed the quarter moon about 45 degrees above ground level. About an hour and a half later, the moon appeared to be about 15 degrees above ground level. Finally, about another half hour later, the moon was no longer visible. I have never seen the moon move so quickly and I'm wondering whether there is a scientific explanation for this?

ANSWER:
I looked up the moonrise and moonset times in Honolulu on this date: 10:02 AM and 10:12 PM. So the time across the sky is about 12 hours. The time it takes to travel 45⁰ would be about (45/180)x12=3 hours. Your times and angles are approximated, so your approximated time, 2 hours, is not surprising. Also, if there was anything abscuring the horizon trees buildings, clouds the time would have been shortened.

QUESTION:
What is the meaning of the moving diagram at the top of your home page? the blue and red balls...

ANSWER:
It supposedly represents a catalytic converter where carbon monoxide, CO hits the metallic crystal into which oxygen has been adsorbed, picks up an oxygen atom, and departs as carbon dioxide, CO₂.

QUESTION:
If I'm not mistaken helium 3 is a very powerful energy source. But there is very little of it on earth. There is lots of it on the moon. so what problems would we run into if we extracted it from the moon why haven't we already done it?

ANSWER:
³He is a potential fuel for a nuclear fusion reactor. The energy producing reaction is ³He+²H >⁴He+¹H+energy; here ²H is heavy hydrogen or deteurium (proton plus neutron plus electron) and ¹H is normal hydrogen (proton plus electron). There isn't necessarily more ³He on the moon, it is just thought to be more abundant relative to the much more abundant ⁴He there. The interesting thing about helium is that it does not form molecules because it is inert; and, because it is so light, if it appears in the atmosphere it moves so fast that it escapes right into space. The best source of helium on earth is to separate it from natural gas with which it has been trapped underground. The reason that we have not already gotten it from the moon is that it is terribly expensive to send spacecraft to the moon and we would also have to develop the technology to extract it from the moon rocks in which it is embedded and transport it back to earth. Even if that were not a problem, the simple fact is that, in spite of decades of trying, we have been unable to build a nuclear fusion reactor which can produce more energy than it consumes and we are still decades away from having a commercial fusion reactor. There is a kind of funny saying about nuclear fusion: "Nuclear fusion is the energy of the future and always will be!"

QUESTION:
I was explaining conservation of energy to my daughter when she was spinning on our office chair, and her rotational velocity increased as her moment of inertia decreased when she pulled her arms and legs toward the rotational axis. Is there an analogous example for linear motion, where the linear velocity increases as the mass decreases? I can't think of a real-world example of a body whose mass decreases (or increases).

ANSWER:
Actually, you were demonstrating conservation of angular momentum, the product of the angular velocity and moment of inertia remains constant for an isolated system. Energy is not conserved because rotational energy is proportional to the product of the square of the angular velocity and moment of inertia. But, you explained it correctly for your example. So, your question is if it is possible for conservation linear momentum, the product of mass times velocity, to result in a changed velocity due to a change of mass. You do not usually see as many examples of this as for angular momentum because rotating things change shape frequently whereas moving objects usually do not have significant change of mass. The classic example is a conveyer belt onto which mass is being dropped from a hopper. If you had a very long frictionless conveyer belt with mass on it, it would have a certain linear momentum. Now, if you start dropping mass on it, it will slow down. Similarly, if you let mass drop off the end of the conveyer belt without replacing it, it will speed up.

QUESTION:
Some time ago I found a quantum lecture series on the web. I thought that even if I didn't "get" all the math, I would still perhaps learn something. Well, the lectures were "here is some math you will need for quantum mechanics," "here is some more math you will need, etc." I skipped ahead many lectures and found the next lecture was "here is some MORE math you need, etc." I am wondering this: Is quantum mechanics something like the Ptolemaic system where the math works even though there is no REAL understanding of the underlying phenomena?

ANSWER:
No question quantum mechanics is quite mathematical as are most advanced topics in physics. It's the nature of the beast, I guess. But it should be possible to convey the essence of just about any topic in physics with minimal mathematics or even none. That, after all, is what I aim for at AskThePhysicsit.com. So, is quantum mechanics comparable as a theory to the Ptolemaic description of the solar system? I would say certainly not. So, originally Ptolemy postulated circular orbits for the planets and satellites which did a fairly adequate job for the 2nd century. But then there were more observations which were not described and one had to add epicycles to the Ptolemaic system, sort of circles on circles. It all became contrived to just fit the data and eventually was given up as a failed idea. Quantum mechanics, on the other hand, matured rather quickly from its sketchy origins (black body radiation, photoelectric effect, Bohr model, Compton scattering) to what today is called nonrelativistic quantum mechanics. If applied to experimental results over a time period of about 80 years, the theory has never failed to be correct. But just because it works wonderfully well does not mean we really understand it. I think most physicists would agree that we do not have an answer as to "why it works" or whether there is an underlying possibly more deterministic physics. Certainly Einstein was never comfortable with quantum mechanics. To compare it to the purely empirical Ptolemaic "theory" really sells it short.

QUESTION:
This might seem dumb, but i cannot understand the laws of thermodynamics applied to gravity. If a moon orbits a planet in a stable orbit, it still pulls on the planet, and the planet pulls on the moon. This seems to generate heat (the heatsource on europa) and motion (tidalwaves on earth). How can this not violate the laws of thermodynamics?

ANSWER:
The first law of thermodynamics, which seems to be the crux of your question, is nothing more than conservation of energy. Energy conservation is true only for isolated systems, so let us think about a single planet and a single moon isolated from everything else. In addition to the simple central force of gravity, the two interact with each other via tidal forces which result from the sizes and relative masses of the two. Just what the effects of the tidal forces are depends a lot on the relative sizes and distances of the two, but, as you note, often appear to violate energy conservation. So, let's look at that more closely. Soon after the moon was created, probably by a cataclysmic collision with an asteroid, it was much closer to the earth than today and it rotated on its axis at a much faster rate than it does today (about 28 days, which is why it always shows the same side to us). The tidal force caused what is called "tidal locking", the same side always facing the earth. It would seem that this would represent a loss of energy since it is not spinning as fast as it used to be, but as this happened, the moon moved farther and farther away from the earth which represents an increase in energy. So, the energy of the whole system stayed the same. Today, the tidal effect is mainly due to the ocean tides on the earth and now it is the earth which slows down its rotation the earth is losing rotational kinetic energy and tending toward being tidally locked with the moon. This is an extremely small effect a day gets about 2 milliseconds shorter during one century. But, just as was the case when the moon was slowing down its rotation, the way energy is being conserved is by the moon moving farther away, resulting in an increase in the earth-moon energy keeping the total energy constant. If the tidal forces are great enough to cause significant frictional heating because of the tidal force distortion of the whole moon (your Europa example), energy is lost because some of the heat is radiated into space. So the first law would be violated, but you would expect that since some energy is escaping from the planet-moon system. You can see that your error was to assume a "stable orbit".

QUESTION:
How long does it take to stop a 7000 lbs vehicle at 45 mph? Time and distance please.

ANSWER:
You have not given me enough information, in particular what are the wheels and the surface made of; surely you realize that a truck stopping on ice will go much farther than a truck stopping on a dry road. I will work it in general and then calculate it for a typical example. The quickest stop you can affect is to apply the brakes hard enough that the wheels are just about to start skidding; that is what anti-skid braking systems do. Therefore the force F which is stopping you (on a level road) is the static friction between the road and the wheels, F=μ_sW where μ_s is the coefficient of static friction between the wheels and the road, W=Mg is the weight of the vehicle, M is the mass, and g=32 ft/s² is the acceleration due to gravity. But, Newton's second law tells us that also F=Ma where a is the acceleration of the vehicle. Therefore, the acceleration is independent of the mass of the vehicle and the acceleration is a=μ_sg. Now that you have the acceleration you can write the equations of motion for position x and velocity v as functions of time t: x=v₀t- at² and v=v₀-at where v₀=45 mph=66 ft/s. Now, you need to specify what μ_s is. For example, μ_s≈0.9 for rubber on dry asphalt, so a≈0.9x32=28.8 ft/s² and I find t≈66/28.8=2.3 s and x=66x2.3- x28.8x2.3²≈76 ft.

FOLLOWUP QUESTION:
I'm trying to figure out if a traffic light at an intersection is timed too short for a heavier vehicle to stop in time. The speed limit is 45mph, the vehicle weighs ~7000 lbs (7200 empty w/driver) , there is a downward slope of which I'm trying to find out. Yes to dry pavement. What would you need to figure surface area of rubber/tire? And I need to time the light still. With all of the above information would you be able to calculate that?

ANSWER:
As I showed above, the weight is irrelevant. Of course, this is an approximation as all friction calculations are, but a quite good one for this situation. It is also important that my calculation is the shortest time and minimum distance, what you would get by flooring the brake pedal with anti-skid braking operating. If you do not have anti-skid braking and you lock your wheels, it will take longer and go farther. Also, there is the possibility that μ_s could be different from 0.9 depending on local conditions (temperature, surface condition, etc.). It is important to include the slope in the calculation. If the slope is down as you say, the acceleration (I am assuming you are not interested in the details) is a=32(μ_scosθ-sinθ) where θ is the angle of the slope; for example, if θ=20⁰ and μ_s=0.9, a=32(0.9x0.94-0.34)=16.2 ft/s², quite a bit smaller than the value of 28.8 ft/s² for a level road. Once you calculate the acceleration, the expressions you can use for time and distance are t=66/a and x=2178/a, respectively. So, for a 20⁰ slope, t=4.1 s and x=134 ft. Again, this is the lower limit. I think an engineer would build in a factor of 2 safety factor. You do not need to know what the surface area of the contact between the rubber and the road is.

QUESTION::
Nothing can accelerate itself by applying force on itself. Besides, isolated forces do not exist and they exist in an action reaction pair. These are the essence of Newton's third law. When an engine exerts force on a bike how can it accelerate the bike taking bike as a system in this argument? After all, a part is exerting force on another part , right?

ANSWER:
The engine exerts a torque on a wheel trying to make it spin. If the bike were on ice, the wheel would spin, there would be no acceleration and therefore there must have been zero net force on the bike+engine as you surmise. However, if there is friction between the wheel and the ground, the ground exerts a force on the wheel which is forward; this is the force which drives the bike forward. Note that the wheel exerts a force backward on the ground (Newton's third law) but the ground does not move because it is, effectively, infinitely massive.

QUESTION:
There is a debate at my office regarding this question If you are on a bus traveling at 20 mph and you run from one end of the bus to the other at 5 mph, how fast are you going? Can you answer and explain?

ANSWER:
I must have answered similar questions a hundred times! Whenever you ask about what a velocity is, you must specify velocity with respect to what. I am assuming you mean velocity relative to the ground. If the bus is going forward and you are running toward the back, your velocity is 15 mph in the same direction the bus is moving; if you are running toward the front, your velocity is 25 mph in the same direction the bus is moving.

QUESTION:
A while back my friend and I were playing computer games my room together. We noticed that it was getting very hot in the room and I said "just think how hot it would be if we didn't have efficient CPU coolers!" He responded, "It would actually be cooler in here if we didn't have efficient coolers because more heat would be in the CPU and less would be in the room." My question is this, would the overall heat in a room occupied by gaming computers be higher with less efficient CPU coolers or would it be higher with more efficient CPU coolers?

ANSWER:
The CPU generates a certain amouint of power in the form of heat. You want to get that away from the CPU so you install some kind of cooler. The simplest would be just fins thermally coupled to the chip to radiate the heat away faster due to increased effective surface area. In the long run, either of these will end up heating the environment at the same rate because both are generating the same power, it is just that the equilibrium temperature of the chip or chip+radiator will be different. The next simplest cooler would be a fan; but a fan itself consumes power and so it adds to the total power dumped into the room. This would come from the heating of the fan motor. Or, you could have some kind of refrigerator; again, this would add more heat to the room than the power generated by the CPU. A refrigerator extracts heat from one place and dumps heat in another. Because of thermodynamics, the heat dumped is always greater than the heat extracted. For example, you should not cool your kitchen by opening the door of your refrigerator because the coils at the back put more heat into the room than the cooler takes out. Finally, if you have some kind of liquid cooling and dump its heat somewhere outside the room, the overall effect would be to heat the room less.

QUESTION:
Does the centrifugal force really exist?

ANSWER:
I guess that depends on what you mean by "exist". In the sense that it is a real force, the answer is no. Newton's laws are not valid if you are accelerating. For example, read an earlier answer on an why you fall over in a bus which is suddenly stopping; this answer will also lead to links on centrifugal force for a bicycle and a car. It turns out that you can make Newton's laws to be valid in accelerating systems if you introduce "fictitious forces" and centrifugal forces are fictitious.

QUESTION:
I'm a non-scientist researching the issue of the relative efficiency of water transportation versus land and air transportation. The studies I consult all indicate that bulk cargo transportation by water is far more efficient than land or air transportation, but they stop short of getting into the physics of why this is so. Something to do with friction, I imagine--but exactly what?

ANSWER:
I am sure there are lots of considerations. Since you mention friction, let's run with that. I will compare ground with water transportation. Imagine a truck or a train, completely empty. For it to drive at a constant speed the engine must provide a certain power, energy delivered per unit time; the reason that it cannot just move forward without an engine is that there is friction of various kinds in the axels, in the rolling of the wheels, and air drag. The drag depends only on your speed, so if we agree to go a certain speed, this friction can be ignored. The rest of the friction is determined by the weight of the train or truck. Now, suppose you load the train or truck with cargo, say 10 times the weight of the empty train or truck. Friction (other than drag) will increase by a factor of 11 because friction is proportional to the total weight. So you need almost 11 times more power to maintain the constant speed. Now think about a ship. All the friction is drag, air drag and water drag. These now depend on speed but there is no friction which depends (directly) on the weight. If you add 10 times the weight as we did before, the ship will go down deeper in the water; this will increase the water drag somewhat, but it will certainly not be 10 times bigger as was the case for ground transport. Roughly speaking, the drag will be proportional to the cross sectional area of the ship in the water and I would be surprised if that doubled when loading a ship.

QUESTION:
My question pertains to sound and how it manages to traverse all sorts of mediums - gas, liquid, solids. Specifically, it is simply amazing to me how well it propagates and we can pick it up with our ears, given that the original source of the sound often has a relatively low energy source. For example: I am lying in my bed at home, have the covers over my ears, my windows are closed, the blinds are down and I can still hear people talk outside on the sidewalk, even though the trajectory of the source (vocal cords, mouth cavity of the speakers) is not linear to my window and bed and ear under the covers. How can the rather minute energy that vocal chords produce manage to traverse such increadible obstacles by making atoms and molecules vibrate in all these different mediums? I just dont get it...

ANSWER:
You are certainly right that sound carries very little energy. We should note that, were it not for the resonant properties of the head, chest, and mouth, the sound made by vibrating vocal chords would be much smaller yet. There are essentially two answers to your question: it takes very little energy to get a molecule vibrating and the ear is a remarkably sensitive sound detector.

QUESTION:
Why don't electrons fall into the nucleus?

ANSWER:
Why don't the planets fall into the sun? Because they are in orbits and the gravity from the sun bends the paths of the planets as it pulls on them and the result is an orbit rather than a fall. Really, a straight fall is just a special kind of orbit; or, put another way, an orbit is a constant free fall. So, think of electrons as like planets and the nucleus as like the sun and the force is electrical rather than gravitational. But there is something different about electric charges. As you can see from the answer two questions below this one, an accelerating charge radiates electromagnetic radiation; so the question is why the electron, which is accelerating in its orbit, does not radiate its energy away and spiral into the nucleus. This is because in quantum mechanics we find that the electron can only exist in certain orbits and a "free fall orbit" is not one of them.

QUESTION:
I am standing in a field. 200 m away someone fires a gun and at the same time in the opposite direction 200 m away someone screams. Would I hear the gun first, scream first, or both at the same time?

ANSWER:
If there is no wind, the sounds would reach you simultaneously. The velocity of sound is independent of the loudness. The speed of sound is about 340 m/s so you would hear each sound 200/340=0.59 s after it was emitted. The reason I stipulate no wind is that the sound travels with respect to the air, not the ground. So, if the wind speed were 100 m/s (over 200 mph, so not likely!) toward you from the direction the train was coming, you would observe the sound from the train approach you with speed 440 m/s and from the girl 240 m/s. You would hear the train at a time 200/440=0.45 s and the girl at a time 200/240=0.83 s.

QUESTION:
How are radio waves created within antennas?

ANSWER:
The way you make radio waves is to accelerate electric charges. So, about the simplest antenna you could make would be a conducting rod. Apply a sinusoidally varying potential difference of frequency f, V=V₀sin(2πft), across the ends of this rod and it will cause the electrons to oscillate with the same frequency such that their positions are of the form x=Asin(2πft), so their accelerations are a=d²x/dt²=-4π²f²Asin(2πft). The result will be electromagnetic waves with frequency f.

QUESTION:
Say I have a syringe fully compressed, then seal the spout end. Now I pull out the plunger creating a vacuum inside. Will the force required to pull the plunger back be the same throughout the length of the pull?

ANSWER:
The force required to hold the plunger at any place is the product of its area times the atmospheric pressure, provided there are no air leaks. This is independent of how far along the length of the syringe you are.

QUESTION:
I looked up how our astronauts breathe on the ISS; splitting water molecules into gaseous hydrogen and oxygen (H2 and O2). So my question is: how much water would natural processes have to had split for there to be as much oxygen that is present in our current atmosphere? I assume that's a tough one to answer without engineers etc. I just wish there was already an answer available.

ANSWER:
This is not particularly "tough" to estimate. The total mass of the atmosphere is about 5x10¹⁸ kg and about 1/5 of that is oxygen; so there is about 10¹⁸ kg of oxygen in the atmosphere. About 90% of the mass of water is oxygen, so the total amount of water you would have to split would be about 0.9x10¹⁸ kg. I believe that the origin of our atmospheric oxygen is from plants (cyanobacteria, to be precise), though, where photosynthesis turns carbon dioxide and water into carbohydrates and oxygen.

QUESTION:
Is there a difference between a proton and photon, besides their difference in charge?

ANSWER:
It is hard to imagine two more different particles:

protons are fermions (spin ), photons are bosons (spin 1)
protons have mass, photons no not
protons are composite particles (quarks and gluons), photons are not
photons are the quanta of the electromagnetic field, protons are baryons
protons have electric charge, photons do not
photons have an "h", protons have an "r"

QUESTION:
Isn't the fact that Andromeda galaxy (being the galaxy supposedly going through a collision with the Milky way in millions of years) defying the dynamics of dark energy? Shouldn't it be moving AWAY?

ANSWER:
The expansion of the universe is the behavior on average, not some kind of absolute rule. Imagine that you drop a stone shouldn't it move away from the earth? Local gravitational fields and initial conditions often trump the overall expansion properties. The Andromeda and Milky Way galaxies are attracted to each other gravitationally and since they never have had a large velocity moving away from each other, they are doomed to fall into each other.

QUESTION:
In the topic of radiation therapy, they say that the photoelectric effect is used. It is said that in radiation therapy "an incoming photon collides with a tightly bound electron." However where does this electron come from?

ANSWER:
Every atom in the material through which the photon is traveling has electrons. They are not added, they are already there.

QUESTION:
When a bullet hits a door and gets embeded in it, no external force acts on the system of door and bullet but why is linear momentum not conserved and the angular momentum conserved? Can you give some examples where angular momentum is conserved but not linear? Can it be possible to have both linear and angular momentum conserved?

ANSWER:
I presume you are alluding to the classic introductory physics problem of a bullet hitting a door mounted on frictionless hinges. Angular momentum is conserved if there are no external torques, and since hinges cannot exert a torque, it is. Linear momentum is conserved if there are no external forces but the hinges exert a force on the door during the collision time and so it is not. If the hinges were not there, the door and the bullet would move forward and rotate about their center of mass conserving both linear and angular momentum.

QUESTION:
What is the change in velocity of the earth's rotation if a person (myself) who weighs 60 kg were to stand on something about a foot tall. Ps this is not a homework question, I'm just a curious teen who's never taken physics. Also it's the middle of the summer for me in New Orleans.

ANSWER:
The glib answer to this question would be, for all intents and purposes, the change in rotation would be zero. It is a good opportunity to talk about the physics involved and to estimate how small small is here. The moment of inertia of the earth is about I_e=8x10³⁷ kg∙m². Your moment of inertia if you are on the earth's surface is about I_y=60x(6.4x10⁶)²=2.5x10¹⁵ kg∙m². The moment of the earth plus you is I=I_e+I_y≈I_e=8x10³⁷. If you increase your distance by the amount 0.3 m, about 1 ft, your moment of inertia increases to I_y+ΔI=60x(6.4x10⁶+0.3)²=I_y(1+4.7x10^-8)²≈2.5x10¹⁵(1+2x4.7x10^-8)=2.5x10¹⁵+ΔI and so ΔI=9.4x10^-8 kg∙m². So you and the earth start with I=8x10³⁷ and end with I+ΔI=8x10³⁷+9.4x10^-8. The operative physical principle here is conservation of angular momentum, the product of moment of inertia and angular frequency ω=2π/T where T is the period, 24 hours: Iω=(I+ΔI)(ω+Δω)=Iω+IΔω+ΔIω+ΔIΔω. Neglecting ΔIΔω, Δω/ω=-ΔI/I=-1.2x10^-45; note that since Δω/ω<0, the frequency decreases, the rotation slows down. Now, it is pretty easy to show that ΔT/T≈-Δω/ω=1.2x10^-45or the day gets longer by 24x1.2x10^-45=2.8x10^-44 hours! Your contribution to the earth's moment of inertia is so tiny that anything you do to change your own moment of inertia will have no measurable effect on the rotation of the earth.

QUESTION:
I know that a subatomic particle can be in two places at once so if you took these particles and set them side by side ( before they managed to disappear) would they have the same mass as just one or one squared or one times two?

ANSWER:
In quantum mechanics we do not talk about the position of a particle, we talk about the probability of finding the particle at some position. Thus, there might be two places where the probability of finding a particle is the same, and that is where the popular notion of a particle being "in two places at once" comes from. However, once you do a measurement which locates the particle, it is all there and somewhere else where it might have been before you observed it no longer has any probability of the particle being there. The measurement is sometimes referred to as collapsing the wave function.

QUESTION:
If I lift a book for thirty minutes I feel tired. Physics says that I did no work but it also says that if energy is lost some amount of work has to be done (though it may result in net zero work). Why do we feel tired without performing "work in the language of physics"

ANSWER:
In the example of simply holding up a weight in an outstretched hand, physics would say no work is being done because the force on the weight is not acting over a distance; you and I both know, however, that sugar is being burned to provide the energy necessary to hold this weight stationary. What is going on there, as I understand it, is that the muscle fibers in your arm are continually slipping and retensioning thereby doing lots of little parcels of work to hold your arm steady.

QUESTION:
My friend believes there is the energy potential in a small rock to run a city for days. Is this theory correct?

ANSWER:
If you could find a way to change all the mass of that stone into energy you could get an amazing amount of energy. For example, suppose the mass were 100 grams=0.1 kg. Then the energy there is mc²=0.1x(3x10⁸)² which is about 10¹⁶ J. Suppose you spread this out for a week; then the power would be (10¹⁶Joules)/[7 day(24 hour/day)(3600 second/hour)]=16.5x10⁹Watts=16.5 GW. This is about twice a large as the largest nuclear power plant in the world. Unfortunately, the most efficient way to generate large amounts of power (nuclear fusion, the energy of the sun and the hydrogen bomb) is only 1% efficient.

QUESTION::
If two magnets are in zero gravity would one magnet repel faster than the other or would they equal the same force? Also if u connect somehow an electromagnet and normal magnet in zero gravity when electoral is turned on would the magnet wit the greater force be able to pull/push the other magnet with it if they connected? What speed would magnet repel magnet at zero gravity? Thank you. If u could control the amount f magnetism would there b a limit on speed at zero gravity?

ANSWER:
You have several misconceptions here. First of all, the fundamental physics is independent of whether you are in zero gravity or not. Just to keep things simple, let's say there is no gravity. If you have two magnet aligned such that like poles are facing each other, repulsion will result as you suggest. The first thing you need to understand is that, even if one is very strong and one is very weak, the force that one exerts on the other is exactly the same as the other exerts on the one; this is Newton's third law. Now, just because you know the force, you cannot automatically find the velocity of either magnet, you need more information; for more information on this, see my FAQ page. What will happen is that the forces (F) will cause each magnet to have an acceleration (a) away from the other, but the more mass (m) the magnet has, the smaller the acceleration will be, a=F/m; this is Newton's second law. It is complicated to calculate how much speed each would acquire, impossible if you do not quantify the strengths and the distance apart. The simplest case would be a very light magnet near a very heavy magnet; if you knew the amount of energy it took the bring the light magnet in from very far away, call it W, the the speed v it would have long after you let it go could be calculated from the equation W= mv² or v=√(2W/m)

QUESTION:
Is the energy needed to increase the temperature by 5 degrees, for example, the same energy needed to decrease the temperature of the same matter by 5 degrees?

ANSWER:
I think you mean "the same energy released to decrease the temperature"; you do not add energy to decrease temperature. The answer to your question is that it depends on how you heat and cool. An example of where it would not be the same is if you heat a gas allowing it to expand at a constant pressure and then cool it keeping its volume constant, the energy you put in would be more than the energy you got out.

QUESTION:
My question is, (I was wondering about this yesterday,) suppose you have a doughnut shaped metal pipe. Within that metal pipe you placed a sphere shaped magnet, being just big enough so as to not touch the inside walls of the pipe. Now inside the doughnut shaped pipe is vacuum, at the same time since the force of gravity is there. What would the velocity be of the sphere shaped magnet inside? Would it increase, decrease gradually with time or stay constant?

ANSWER:
This is just a variation of the classic problem of dropping a magnet down a conducting pipe. If you put inside at rest it will stay at rest. If you give it a velocity, currents induced in the pipe will exert a force on it which causes it to stop.

QUESTION:
When calculating the frequency of a radiowave, the formula to use is: frequency = c / wavelength. However, if the wavelength is 12mm, and we assume c to be rounded up to 3 x 10^8, when I get the answer 25,000,000, how do I know if this is in hertz or kilohertz? I've used online calculators to check the answer and it corresponds to 25,000,000 kilohertz but I want to know why was the figure 25,000,000 in kilohertz and not hertz.

ANSWER:
You can understand what is going on by showing the units in your calculation. f=c/λ=(3x10⁸ m/s)/(12 mm)=0.25x10⁸ m/(mm∙s). If you had used consistent length units, not mixed millimeters and meters, the length units would have cancelled out. For example, writing λ=12x10^-3 m, f=c/λ=(3x10⁸ m/s)/(12x10^-3 m)=0.25x10¹¹ s^-1=0.25x10¹¹ Hz=0.25x100⁸ kHz. Scientists usually try to get everything into the same system of units before doing any arithmetic.

QUESTION:
If an astronaut caught a ball in space, the ball would cause the astronaut to move backwards with the force the ball was moving at correct? then, as the astronaut is still moving backwards and throws the ball back where it came from, would the astronaut move even faster with the force of throwing the ball? or would their speed remain the same?

ANSWER:
When she catches the ball, she exerts a force on it to stop it (relative to her); the ball exerts an equal and opposite force on her causing her (and the caught ball) to move in the direction the ball was originally moving. When she throws it back, she must exert a force on it opposite the direction she is moving; the ball exerts an equal and opposite force on her causing her to move even faster in the direction she was moving. These are examples of Newton's third law.

QUESTION:
If one were in a deep hole 100 miles from the center of the earth, would gravity at that point be less than at the surface of the earth?

ANSWER:
Yes. Here is the reasoning: if you have a spherically symmetric mass distribution, meaning that the density does not depend on longitude and latitude, the only mass which contributes to the gravitational force if you are inside the sphere is the mass inside the sphere where you are. So, if you are at the center of the sphere, there is zero gravitational force. An interesting historical note is that this problem was one of the more important reasons Newton had to invent calculus. He had to show that a spherically symmetric mass distribution had the same gravitational field outside as if the mass (a planet, for example) were a point mass at its center. I have read that struggling with this problem caused the publication of his gravitational law to be delayed by 20 years. (I do not really know if that is true or not.)

If, in addition, the mass distribution is uniform, the density does not depend on how far you are from the center, you can easily calculate how the gravitational force varies inside. Outside the force on a mass m due to the earth with mass M is GmM/r² where r is how far you are from the center. Inside, the mass below you can be written M'=M(4πr³/3)/(4πR³/3)=M(r³/R³) where R is the radius of the earth. So the force inside is GmM'/r²=GmMr/R³ which decreases linearly as you go in.

QUESTION:
I read that the average person can tolerate about 5 g-force before they black out. Hypothetical: if every atom of air around you (and those of the Earth below) emitted 5 times their normal gravity, would this be harmful? or because it's pulling from all directions would the forces kind of cancel out? ANSWER:
Essentially, you are asking what if the earth had the same size and five times the mass; also the air were made of molecules five times more massive than normal atmosphere. If your weight now is 200 lb, your new weight would be 1000 lb and your legs would likely not be able to hold you up. The pressure of the air around you would be greatly increased and that pressure would likely be what would kill you, not the weight of your body. (Since gravity is 5 times stronger and the mass of air molecules is five times greater, I am guessing that the atmospheric pressure would be about 25 times greater.)

QUESTION:
What is mass? ANSWER:
There two kinds of mass. Inertial mass is the property an object has which resists acceleration when a force is applied; the harder it is to accelerate something, the more inertial mass it has. Gravitational mass is the property an object has which allows it to feel and create gravitational forces; for example, the more gravitational mass an object has the greater the force it will feel due to the earth's gravity the more it will weigh. It turns out that the two masses are actually identical; this fact is one of the cornerstones of the theory of general relativity.

QUESTION:
What is the formula to calculate the total energy of an object that is moving at relativistic speeds? (Ignoring any potential energy) ANSWER:
E=√(m²c⁴+p²c²) where p=mv/√(1-v²/c²) is the linear momentum, m is the rest mass, v is the speed, and c is the speed of light. This can also be written as E=mc²+K where K is the kinetic energy (which is not mv²). Comparing these two expressions and doing some algebra, you could find an expression for K. Also, it may be written E=mc²/√(1-v²/c²).

QUESTION:
As I understand one particle and an antiparticle would, if they come in contact completely, annihilate each other and turn in to energy equaling the mass of both of the particles. But if a particle is moving at close to 1c relative to the observer and the other particle was not moving, the speeding particle would have a relativistic mass. In other words the mass of both particles were not balanced. Would the larger mass of one particle result in more energy, or creation of some other particles? Or would the extra mass from the speeding particle simply transform to normal kinetic energy? ANSWER:
The questioner used hydrogen-antihydrogen as an example; I have edited this out since those are composite particles and just muddy the water when trying to discuss what could happen. The most common example is the electron-positron pair. If they interact at low speeds the only thing which is possible is the creation of two (or more) photons because there is not enough energy available to create more massive particles. If one (or both) of the pair have significant kinetic energy when they collide, other particles may be created as the questioner suggests. There are selection rules which restrict which kinds of particles may be created, but if there is sufficient energy just about anything allowed could happen the constraint is that energy must be conserved. In this kind of collision, though, you must also conserve momentum. For example, suppose that you wanted to collide very high-energy positrons with electrons at rest and create a pair of particles with total rest mass M+2m_ewhere m_e is the electron (or positron) rest mass. Your first thought might be that the kinetic energy of the positrons would need to be Mc². But this would not work because the particles would have to be at rest which would violate momentum conservation; so the energy would have to be considerably larger. Proton antiproton interactions are considerably more complicated because they have sufficient rest mass energies to create massive particles even at rest and because they, unlike electrons and positrons, are composite particles made up of quarks. They usually annihilate into mesons which, being unstable, decay ultimately to some combination of gamma rays, electrons, positrons, and neutrinos.

QUESTION:
when a drop of water is dropped on to a hot iron plate why does it become spherical before evaporating? ANSWER:
Any place where the water touches the plate the water will almost instantly vaporize. But the water must touch the plate for the weight of the drop to be held up. The result of these two competing things is that the water touches the plate only in a very small area. It is not spherical, but close enough to look that way.

QUESTION:
Is it mere observation that light has the same speed in every frame of reference? Or, is there any deeper reason? ANSWER:
Very deep! Read the FAQ page.

QUESTION:
Outside the nucleus, free neutrons are unstable and have a mean lifetime of 881.5 1.5 s. What is it in the nucleus that keeps the neutrons stable? ANSWER:
Although somewhat simplistic, you might think of neutrons as stuff inside a nucleus which keeps the protons from getting too close to each other so the Coulomb repulsion will not blow it apart. There is no stable nucleus (apart from ¹H) composed of only protons. On its own, a neutron will beta-decay; quite simply, this is determined by energetics energy is released if a neutron decays into a proton, a neutrino, and an electron. To find out what happens inside a nucleus, you again look at energetics; if a neutron inside a stable nucleus were to decay, energy would have to be added, so it does not happen.

QUESTION:
My question is for a legal nature. I was recently in a head on collision. The crime scene analysis could not determine the speed of my vehicle but police reports indicated the other vehicle was doing 45+- mph. We were on a dirt road on impact. The vehicle going 45+- continued to travel 20 ft past impact pushing my vehicle back 40 ft. His vehicle is a 1 1/2 ton dodge ram and my vehicle was a 1 3/4 ton dodge caravan. What speed was my vehicle? This will be instrumental in a lawsuit currently being filed. Your help would be greatly appreciated.

ANSWER:
I am afraid that the speed cannot be determined from this information for several reasons:

I do not know how much energy was lost in the collision.
I do not know whether the wheels were locked (brakes applied) or not.
I do not know the frictional forces which ultimately brought the vehicles to rest.
I believe the weight of the Dodge Ram is wrong. The Dodge Ram 1500 is referred to as 1 tons because this is the maximum recommended load. An unladen Ram 1500 weighs more than 6000 lb; I found that the weight of the Caravan is about 1.75 tons, though.

QUESTION:
If a capacitor stores large amount of charge then can it be considered as a battery?? ANSWER:
In the sense that it can provide a steady potential difference, yes. In the sense that it can provide a steady electric current, no. As soon as you begin trying to take energy from the capacitor, its potential difference begins to fall.

QUESTION:
Friction opposes the relative motion between two surfaces. when a car travels on a circular path , how can the friction act sideways to provide necessary centripetal force. The friction should act backward relative to the motion of the car. the car doesn't tend to go sideways outward, then, how does friction act sideways?

ANSWER:
There are two important classes of friction, kinetic friction which occurs when two surfaces are sliding on each other and static friction when they are not sliding. Kinetic friction is the one which usually (but not always) acts opposite the direction of motion; an example of kinetic friction acting in the direction of motion is a car which is accelerating from rest and spinning its wheels the friction force on the spinning wheels by the road is forward. Static friction can point in any direction, depending on the situation. If a box is sitting at rest on an incline, the frictional force points up the incline to keep it from sliding down. If a car is moving but not skidding, the appropriate friction to think about is the static friction between the wheels and the road. Think about a very icy road; to drive around a curve at high speed is impossible because there is no static friction and the car simply continues going straight regardless of whether you turn the steering wheel or not.

QUESTION:
Gravitational potential energy is the term that means the work done by the gravitational force to take an object to the gravitational field. Here the displacement is towards the force.So,it (Gravitational potential energy) should be positive.But it is negative.Why?

ANSWER:
You need to be a little more careful in how you define potential energy. And, what is actually defined is the potential energy difference between two points in space. The definition is ΔU=U(r')-U(r)=-_r∫^r'F∙dr, where F is the force of gravity on m due to the presence of M. Now, if I choose increasing r to be in the upward direction, F∙dr=-(MmG/r²)dr. So, -_r∫^r'F∙dr.=-_r∫^r'[-(MmG/r²)]dr=-MmG[(1/r')-(1/r)]=U(r')-U(r). This is completely general. It is customary to choose U(∞)=0, so if r'=∞, U(r)=-MmG/r. (You have to be very careful of all these minus signs!) So, you see that the potential energy is determined by where you choose it to be zero and the choice of coordinate system; if we had chosen r to increase in the downward direction and U=0 infinitely far away (at r'=-∞), U would have been everywhere positive.

QUESTION:
Could fusion energy be powered by other hydrogen isotopes or other light elements?

ANSWER:
Yes, fusion produces energy up until you get around iron as the final fusion product. Thereafter it costs energy to fuse nuclei. However, the general trend as you go to heavier nuclei is that the fractional energy output compared to mass gets smaller. Another problem if you are thinking of a reactor is that heavier nuclei have higher electric charge and are therefore harder to bring close enough together to fuse.

QUESTION:
I read a fact that a compressed spring weighs more than a relaxed spring...why is it so??

ANSWER:
It is true but unmeasurable. Suppose the spring constant is k=2,000 N/m and you compress it by x=1 cm=0.01 m. The work you did is E= kx²=0.1 J. This energy will end up as added mass m=E/c²=10^-18 kg.

QUESTION:
I was reading a novel the other day which takes a surprisingly realistic approach to theoretical space-combat (namely ships attacking each other with missiles from many millions of kilometers away), albeit with missiles moving at significant fractions of the speed of light (around 30-40%). This made me wonder, say two space ships are fighting and one fires a missile traveling we'll say 50% the speed of light. I'm having real trouble understanding the effect time-dilation would play (and the author completely avoided the subject). I tried reading some of your past answers on time-dilation, but I just ended up confusing myself. My thought is that the missile which is traveling at the significant fraction of c (while the ship is not) would have a huge advantage. With time passing slower the missile would have the net effect of having more time available to itself; to which it could use to account for the ship's attempts to evade, to itself evade any counter-measures the ship might employ, all in addition to being difficult to detect and track due to its speed. Would the theoretical missile in this situation have those benefits, and the target ship be suffering a serious disadvantage; or does time-dilation not work that way?

ANSWER:
Time dilation really does not come into play here if I understand things correctly. If you are thinking that the missle is robotic and able to adjust to evasive movement of the target, there is no advantage because the missle sees the distance to the target shrink by the same factor that its clock slows down. As seen from the stationary fleets, there is just a projectile with half the speed of light. Since the speed of light is 300,000 km/s, the attacked fleet will observe the missle coming with a speed of 150,000 km/s and many million kilometers, let's say 150 million for illustration, means that it will take 1000 s to get to the target as measured by the fleets. The target fleet will not know it has been attacked until 500 s before the missle arrives, but that still gives time for evasive action. The main problem is that because of the high speed, evasive action becomes increasingly difficult as the missle gets close but this has nothing to do with time dilation. The advantage to the missle is due to its high speed and the limited reaction time of the target.

QUESTION:
ok, two stones. Both spherical and same mass and density evenly spread in each stone. Set each about 1/2 the distance to the moon. One leading the earth's orbit and one following the earth's orbit. Both not moving relative to the earth, yet the same speed as the earth as it moves around the sun. No tangent or orbital speed, the stones are starting in freefall. Which gets to earth's surface first, neglecting air drag.

ANSWER:
Since you stipulate that the stones are not orbiting, the stones are at rest with respect to the sun and the earth is not. Therefore, the stone on the leading side of the orbiting earth will win the race because the earth is moving toward it and away from the other when the stones start dropping.

BETTER ANSWER:
I see that I misread this question. I guess you meant there is no orbital speed around the earth. To make this manageable at all I will neglect the influence of the moon and assume a spherically-symmetric mass distribution of the earth. In the figure above, I show your two stones and the forces (blue arrows) on them. The down-pointing forces are the from the sun (keeping them in orbit) and the horizontal forces are the weights making them want to fall toward the earth. The distances and forces are not drawn to scale; when the stones are about 30 earth radii away from the earth center (the moon is about 60 earth radii away), the weight forces are about 2 times larger than the sun forces. The big blue arrow shows the direction everyone is orbiting the sun. So, the leading stone slows down its orbital speed and so will slightly fall toward the sun as it falls toward the earth; the trailing stone increases its orbital speed and so will slightly fall away from the sun as it falls toward earth. These deflections are shown (probably quite exaggerated) by the red arrows. Given the symmetry of the situation, I would expect the two to be at the same distance from the center of the earth at any given time which is the crux of your question, I think. To actually do this more quantitatively, though, would be very hard because as the stones were deflected the weight force would change direction now having a vertical component in the figure.

QUESTION:
Physics is my hobby, but, language is my education (MA). I realized a while ago that there is no definition for motion beyond "when something goes from point a to point b". There are archives of "descriptions" of motion (e.g. Newton), but no "definition". After 3 years, I have found a definition! So, what do I do? Journal article? Copyright? Show up at some place and submit my idea? Please assume that what I am saying is true.

ANSWER:
The reason that you cannot find an operational definition is that there is none. There are generally two types of concepts in science, quantitative and qualitative. If you are a "language guy" you must understand that. Qualitative concepts are those which we understand in terms of language, they are not quantitatively defined. Everyone understands that motion is when something moves to some other location in some time. Asking what the definition of motion is is like asking what the definition of wet or large or speedy is there is none. For motion, though, there is an archaic definition. When Newton stated his second law he wrote it as The alteration of motion is ever proportional to the motive force impress d". Today "alteration" is stated as "rate of change", "motion" is "linear momentum" which is mass times velocity, and 'motive force" is simply "force", so rate of change of momentum (d(mv)/dt) is proportional to force. So, you might satisfy yourself that motion is mv where m is operationally defined (kilogram, e.g.); v is rate of change of position, a length divided by a time and both length (meter, e.g.) and time (second, e.g.) are operationally defined. Again I emphasize that this is archaic and motion today is qualitative.

QUESTION:
I have a 10' long trailer I am using to haul a 4,000# symetrical object that is 6 ft in length. I was told to move it forward of the axle on the trailer to put some weight on the tongue for safety and better hauling. The maximum wt my pick up truck can hold on its hitch is 650#. I have tried to find equations for this on-line as it would be very useful for me to know how to adjust other loads as well. There are plenty of equations on-line that deal with finding the CG, but I can't find one that discusses how the weight on the tongue changes as the load is moved fore or aft of the axle. Trailer/towing experts just wing it. Do you know of an equation that would help me?

ANSWER:
I will assume that the unladen trailer will have approximately zero force on the hitch (which would mean that its center of gravity (COG) is at the axle). You need to know where the COG of the load is; in your specific case, you know that it is at the geometrical center (you said it is symmetrical), 3' from either end. For loads not symmetrical, you need to find it. I will call the distance between the axle and the hitch L. Suppose that the COG is a distance x from the hitch. Then, the sum of the torques about the axle must be zero, so Hx=W(L-x) where H is the force (up) by the hitch and W is the weight (down) of the load. Solving this, x=[W/(H+W)])L. For your case, with H being 650 lb (although I cannot see why the maximum would be the optimal) x=(4000/4650)L=0.86L. If your trailer has more than one axle or its COG is not over the axel, I would need more information like the geometry and weight of the trailer.

QUESTION:
When I have a rope attached to a vertical wall and a climber using the rope to climb perpendicular to the vertical wall,as the climber goes up the rope the tension in the rope increases, Why?

ANSWER:
To move upward you must pull down on the rope and that pull increases the tension.

QUESTION:
What effect (if any) would there be on the solar system if the sun were to lose the mass of Jupiter? This question is based on various schemes to power a "warp drive" by converting mass about the size of the planet Jupiter into pure energy. Since vaporizing Jupiter is probably not a good idea, it might make more sense to get the necessary mass by skimming off a chunk of the sun's surface. Assuming mankind might have the technology to do this someday, would the sun be appreciably different if it lost the mass of Jupiter? Would the orbits of the planets change to a significant degree?

ANSWER:
I usually do not answer astronomy questions, but will take a stab at this one since I have earlier talked about effects which Jupiter has on other objects in the solar system. If Jupiter disappeared it would have a negligible effect on other planets. It would, though, have a large impact on many asteroids whose orbits are controlled by Jupiter's gravity (see that earlier answer). This would release a swarm of asteroids into earth-crossing orbits considerably increasing the likelihood of catastrophic collisions with earth. The mass of Jupiter is only 0.1% that of the sun, so taking that much mass from the sun would probably cause fairly minor effects on solar system orbits. "Skimming" that much mass from the sun could have pretty serious short-term effects on the sun's radiation. Oh, by the way, there is no such thing as warp drive! (PS your email server rejected my mail as spam.)

QUESTION:
I've been arguing with a friend about this, and I'm sure you've answered it before, but I spent about 30 minutes searching the web and I couldn't find anything! So to the question, if one were to make a "phone" out of a paper/styrofoam/tin cup connected to a string, would it work in space? I believe it would because the strong would act as a medium.

ANSWER:
Yes and no. If you tapped on one cup that vibration would be transmitted via the string to the other cup. But if what you had in mind is speaking into one end and someone hearing on the other end, it would not work because your voice is sound in the air which gets the cup vibrating and there is no air.

QUESTION:
A 5.0 Mev electron makes a head-on elastic collision with a proton initially at rest. Show that: a) The proton recoils with a speed approximately equal to (2E_e/E_p)c and b) the fractional energy transferred from the electron to the proton is (2E_e/E_p), where E_e is the total incident energy of the electron and E_p is the rest energy of the proton.

ANSWER:
I have verified that this is not a homework problem. The solution may be seen here.

QUESTION:
The definition of "electric current" I find in my school books is: "directed flow of electrons". The power stations here in my country use hydro power to make work some huge generators which create electricity, i.e directed flow of electrons. But their functioning is not based in obtaining electrons from something (not the water nor the metal), instead its function is to create some magnetic fields which seem to be essential in creating electric current. My question: Is the above definition of electric current correct? If yes, where do the continuous flow of electrons come from? Are really magnetic fields a limitless source of electrons, if not, how do they generate limitless electricity?

ANSWER:
The electrons which flow in a wire were already there before the current started. In materials which are conductors there are electrons which are very easy to move around. Magnetic or electric fields may be used to cause these "conduction electrons" to move. Electrons are not being injected into the wire.

QUESTION:
Here's a question that I have been pondering. If a truck is driving on the freeway and a car pulls in closely behind the moving truck to take advantage of the draft created by the truck is there an energy cost to the truck or is having a car in it's wake energy neutral? My gut feeling is that there would be a slight energy cost to the truck due to it's turbulence wake being interfered with.

ANSWER:
This, I discovered, is not a trivial question. For a lengthy discussion, see The Naked Scientists. Here is my take on it. There is no question that the trailing car consumes less gas. The reason for this is not so much that the truck is pulling the car but that the car experiences a much lower air drag when drafting; the drag is approximately proportional to the square of the velocity and the truck's wake is moving forward with the truck. What seems to be controversial is the crux of your question—is there a cost to the truck? Some argue that the composite truck-car system has less total air drag, others that there is a net cost to the truck which need not (and almost certainly will not) equal the gain by the car. There is certainly no conservation principle here because the new system has different forces on it than the separate systems. My feeling is that there is at least a small cost to the truck and I base this on an observation from nature. Why do geese fly in a V? There is less overall air drag than if the flock all flew individually. But periodically, the leader drops back and another goose takes a turn at the front; must be because the leader has to do more work.

QUESTION:
A friend asked me this and we disagreed with the answer. If we put 25 kg of weight on top of 25 kg person, how much force would he feel?

ANSWER:
Technically, a kilogram is not a weight but a mass. But, since so many countries use it as a weight, I will do that for this problem. The person feels the downward force of her own weight, 25 kg; the downward force of the object pushing down on her, 25 kg; and the upward force of the floor pushing up on her, 50 kg. The net force is zero because she is in equilibrium.

QUESTION:
Is there a speed or time measurement for how long it takes the nuclei of Uranium or Plutonium to transfer from Matter to Energy in nuclear fission after the neutron has split the atom?

ANSWER:
I found a paper which lists the "prompt energy release time" as in the range of 10^-20-10^-7 s. This is the time between the scission and the end of prompt gamma rays and neutrons. I presume this huge range is because of dependence on the fissioning nucleus and the fission products.

QUESTION:
If an object approached a plane mirror at 3/4 the speed of light, would it be approaching its image at 1.5 times the speed of light?

ANSWER:
No, because the image does not exist. Light leaves you and travels to the mirror at the speed of light and then the reflected light comes back to you at the speed of light. The light you emit and the light you receive would both be doppler shifted toward the blue so what you saw would not be true color.

QUESTION:
A parallel plate capacitor has a small gap between its two plates , then how it completes the circuit when it is connected by a battery?

ANSWER:
Current flows until the capacitor is fully charged and then it stops. So, except for a short time when you first connect to the battery, it acts like an open circuit.

QUESTION:
Has any human been more then 1,000,000,000 miles in space and returned?

ANSWER:
The farthest any human has been is the moon which is about 240,000 miles from earth.

QUESTION:
I'm in a space ship moving at the speed of light. I put my space suit on, go out on the deck of the ship and tee up a golf ball. Assuming I have the skill necessary to hit the ball, will I be able to hit the ball forward? It seems to me that in the vacuum of space, the energy required to hit the ball forward would not change with my vehicle's speed, but it's also my understanding that the speed of light is an absolute maximum speed. One of these is apparently wrong. I think you are going to tell me I can't hit the ball forward. Can you explain why?

ANSWER:
As I have said a thousand times, you cannot move at the speed of light. See the faq page if you have a problem with this. However, we can address your question by saying your ship has a speed 99.999% the speed of light u=0.99999c. Suppose that you can launch a golf ball at a speed of 10% the speed of light, v=0.1c. (Of course, it is impossible to make it go that fast because you are not strong enough, but it makes this example easier to quantify.) If you were able to do this, there would be absolutely no reason why you could not do it on the moving ship: you would simply see the ball move away from you with speed of 0.1c just as if you were here on earth. Now, classical physics (and your intuition) would have the speed seen by someone watching this to be V=(0.99999+0.1)c=1.09999c, almost 10% faster than the speed of light. However, classical physics does not work here; again, see the faq page. Instead, V=(0.99999+0.1)c/(1+0.99999x0.1)=0.99999182c.

QUESTION:
Is there a magnetic field or not? If there is a charged particle with no velocity relative to viewer A there is no current and no resultant magnetic field, right? But if the same particle is viewed by another observer moving at some velocity relative to it then it could be said the particle is moving at -v and should show both a current and magnetic field. How can you reconcile this?

ANSWER:
Let me pose an analogous question. Is there a velocity or not? If there is a particle with no velocity relative to viewer A there is no velocity, right? But if the same particle is viewed by another observer moving with some velocity relative to it, then it could be said that the particle is moving with some velocity. How can you reconcile this? Like most observables in nature, magnetic fields depend on the frame from which they are observed and, in some special cases like your example, you can actually find a frame in which the magnetic field is zero. In your example, if the observer moves with speed 100v, the magnetic field will be much stronger than if he moved with speed v. Electric fields also behave this way and the electric field observed by the moving observer in your example will differ from the field seen in the frame where the charge is at rest. Actually, there is only one field, the electromagnetic field; the idea of separate electric and magnetic fields is a historical artifact. You might be interested in a similar question about electromagnetic radiation fields.

QUESTION:
My brother and I recently had a lively debate about the mechanics of temperature change within a glass of ice water (i.e. tap water sharing a glass with ice cubes). My brother remembers a Physics professor saying that the temperature of the (liquid) water within the glass won't change until the ice completely melts. Contrarily, I believe the temperature of the water within the glass begins changing immediately (after the liquid water has settled in the glass with the ice). Can you enlighten one (or both!) of us? '

ANSWER:
Imagine doing the experiment in a well-insulated cup; a styrofoam coffee cup would be a fair approximation. I just want to not worry about the interaction with the rest of the environment. The tap water is certainly warmer than the ice. What we know for sure is that if two objects are in contact with each other, heat flows from the hotter to the colder. So, heat flows from the water (cooling it) into the ice. This energy absorbed by the ice is used to melt some of the ice meaning that some water at 0⁰C mixes with the already-present water making it cooler yet. This continues until all the ice is melted (or all the ice and water is at 0⁰C) and the whole system is now at a considerably lower temperature than when you started. But, if you have ever made and drunk a glass of ice water it should be obvious that your brother is wrong the water is much colder than tap water shortly after the ice has been added and long before all the ice has fully melted. What does not change is the total energy inside the cup.

QUESTION:
I'm having a little debate right now that centers around my stance that materials will have a large role on the crash results of an auto accident vs my opposition that says that materials do not matter and that a moving object will take more damage b/c its hitting a non-moving object. Situation: A 3,900 pound 1983 steel mercedes benz going 30 mph were to impact a primary plastic SUV that weights 4,100 pounds and its going 10 miles per hour perpendicular to the Mercedes (it ran a red light) after we both slammed on our brakes. So what happens when 2 object hit. Do you add up the net speed of 30 plus 10 to say that both get hit at 40 miles per hour?

ANSWER:
There is really not enough information to say definitively. Also, your preliminary remarks about materials and non-moving do not really address the question you pose afterward ("what happens?") Suppose the two stick together (called a perfectly inelastic collision). Then you need to conserve momentum, mass times velocity. Before the collision the Mercedes has 3900x30=117,000 units of momentum say north; the SUV has 4100x10=41,000 units of momentum say east. The net momentum after the collision is √(117000²+41000²)=124,000=(3900+4100)V=8000V, so the speed V is about 124000/8000=15.5 mph. The direction of the velocity afterwards is mainly northward with a small eastward component. Brakes were probably applied too late to change any speed by much, would hasten the stopping of the collided cars. Certainly the composition of the cars will affect the damage each suffers.

QUESTION:
Does the velocity and direction of wind make any difference to sound wave ? and if it does , how much?

ANSWER:
Suppose you have a source of sound which emits sound waves with a velocity V_sound in still air. Now suppose that there is a wind with velocity V_wind. The new velocity of the sound (relative to the ground) is V=V_sound+V_wind. Be sure to note that this is a vector equation.

QUESTION:
What is a vernier calliper? ANSWER:
An instrument to measure lengths. See the figure to the right.

QUESTION:
The less density an object has the more it rises in the atmosphere but i was wondering why doesnt it rise at all if it has zero density . I mean zero kg per m³ ..which means a vaccum or a vaccum chamber.

ANSWER:
It is the overall average density which matters, not the density of just what is inside a container. If the weight of the whole vacuum chamber is less than the weight of an equal volume of air it will rise.

QUESTION:
I want to ask that scalar quantities can also be negative i.e. time is negative if it is before origin and positive after origin . Then distance should also follow the same rule. now suppose in case of straight line motion a body covers first +5 units and then-5 units. Then whether the distance will be 10 or 0. I know that it should be 10 but according to the first statement it should be 0. if it is 0 then what is the distancebetween distance and displacement ANSWER:
Certainly, some scalars can be negative. For example, as you note, time before t=0 is negative. Or, temperature on the Celsius scale below zero is negative. However, the magnitude of a vector is positive definite. Your example of a distance being negative is not really correct. In one dimension the distance is the magnitude of the displacement vector and the direction of the vector is determined by the sign.

QUESTION:
"Tritium is produced in nuclear reactors by neutron activation of lithium-6" my question would be; If lithium-7 (the product of the neutron capture) is a stable isotope why does it split? And is this splitting α decay, or fission, that just happens to result in an alpha particle & a tritium nucleus?

ANSWER:
Because the ⁷Li does not get formed in its ground state, rather it is highly excited. Or, you might want to look at it as a nuclear reaction since it happens very quickly:⁶Li+n->⁴He+³H. It is not surprising that this reaction goes because the alpha particle is extraordinarily tightly bound; that is one of the reasons heavy nuclei often undergo alpha decay because it is such a tightly bound nucleus, there is a reasonable probability that it will spontaneously form inside a nucleus.

QUESTION:
If you have a boat floating in a bowl of water and you increase gravity , does it float even more or does in stay at the same place ?

ANSWER:
If you increase g, the buoyant force, the weight of the displaced water will increase by the same amount. But, the weight of the boat will increase by the same amount and float the same.

QUESTION:
Given that a proton has a magnetic dipole moment, do researchers currently use magnetic field to rotate them in addition to accelerating them to further increase the energy of their collisions?

ANSWER:
A magnetic field exerts a torque on a dipole, but no force. So you cannot use a magnetic field to accelerate them.

QUESTION:
If an ultra high energy cosmic ray with energy of 10²⁰ eV were to strike an astronaut will that kill an astronaut?

ANSWER:
This is only about 6 J of energy. That is the energy needed to lift 1 kg about 60 cm. And, it would probably not leave all its energy in the astronaut. Certainly would not kill her.

QUESTION:
if you have 3 flywheels of the same material, with the same dimensions, and attached to similar engines. The wheels all have equal amounts of weight... one with the weights in the center, one with the weight at the outside of the wheel, and the third one with the weight between the center and the outside. If all three engines are running for the same time and the same speed and the power is shut of simultaneously, which one will spin the longest? I ask this because I am studying for a firefighter test and they have given me wrong answers before. According to the book they say the wheel with the weight on the outside, however i think back to my high school physics class and the example of the figure skater that spins faster with their arms tucked in.

ANSWER:
The issue here is how much energy each flywheel has. If the angular velocity of a rotating object is ω and its moment of inertia is I, then its kinetic energy is Iω². The quantity called moment of inertia depends on how much mass there is and how it is distributed. For example, the flywheel with most mass far from its axis is harder to get rotating or stop rotating than if most of the mass were close to the axis. Suppose we model the three flywheels as rings of mass M and radii R, R/2, and R/10; the moment of inertia of a ring is MR², so the moments of inertia would be MR², MR²/4, and MR²/100 respectively. Therefore with equal starting rotation rates, the energy of the largest ring would be bigger by factors of 4 and 100 than the other two. Your figure skater is an example of angular momentum, Iω; if she pulls in her arms, her moment of inertia decreases and so her angular velocity must increase to keep angular momentum constant. For the three rings, angular momentum of the largest ring is bigger than the other two by factors of 4 and 100 just like for the energies.

QUESTION:
I have been searching all over the internet to find out how to calculate how much wind it would take to move and lift a person, car or other things i might find interesting? I do not have any background in physics, but basic knowledge of maths. so could you give me some god insights to what mechanic's that are in play, and how they work I would be gratefully thankful.

ANSWER:
To vertically lift something you would have to know its terminal velocity when falling; that would be the upward wind velocity necessary to levitate it. For example, the air drag force on a ball is about f=0.22D²v² where D is its diameter in meters and v is its speed. A basketball has D=0.24 m and a mass m=0.62 kg, so the weight is mg=0.62x9.8=6.1 N and f=0.11x.24²v²=0.013v². Since f is the force up of the wind, to levitate the basketball the speed must be v=√(6.1/0.013)=21.7 m/s=22 m/s=49 mph. If there were no wind and the ball were falling, this would be the maximum speed it would achieve. To move something horizontally would require that you specify a lot more than its size and shape; you can see this since it is a lot easier to move a box on ice than on concrete, for example.

QUESTION:
How would you go about calculating the work done on an object being pushed up a curved inclined plane? Do you have to use calculus?

ANSWER:
If friction is negligible, all that matters is how high you lift the object, not the path you took. If there were friction you would have to use calculus, know the mathematical shape of the path.

QUESTION:
If an object (a ball) is thrown vertically, will the air resistance of both sides of the trip (upwards and then downwards) cancel themselves out, giving the exact, but mirrored, travel time/travel speed/etc. for both sides of the trip? Or will the slowing-down effect of friction give a slower velocity, and a longer descending time, for the second half of the ball's trip?

ANSWER:
No. The motions in the two directions have different dynamics. The two forces on the ball are its weight and the air resistance; the weight is down for both up and down, but the air resistance is down on the way up and up on the way down. Also, the air resistance depends on the speed.

QUESTION:
I have a question releated to weight/mass placement on a bar. My friend and I are weight lifters. We got into a discussion about the center of gravity on the bar. Here is the question. If we are using a 45 pound plate on each side and also have a 5 and 10 on each side. Each taking up the same space and the end of the bar is the same distance from the last weight and will not change. Does it change anything if the weights are not in the same order, from one side to the other? My friend says the side with the 45 pound plate close to the end is slightly heavier becuase the ratio has changed. I say nothing has changed becuase the weights on the bar are still taking up the same space. I believe it would only change if the distance to the end of the bar is changed, which it is not. I hope I explained this well enough.

ANSWER:
Assuming that the bar itself is uniform (has its center of gravity (COG) at its geometrical center), the COG of the total barbell depends on the location of the weights. Relative to the center of the bar, the position of the center of gravity may be written as COG=(45x₁+10x₂+5x₃-45x₄-10x₅-5x₆)/120 where the x_is are the distances of weights from the center. Suppose that the weights are placed symmetrically (x₁=x₄, x₂=x₅, x₃=x₆); then COG=0, the center of the bar. Now, suppose we interchange two of the weights, exchange the 45 lb with the 10 lb on one side: COG=(45x₂+10x₁+5x₃-45x₄-10x₅-5x₆)/120=(45x₁+10x₂-45x₂-10x₁)/120=(35/120)(x₁-x₂); since x₁≠x₂, COG≠0, the barbell is no longer balanced. If that explanation is too mathematical for you, try a more qualitative argument. Each weight W a distance D from the center exerts a torque about the center and the magnitude of that torque is WD. The net torque due to all weights must be zero if the bar is to balance at its center. This means that the sum of all the WDs on one side must be precisely equal to those on the other if the barbell is to be balanced about its center. If you change the Ds on only one side, the bar will not be balanced at its center. (This qualitative argument is just the mathematical argument in words.) What certainly does not change is the total weight.

QUESTION:
I understand, if a twin leaves earth and accelerates at 1 g for 10 years, decelerates at 1 g for 10 years, turns around and comes back, accelerates at 1 g for 10 years, decelerates at 1 g for ten years, then, the space fairing twin s clock would elapse 40 years and the earth bound twin s clock would elapse 59,000 years. I understand the breaking of symmetry and the time dilation because of the space fairing twin s acceleration (I think I do). My question is, is not the earth bound twin subject to the same 1 g of acceleration because of gravity, for the same duration? If both twins were in rooms with no windows, could one twin tell if they were the out bound or the earth bound twin? If both are experiencing 1 g, why the elapsed time difference?

ANSWER:
There are two kinds of time dilation, gravitational and velocity. The first is very small if gravity is small and the earth's gravity is small in this context; therefore, the acceleration of the ship is really of no importance in your example. As you seem to surmize, the gravitational time dilation for both observers will be the same; but since the total time loss depends on the total elapsed time, the contribution for the earth twin will be about 59,000 times a tiny number bigger than for the moving twin (assuming that number is right). The second is very small if the velocity is small compared to the speed of light; if you accelerate with an acceleration g, eventually your speed will get big enough that there will be appreciable time dilation; this will be the main contribution to the clock discrepancies. I do not know where you got your numbers from, but they could very well be right; certainly the 4 10-year times are right since they are basically defined. It is complicated to calculate elapsed time for an accelerated ship because it is always changing, but I will not get into that because the answer to your question is that the gravitational shift will be negligible for both observers. For detailed information on calculating how to calculate the time dilation, see the reference in an earlier answer. Also, another of my earlier answers shows the speed of the spaceship as viewed from the earth; note that this is not v=gt as you might expect classically because the earth does not see the same acceleration as the ship does.

QUESTION:
This question has bugged me for years. If the Moon orbits Earth every 28 days, then why the heck do we have 30-31 dqy months? Shouldn't we abridge our months to 28 days and add a thirteenth month to our year if we wanted our time measurement to be scientifically accurate?

ANSWER:
Seriously, you think the period of the moon's orbit has anything to do with "time measurement [being] scientifically accurate"? The unit of time, the second, is defined as "the duration of 9,192,631,770 periods of the radiation corresponding to the transition between the two hyperfine levels of the ground state of the caesium 133 atom". In other words, time is defined by using an atomic clock and has nothing whatever to do with any astronomical period. It is a lucky thing, too, that time is no longer defined in terms of the orbital period of the earth (year) or rotational period of the earth (day) or the orbital period of the moon (roughly a month) because all these periods change over time. It was natural for the ancients to measure time using naturally-occuring periodic events, but we have "come a long way, Baby"! The definition of the months is a historic relic and not to be taken as some kind of scientific standard. It is interesting since the phases of the moon were related to agriculture and the ancient Romans apparently had 10 months with a "two moon" period in the winter when there was no farming; later two more months were added in the winter, Januarius and Frebruarius. Many times in history the calendar was revised as the year became better understood. If you are interested in the history of time units, the ancient Egyptians divided day and night each into 12 time units, later the Greeks adopted a "mean hour" but still used a total of 24 per day. The hour was subsequently subdivided sexigesimally (by 60) into minutes, and then again into seconds. If you wonder about the frequent appearance of the numbers 12 and 60, still the basis for angle measure, time measure, and until recently the British currency, it is because having numbers with many factors was convenient; 12 is divisible by 2, 3, 4, and 6, 60 is divisible also by 5 and 10.

QUESTION:
How come when visible light photons interact with electrons in an atom they simply move the electrons to higher energy levels however when photons of x-rays and gamma rays interact with the atom's electrons they're completely ejected from the atom (ionized)?

ANSWER:
The energy E of a photon may be written as E=hc/λ where h is Planck's constant, c is the speed of light, and λ is the wavelength of the corresponding electromagnetic wave. Wavelengths of x-rays and γ-rays are much shorter than for visible light and therefore they have much higher energy, enough to completely remove electrons from atoms.

QUESTION:
Why does moving at very high speeds generate G-Forces?

ANSWER:
It doesn't. Having very large accelerations generates large g-forces.

QUESTION:
We know, as we go deeper in a fluid the pressure increases and it's known that pressure is direct consequence of change in momentum of the fluid molecules, so can we conclude that as we go deeper in fluid the molecular velocity increases(as more is the velocity more is the force applied by collision or is my assumption wrong?

ANSWER:
The notion that pressure is related to molecular velocity comes from the theory of ideal gases, and water is certainly not an ideal gas. In a liquid, the pressure increases with depth because the deeper you go, the more water there is above you, the weight of which is pushing down.

QUESTION:
After watching the Bond classic YOU ONLY LIVE TWICE, I read that the scene where a craft in space overtakes a capsule ahead in the same orbit in order to "swallow" it, but would be impossible because it would have to be in a separate orbit. Then when it catches up, turn vertical and move upward. Why is it not possible for an object to accelerate in the same orbit as a slower object?

ANSWER:
If the two satellites were in the same orbit, they would maintain the same separation. If they were in different but crossing orbits, you could have them come together if properly synchronized; if you were to observe this, say from the perspective of the "chased" satellite, it would appear that the other satellite was coming at you from slightly above or below. Finally, if the "chasing" satellite had rockets which he could point in any direction with any thrust, he could move exactly on the same path as the "chased" satellite but with a different speed.

FOLLOWUP QUESTION:
I don't grasp the physical law that would prevent the "chasing" capsule to catch up in the same orbit (as we see in the movie) if its thrusters accelerate it.

ANSWER:
It is easiest if we just think about circular orbits; near-earth orbits are nearly circular and I will consider only orbits whose altitude is very small compared to the radius of the earth. With each orbit there is one special speed v for an orbiting satellite where the centripetal force equals the weight, mv²/R=mg or v=√(gR) where g is the acceleration due to gravity, R is the radius of the orbit (approximately R_earth), and m is the mass of the satellite. If you are going faster or slower than that you will not be in that circular orbit but some elliptical orbit which happens to cross the circular orbit. But, let us just suppose that you are going a little faster than v, say v+u where u<<v; you got there by briefly firing your rockets out the rear tangent to the orbit. If you do nothing else, you leave that orbit. However, the force necessary F to keep you in that orbit would be m(v+u)²/R=F. But part of F is the weight, so you can write F=mg+f where f is what your rockets have to do. Therefore f=m(v+u)²/R-mg=m(v²+2vu+u²)/R-mg. Now, mv²/R=mg from above and you can neglect u² because it will be very small compared to 2uv, so f≈2muv√(g/R); you would have to point your rockets away from the center of the earth so that this force would be down but you would keep on that circular orbit going faster than other satellites in that same orbit.

QUESTION:
The speed of sound increases dramatically in water while the speed of light is decreased...why?

ANSWER:
The speed of sound v is determined by the bulk modulus K (related to how hard the material is to compress) and the density ρ of the material, v=√(K/ρ). For water, ρ=1000 kg/m³ and K=2.2x10⁹ N/m²; for air, ρ≈1 kg/m³ and K≈1.4x10⁵ N/m². So the prediction by the model equation is that sound is about 4 times faster in water, a result due mainly to the much larger bulk modulus. Light always travels faster in a vacuum than through any other medium and you should think of any material medium as impeding the motion of the waves; for all waves other than light, the medium supports the wave. To light, air is extremely close to being a vacuum but water is considerably more interactive with the light slowing it down.

QUESTION:
For a statement to be a law it must be based on observations and experiments. Newton, certainly didn't perform experiments to verify his universal law of gravitation. Was it correct then to state it as a law?

ANSWER:
Newton may not have done the experiments, but his law was the result of experiments done by others. Most important were Kepler's three laws which were empirical summaries of a large body of data on the motions of the planets. His law of gravitation, F=-MmG/r², provided a complete explanation of Kepler's laws. However, since the mass of the sun was not known, only the product MG could be determined from the data. A good measurement of G was not done until more than 70 years after Newton's death. Because gravity is such a weak force, this is a very difficult measurement to make on a laboratory scale.

QUESTION:
I have recently begun learning how to play the electric guitar, and the other day my teacher was explaining to me how I can change between different pick ups on my guitar to get a different sound. I am studying Year 11 Physics and have learnt about the harmonic series and I was wondering why the tone of my guitar changes when I change what pickup I'm using. Are there different harmonics and overtones that are accentuated by different pick ups? ANSWER:
First, you might be interested in a recent answer. I have shown the first few possible ways a guitar string can vibrate. In an actual instrument, these and many more are all happening at once in relative amplitudes determined, in part, by how the instrument is played. Now, I presume that you have several pickups placed in different places along the length of the string. As you can see, the contribution from each overtone depends on where you look at it and each is unique. One example would be if you put a pickup right in the center of the string, none of the odd overtones would be detected. Another example would be if you put the pickup 1/3 the length of the string, the second, fifth, eighth, eleventh, etc. overtones would not be detected. (I will let you see if you can figure out how I got that series!). Basically, your intuition was right, different pickups pick up different overtones.

QUESTION:
This question is in regards to flowing water and buoyancy. Lets say I have two connected reservoirs at different heights, therefore creating a pressure difference and fluid flow between them. There is a pump that refills the higher reservoir so the flow is constant, and there is a section of tubing that is vertical. If I were to put a buoyant object like a balloon in the vertical section of tubing with flow, could I keep it from floating to the top with enough flow? Basically can fluid flow in the opposite direction of the buoyancy force keep it from floating. I feel like it can but I am having trouble understanding why (seems like the only factor is density/displacement, maybe fluid flow increases drag?), just curious because my friend and I got into a random debate about it.

ANSWER:
Usually when we think of buoyant forces we are thinking about fluid statics, all fluid at rest. Your balloon in the tube will experience a buoyant force up and a force down from its weight just as it would in a nonmoving fluid. If the water is moving down, the balloon will also feel a downward force due to the drag it experiences. What this drag force is will depend on the size of the balloon, the size of the tube, and the speed of the water. It would be very complicated to calculate, but I am sure there would be a correct speed for any geometrical situation where the balloon would remain stationary. If that is enough, you can stop reading here. If not, here is an example below:

Basically, this is just a terminal velocity problem. Suppose that we imagine just releasing a spherical balloon with radius R, volume V=4πR³/3, cross sectional area A=πR², and mass m under water. The net force upward would be F=ρgV-mg=4ρgπR³/3-mg where ρ=1000 kg/m³ is the density of water and g=9.8 m/s² is the acceleration due to gravity. The drag force can be approximated as f=πv²R²ρC_d/2 where the drag coefficient for a sphere is C_d=0.47 and v is the speed of the balloon. So the net force is F_net=4ρgπR³/3-mg-πv²R²ρC_d/2 and this is zero when v=v_t, the terminal velocity, v_t=√[(8gR/(3C_d))-2mg/(πR²ρC_d))]; the second term in the square root is much smaller than the first because the mass of the balloon is very small (about 0.04 kg if R=0.1 m and the air is at atmospheric pressure) compared to the buoyant force. This is how fast a balloon would rise in still water. So, that would be the speed the water would have to be moving down for the balloon to stay in place. I did a rough calculation for R=0.1 m and found v_t≈2.4 m/s. These estimates are all for the size of the pipe much greater than the size of the balloon. Things get much more complicated if that is not the case, but you would still be able to use the water flow to keep the balloon in place.

QUESTION:
If a dome were on the moon which controlled temperature and radiation inside. Would an Olympic sized swimming pool be able to be swum in or would the water become airborne?

ANSWER:
There is still gravity on the moon, it is about 1/6 what it is on earth. So, the water would definitely stay in the pool; a splash would go much higher and farther than on earth, though. Swimming would be pretty much the same as on earth, though, because, since you would be lighter than the water in the same proportion, your buoyancy would be the same. And, since most of swimming motion is horizontal, motion not affected by gravity, it would be more or less the same as on earth. If you were to swim down vertically, the pressure would increase about six times more slowly since the pressure increase is proportional to the gravity.

QUESTION:
Using a hot plate as the sun, I am trying to use a magnifying lens to focus the radiant energy onto a thermometer. I measured the focal point length of the lens using white light at about 22 cm. When I try the experiment, there is no change in the thermometer. Why?

ANSWER:
The lens focuses visible light. Your hot plate is mainly infrared light and the lens is not transparent to infrared light, it is blocked. So your problem is that the hot plate is a very poor replica of the sun because the sun is most intense in the visible part of the spectrum.

QUESTION:
In an acoustic guitar, why does the timbre of a note change depending on where the string is plucked? Is it to do with different wave lengths? ANSWER:
For nonmusical readers, timbre refers to how a musical note sounds. How can you tell the difference between a middle C on a piano, a guitar, and trumpet? They all play the same "fundamental" note which has a frequency of about 262 cycles per second. But, depending on the design of the instrument and how it is played, other frequencies called overtones are also present. It is the relative mixture of these overtones which determine the timbre of the instrument. A guitar is a relatively simple instrument in that its overtone possibilities are all integer multiples of the fundamental. However, the mixture of overtones may be controlled to some extent by the player by how he plucks the string. Plucking it at the center, for example, emphasizes all the even overtones, vibrations which have a maximum at the center of the string (see the figure to the left). A more lengthy discussion can be found here.

QUESTION:
My question deals with the "potential energy" of a gravitational field, in relativistic terms. As an object falls in a gravitational field, a tiny bit of its mass gets converted to kinetic energy, a small reduction in mass. However, according to Special Relativity, as an object accelerates, gets closer to the speed of light its mass (how it interacts with spacetime) increases. So, does an object accelerating (increasing kinetic energy) in a gravitational field lose mass, as a result of falling in a gravitational field, or gain mass as a result of approaching the speed of light? OR is there some breakeven point were the reduction of mass from falling stops, and then mass starts increasing again as a result of approaching the speed of light. If the mass starts increasing because of approaching the speed of light, wouldn't the gravitational potential approach infinite?

ANSWER:
You are the same questioner from earlier similar questions. The reason you do not want to simply say that there is "a small reduction in mass" if it is falling is that it is not at rest when you look at it later. As I explained in the earlier answers, you need to calculate things relativistically to find out how the mass changes. That is essentially what the last link I gave you in my earlier answers does, calculates β=v/c as a function of time for a constant force. To know how the mass varies (if you interpret relativistic momentum that way), just calculate m₀/√(1-β²) where m₀ is the rest mass. It occurs to me, though, that it might be of interest to redo that calculation for a force which is not constant but which has a value mg=m₀g/√(1-β²) where g is the acceleration due to gravity in whatever strength field you wish to examine. I will not give all the details here, just the results. We start by integrating the relativistically correct form of Newton's second law,

m₀g/√(1-β²)=dp/dt=(d/dt)[m₀v/√(1-β²)] integrated gives
gt/c=½ln[(1+β)/(1-β)] solved for β gives
β=(1-exp(-2gt/c))/(1+exp(-2gt/c)); put this into the mass and get
m/m₀=1/√(1-β²).

These are plotted to the right above. Note that the result for a constant force m₀g is plotted in red for comparison. Potential energy is not a useful concept here. Note that the time to get to near c, t≈3c/g, is about 2.9 years for g=9.8 m/s². At that time the moving mass is about 10 times greater. A more lengthy discussion of a mass in a uniform gravitational field (including general relativity) can be seen here.

QUESTION:
Using real-world estimates for the coefficient of friction between his feet and the ground, how fast could the Flash run a quarter-mile? Assume that the limiting factor for his acceleration is the force parallel to the ground that his feet can apply.

ANSWER:
Suppose he is running on a dry asphalt road with rubber-sole shoes. Then the coefficient of static friction is approximately μ≈0.8. The maximum force of friction on level ground would be f_max≈μN=μmg≈8m where m is his mass. So, his acceleration would be a=f_max/m=8 m/s². A quarter mile is about 400 m, so assuming uniform acceleration the appropriate kinematic equation would be 400=½at²=4t², so t=10 s.

QUESTION:
Why is it that hot objects such as lightbulb filaments emit light while cold objects such as ourselves emit no light at all?

ANSWER:
Well, let's first define "light" as any electromagnetic radiation, not just the visible spectrum. All objects radiate light and the wavelengths they predominantly radiate depends on temperature. A human body has a temperature around 300 K (80⁰F) and a tungsten filament has a temperature of around 3000 K (5000⁰F). The picture to the right shows the radiation for both of these temperatures; also note the visible spectrum indicated by the colored vertical bands near 0.7 microns. At 3000 K, the radiation is most intense in the region of visible light; at 300 K there is almost no intensity of visible light and the spectrum is most intense around 10 microns which is in the "invisible" infrared spectrum. Night vision goggles are sensitive to infrared radiation and enable you to see "cold" objects in dark situations.

QUESTION:
I have a doubt about static friction and number of wheels. As for elementary physics principles
1) static friction depends is mass times the coefficient of static friction
2) static friction does not depend on surface static friction is independent by the number of wheels.
... but it is hard to accept to me! Let's suppose to design a cart to be pushed by a worker. The total weight (cart + content) is about 1000 kg. The question is: as for the static friction it is better to use 4 or 6 wheels? ANSWER:
If the cart is to be "pushed by a worker" it is not static but rather kinetic friction which is in play unless all the wheels are locked. And this is not friction due to the contact between the wheels and the ground but friction due to the axles rubbing on the wheels. But, let's talk about friction anyway because you seem to have a serious misconception. First of all, the friction is proportional to the normal force which presses the wheel to the road, not the mass. If there were one wheel, the maximum static frictional force you could get before the cart started slipping (call that f_max) would be the weight W times the coefficient of static friction μ_s (on level ground), f_max=μ_sW. If you had two wheels, each wheel would hold up half the weight so the maximum static frictional force you could get from each wheel would be μ_sW/2; but the total force is still μ_sW. Things are more complicated on a slope, but the conclusion is still that you do not gain an advantage regarding traction by having more wheels. The reason big trucks, for example, have many wheels is so that each wheel does not need to support so much weight, not to get more traction.

QUESTION:
I understand that acceleration due to gravity decreases with distance, specifically by the inverse square law. That being said, what is the maximum distance for which one can use 9.81 m/s² as g for Earth? ANSWER:
That depends entirely on how accurate you want to be, there is technically no place other than the surface of the earth where this is the acceleration. Furthermore, the number 9.81 is simply an average value; it varies over the surface of the earth due to local density variations, rotation of the earth, influences of the moon's gravity, altitude variation, etc. You need to ask something like "at what altitude h from the surface is the value of g changed by X%?" Then X/100=((1/R)²-(1/(R+h)²))/(1/R)² where R is the radius of the earth. Provided that h is small compared to R, you can solve this equation approximately as h≈XR/200. For example, g will be reduced by 2% when h≈R/100. Another example: the International Space Station is at an altitude of about 230 miles, about 6% of the earth's radius. Then X_ISS≈200x0.06≈12% smaller than 9.81 m/s².

QUESTION:
In a good fireplace the smoke goes up the chimney rather than out into the room even if the fire is not directly beneath the hole.What causes this draft and why is it better the taller chimney?Why is the draft better on a windy day? And why do chimneys puff?

ANSWER:
The main reason that the smoke goes up the chimney is that hot air rises. The hot air from the fireplace fills the chimney and this rising air results in a lower pressure at the bottom of the chimney. So all air and smoke at the bottom of the chimney is drawn upward. A taller chimney is better because there is a larger volume of hot air resulting in a lower pressure at the bottom; there is a limit, however, determined by whether air higher up has cooled and by frictional drag considerations over a longer distance. If a wind blows over the top of the chimney, Bernoulli's equation tells us that the pressure is lowered there providing an even greater lift of the air column; from what I have read, though, the hot air rising is the most important consideration. I do not know what you mean by "puff".

QUESTION:
If I am driving my car with a bowling ball in the trunk, does it take the same energy to accelerate the vehicle to a given speed at a given time if the ball is free to roll around as it would if it were fixed to the vehicle? I assume that the net energy use would be the same in both situations (same total vehicle mass), but the acceleration rates would be different - ie: the fixed ball would result in a constant acceleration to speed, while the rolling ball would result in a non-constant acceleration. If this is true, could I harness the energy of the ball's movement relative to the vehicle (using some sort of linear generator) without causing parasitic energy loss to the vehicle?

ANSWER:
As long as the ball and the car end up going the same speed, the total energy to get them there is the same (neglecting frictional and air drag forces). If you devise some way to take enegy away from the ball, that energy ultimately must come from the engine.

QUESTION:
What would the yield of a 5000 ton iron slug accelerated at 95% of C by say a bored Omnipotent be? Would it be enough to mass scatter a planet?

ANSWER:
I get the strangest questions sometimes! So, 5000 metric tons=5x10⁶ kg. The kinetic energy would be K=E-mc²=mc²[(1/√(1-.95²))-1]≈10²⁴ J. The energy U required to totally disassemble a uniform mass M of radius R is U=3GM²/(5R) where G=6.67x10^-11 is the universal gravitational constant. So, taking the earth as a "typical" planet, U=3∙6.67x10^-11∙(6x10²⁴)²/(5∙6.4x10⁶)≈2x10³² J. So your god's slug is far short of supplying enough energy to totally blast apart the earth.

QUESTION:
I am baffled by the fact that if you have a light source and two (or more) mirrors, you multiply the total amount of light that illuminates a room by the number of reflecting surfaces. Does this mean that we increase the total amount of light energy without having to add energy to the light source? Or does each reflection carry less energy than the incident beam from the original light source?

ANSWER:
Yes, you can brighten a room with mirrors; but there is no problem of energy conservation because you are simply using light which would otherwise have been absorbed by the walls. But, not 100% of the light is reflected because if it were, you could turn off the light and the room would not go dark; and the room would just get brighter and brighter if you left the light on. I can do a rough calculation to give you an idea of how long light would bounce back and forth. Suppose that the mirrors were 99% reflective (much more than actual mirrors are) and you had two parallel mirrors separated by a distance of 3 m. Since the speed of light is 3x10⁸ m/s, the time between reflections is 3/3x10⁸=10^-8 s. There are therefore 10⁸ reflections per second. At each reflection the intensity decreases by a factor of 0.99, so after n reflections the intensity has been reduced by a factor of 0.99ⁿ. Suppose we look at how much light is left after 0.1 ms=10^-4 s, 10,000 reflections: 0.99¹⁰⁰⁰⁰≈2x10^-44 or 2x10^-42%. I think we can agree that it is almost instantaneously gone!

QUESTION:
I was hoping for clarity regarding peak power and its relationship to the amount of joules in a pulse. Take this simple example. Supposing you have 1 Watt average power, a pulse width of 1 femtosecond, with a repetition of 1 Hz. The average power would be 1 watt, the peak power would be 9.3x10¹⁴ watts. My question is, since the average number of joules would be 1J, would there also be peak Joules of 9.3x10¹⁴ Joules?

ANSWER:
The energy comes in a 1 fs=10^-15 s pulse and there is one of these per second. I do not know where you got 9.3x10¹⁴ W unless you know something about the shape of the pulse that I do not. The average power P over one pulse would be P=10¹⁵ W. Since power is energy per unit time, P=E/t or E=Pt where E is the energy per pulse, E=1 J. Since there is one pulse per second, this hangs together because we then have 1 J per second=1 W.

QUESTION:
How does 'ball lightning' come about in the atmosphere?

ANSWER:
See an earlier answer.

QUESTION:
Around 1905 when Lorentz came up with his tranformation equation, it was assumed that c was constant, but was it also assumed that c is the speed limit of the universe? Why didn't he assume that v could be greater than c in some cases? Why didn't he put a corrective constant next to c in the equation to allow for the case that time might stop BEFORE or After v reaches c? The reason I'm asking this question is because the Lorentz transformation has time stop exactly when v=c. How did he know that should be the case?

ANSWER:
You have your history a little skewed, I think. Lorentz was working with electromagnetic theory, Maxwell's equations in particular. The transformation which bears his name was arrived at empirically with no reference to the speed of light. He noticed that if you took Maxwell's equations and transformed them into a moving frame of reference that they took on a different form which was unacceptable because they had internal inconsistencies which could not be borne out by experiments. He found that if he used a somewhat different transformation, now called the Lorentz transformation, the transformed equations were self consistent; he had stumbled on a truth without understanding what he had. And he certainly did not consider the speed of light constant because he was one of the foremost proponents of the luminiferous ther, the special medium which supposedly supports light waves. To my mind it is unfortunate that the transformation is named for him since it was an accidental empirical discovery, not based on any fundamental physics. Some books try to refer to the Lorentz-Einstein transformation, but that hasn't really caught on. To understand why c is a constant and why it has the value it does, go to my FAQ page; there you can also see earlier questions about why c is the speed limit. Your question about a "corrective constant" makes no sense since universal constants by definition do not need correcting. Since no clock can ever reach c, time never "stops". I doubt that Lorentz ever thought about time stopping in any context.

QUESTION:
What do you think may be some possibilities if heat all of a sudden ceased to exist? Or, is it possible to completely neutralize heat within a contained space?

ANSWER:
First of all, read an earlier answer regarding what heat is. Heat is the transfer of energy, not the content of energy. Therefore the answer to the first question you ask is that if there were no transfer of energy, everything would simply stop, I guess. Regarding your second question, heat flow can be stopped or slowed down for isolated systems; for example, a thermos bottle.

QUESTION:
Perhaps you can help solve a disagreement we have at work. The question being "Does a person's initial velocity during a jump equal their final velocity once the land?" My contention is "no" in that the jumper could theoretically produce any velocity on the way up, but downward would be limited to terminal velocity. Who's right?

ANSWER:
Technically, you are correct. If air drag is present, energy is lost which results in the landing speed being less than the launch speed. In practice, however, for a person jumping into the air the height acquired is not high enough for this to be a measurable effect; that is, this is an example where we can say, as we often do in an elementary physics course, that air drag is negligible. A typical terminal velocity for a human is about 120 mph≈54 m/s. If you jumped with this speed you go over 100 m high, obviously not in the cards. I did a rough estimate assuming the maximum height you could jump would be about 2 m; if the person drops from 2 m his speed at the ground would be about 6.32 m/s without drag, 6.30 m/s with drag, a 0.3% difference. For comparison, dropping from 100 m the speeds would be roughly 44.1 m/s and 37.1 m/s for no drag and drag, respectively. It is good to be precisely correct as you are, but it is also good to be able to make reasonable estimates in real-world situations.

QUESTION:
It is said that charges are quantised.Also if we bring two identical solid conducting spheres in contact with each other,their charges are equally distributed among them. Now suppose if we have a body A with -5e charge and body B with 0 charge.Now what will be the charge distribution between the bodies if we bring them in contact and then separate them?Since charges are quantised,we cannot have 2.5-2.5 distribution.So will it be 3-2 or 2-3 distribution or what?

ANSWER:
The rules you learn like the one you quote apply only when the charge can be thought of as a continuous fluid which can spread itself out on any surface, no matter how large, and have zero thickness. Because the electron charge is so small, these rules work very well to describe electrostatics for normal circumstances. Obviously, a case like you describe, with 5 excess electrons is in no way like a fluid and I would guess that anything from 5-0 to 0-5 could be the distribution over a time. In principle, the 5 electrons would arrange themselves so that each was as far away from the other 4 as possible, but there would not be a unique such distribution.

QUESTION:
I was looking at a few years old burn scar on my hand today and it got me to wonder what happened on a subatomic level to the electrons and protons of my hand when I received the burn. So could you tell me what happened to the electrons and protons of my hand when I accidentally touched the inside wall of my oven when takibg the food out?

ANSWER:
What happens when you cook meat? Chemistry. Adding sufficient heat will cause some molecules in the meat to break apart and make new molecules. That same thing happens in your hand when you burn it just a lot of chemistry going on. Then later biology takes over and takes all that burnt flesh and sheds and absorbs the burnt flesh and creates new molecules specialized to be scar tissue, again basically chemistry. So, electrons and nuclei of all just move around as molecules are destroyed and then rebuilt. It is all just a bunch of chemical reactions.

QUESTION:
If I consider a tube both end open and dip one end in water (like pipette in chemistry lab) and close the other by thumb, water remain hanged in the tube. If we say it is because the atmosphere that pushes up on the water in the tube is same as that of remaining air in tube pushing down on the water. Won't the water fall out due to its own weight as the upward and downward forces are the same?

ANSWER:
As you lift the tube out of the water, the weight of the water in the tube pulls it down and the volume of air between your thumb and the top of the water in the tube increases. Because the volume increases, the pressure decreases so that when the bottom end of the tube is pulled out of the water the pressure at the top of the column of water is smaller than atmospheric pressure at the bottom. Therefore, the water column has three forces on it which are in equilibrium: its weight down, a force down due to the pressure at the top, and a force up due to the pressure at the bottom. Your error was in assuming that the pressure at the top of the column is atmospheric.

QUESTION:
If an electron in an atom can only orbit in fixed orbitals at certain frequencies, how does a gas molecule increase its speed when heated. It implies that the gas pressure also would be allowed only at certain energy levels but the pressure seems to increase in a linear way and jump to fixed pressures when the molecule has attained enough energy.

ANSWER:
The question is, how can energy be added to a gas? Let's think about a single molecule in the gas. It has, essentially, two kinds of energy internal energy which are allowed states of the molecule and are quantized, that is restricted to certain discrete values, and the kinetic energy associated with the molecule's motion as a whole as it hurtles across the room. This kinetic energy is not quantized. So, when you heat up a gas you are adding to its kinetic energy for normal temperatures. You have to get to extremely high temperatures before you start excite atomic states. You might be interested in why internal states are quantized and kinetic energy is not. In quantum mechanics, systems which are bound (like electrons in atoms) are quantized whereas systems which are not bound (like your freely moving molecule) are not.

QUESTION:
When a ball is thrown vertically upwards ignoring air resistance, and another ball is also thrown upwards with air resistance, the time taken is less for the ball with air resistance to reach max height. Why is this "because average acceleration/force is greater"? Wouldn't there be less acceleration/force because the air resistance cancels some out?

ANSWER:
The reason is that the ball with air resistance does not go as high. The force on the ball without resistance is the weight of the ball pointing in the downward direction; but the downward force is greater for the ball with air resistance because the drag force is also pointing down. Therefore the ball with resistance slows down faster so it stops more quickly. Think of an extreme example: if you throw the ball upwards in honey which has very great resistance, it stops almost immediately.

QUESTION:
If I had a graph of an object's momentum against time, and at t₁ momentum is p₁ and at t₂ momentum is p₂. p₁ is a positive y value but p₂ is negative. Wouldn't the average force be (-p₂-p₁)/(t₂-t₁) instead of (p₁-p₂)/(t₂-t₁)?

ANSWER:
The fact is, both force and momentum are vectors so the signs of these quantities mean something in one dimension which is evidently what you are asking about. A one-dimensional vector with a negative value points in the negative coordinate system direction. Let's take a particular example of your supposition, p₂=-5 kg∙m/s, p₁=1 kg∙m/s, and t₂-t₁=1 s. This means that the particle started out moving in the positive direction and ended up moving in the negative direction; therefore the average force causing this must have been pointing in the negative direction, right? The average force F is defined to be F=(p₂-p₁)/(t₂-t₁)=(-5-1)/1=-6 N. Your proposal would give F=(-p₂-p₁)/(t₂-t₁)=(5-1)/1=4 N, obviously pointing in the wrong direction. (I have no idea where your F=(p₁-p₂)/(t₂-t₁) came from; this is also wrong.)

QUESTION:
Why do physicists refer to the non-visible portions of the electromagnetic spectrum as "light"? I thought only the visible portion was considered "light".

ANSWER:
This is really just semantics and of no real importance. We often refer to "the speed of light" which is universally understood as the speed of electromagnetic radiation. I never heard of anyone use the word light to refer to gamma rays or AM radio waves, for example. If we want to be very clear, we say "visible light" when referring specifically to the visible spectrum.

QUESTION:
How is it possible to measure the the distance between an electron and a nucleus? I don't mean calculate, but measure. In other words, what is the operational definition of the Bohr radius?

ANSWER:
It is not possible to measure the distance because it has no fixed value. All you can do is predict the probability of finding the electron some distance from the nucleus. There is no operational definition of the Bohr radius, it is just defined guided by history (Bohr model); you cannot measure it.

QUESTION:
Whenever we roll a ball or spin a quarter it will slow down and eventually stop, since energy cannot just dissapear where does it go?

ANSWER:
The kinetic energy is being taken away from the ball or coin by friction. That energy shows up as thermal energy, the ball/coin-table-air all get a little bit warmer. Also, since you can hear the ball rolling and the coin spinning, some of the energy must be lost to sound.

QUESTION:
How can the speed of light be constant, when time is not? In my understanding, the rate at what time passes changes, relative to speed of motion and gravitational forces. So if the speed of light is 186,000 a second, but a second could flow at different rates, how does this affect the speed of light?

ANSWER:
This is a chicken & egg sort of thing. Time not being "constant", moving clocks running slow, is a result of the fact that the speed of light is a universal constant. See my earlier discussion of the light clock which demonstrates this. Not only do clocks run slower, but lengths get shorter by exactly the same factor. So, if it takes a pulse of light 1 s to travel between two points A and B separated by 3x10⁸ m for one observer, another observer moving with respect to the first might observe the same light pulse take 0.5 s to travel between A and B which are now separated by 1.5x10⁸ m, exactly the same velocity.

QUESTION:
If I raise a mass above the earth, you would say that I'm increasing the potential energy by mg x delta(h). So, on a macroscopic scale , if I raise a mass above the earth, doing work, Im actually adding mass to the earth-mass system? and the increase in potential energy (height classically) is actually a small, virtually undetectable increase in mass of the earth/mass system?

ANSWER:
Let's look at several ways to understand this situation. Start with the work-energy theorem which says that W_ext=ΔK=½mv₂²-½mv₁², work done by external agents equals the change in kinetic energy. So, when you lift it to height h, gravity does W_earth=-mgh and you do W_earth=mgh units of work and so kinetic energy is unchanged. This ignores a couple of things, one being that the earth is not flat and the field is not uniform and another is that you do work on the earth-mass system (EMS) thereby increasing the mass of the EMS. (Since you do work on the EMS, it does the same magnitude of negative work on you, so your mass decreases by the same amount as the EMS mass increases; energy is conserved if all forces are internal, that is if we think of you as part of the earth.) The work done on the mass is enormously larger than the work done on the earth because both experience the same force but the earth hardly moves at all while the mass goes (almost) h, so almost all of the mass increase will be in the mass, not the earth.

To get an idea of the magnitudes of these effects, I will do a specific example, m=1 kg, h=1 m, R_earth=6.4x10⁶ m, M_earth=6x10²⁴ kg.

Work done on m is mgh≈10 J.

Increase in mass of m is mgh/c²=10/9x10¹⁶≈10^-14 kg.
Earth will move only about 10^-23 m, work you did on it will be only about 10^-22 J, mass increase about 10^-37 kg.
I estimate that g will be smaller by about 3x10^-5 m/s² at 1 m above the surface, a very small difference. But, it is huge compared to the mass increase. The effect on g is a 3x10^-4% effect and the mass increase is a 10^-12% effect.

Note that I have not said anything at all about potential energy yet. I have always thought, at an elementary physics level, of potential energy as just a clever bookkeeping device to automatically keep track of work done by a force which is always there, gravity in this case. To illustrate, W_ext=ΔK=W_gravity+W_you, so ΔK-W_gravity=W_youso we define the potential energy difference to be ΔU≡-W_gravity and, voilá, ΔE≡ΔK+ΔU=W_ext where external work now excludes work done by any force for which we have introduced a potential energy function; we have simply internalized the work done by a force always there. This has no effect on my discussion of mass changes above.

FOLLOWUP QUESTION:
So, when the mass falls back down thru the distance, the very small change in mass we had on the way up gets converted into Kinetic energy. now, lets do work in the horizontal plane, so gravity is not a factor. I have a mass, I perform some work on it (adding energy) now energy has mass, but at very very low speeds, compared to light, nature allows MOST of this work supplied to go into a velocity increase, with a very small amount (undetectable amount) to go into mass increase. As such, at low speeds, almost all of the energy supplied (work done) shows up as a velocity increase. Now, at very high speeds approaching light speed, nature in order to not exceed the speed of light, takes most of the supplied energy (work done) and puts it into a mass increase, with very little going into a velocity increase. Is this a correct picture I have of this?

ANSWER:
You have it about right. There are some subtleties, though: if you are going to talk about mixtures of kinetic and mass energies and introduce particles with large speeds, you are going to have to start doing things relativistically. I have emphasized before that you have to be careful about what you call mass. My own preference is to work only with rest mass which I usually denote as m. In your earlier question, all particles which we were looking at were at rest, so when I talked about increase in mass, that was increase in rest mass. If the object is moving, what do we mean by mass? In spite of what many text books say, I do not say that mass increases with velocity but rather that linear momentum has been redefined in such a way that it is no longer simply mass times velocity. Now, the energy E of a particle with rest mass m and speed v is E=√(m²c⁴+p²c²) where p is the linear momentum defined as p≡mv/√[1-(v²/c²)]. Now, you will note, if the particle is at rest, E=mc²and p=0, and as long as v<<c, p≈mv. Also note that kinetic energy is no longer ½mv², rather it is that amount of energy which is not rest mass energy, K=E-mc²; you can easily show that if v<<c, K≈½mv². For example, v=0.8c=2.4x10⁸ m/s, m=1 ng=10^-12 kg:

p=10^-12∙2.4x10⁸/0.6=4x10^-4 kg∙m/s, wheras mv=2.4x10^-4 kg∙m/s;
pc=1.2x10⁵ J;

mc²=9x10⁵ J;

E=9.08x10⁵ J;

K=8x10³ J, whereas ½mv²=2.9x10⁴ J.

YET ANOTHER FOLLOWUP QUESTION:
I just wanted to add that in my previous question, concerning mass changes with increasing velocity, what I'm calling "mass increase"is not a " physical structure" change of an object. I view this increase of mass with velocity as a " change in how the object interacts with space time. Therefore, I believe that the gamma correction at large velocities is how the object is changing how it interacts with space time. I think that some people believe this "mass increase" with velocity is an actual accumulation of matter or a physical enlargement of the object. I hope my thinking is correct on this?

ANSWER:
You are essentially correct. If you want to interpret the new definition of momentum as meaning that the mass m' of a particle with rest mass m and moving with speed v is m'=m/√[1-(v²/c²)], that is fine; with this interpretation you are saying that the momentum is still mass times velocity but that the mass depends on velocity. But m' should be thought of as the inertia of the particle and not some measure of "the amount of stuff" as you suggest. The faster something goes, the harder it becomes to make it go faster (which means that its inertia is increasing) until eventually you run against the wall that v must always be less than c. You may be interested in a previous answer regarding the kinematics of a particle which experiences a constant force.

QUESTION:
Generally, we are trying to determine how much electricity will be generated by falling water . With that in mind, an engineering group has proposed a project whereby they place one of their machines inside a tube. We have all of the electrical equations worked out from the movement of the blades inside the machine -- the question for the Physicist is this: what is the speed of the water if it falls 5M or 10M or 20M? If the diameter of the pipe is an important variable, the answer is that we can make the mouth of the pipe as wide as we want: 5M, 10M, 20M etc. Bottom line, we want to achieve a water speed of at least 6 meters/second.

ANSWER:
You have not given me any details about the source of this water. This sounds like a classic Bernoulli's equation problem in elementary physics where you have a deep reservoir and there is a hole at a depth h in the dam; what is the speed with which the water squirts out? This hole could be your pipe and the cross section of the pipe does not matter as long as its diameter is small compared to h. Bernoulli's equation states that ρv²+ρgy+P=constant where ρ is the density of the fluid, P is the pressure, v is the speed of the fluid, y is the vertical position, and g=9.8 m/s²≈10 m/s²is the acceleration due to gravity. In your case, I would choose y=0 at the bottom of the dam where your pipe is, so y=h at the surface; the velocity at the surface of the lake is approximately zero and the velocity in the pipe is v; the pressure at the top and the bottom is the same, atmospheric pressure P_A. So, Bernoulli's equation is ρv²+ρg∙0+P_A= ρ∙0²+ρgh+P_Aor, ρv²=ρgh, or v=√(2gh). Interestingly, this is exactly the speed the water would have if you just dropped it off the top of the dam. For example, at a depth of 5 m the speed should be about 10 m/s before you put your machine in it.

Bernoulli's equation is, essentially, just conservation of energy for an ideal fluid; water is not an ideal fluid, but close enough for this to be a pretty good approximation. However, you will be asking this moving fluid to do work on your generator which will take energy away and slow the water down. So, maybe it is useful to calculate the energy which this moving water has. The quantities in the equation are energy per unit volume of the water, so E= ρghV where E is the energy contained by a volume V of the water. The power P (not to be confused with pressure), is the rate at which the moving water is delivering energy, P=dE/dt=ρgh(dV/dt). If the cross section of your pipe is A, then dV/dt=Av so P=ρghAv. Here is an example: taking h=5 m with v=10 m/s as above, using ρ=1000 kg/m³, and assuming a pipe with a diameter of A=1 m², P=5x10⁵ W=500 kW. You could not get any more power than that from this water.

QUESTION:
In Galiean Relativity, there is no such thing as absolute velocity, as all velocities are relative. However, there is such a thing as absolute acceleration in Galiean Relativity. In Special Relativity, there is no such thing as absolute velocity, however there is also no such thing as absolute acceleration (unlike Galiean Relativity). Isn't the connection/correction to acceleration the Lorentz Transforms Gamma correction, G=1/square root 1-v/c^2?

ANSWER:
The Lorentz transformation for acceleration is very complicated and I will not write it because it really has very little interest or applicability in the theory of special relativity. As you note, all Galilean observers will measure exactly the same acceleration. The reason that this is so important is that Newton's second law, if written as F=ma, on which so much of classical mechanics hinges, is true only if all observers measure the same acceleration because surely all observers will agree on the force applied. Interestingly, Newton did not write his second law in this form but rather in the form F=dp/dt where p is linear momentum. You can learn more about relativistic momentum here and here. Also of interest here is the motion of a particle which experiences a constant force; the acceleration cannot be constant because the particle cannot go faster than c.

QUESTION:
Does Newton's third law apply only to contact forces or to non-contact forces (e.g. gravity, electrostatic force)? Our teacher says that it applies when the bodies come in contact with each other, but I think it should be applicable to both. Which is the case?

ANSWER:
Well, it depends on how you state the third law. If you state it as "if particle 1 exerts a force on particle 2, particle 2 exerts an equal and opposite force on particle 1", then there are certain cases where this law does not apply: see an example for electromagnetism in an earlier answer. However, when fields are present, Newton's laws are much more subtly stated because the fields themselves contain energy and momentum. You can read a more detailed discussion, concerning linear momentum conservation which crucially depends on Newton's third law, in another earlier answer.

QUESTION::
My question is concerning the energy required to pry apart (binding energy) of molecules ( not nuclear). Such as the burning of hydrocarbon fuels. When you do work against gravity, the work (energy) you put in goes into potential energy above the earth. When you pry apart the molecules, you are doing work against the attractive forces holding the molecule together( binding energy)Does that work appear as potential energy (distance) between the molecules ( if the forces remain attractive) OR does the attractive force between the molecules change from attractive to repulsive or vanish with distance? If the forces remain attractive then the work supplied should become potential energy between the molecules. If the forces vanish, or become repulsive, the work (energy) supplied, prying them apart, should manifest as a small increase in mass of the molecules forced apart. I'm not sure how the forces between bound molecules behave with distance as you separate them.

ANSWER:
Molecules are held together by electromagnetic forces, so it is useful to get an order-of-magnitude idea of the binding energy compared to mass energies. Consider a hydrogen atom with an electron and a proton bound together by their electric attraction. The energy necessary to move the electron very far away is 13.6 eV. The rest-mass energy (mc²) of a hydrogen atom is about 1 GeV. Therefore, since you have added energy by ionizing the atom, the atom is lighter by about 100x13.6/10⁹ %≈10^-6%. Any molecular binding energy will be of the same order-of-magnitude. The energy we get from chemistry comes from mass and it is extremely inefficient. So, although E=mc² is at the heart of things, you usually do not have to worry about mass changes in molecular chemistry because they are so tiny. To do detailed calculations of chemical reactions usually requires that you do things quantum mechanically which requires a potential energy function. These calculations can be very complex and approximate models are used to simulate the potential energies of the molecular systems. Once you get beyond the simplest atoms and molecules, the calculations can only be done numerically and approximately on computers. An example of a potential energy function, the Morse potential, for a diatomic molecule is shown in the figure to the left. The form of this potential is V_Morse=D_e[1-exp(-(r-r_e))]²; note that, for one of the atoms in the molecule, the force (slope of the potential energy function) is repulsive for r<r_e and attractive for r>r_e. This is expected since the molecule has a nonzero size because of repulsion but is bound because of attraction. A first approximation often used for bound molecules is a harmonic oscillator potential (masses attached to a spring).

QUESTION:
Suppose an ice cube is suspended in a gravity free region (having room temperature). Now as the ice melts,what shape will the whole system(including melted water) will attend and why? What shape will the solid ice have(i.e will it maintain its cubical shape as it melts)?

ANSWER:
First, think about an ice cube sitting on a table. Why does it melt? Because heat flows through the surface to the small volume of ice right below the surface causing that small volume to melt when sufficient heat has been added. But, a small volume near the edge of the cube has a larger area and a small volume near a corner has a larger area yet. This is illustrated in the figure to the left: each little cube on the surface of the big cube has a volume V and faces with areas A. As you can see, the cube on the face of the big cube has an area A through which heat can flow, one on the edge has an area 2A, and one in the corner has an area 3A. Therefore, the cube will melt fastest on the corners, next fastest on the edge away from the corner, and slowest elsewhere. That is why a cube gets more rounded as it melts. I cannot think why this would be any different in a zero-gravity situation except that the water would not flow away. So, you would have a layer of water around the still unmelted ice but, eventually, when there was enough water, you would have the unmelted ice inside a sphere of water.

QUESTION:
If I observe an object which is in relative motion it will have increased mass and due to length contraction an increased mass density. So can it become a potential "relativistic" black hole?

ANSWER:
No. In the frame of reference of the object, everything is perfectly normal. Besides, a black hole is a singularity, infinite density and zero size, so the object would have to be going the speed of light which is not possible.

QUESTION:
The speed of a mass thrown from a moving object is effected + or - by the speed of the thrower. Not so with the speed of light. If light speed was limited by some unknown phenomena, that would cause questions in one area - but, with a source moving opposite to the direction of the light, the speed is still the same. Light is not affected + or - by the movement of the source. It cannot travel faster, nor can it travel slower based on external forces, though it can be vector shifted by gravity and the medium thru which it passes. Why? Unless we can find a reason, say in quantum mechanics, like the metaphysical identity of an electron, these known truths appear to contradict the laws of cause and effect.

ANSWER:
The speed of light in vacuum, as you note, is constant regardless of the motion of the source or of the observer. Strange, I know, but you seem to think that the reason for this is unknown. Indeed, it is fully understood why the speed of light in a vacuum is a universal constant; see earlier answers.

QUESTION:
I was watching Babylon 5 and in there there was a description of an Earth Alliance space ship weapon. The weapon was a gun that has two very conductive parts on both sides and a conductive armature in the middle and electricity somehow launches the projectile. The gun is 60 meters long, has two barrels with each capable of firing two shots per second simultaneously. The projectile is 930 kg in mass (1.7 m long 20 cm in diameter) to a velocity of 41.5 km/s. The barrel of this electric gun is 60 meters long. Is this kind of gun physically possible to build?

ANSWER:
I always like to look first at the energetics when answering questions like this. Assuming that the acceleration of the projectile is uniform, I find that the time it would take to traverse the barrel is 0.029 s and the average acceleration is 1.4x10⁶ m/s². Thus, the average force on the projectile would be F=ma=1.4x10⁶x930=1.3x10⁹ N=280,000,000 lb. I do not think you could have a projectile which would not be destroyed by such a force. But, suppose the projectile could withstand this force; the energy which you would have to give it would be E= mv²=8x10¹¹ J. Delivering this energy in 0.029 s would require a power input of P=8x10¹¹/0.029=2.8x10¹³ W=28 TW; for comparison, the current total power output for the entire earth is about 15 TW. Or, if you think of the energy being stored between shots, and there are four shots per second, P=8x10¹¹/0.25=3.2x10¹² =3200 GW; the largest power plant currently on earth is about 6 GW. And, this power source needs to be on a ship? I do not think this gun is very practicable!

You might be interested in similar earlier questions I have answered.

QUESTION:
Something ridiculous I thought of, if the Moon suddenly stopped moving and began to fall toward the Earth, how long would it take to impact? I'm stumped as to how to calculate this, as the force on the Moon gradually increases as it falls, and the Moon also pulls the Earth toward it, and the radius of each object would have to be included.

ANSWER:
I guess I am going to have to put questions like this one on the FAQ page. You should read the details of these earlier questions since I do not want to go over all the details again. It is tedious and uninstructive to try to do this kind of problem precisely. I, being a great advocate of "back of the envelope" estimates, use Kepler's laws to solve this kind of problem; I have found that a very excellent approximation to fall time can be found this way. I note that the mass of the moon is only about 1% of the mass of the earth, the period of the moon is about 28 days, and the moon's orbit is very nearly circular. The trick here is to use Kepler's third law and recognize that a vertical fall is equivalent to the very special orbit of a straight line which is an ellipse of semimajor axis half the length of the line. Kepler's third law tells us that (T₂/T₁)²=(R₂/R₁)³ where T_i is the period of orbit i and R_i is the semimajor axis of orbit i. Now, T₁=28 and R₂=R₁/2 and so T₂=T₁/√8=9.9 days. But this is the time for this very eccentric orbit to complete a complete orbit, go back out to where it was dropped from; so, the time we want is half that time, 4.9 days.

But this is not what you really wanted since I have treated the earth and the moon as point masses. What you really want is when the two point masses are separated by a distance of the sum of the earth and moon radii, 6.4x10⁶+1.7x10⁴≈6.4x10⁶m. To see how much error this causes, I can use the equation for the velocity v at the position r=6.4x10⁶ m if dropped from r=R_moon-orbit=3.85x10⁸ m which I derived in one of the earlier answers: v=√[2GM(1/6.4x10⁶-1/3.85x10⁸)]=1.1x10⁴ m/s. It would continue speeding up if the collision did not happen, but even if it went with constant speed the time required would be about t=R/v=6.4x10⁶/1.1x10⁴=580 s=9.6 min. This is extremely small compared to the 4.9 day total time, so, to at least two significant figures, 4.9 days is the answer to your question.

An important part of doing physics, or any science, is knowing when to eliminate things which are of negligible importance!

QUESTION:
If our solar system was formed from a cloud of dust, created by numerous super nova explosions, where we get various elements, and the sun is comprised of the greater part of this cloud, how is it that the sun is comprised of hydrogen burning to make helium? How did the sun separate all the other elements?

ANSWER:
Again, I am not an astronomer/astrophysicist. However, I was just reading in the April 2014 Scientific American an article about stars early in the universe. When the universe was young, it was composed almost entirely of hydrogen with only a tiny amount of helium and lithium. When stars form, they contract gravitationally and heat up as they do so. But, heating up, the pressure increases and keeps the star from collapsing further until it can shed some of the heat so that it can continue contracting. Eventually, a core dense and hot enough forms where the fusion can ignite. It turns out that hydrogen is not very good at getting rid of the heat and so more and more hydrogen accumulates; eventually ignition occurs but the typical early star, because of the inabilty of hydrogen to cool, is hugely bigger than the sun, anywhere from 100 to 1,000,000 solar masses. These giant stars now eventually die after burning much hydrogen and creating lots of heavier elements up to iron; they explode in supernovae and fall back into black holes, scattering the heavy elements (including heavier than iron made in the supernovae) into space. Now, to answer your question, the sun has lots of the heavier elements in it, just a smaller fraction than the planets. It turns out that these heavier elements are much more effective in cooling the protostar as it is forming which allows much smaller stars like the sun to form.

QUESTION:
Would it be possible to suspend an electron via electrostatic levitation in a uniform magnetic field? And if the voltage was decreased enough so that the electrostatic force on the electron was lower than the force due to the electron's weight would the electron then experience a 'fall' due to gravity?

I'm asking this because I'm wondering if placing a positron in a uniform magnetic field (in a vacuum) and lowering the voltage to a very small value so that the electrostatic force is lower than the weight would cause a positron to also experience a 'fall'. And if it did experience a fall it could be ascertained whether it would fall upwards or downwards.

I'm 17 years old and in the UK about to study physics at university in september and was just curious about this since one of our topics this year was electrical phenomena and we talked about millikan's oil drop experiment which featured a similar sort of suspension when the electrostatic force and force due to gravity were balanced.

It's a thought I had when wondering if antimatter was affected by gravity the same way matter is.

ANSWER:
It is always nice to see young folks asking interesting questions. First, I need to correct one thing: everywhere you refer to a "magnetic field" you should say "electric field"; a magnetic field exerts no force on a charge at rest which is what you want to observe, la Millikan. Now, it is known to extraordinary precision that the inertial mass of a positron is equal to the inertial mass of an electron. By inertial mass I mean the ratio of the force you apply to it divided by its acceleration, in other words its resistance to being accelerated. (A more correct way, relativistically, to say this would be that they have equal momenta for equal speeds.) I believe it is true that nobody has ever "weighed" a positron by measuring the force it experiences in a gravitational field. But, if the gravitational mass were different from the inertial mass, this would fly in the face of the theory of general relativity. But, let's talk about the feasibility of your experiment. The mass of an electron is about 10^-30 kg so its weight would be about 10^-29 N (taking g≈10 m/s²). The electron charge is about 1.6x10^-19 C and so the electric field required to levitate an electron would be 10^-29/1.6x10^-19≈6x10^-11 V/m. Suppose we use a parallel plate capacitor to create this field. The charge density σ on a plate with field E is about σ=ε₀E≈10^-11x6x10^-11=6x10^-22 C/m²which would correspond to an electron density on the plates of about 6x10^-22/1.6x10^-19≈0.004 electrons/m²! This would correspond to about one electron for every 250 square meters! That would not give a very uniform field would it? There is no such thing as a uniform surface charge density because charges in nature do not comprise a continuous fluid; so really tiny uniform fields are not possible. I did all that just for the fun of it, but there is an even more serious consideration the earth itself has an electric field near the surface of typically 100 V/m pointing down, so an electron would be repelled upward. To do your experiment you would have to get rid of that field and I do not believe that it would be possible to be assured that you could make the residual field much less than your 6x10^-11 V/m. Back to the drawing board! Keep asking those hard and interesting questions, though, and good luck with your university studies.

ADDED NOTE:
A recent article discusses a new proposal to compare matter and antimatter weights.

QUESTION:
If there is a planet on the opposite side of the sun, directly across from the earth, rotating at the exact speed as the earth, could it go unnoticed?

ANSWER:
Certainly not in this day and age when we have space probes all over the solar system. It seems that in earlier times such a planet would be detectable only by its gravitational effects on other bodies, for example comets or asteroids, which have orbits which cross earth's orbit.

QUESTION:
I'm a Science Olympiad coach trying to optimize the performance of our "Scrambler", a car which must be accelerated by only a falling mass. Most competitors simply tie a weight to a string and route that string over a set of pulleys (using no mechanical advantage to convert the vertical falling acceleration horizontal. Read a whole lot more!

ANSWER:
Sorry, but if you read site groundrules you will see that "concise, well-focused questions" are required.

FOLLOWUP QUESTION:
I was hoping you'd like the challenge of a motion/force problem that must span across several formula -- PE, KE, PEspring, velocity solved by acceleration and distance only, etc. Something to sink your teeth into...

ANSWER:
It is really not that interesting to work the whole thing out, but on second thought it is interesting to talk qualitatively and generally about the questioner's proposal; so I will do that. I will summarize the situation since I am sure none of you loyal readers will want to read the whole original question. By using a falling mass M attached to a car of mass m, it is wished to maximize the speed v of the car for M having fallen through some some distance H. The car moves only horizontally. The simplest thing to do is to have the two simply attached by a string over a pulley. Then, using energy conservation, 0= (M+m)v²-MgH or v=√(2MgH/(M+m)). What the questioner proposes is to hold the car at rest and insert a rubber band in the string so that the falling weight stretches the rubber band which has been carefully chosen to be just right that, when M has fallen H, it has just come to rest and is held there. Now, presumably, the rubber band has a potential energy of MgH. If the car is now released, the rubber band will presumably contract back to its original length giving its potential energy to the car, MgH= mv²or v=√(2MgH/m), a considerable improvement. My suggestion would be to use a spring rather than a rubber band since a rubber band has much more damping (energy loss due to internal friction) and hysteresis (will not return to its original length). Since the rules fix M and H, one obviously wants m to be as small as possible.

QUESTION:
What is the moon's orbit around the earth? I was wondering if you could send me pictures and diagrams or whatever you could about the orbit.

ANSWER:
I am not sure what you want. The moon has a nearly circular orbit of radius about 385,000 km. It takes about 28 days to go around earth once (which is how it came to be that a month was a standard time measurement). The same side of the moon always faces earth which means it also takes about 28 days to rotate once on its axis. The picture above is drawn to scale. For more detailed information, see the Wikepedia entry.

QUESTION:
Why is charge a scalor quantity if different charges are given positive and negative signs and also if the flow of current which is mainly due to the flow of electrons but has direction,by convention,same as that of protons??

ANSWER:
What makes you think that a scalar quantity cannot have a sign? How about Celsius temperature, -40⁰C or +20⁰C? How about time, -5 s is 5 seconds before t=0. The fact that electrons have negative charge is just an accident of history. All important aspects of electromagnetism would be just the same if you called the electron charge positive. The reason that current is defined to flow in the direction of positive charges has to do with the definition of current density which is a vector quantity.

QUESTION:
What is the size of an image as a function of its constant velocity ? i.e. what is the percentage increase of the image of a square travelling at 1 m/s towards a fixed camera? Is there some equation to calculate this?

ANSWER:
Since you do not give any details about the camera, I assume it has a fixed focal length f. I will call the size of the object L, the size of the image h, and the distance from the camera to the object R. You wish to relate the rate of change of h, u=dh/dt, to the rate of change of R, dR/dt=-v where v is the speed the object is approaching the camera; note that if the object is approaching the camera, R is decreasing, i.e. dR/dt<0. (If you do not know calculus, you will not understand my work here but you will end up with a formula for the rate at which h changes.) Because of the geometry of the situation (shown to the left) we can write L/R=h/f or h=Lf/R. Differentiating, dh/dt=-Lf(dR/dt)/R² or u=Lfv/R². This is the rate at which h is changing. Suppose that f=5 cm=0.05 m, v=1 m/s, L=10 cm=0.1 m, and R=2 m; then h=0.05x0.1/2=0.0025 m=2.5 mm and the image is growing at the rate of u=1.25 mm/s. After 1 s, the object has moved 1 m and so now h=0.05x0.1/1=0.005 m=5 mm and is now increasing at the rate of 5 mm/s. So, you see, the rate the image increases in size depends not only on v but also on how far away the object is (R) and how far the image is from the lens (f). If you need to know what h is as a function of t, you need to also know where the object was at some earlier time which I will call t=0; then R₀=R(t=0) and so R(t)=R₀-vt. Finally, we can write h(t)=Lf/R(t)=Lf/(R₀-vt). For the example above and choosing R₀=2 m, the graph on the right shows the image size as the object approaches the camera; note that both the size and the rate of growth approach infinity as R approaches zero (t approaches 2 s).

QUESTION:
If you have a glass tube with an object inside and the tube contains a vacum, why can you see the object in the tube and why doesnt the inside of the tube go dark like outer space. What are the photons interacting with?

ANSWER:
The air has almost no effect on light light entering your tube behaves almost as if the air were not there. In space, the vacuum itself is not dark and transmits light just fine. What is dark is the "sky" because although the air on earth has almost no effect on light, a very tiny fraction of light does scatter from the air and, because the sun is so intense, the whole sky is brightened by our seeing this scattered light.

QUESTION:
If an infinite number of bullets were fired from a gun at a solid target, would one of those bullets "pass through" without making a hole/dent ?

ANSWER:
No. See earlier answers.

QUESTION:
If the speed of light is constant for all observers, then why does the Doppler effect for light take place?

ANSWER:
Because light frequency is a clock subject to time dilation and light wavelength is a distance subject to length contraction. Because of the constancy of the speed of light, however, the Doppler effect for light is independent of whether it is the source or the observer who is moving, unlike the situation for sound.

QUESTION:
How far apart must electrons be from one and other before they stop exchanging photons or does that only happen when atoms are sharing electrons in the same valence shell (assuming my question has a basis - I have no formal physics background, just what I read in places like this great site and others!)

ANSWER:
Photon exchange should not be taken too literally. It is a cartoon attempting to demonstrate how the field quanta (photons) convey the force (electromagnetism). And, it has nothing to do with whether or not the electrons are sharaing some shell. Any time charges are in an electric field there are virtural photons. Regarding the distance, there is theoretically no cutoff distance where "they stop exchanging photons" because the electric field from a single electron extends all the way to infinity.

QUESTION:
Without having wings, how does a helicopter turn in air?

ANSWER:
First of all, a helicopter does have wings. The rotor is shaped like a wing and moves through the air by spinning thereby creating lift. Imagine the path of the overhead rotor as a disk. The rotor is connected to a plate called the swashplate (the thing between the rotor and the fuselage in the picture) which can be tilted relative to the main shaft to the motor and which causes the disk of the rotor to tilt relative to the horizontal plane. Tilt it forward and the helicopter goes forward, to the right and it goes right, etc. The swashplate is controlled by a joystick in the cockpit called the cyclic. The tail rotor is also used in turns, controlling yaw (rotation about a vertical axis); yaw is controlled with foot pedals.

QUESTION:
Suppose there are 2 mirrors facing each other and I light a laser perpendicularly to one of the mirror and instantly remove my hand . Will the light beam continue striking from one mirror to the other?

ANSWER:
See an earlier answer.

QUESTION:
How do we convert electron volts to wavelength or vice versa, example: the wavelength of 50 KeV x-rays?

ANSWER:
The energy of a photon is E=hν=hc/λ where h=4.14x10^-15 eV∙s is Planck's constant, c=3x10⁸ m/s is the speed of light, ν is the frequency, and λ is the wavelength. So, E=1.24x10^-6/λ eV or λ=1.24x10^-6/E m.

QUESTION:
Does acceleration have momentum? In other words if you fire a rifle, does the highest velocity of the bullet occur as it exits the barrel or does the acceleration increase after it leaves the barrel?

ANSWER:
In terms of physics nomenclature, your first question has no meaning. But your second question seems to clarify what you mean: if something has an acceleration does it keep accelerating even if there are no forces on it? The answer is an unequivocal no. The only thing which causes acceleration is force and when the bullet exits the barrel of the gun the force which was accelerating it disappears. If there were no new forces on it, it would continue with the same velocity it had when it exited. There are, however, two important forces on the bullet when it is outside the gun gravity and air drag. Gravity causes it to accelerate toward the ground and air drag causes it to slow down.

QUESTION:
I would like to know if amplitude is a scalar or vector quantity. The definition of amplitude of a wave is written as the maximum displacement of a point from the rest position but why doesn't the calculated amplitude have a plus or minus sign before the magnitude? If we have a type of sound waves that have a maximum negative displacement of 5 cm and a positive displacement of 3 cm, what is then the amplitude of the wave?

ANSWER:
Amplitude is a positive-definite scalar and is defined as "the maximum displacement or distance moved by a point on a vibrating body or wave measured from its equilibrium position". In the example you cite, amplitude is 5 cm. For a traveling wave, there is one amplitude of the wave; for a standing wave, each point in the wave has a different amplitude ranging from zero (nodes) to some maximum value (antinodes). A closely related quantity is the root mean square (RMS) displacement where displacement is squared, averaged over one cycle, and then the square root taken.

QUESTION:
We have a metal ruler 1 yard in length. If held in exact balance center then "pinged" it causes vibrations. nothing new there BUT at exact equal distances to either side there is a point at which it appears that the vibrations stop (a calm spot) for about 1 inch then the vibrations start again and continue to the end. We've tested and the vibrations don't stop, they are just a much smaller wave length. What is is this "calm spot" phenomenon? What causes it? Does it happen with earthquakes too? Really geeked out about this! Way cool!

ANSWER:
You are exciting standing waves when you "ping" the stick. These are waves which bounce back and forth from the ends of the stick and, for special wavelengths, are just right to to resonate like a guitar string or an organ pipe. The various wavelengths for which resonance occurs are called the modes of oscillation. For a stick clamped at the middle, the lowest mode, called the fundamental, has approximately 1/4 of a wavelength on either side of the center as shown by the upper part of the figure above. A point with zero amplitude, the center for the fundamental, is called a node and points with maximum amplitude, the ends for the fundamental, are called antinodes. What you are seeing is the next mode, called the first overtone, which has approximately 3/4 of a wavelength on either side; this mode has three nodes and four antinodes. To the right are animations for a stick clamped at the end but they are exactly what your stick is doing on one half. Here the nodes are near the darkest blue. Earthquakes are traveling waves and therefore do not have nodes.

QUESTION:
I have points A, B, and C which are moving in 3 dimensional space. If I use point A as a frame of reference, I know the direction and velocity of points B and C. If my points are moving arbitrarily close to the speed of light, what equations would I use to calculate the relative velocity of C from B's frame of reference, and the difference in clock speeds between those two points? I understand how I would do this if the speeds were nowhere near the speed of light, but things get a tad complicated after accounting for relativity.

ANSWER:
I have previously dealt with relativistic velocity addition if the velocities of B and C are colinear: in your notation, V_CB=(V_CA+V_AB)/(1+(V_CAV_AB/c²)); read V_XY as velocity of X relative to Y. This may be generalized to noncolinear motion as V_CB=(V_CA+V^‖_AB+γ_CA^-1V┴_AB)/(1+(V_CA∙V_AB/c²)) where V^‖_AB and V┴_ABare the components of V_AB parallel and perpendicular, respectively, to V_CAand γ_CA^-1=√[1-V_CA²/c²]. Note that if the speeds are small compared to c, V_CA∙V_AB/c²≈0 and γ_CA^-1≈1 so V_CB≈(V_CA+V^‖_AB+V┴_AB)=(V_CA+V_AB).The reason that the transformations are different for parallel and perpendicular motions is that there is length contraction for the parallel motion but not for the perpendicular motion. (Note: V_XY=-V_YX.)

QUESTION:
I've been reading about rotational space habitats for a while now and haven't found an answer to this question by googling. So how would a space habitat be rotated? By cogs? By propulsion systems?

ANSWER:
If you want to read more about the details of such habitats, see my earlier answers (1 and 2). In answer to your question, you would need thrusters to get the ring spinning and then to occasionally correct minor changes, but once it was spinning, it would continue to spin just the same forever if there were no external torques on it as would be the case in empty space.

QUESTION:
The moon's gravity is one sixth that of the earth. Thus if you kicked a box with a force of 60 N across a frictionless floor on earth, the box would travel the same distance in 1 second as when the same box was placed on a frictionless floor on the moon and kicked with a force of 10 N. Am I wrong?

ANSWER:
You are wrong on both your conclusion and on your " kicked with a force of " premise. First the premise: you need to go the FAQ and read the link from the question about how much force does it take to make something move with some speed. Just knowing the force you cannot know the resulting speed; you need either how long the force was applied or over what distance it was applied. Now, your question implies that you think it will be easier to get the box on the moon moving with the same speed as a box on earth with that speed. But, in fact, the box has the same mass on both the earth and moon and you are not lifting it against gravity, so it is equally easy to move a box horizontally on earth or the moon. It is six time harder to lift a box on earth as on the moon. If there were friction, however, it would be harder to move the box on earth than on the moon because the frictional force on the moon would be six times smaller.

QUESTION:
I know that neutrons is placed in a wax container to contain the neutrons, but why is this an effective way to contain the neutrons. I want to take in account the linear momentum and the energy, but I'm not sure how to begin or where to do the research?

ANSWER:
The wax does not "contain" the neutrons. Most neutron sources yield fast neutrons and most uses for neutrons need slow (called "thermal") neutrons. Thus the source is encased in a block of paraffin which serves as moderator, i.e. the paraffin slows the neutrons down. The reason that paraffin is good is that it is a hydrocarbon and has lots of hydrogen in it. Hydrogen is good to slow down neutrons because the best way to slow down a fast moving object is to collide it with an object at rest which has about the same mass (think of a head-on collision of two billiard balls); hydrogen has about the same mass as a neutron.

QUESTION:
How do CDs create rainbows when we shine white light on them?

ANSWER:
Because they have many closely spaced lines which act like a diffraction grating.

QUESTION:
What is the difference in time dependent and time independent Schr dinger equations?

ANSWER:
The solution of the time-dependent equation is the wave function as a function of space and time; the solution to the time-independent equation is the wave function as a function of only space.

QUESTION:
why does a piece of chalk produce hideous squeal if you hold it incorrectly?

ANSWER:
It is caused by friction. If the conditions are right (holding it incorrectly), the chalk will stick, slip, stick, slip, stick, slip very rapidly, hundreds or thousands of times per second. This results in a resonant response of the chalk itself, vibrating loudly. The same slip-stick friction is responsible for how a violin bow works.

QUESTION:
How can the speed of light be the fastest thing in the universe if it is a relative measurement? I mean that if you had two light particles going in opposite directions, wouldn't one go twice the speed of light relative to the other?

ANSWER:
See my FAQ page. It is a relative measurement, but your idea of the relative velocities is incorrect at large speeds. You want to use v'=(u+v), but the correct expression is v'=(u+v)/[1+(uv/c²)] where c is the speed of light; notice that if u and v are both small compared to c, your expectation is very nearly correct. For your particular example, u=v=c and so v'=(c+c)/[1+(c∙c/c²)]=c. You can also find other answers about light speed, the maximum velocity possible, etc. on the FAQ page.

QUESTION:
I read a question on your site about using magnets to propell a spacecraft. I want to expand on that question. How would it affect the movement of the ship if we'd replace the bar magnets with electric magnets and then make one magnet stronger than another? Shouldn't the direction with weaker magnet start to move since the the weaker magnet can not completely nullify the effects of the stronger magnet?

ANSWER:
Newton's third law always applies: if one magnet exerts a force on the other, the other experiences an equal and opposite force from the one. All forces interior to the spaceship must add up to zero.

QUESTION:
I am confused about 1/2mv^2 and mgh. If an object is lifted vertically up at a constant speed, the formula is mgh. But wouldn't the object also gain k.e. too due to the constant velocity? So wouldn't the total energy be mgh+1/2mv^2?

ANSWER:
If it is still moving when you get to h, then its energy is indeed mgh+ mv². In a textbook, we usually envision the mass being lifted from rest at one location to being at rest at some higher location. During this process, the total amount of work you do is mgh and the total amount of work the weight (gravity) did is -mgh so the total work done is zero and the change in kinetic energy is thus zero.

QUESTION:
If you have two permanent cylindrical magnets (the kind with a hole in the center) and you stack them with poles opposite on a pencil, the top magnet will "float" above the bottom magnet. Energy is being expended to keep the magnet "up" the pencil. Where is the energy coming from? The bottom magnet will be pushing down with an equal but opposite force, but that does not cancel the energy needed to float the top magnet as far as I can see.

ANSWER:
I am afraid you do not understand energy. The lower magnet exerts a force on the upper magnet. The force holds it there in equilibrium, it does not require energy to hold it there. It is no different from saying that if one of the magnets were hanging from a string, where does the energy to hold it there come from? Or, if one of the magnets were sitting on a table top, where does the energy to hold it there come from?

QUESTION:
I read of "gravity assist" swingy-bys of Jupiter to speed a spacecraft up to reach the outer planets. As the spacecraft approaches Jupiter, it speeds up. But it retreats from Jupiter on a symmetric path (a hyperbola I think) and Jupiter will therefore slow the spacecraft down by the same amount on the outbound path. It appears there should be no net increase in speed, just a bending of the spacecraft path. But bending a spacecraft path also takes energy. So Jupiter is providing the energy in some manner though it is unclear to me how.

ANSWER:
The trick is that the planet, with much greater mass than the spacecraft, is moving in its orbit and the boost comes from using the speed of the planet to speed up the spacecraft. The figure shows the idealized one-dimensional interaction with planet; because the mass of the spacecraft is much less than the mass of the planet, the spacecraft picks up twice the speed of the planet. For those who have studied elementary physics, this should look vaguely familiar: a perfectly elastic collision between a BB at rest and a bowling ball with speed U results in the BB going with speed 2U and the bowling ball still going U. (Of course, this is only approximately true if the mass of the BB is much smaller than that of the bowling ball; the bowling ball will actually lose a very tiny amount of its original speed.)

QUESTION:
The number of known Mars Meteorites on Earth at last count I know of was greater than the number of Lunar meteorites. This is the opposite of what you would expect: The moon is 140 times closer than Mars with a weaker gravitational field to recapture the meteorites and no atmosphere to slow material blasted off the planet. The number of Lunar meteorites on Earth should be hundreds of times greater than Mars Meteorites.

ANSWER:
As always, a disclaimer that I am not an astronomer. The origins of either lunar or Martian meteorites are major asteroid or comet impacts; after impact, some of the debris has enough velocity to escape the gravitational field. First of all, the cross sectional area of the moon is about 4 times smaller than Mars, so all things being equal, the probability of a major impact is 4 times greater for Mars. Second, Mars is closer to the asteroid belt and would therefore, I presume, be more likely to suffer an asteroid impact. Most major impacts occurred early in the history of the solar system when embryonic planets were "sweeping up" material in their orbits and there was a much greater potential of major impacts. These early meteorites from the moon which landed on earth would have been eroded away or taken under the crust by tectonic action; those from Mars would have gone into orbits around the sun and land on the earth over a much longer period, probably still landing today.

QUESTION:
My son and I are trying to build a Foucault pendulum. We have 11' ceilings so we need to dampen ellipsoidal motion and provide a drive mechanism to keep the pendulum moving. I've seen pendulum driver circuits that operate via magnetic induction with relays, transistors, etc. but do not prefer these; it's not clear that the pendulum and Earth are doing the magic, as opposed to the electronics. I'm thinking of the following design: bend a 20' steel 1" conduit into a circle. Then wrap say 16 gauge bare copper wire evenly and entirely around the doughnut creating a precision ring shaped electromagnet. Then power the ring shaped magnet with a variable DC supply to create the proper pull on, say, a 6lb steel pendulum that swings inside the ring. The idea is to adjust the voltage to give the electromagnet enough pull to keep the pendulum swinging, but not so much that it overcomes the force of gravity pulling the pendulum back to the center, thereby sticking the bob to the electromagnet. That solves the problem of keeping the pendulum moving.

ANSWER:
After thinking about this a bit, I believe there are serious flaws here and your idea will not work. If you put a ferromagnetic material (e.g., your pendulum bob) in a uniform external magnetic field, that field will polarize the material and essentially make it look like a bar magnet with north and south poles. The south pole will feel a force opposite the direction of the field and the north pole will experience the same magnitude force in the direction of the field a bar magnet in a uniform field experiences zero net force (see left figure above). Admittedly, the field due to your ring (see figure at the right above) is not uniform but, over the size of your bob, it is approximately uniform. Forces due to the small nonuniformity would be mainly in the vertical, not horizontal, direction.

QUESTION:
the bond between carbon and oxygen atoms in a carbon dioxide molecule behaves like an elastic spring and vibrates the molecule.why?

ANSWER:
It is easier to just talk about a diatomic molecule like O₂. When you understand that you can generalize to any molecule. Think about the forces between two atoms. If you try to pull it apart, this force tries to pull it back together or else it would not be a molecule; and, since the molecule has a size larger than the size on one atom, if you try to push the atoms together, the force tries to push them back apart. This is exactly the way a spring works and so a reasonably good model for a molecule is that all the atoms are attached by tiny springs. Of course, they are not but this still is a model which can help you understand many molecular properties. So, one of the ways you can excite (add energy to) a molecule is to get it vibrating.

QUESTION:
why does the ground frost go deeper when the surface begins to thaw?

ANSWER:
The way you frame this question is that frost has gone to certain depth before the thaw and then goes deeper because of the thaw. In fact, there is what is called a frost front, the line between frozen and unfrozen soil, which moves slowly downward if the air temperature is below freezing. When the air temperature goes above freezing, the top thaws but the frost front continues moving down for a while because there is still a frozen region above it which prevents it from getting the information that the air has warmed. So, the thaw does not cause the frost to deeper, it is just continuing what it was already doing. Eventually the thawing will reach the frost front and all the soil will be thawed; in some arctic and antarctic regions, the thaw will never reach all the way to the frost line and permafrost results.

QUESTION:
If an elementary particle has a mean lifetime of say 1 second and there are 1 trillion particles in the system then about how many particles will be left after say 100 seconds?

ANSWER:
See a recent question and then do the calculation: N=N₀exp(-t/τ)=10¹²exp(-100)=3.7x10^-32. Although there is a nonzero probability (3.7x10^-30%) that there will be a particle left, for all intents and purposes there are none left. (Incidentally, there are two meanings of the word "trillion". In the US it means 10¹² but in some countries it means 10¹⁸. Look here for more detail.)

QUESTION:
During a discussion with my 6th grade class about the Law of Inertia and space travel, a student asked: "If a spacecraft leaves a solar system, for example Voyager 1, will its velocity increase due to the lack of gravity from the sun." Fairly certain the answer is no; however, it did spark a rather interesting debate. Can you explain?

ANSWER:
Perhaps the key is to note that "leaves the solar system" does not mean that there is no longer any gravity from the sun. Rather there is a boundary where the solar wind, particles like protons and electrons, stops; this is called the heliopause and has been definitely observed by the Voyager 1 spacecraft as shown in the graph to the right. This shows the amount of solar wind the Voyager detected as it moved during the months of 2011-2. In September 2012 it dropped to near zero. This is where we define the edge of the solar system to be and it is about 50 Astronomical Units (AU) from the sun; the earth is one AU from the sun. But the gravity from the whole solar system is still present and the craft will continue slowing down, but ever so slightly since as you get farther away from a mass the gravity gets weaker. This craft has enough speed that, if it never encountered any other mass it would keep going forever. I did a rough calculation and found that the acceleration of Voyager is about a=-0.01 mi/hr² which means that it loses about 1/100 mph per hour; but the speed is about 40,000 mph, so I think we could agree that it is moving with an almost constant speed. As it gets farther away, the acceleration will get even smaller (physicists call slowing down negative acceleration, not deceleration). Until it gets close to something else, like some other star, it will keep going with an almost constant speed. When it does approach another star it will begin speeding up. Your students should appreciate that the only thing which can change the speed of something is a force, a push or a pull.

QUESTION:
I am building a cold frame to keep veggies alive in the winter. It will be 3' x 6' with two "doors" (called lights) each 3' x 3' that will be hinged to the frame. The frame probably weighs about 10 - 15 lbs. The doors will be quite lightweight, possibly only 3lbs each. I wanted to use magnets to keep the doors closed at night or when I am not venting the cold frame. We often get very windy days with 40mph wind speeds and gusts to 60 mph. From what I've read, magnets have different "pull force" properties. I'd like a way to figure out what pull force the magnet for each door needs to have to withstand the winds we get. Please don't tell me to just hook the doors closed -- shockingly, it appears magnets are a more cost effective solution.

ANSWER:
I must say that I cannot believe that you could not buy a couple of simple hooks/latches for under $5, but I will do a rough calculation for you to estimate the force you would need to apply at the edge of the doors to hold it down in a 60 mph=26.8 m/s wind. When a fluid moves with some speed v across a surface, the pressure is lower than if it were not moving; this is how an airplane wing works and why cigarette smoke is drawn out the cracked window of a car. To estimate the effect, Bernoulli's equation is used: ρv²+ρgh+P=constant, where ρ is the density of the fluid (ρ_air≈1 kg/m³), P is the pressure, g is the acceleration due to gravity, and h is the height relative to some chosen h=0. For your situation both surfaces are at essentially the same height so P_A=P+ ρv²where the pressure inside your frame is atmospheric pressure (P_A) and the velocity inside is zero. So, P_A-P=ΔP= ρv²= ∙1∙26.8²=359 N/m²=7.5 lb/ft². This would be the pressure trying to lift the door. So the total force on each door would be F=AΔP=9∙7.5=67.5 lb where A=3∙3 ft²=9 ft² is the area of the door. But, this is not the answer since we want to keep it from swinging about the hinges, not lifting into the air. So, assuming that the force is distributed uniformly over the whole area, you may take the whole force to act in the middle, 1.5 ft from the hinges, so the torque which is exerted is 1.5∙67.5≈100 ft∙lb. But, the weight of the door also exerts a torque, but opposite the torque due to the wind (the weight tries to hold it down) -3x1.5=-4.5 ft∙lb. So, the net torque on the door about the hinges is about 95 ft∙lb. To hold the door closed, one needs to exert a torque equal and opposite to this. To do this, it would be wisest to apply the force at the edge opposite the hinges to get the maximum torque for the force. The required force from your magnets would then be F=95/3=32 lb. Note that this is just an estimate. Fluid dynamics in the real world can be very complex. Also note that, if my calculations are anywhere close to correct, you should probably be sure the whole thing is attached to the ground or the side of your house since the total force on the whole thing would be 67.6+67.5-3-3-15=114 lb, enough to blow the whole thing away in a 60 mph wind! Also, once the door just barely opens, the wind will get under it and simply blow it up, Bernoulli no longer makes any difference.

QUESTION:
If a particle has a lifetime of say 100 years then what are the chances that that particle will decay in 1 year. How will one go about calculating particle decay probability?

ANSWER:
Lifetime has no meaning for a single particle. It is a statistical concept as I will show. The rate at which a large ensemble of particles decays is proportional to how many particles there are, dN(t)/dt=t/τ where N(t) is the number of particles at some time t and τ is the mean lifetime. The solution of this differential equation is N(t)=N₀exp(-t/τ) where N₀=N(t=0). So, when t=τ, N=0.37N₀, there are 37% of the original particles left. For your question, you might want to ask what is the situation when t=1 if N₀=1 and τ=100: N=exp(-0.01)=0.99. If you want to interpret that as a 1% probablility that the particle has decayed, I guess that that would be ok. But, of course, there is no such thing as 99% of a particle. Keep in mind, though, that this is not linear; for example, if t=50=τ/2, N=exp(-0.5)=0.61, not 0.5.

QUESTION:
where does electron go that created by beta emission?

ANSWER:
The electron is not "created" in a vacuum, a proton and a neutrino are also "created" and a neutron "destroyed." The net effect is that there is still the same amount of charge, zero, after the decay as there was before. One possibility is that the electron never leaves the source and, since the atom it left is now positively charged, the electrons in the source all move around until all the atoms have the correct number of electrons. Or, if it leaves the source, it will encounter other matter with which it will interact, lose energy and either find a positive ion somewhere to join or stick to a neutral atom which becomes a negative ion. Charge can build up to some limit on anything.

QUESTION:
Galileo was punished by the Church for teaching that the sun is stationary and the earth moves around it.His opponents held the view that the earth is stationary and the sun moves around it.If the absolute motion has no meaning, are the two viewpoints not equally correct or equally wrong? i.e, By the concept of relative motion can't we say both?if so, then why do we usually say that earth goes round the sun,and the other way round?

ANSWER:
If the sun and earth were both just moving with constant velocity and not interacting in any way, you would have a point. However, because of their gravitational interaction and the fact that the mass of the sun is hugely bigger than the mass of the earth, there is no way you can sensibly argue that the sun orbits the earth. Each exerts a gravitational force on the other (equal and opposite) but because the sun is so massive, the force has almost no effect on it. If the earth were not orbiting but simply released from rest, it would fall into the sun. There would be no question which object had the greater acceleration it would be obvious to any observer that it was the smaller mass, the larger mass practically unaffected. So, if any frames are accelerating, they are not equivalent to those not accelerating. What you call "the concept of relative motion" applies only to unaccelerated frames.

QUESTION:
Two spherical bobs, one metallic and other of glass, of same size are allowed to fall freely from the same height above the ground. Which of the two would reach earlier and why?

ANSWER:
Two objects with identical geometries (same size and shape) experience identical air drag force while moving with a speed v. While falling, the force has a greater effect on the less massive causing it to slow down more. Therefore the mass, not whether glass or metal, determines which gets to the ground first; the winner is whichever has more mass. You can find links to many old answers about air drag on the faq page.

QUESTION:
Suppose you have radiation detectors fixed on the ground on Earth. Will they detect radiation coming from a charged particle in free fall near them? The first answer that comes to mind is: Yes, they will detect radiation because the particle is accelerated, and electrodynamics predicts that accelerated charges must radiate in this situation. According to the Equivalence Principle, this situation is equivalent to detectors fixed on an accelerated rocket with acceleration g moving in the outer space and far away from the influence of other bodies. If the answer to the previous question is yes, then the detectors on the rocket should also detect radiation coming from a charge in free fall as observed by the reference frame of the rocket. But a charge in free fall in this reference frame is at rest in the inertial reference frame fixed with respect to the distant stars, and a charge at rest in an inertial frame should not radiate. Is it possible that detectors fixed on the rocket detect radiation but detectors at rest in the inertial frame do not? Is radiation something not absolute, but relative to the reference frame?

ANSWER:
This is a fascinating question and points to an experiment which would seemingly violate the equivalence principle. The answer to your first question is an unequivocal yes, an electric charge accelerating in free fall in a gravitational field radiates electromagnetic waves, an electric charge not accelerating does not radiate. But, suppose that you are falling along with the charge; relative to you the charge is not accelerating and therefore not radiating. Or, equivalently, suppose that you are in a spaceship in empty space with your rockets turned on. If you release an electric charge inside, it will "accelerate" toward the rear of the ship and therefore radiate because the equivalence principle states that there is no experiment you can perform which can distinguish between the accelerating frame and a static gravitational field. However, the charge will move with constant speed relative to an inertial observer nearby and therefore not radiate. In both cases we have an electric charge both radiating and not radiating, a seeming paradox. Although I had not heard of this paradox before, apparently it has been a topic of many articles. The most recent of these, by Almeida and Saa, has evidently laid the paradox to rest. They demonstrate in this article that observers for whom the charge is not accelerating "…will not detect any radiation because the radiation ﬁeld is conﬁned to a spacetime region beyond a horizon that they cannot access…" and "…the electromagnetic ﬁeld generated by a uniformly accelerated charge is observed by a comoving observer as a purely electrostatic ﬁeld." Like all "paradoxes" in relativity, there is not really a paradox; rather a radiation field in one frame may be a static field in another. Basically, you nailed it when you said "radiation [is] something not absolute, but relative to the reference frame."

QUESTION:
What would happen if a needle that weighed as much as the Earth and everything on it was placed on the surface of the planet? Where would the pin end up? What would be the impact on the Earth? Would the outcome be different if it was set on land or water?

ANSWER:
This is a wacky question but I guess I can do a wacky question now and then. I will take "placed on" as being synonymous with "suddenly appears at the surface". It will also be easier to think about if the needle appears at the equator. I will also assume that no matter what happens, the needle retains its size and shape. If the needle just sat there, the effect would be that the earth would now rotate not about its axis but about a parallel axis which is halfway between the center of the earth and the needle; the length of the day would increase to about 5 times the current 24 hours. But, it would certainly not stay on the surface of it. The force which the needle would exert on the surface of the earth would be about 6x10²⁵ N. But this force is over an area of a needle, very tiny, so the pressure would be astronomical. This would cut into the earth and the needle would end up, probably after oscillating back and forth for a while, at the center of the earth. The length of the day would return to about 24 hours and everything on the surface would weight twice as much. If the needle were to appear at a pole, there would be no effect on the length of the day at all.

QUESTION:
If a dogs bark measures approximately 60-80 decibel, how much will it reduce traveling in air (average 20 Celsius) over 500 meter. Could you please brake down the formula for me so I can calculate other distances.

ANSWER:
You should first read an earlier answer for a detailed explanation of what a decibel (dB) is. The main reason for loss of sound intensity I (measured in watts per square meter, W/m²) is that the sound waves spread as they get farther away so the energy per second (power) striking your ear gets smaller. The intensity falls off like 1/R² where R is the distance from the source. You do not specify the distance from the dog that the 60-80 dB level is measured, so I will arbitrarily put it at 1 m. Therefore the ratio of intensities at 500 and 1 m would be I₅₀₀/I₁=1/500² or I₅₀₀=4x10^-6I₁. But, the catch is that dB is a measure of the level L (a logarithmic scale), not the intensity. So we need the equations to convert between L and I. These are L=10∙log₁₀(I/10^-12) and I=10^-12∙10^(L/10)where I is in W/m². So, as an example I will choose L=70 dB, so I₁=10^-12∙10^(70/10)=10^-5 W/m²and I₅₀₀=4x10^-6∙10^-5=4x10^-11 W/m². Finally, we find what the dB level of I₅₀₀is: L₅₀₀=10∙log₁₀(4x10^-11/10^-12)=10∙log₁₀(40)=16 dB. I believe that this will the main source of quieting with distance, not any absorption of the sound by the air. Wind can also have an effect on the intensity if it is across the direction the sound travels to reach you. By the way, when the intensity reaches 10^-12 W/m², it will be below the "threshold of hearing" and you will no longer hear it; that corresponds to 0 dB.

QUESTION:
My question is that whether gravitational field an be reversed in Earth`s atmosphere? it would bring revolutionary changes in Aerospace engineering.

ANSWER:
No.

FOLLOWUP QUESTION:
but space organisations train their astronauts on how to deal in zero gravity in camps. then they have to create artificial zero gravitational field. how is it possible??

ANSWER:
You can simulate zero gravity but you certainly do not do it by reversing the gravitational field. We are stuck with the field we have and to simulate zero field there are two ways. The first is to put the astronaut trainee in a free-fall situation. If you are in a freely falling elevator, for example, it will seem like there is no gravity. The more practical way to do this is to ride in an airplane which is moving over a parabolic path it would follow if it were simply a projectile; inside that airplane it will seem like there is no gravity. The plane which does this is affectionlately known as the "Vomit Comet". A second way you can simulate weightlessness is to build a giant tank of water and then the buoyancy will provide a force opposite to your weight making the net force on you equal to zero.

Finally, it should be noted that when you are in orbit you are also not truly "weightless". In orbit you are constantly in free fall so, although you still have your weight, you are in free fall just as you were on the Vomit Comet.

QUESTION:
If we are given a graph showing pressure versus distance for a sound wave, does a higher pressure amplitude indicates a louder sound produced?

ANSWER:
Sound waves are longitudinal waves of pressure as shown in the animation at the right. You are right that the greater the pressure difference between the lowest and highest pressures, the louder the sound. You should not think of the regions of high pressure being the regions of loudest sound, though. Because the elapsed time between low and high pressure hitting your ear is so short, your ear/brain averages the square of deviation of the pressure in the wave from normal atmospheric pressure to determine loudness. The information from the time difference is also processed by the brain and interpreted as the frequency or pitch of the sound.

QUESTION:
Let's say that we started mining for metals and such somewhere other than earth. What if any would the effects of large scale increase (and I mean really really big) in planet mass be? Also what would happen to the other planets we were robbing of minerals as their mass decreased?

ANSWER:
As long as the mass of the orbiting body is much less than the mass of the orbited body, the orbital motion is independent of the mass of the orbiting body. So, the length of the year would be unchanged. However, increasing the mass and radius of the planet would increase its moment of inertia, so its rotational speed would decrease and the day would get longer. Keep in mind, though, that a huge amount of stuff would have to be added for any real difference to occur.

QUESTION:
In case of string, when only a pulse is sent then why do the particles vibrate just above the mean position and not below the mean position? Please answer in a way that i could understand. If your answer include some mathematics esp the calculus please simplify so that i could understand

ANSWER:
There is an equation, called the wave equation, which to a fair approximation applies to waves on a string. One of the properties of this equation is that the wave retains its shape as it propogates. Hence, if you start with a pulse above the string, it will remain that way.

QUESTION:
I am trying to determine the buoyancy of a 10 kg polyethylene tray (density = 0.95 g/cm³=950 kg/m³) that is used in lobster pounds to hold lobsters. The tray holds 60 kg of lobster, and fills up with water up to the lid (which floats at the top of the water). The volume of the tray is 0.1223 m³. The lobsters are held in seawater (density = 1026 kg/m³). So, my question is how much air is required to be placed in cavities in the lid to keep the tray floating at the top of the water?

ANSWER:
I will take the "volume of the tray" to be the inside volume, that is the volume occupied by lobsters and water. The density of a lobster is sure to vary from animal to animal, but it certainly must be larger than the water since the creatures dwell on the bottom. I actually found a measurment of a lobster's density to be 1187 kg/m³, so I will use that as an approximation for all lobsters. So, the volume occupied by 60 kg of lobsters is V_lobsters=60/1187=0.0505 m³. So, the volume of water needed to fill up the tray is V_water=0.1223-0.0505=0.0718 m³; so the mass of the water is M_water=1026x0.0718=73.7 kg. The volume of the polyethylene is V_poly=10/950=0.0105 m³. So, the total mass is 60+10+73.7=143.7 kg and the total volume is 0.1223+0.0105=0.1328 m³. The density of the full tray is then ρ=143.7/0.1328=1082 kg/m³; since this is greater than the density of the water (1026 kg/m³), it will sink, hence presumably the point of this question. In order for it to "just" float, volume must be increased until the density is equal to 1026 kg/m³, i.e. 1026=143.7/V or V=0.1401 m³; so, the volume of the air should be V_air=0.1401-0.1328=0.0073 m³=7300 cm³. This would be a volume of a cube about 20 cm on a side. I guess I would increase that by at least 50% as a safety factor. (Note that I have neglected the density of the air which is about 1 kg/m³.)

QUESTION:
What happens to gamma rays when they are blocked by materials like lead, and how come they're not blocked by some other materials?

ANSWER:
For all intents and purposes, gamma rays interact only with electrons in the material. The two most important ways they interact are the photoelectric effect where the photon gives all its energy to an electron and Compton scattering where the photon scatters from an electron, giving some of its energy to the electron. Which of these is more important depends on photon energy and other factors. But, as you would think, the density of electrons in the material is very important. To a very rough approximation, atoms are all about the same size. That means that there are about the same number of lead atoms in a cubic centimenter of lead as there are aluminum atoms in a cubic centimeter of aluminum. But every lead atom has 82 electrons whereas every aluminum atom has 13 electrons. There are therefore roughly 6.3 times more electrons in a cubic centimeter of lead than in a cubic centimeter of aluminum; therefore lead will be much more effective in shielding against gamma rays. Regarding "what happens" to the gamma rays, they disappear completely if photoelectric effect occurs or become gradually less energetic and changed in direction if Compton scattered.

QUESTION:
To tighten the loose head of a hammer, the base of the handle is sometimes struck on a hard surface. Explain the physics behind this maneuver.

ANSWER:
This sort of sounds like homework. The physics is inertia. The head of the hammer is pretty massive and so it has a lot of inertia, it wants to keep going. When the handle suddenly stops, the inertia of the head keeps it moving so that it moves down a bit on the handle before stopping, thereby becoming more tightly bound to the handle.

QUESTION:
What would happen to a beam of proton particles if the pass through a coil (perpendicularly) imagine a closed circle shaped loop. Currwnt is passing through this coil hencr generating a magnetic field. Will this B field make the particles vibrate? Accelerate them?

ANSWER:
I assume that you have the beam of protons very narrow and moving along the axis of the loop. As you can see from the figure to the right, the on-axis field is along the axis. Therefore, a beam of protons on the axis would pass through unaffected because the force on a charged particle is zero if the velocity and field are parallel. If the proton were off axis, there would be a small force which, if the proton were in the plane of the page here, would point either into or out of the page.

QUESTION:
Isnt the gravitational pull of an object determined SOLELY on the mass of that object and NOT its size or density? obviously distance from said object plays a role just as size and density affect mass.... however, if what i believe is true, there can be no such thing as black holes, which even today their existence is only theoretical, not proven... anyway my point is this... i believe that if our sun was the size of a basketball but still had the same mass it has today, (giving it almost infinite density)..(what many scientists today consider a 'black hole') but the Earth was still the same 93,000,000 miles from the actual surface as it is today, the orbit of the Earth and the planets would remain, and the sun would not be considered a 'black hole', nor would it behave like one, (sucking in all its orbiting masses, and even light itself).. it would be much smaller, but given the exact same mass and distances surface to surface, little would change as far as orbits go... could this be correct theoretically?

ANSWER:
If the object is spherically symmetric and you are totally outside the mass distribution, then you are right only the total mass matters. But, this does not mean that black holes do not exist. It is dramatic to say that nothing can escape a black hole, not even light, if you are inside a critical distance called the Schwartzchild radius, but that does not mean that objects could not orbit the black hole, either inside or outside that radius. If the sun were a black hole, its Schwartzchild radius would be R=2GM/c²=2x6.7x10^-11x2x10³⁰/(3x10⁸)²=3,000 m; so all the planets would be outside and would orbit just fine; they could even be dragged away if you wanted. Only objects inside 3000 m would be "trapped" but even they could still orbit the black hole. The figure to the left shows some orbits of stars around the supermassive black hole at the center of the galaxy which have been observed by astronomers.

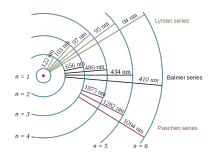
QUESTION:
I don't understand the hydrogen spectrum..the theory given in my book says that Balmer series will be observed when electron falls from n=3,4,5......(up to infinity) to n=2 but isn't n=2 for an electron of the hydrogen also an excited state,so it must fall back to its ground state?? Or am i getting something wrong here??

ANSWER:
Yes, it must get down to the ground state again once it reaches n=2. But that is part of a different series, called the Lyman Series. The radiation from that transtion is called the Lyman alpha line and is in the ultraviolet region of the spectrum. The rest of the series is n=3,4,5 going to n=1; all the lines in this series are in the ultraviolet. Every level has a series terminating on it.

QUESTION:
if i create vaccum on earth ,like in a spherical shell,if pierced it will suck air around it, its not the case with atmosphere of earth,why it is not that space vaccum suck it?gravity is indeed much stronger near earth and atmosphere much more denser ?

ANSWER:
The reason that air does not leak into space is that gravity holds it to the earth. Think of the air as a collection of molecules all moving around with different velocities. The velocities are distributed according to the Maxwell-Boltzmann distribution. At normal temperatures, most molecules (e.g., O₂, N₂, H₂O, CO₂, ) have almost no molecules have velocities large enough to escape the earth's gravitational pull. Lighter molecules, in particular H₂ and He, have much higher velocities and do escape into space; you will find almost no hydrogen or helium in our atmosphere.

QUESTION:
With respect to special theory of relativity, light speed c is an invariant, but wouldn't rest mass also be an invariant? If true, why is it not one of the postulates? Velocity and acceleration are not invariants, but are there other properties that are invariants?

ANSWER:
Light speed is not usually referred to as an invariant; it is simply a universal constant. Furthermore, as I have stressed before, I see no need to call the constancy of c a postulate; it is simply a result of the principle of relativity which states that the laws of physics be the same in all inertial frames. Rest mass is the inertia something has in its own rest frame, so of course it must be a constant. The word invariance usually refers to any quantity which remains constant when a Lorentz transformation is performed. For example, the total energy of an isolated particle E=√(m₀²c⁴+p²c²) is an invariant.

QUESTION:
I've been trying to calculate how much impact force an object weighing 2,000,000 lbs traveling at 120,000mph will generate. I've run calculation conversions that tells me that the Newtonian Force is a whapping 48665704243.2 but what does that mean? I read about how an 80 pound 1 foot long object traveling at 52,000mph hit the surface of the moon with a force equivalent to 5 Tons of TNT exploding, creating a 65 foot wide crater! What would my 1,000 Ton 157.64 Mach object do?

ANSWER:
For the umpteenth time, you cannot calculate a force by knowing the mass and velocity of something; see my FAQ page. What you can do, as your example does, is calculate the energy which the object carries in. I will not do what I usually do and convert to SI units since you seem to like English units. The kinetic energy something has is K= MV² where M is the mass and V is the speed. Using your example, K_TNT=5 if K= ∙80∙52,000²=1.1x10¹¹ lb∙mph², so to convert energy in lb∙mph², to energy in tons of TNT you need to multiply by a factor of 5/1.1x10¹¹ lb∙mph²=4.6x10^-11. So, ∙2x10⁶∙120,000²∙4.6x10^-11=6.6x10⁵ tons=0.66 Megatons of TNT. For comparison, the bomb dropped on Nagasaki in WWII was about 0.02 Megatons.

QUESTION:
Why are loops provided for transporting oil/water for longer distances?

ANSWER:
When the temperature of the pipe changes it changes length. In the figure to the left, the pipe will expand if you heat it up and contract if you cool it down. If it were just a straight pipe, the resulting forces on the pipe along its length could be large enough to cause it to buckle and fail. Inserting loops allows the length changes to be taken up by the size of the loop as shown.

QUESTION:
Can u get pure energy? Can you make it visible with power modulation?

ANSWER:
Since mass is just another form of energy, everything you can see or feel is pure energy. Maybe you mean energy without mass? In that case, the answer would be electromagnetic radiation like light or radio waves. I have no idea what your second question means.

QUESTION:
My question relates to time dilation and the speed of light. When we consider the passage of time, and the speed of an observer or traveler on Earth, how does the speed of our planet around the sun, our arm of the galaxy and the speed of the galaxy itself play into the speed of time for someone on Earth? We talk about a spaceship travelling at a certain speed, but isn't the Earth itself like a spaceship for its inhabitants? And isn't the Earth rotating around the sun at a certain speed, and the spiral arm of our galaxy also rotating at a certain speed? So isn't the Earth also?

ANSWER:
Time dilation is meaningful only as a relative thing. One observer can measure the rate at which the clock of another observer runs. Relative to a clock on the sun, an earth clock would run slowly. Relative to the center of the galaxy, an earth clock would run slowly differently. Relative to a clock on Andromeda galaxy, an earth clock would run slowly differently. This is the thing about relativity it is only relative measurements that mean anything; that's why they call it relativity. Underlying your question is the misconception that there exists some absolute rest relative to which all clocks in the universe will run. There is no such thing as "absolute rest"; any inertial frame may be thought of as being at rest. Incidentally, for all but the most accurate measurements, the time dilations I have alluded to are negligibly small because the relative speeds are small compared to the speed of light.

QUESTION:
I don't understand angular momentum but as a first step here is my question. If you hold out weights and turn on a pivot and bring the weights in; are the weights moving at the same velocity and you are spinning faster so that the weights move at the same velocity or are the weights actually moving faster?

ANSWER:
The angular momentum L of a mass M a distance R from the axis around which it is rotating is of L=MVR where V is its speed. It requires no torque to change R, so L will stay the same if R is changed because angular momentum of a system on which there are no torques is constant (conserved). So, for example, if you change R to R, V will double. If there were no person spinning with the weights and no external torques (like friction), this would be your answer. It is more complicated if you have anything other than the weights. You probably want to ignore the rest of the answer below, but I want to do it for completeness.

If you take into account the presence of the person you need to introduce the moment of inertia I. The angular momentum now is written as L=Iω where ω is the angular velocity (in radians per second) of the system. If a point in the system is a distance R from the axis of rotation and moving with a speed V, the angular velocity is ω=V/R. So, comparing with the idealized situation above you can see that the moment of inertial of a point mass must be I_point-mass=MR². Now, the man holding the weights has some moment of inertia which I shall call I_man; I will ignore the contributions from his arms, so his moment of inertia does not change when he pulls in the weights. The total angular momentum at the beginning is now L₁=(I_man+2MR²)ω₁=(I_man+2MR²)V₁/R. So let's again reduce the distance to R. The new angular momentum is L₂=(I_man+2M( R)²)ω₂=(I_man+ R²)V₂/( R); this needs to be the same as the original angular momentum, so (I_man+ MR²)V₂/( R)=(I_man+2MR²)V₁/R. Solving, V₂= V₁(I_man+2MR²)/(I_man+ MR²). Note that if I_man=0, you get the same as above, V₂=2V₁. If I_man>>2MR², there will be negligible change in angular velocity and so V₂= V₁ because V=Rω and ω did not change. Finally, there must be a case where I_man is just right that V₂=V₁; that would be if I_man=MR²and would correspond to ω₂=2ω₁.

QUESTION:
Where can I find the assumptions for conservation of linear momentum? I'm specifically looking for a source which says that both bodies in a linear momentum problem have to attain a common velocity. The assumption or condition I'm looking for is so basic I can't find it.

ANSWER:
You can read my earlier answer on momentum conservation. I am afraid I have no idea what you mean by "both bodies have to attain a common velocity". In fact, that does not even sound right to me for a general case.

QUESTION:
The question, "Why does water freeze at 32 deg. F?" has been proposed all over the internet. There is not an adequate answer to be found. The answers will describe how it freezes, and what takes place, but never why it freezes. The freezing point of hydrogen is -241.746 degrees celcius and the freezing point of oxygen is -218.79 C. So why does water freeze at 0 deg C?

ANSWER:
Iron freezes around 1200⁰C, mercury at -39⁰C, etc. The temperature of the phase transition between solid and liquid depends on the material; it is not a simple thing to predict exactly where that transition will occur, but it can be done in some cases. It is also dependent on the pressure. But I am curious: Why do you particularly ask about water? Why didn't you ask me "why does molybdenum freeze at 2620⁰C?" This question is "all over the internet"? Guess i missed that.

QUESTION:
Hello,when we look at objects with triangular prism instead of of eye glasses we see spectrum or rainbow on objects edges ,why this spectrum is always formed on the edges. I mean the edges of objects for example chair,wall etc. I mean things i look at through prism instead of eyeglasses.

ANSWER:
The ideal situation for seeing a spectrum from a prism is if you have white light, all coming from the same direction and preferably through a narrow slit. If you just hold the prism up and look through it, light of many colors is coming from all directions. When you have an obstruction, like the edge of a table leg, for example, it lis like "half a slit" and light coming from the edge is restricted to come mostly from one direction. If that light has a fairly large fraction of white light, you are likely to see a rainbow spectrum.

QUESTION:
Hi I'm a high school student and having hard time understanding something about electric potential energy. Do electrons moving in a circuit have potential energy because battery has done some work moving them against electric field (from low potential to high potential)? If yes, suppose that I just put the wires around the battery and think about the moment when it took 1 electron from low potential to high potential. That electron now has a potential energy equal to the energy spent when moving the charge from low to high potential. But since a battery doesn't generate electrons (and just providing force to move them) and all other electrons were already there in the copper wire, how did other electrons get their potential energy? The battery hasn't done any work for them (or did it?).

ANSWER:
The electric current in a conducting wire is not the best way to learn about potential energy of charges because it is a rather complicated process. What happens is that the potential difference across the ends of the wire cause there to be an electric field inside the wire. Electrons see this field and therefore each believes that it is in an approximately uniform field and therefore it accelerates and gains kinetic energy as it loses potential energy it has by virtue of the field. But what next happens is that little electron almost immediately encounters an atom in the wire, collides with it, and loses some or all the kinetic energy it has just acquired and has to start all over again. So each electron bounces slowly along the wire, repeatedly gaining and then losing kinetic energy. On average, there is a net drift of electrons down the wire but it is really quite slow and we consider the average electron to move with a constant drift velocity. So any electron, moving from the negative terminal of the battery to the positive terminal of the battery of potential difference V moved, on average, with no change of kinetic energy but it has lost eV Joules of potential energy. Where did that energy go? Put your hand on the wire and you will see that it has warmed up. If you want a more lucid example of electrons and potential energy, imagine a uniform electric field of strength E (like in the gap between plates of a parallel plate capacitor) with an electron released at some point. Then as the electron moves, accelerating along the direction opposite the field (because it is a negative charge), it loses potential energy eEz where z is the distance it has traveled. After it has gone a distance z, it will have acquired a kinetic energy mv²=eEz.

QUESTION:
I am reading the ABC of Relativity. This is what I don't understand. If I get on a train in London and travel to Edinborough why is it equally true that Edinborough is travelling towards me? It am the one who initiates the motion. If Edinborough is really moving towards me, how is it also moving towards someone approaching it from the North, at the same time?

ANSWER:
The important thing to understand is that there is no test you can do which determines who is "really" at rest there is no such thing as one frame of reference which is absolutely at rest. The way this is expressed in the theory of special relativity is the laws of physics are the same in all inertial frames of reference. (An inertial frame is one which moves with a constant velocity in a straight line, no acceleration allowed.) This means that there is no experiment you can do inside your train which will have different result if you were in Edinborough or any other train. All velocities are relative and it does not matter whom you consider to be at rest to do physics. You, before you left London, were in the same frame as Edinborough, and nobody would argue that it was you which caused the change to a different frame since you were the one who accelerated and consumed diesel fuel; that does not change the fact that the two frames are, in all ways, equivalent once you are done accelerating.

By the way, it turns out that the laws of physics have to be the same in all frames, including accelerating frames. This is the basis for the theory of general relativity. But, you need not worry about this is you are just studying special relativity. If you are interested, see my FAQ page

QUESTION:
I was playing a game known as ''Fallout 3'' and in the game there are laser weapons. The laser weapons are powered by a marshmallow sized microfusion cells that are basically miniature nuclear reactors that fuses hydrogen atoms. In the game they produce enough power to turn a 500 kilogram bear into ash in one second. So could a reactor that small produce enough power for the gun and how energy much would a marshmallow sized blob of fused hydrogen produce? A normal microfusion cell in the game has enough energy to fire 24 of these shots. So would it be possible in any way for these laser weapons to be able to be this powerful with an energy source like the microfusion cell?

ANSWER:
I have no way to estimate the "power to turn a 500 kilogram bear into ash in one second". I am sure you realize that, with today's technology, the possibility of there being such a power supply is zilch. Let's just do a few estimates to show how hard this is. One gram of hydrogen fuel (deuterium + tritium), if fully fused into helium+neutrons, releases something on the order of 300 GJ of energy; so, if released in 24 one second pulses, each pulse would be about 10 GW. That is probably way more than your bear burning would need, so let's say 100 MW would do it; so, we would need about 10^-2 g of fuel. I calculate that to confine that amount of gas in a volume of 10^-5 m³ (about 1 in³) would require a pressure of about 5,000,000 atmospheres! That, in itself, should be enough to convince you that this machine could probably never be possible. If you need more convincing, consider shielding: 80% of the energy produced is in the kinetic energy of neutrons. How are you going to harvest that energy in such a small volume and how are you going to protect yourself from the huge neutron flux? And surely there needs to be some sort of mechanism to control the process and convert the energy into usable electrical energy to power the laser; all that is supposed to fit into 1 in³? This truly is a fantasy game with no connection to reality!

QUESTION:
I read that when no external forces act on a system, the internal forces are paired to balance the momentum. So if the Universe is considered as such a system where momentum is indeed conserved, every action will have equal and opposite reaction. Is this a sufficient explanation to consider Newton's third law to be a special case of Newton's second law?

ANSWER:
Suppose that Newton's third law were not true. For any single particle in a system of particles Newton's second law would be true but you would not be able to apply it to the system as a whole. Without Newton's third law, the total linear momentum of an isolated system of particles would have no physical significance, it would not be a conserved quantity. Newton's second law refers only to a single particle and is useful for systems of particles only because Newton's third law is also true. I fail to see how that makes the third law a "special case" of the second.

QUESTION:
If you were to take a 2 liter bottle of air, down to 10 meters and were to let a slight amount of water into the bottle and then sealed the bottle, what would happen to the bottle when you brought the bottle back to the surface?

ANSWER:
It is always hard for a scientist to deal with amounts like "a slight amount"! If you did not let any air out of the bottle, the pressure inside would be "a slight amount" higher. If you let in as much water as would go in without any air escaping, the gauge pressure of the contained air would be about 1 atmosphere, that is, twice atmospheric pressure.

QUESTION:
If two deuterium atoms collide at an ideal velocity and at an ideal angle, are there conditions where fusion will not occur and some sort of scattering will take place? If so, why or how would scattering occur?

ANSWER:
I do not know what you mean by "ideal velocity" or "ideal angle". I can tell you that whenever the two deuterons come close enough together to interact, there are a great many things which can happen, all with specific probabilities ("cross sections" in the usual parlance). Essentially any possible reaction allowed by conservation laws and selection rules can happen. Nearly always, the most likely thing to happen is elastic scattering where two deuterons exit after the interaction.

QUESTION:
Assuming I had a piece of string that was a light year long and I was holding it on 1 end and my friend was 1 light year away holding the other end, with no slack in the string. If I pulled it would they feel the immediately, or no? If not, why?

ANSWER:
You would not believe how many times I have gotten variations of this question. See my faq page for a similar question. In a nutshell, the information would travel at the speed of sound in your string so it would take far longer than one year before your friend felt your tug.

QUESTION:
What would happen to a fly while an airplane takes off assuming that the fly was already flying forward while the plane was at rest?

ANSWER:
The effect on the air due to the acceleration of the airplane is quite small; the air pressure at the rear of the plane would increase very slightly. In other words, the air stays almost at rest relative to the airplane. Since the fly flies relative to the air, he is mostly unaffected. He is, however, accelerating along with the air and the plane, so he would have to fly a little harder in the forward direction of the plane to continue moving the same as he was; since a typical acceleration of a commercial jet is about 3 m/s², this additional forward force would be about 1/3 of his weight.

QUESTION:
why are soap bubbles colorful?what colors are observed when a soap bubble is illuminated by monochromatic light?

ANSWER:
The reason is that a soap film has two surfaces from which reflection of light can occur and they are very close to each other. Therefore, light of certain colors will interfere constructively while light of other colors will interfere destructively. A pretty clear explanation as well as a calculator may be found on the hyperphysics site. If you illuminate the bubble with monochromatic light, you will only see that color or no light reflected at all.

QUESTION:
I just watched a nature document about the largest snake that ever lived on Earth that was the Titanoboa that could exert a pressure of 400 pounds per square inch and that lived in the water mostly. So my question is that could a Titanoboa destroy a submarine by coiling around it with the pressure of 400 pounds per square inch assuming that the submarine is small enough for the 48 foot snake to coil around it?

ANSWER:
The pressure under water increases by about 0.44 psi/ft. To get to 400 psi, therefore, a submarine must go to a depth of 400/0.44=909 ft. WWII U-boats had collapse depths of 660-920 ft. Modern submarines have collapse depths of around 2400 ft. So, it depends on the submarine, but most modern ones could withstand 400 psi. The only proviso is that they are designed to have the pressure uniformly distributed over the whole surface, not a narrow band where a snake would squeeze; a very localized pressure could cause a structural failure at a lower pressure.

QUESTION:
I came across this just now. It implies a balloon full of air weighs more than the same balloon empty. That doesn't feel right to me as an inflated balloon is surely buoyant by the amount of air it contains. i.e. The extra weight of the air is cancelled out by the buoyancy. The site above is a respected organisation and I would be surprised if is recommending an experiment based on a false assumption. However, I cannot see how the instructions they give could possibly be used to determine the density of air.

ANSWER:
You are right, the experiment ignores the buoyant force on the balloon; if the density of the air in the balloon were identical to the density of the air outside the balloon, the experiment would fail to find any weight of air. However, the pressure inside the balloon is greater than the pressure outside and therefore the density of the air inside is larger than normal atmospheric density. The experiment then measures the difference between the mass inside the balloon and the mass of an equal volume of air at atmospheric pressure. This would still be a reasonable order-of-magnitude measurement of the density of air. A more accurate experiment would be to measure the pressure to which the balloon was inflated; with that information you could do a better measurement of the air density.

QUESTION:
I'm interested in why we loose our signal when two walkie talkies get too far apart even there is no obstacle between us. Range of reach of EM waves is infinite, so what's the reason?

ANSWER:
You are right, in a vacuum electromagnetic waves last forever. You are not in a vacuum, but absorption by air is probably very small for your walkie talkies. The problem is intensity of the waves. If you are far away compared to the size of the unit, you can say that the signal spreads out from the sending unit like a sphere. But, there is only a certain amount of energy in that wave and so the amount of energy intercepted by the receiving unit gets smaller and smaller the farther away you go. Eventually, the receiving unit is not sensitive enough to detect the signal.

QUESTION:
What should be the temperature of a gas molecule if it needs to escape out of earth's gravitational pull suppose if we take the case of oxygen at what temperature its average velocity will be enough to escape earth's gravitational pull?

ANSWER:
Temperature of a gas is a statistical quantity, no single gas molecule has a temperature. A gas of a particular temperature has a distribution of speeds called the Maxwell-Boltzmann distribution which contains all possible speeds. The figure to the left shows this distribution for N₂ for several temperatures. The escape velocity from the surface of the earth is about 11,000 m/s, so you can see that a heavy molecule like nitrogen has almost no molecules going that fast at normal temperatures (300 K), or even if T=1000 K. The picture to the right compares the distribution of speeds for N₂ with that for H₂, about 14 times lighter. You see that the most likely speed of H₂is about 4 times faster than N₂. Comparing with the figure to the left, 300 K hydrogen gas would have a most probable speed of more than 1200 m/s. Although there are still very few with speeds higher than 11,000 m/s, there are still a few which escape. Eventually, as the slower molecules speed up to fill in the distribution, they would essentially all leak out of the atmosphere. That is the reason why there is almost no hydrogen or helium in the atmosphere. Most hydrogen on earth is locked up in water and other molecules, but since helium is inert, the only source of it is from underground, usually as a byproduct of natural gas wells. The form of the Maxwell-Boltzmann distribution is given by 4π[m/(2πkT)]^3/2v²exp[-mv²/(2kT)].

QUESTION:
Assume we're in a very large hollow sphere. (let's say r = 1 light year). Then we take a very powerful laser with extremely low diffraction and we fire it while effectuating a full rotation with it. Wouldn't the laser point travel faster than light on the inside surface of the sphere? Or does it fall as into the immaterial category and is exempted from the rule?

ANSWER:
You do not need such an extreme condition to do what you want. The distance to the moon is about R=3.8x10⁸ m, so if you swept your laser beam across the surface of the moon the speed of the spot would be equal to the speed of light c=3x10⁸ m/s if c=Rω where ω is the angular velocity you are rotating the laser. So, ω=3x10⁸/3.8x10⁸=0.79 radians/second=45⁰ per second really easy to do. But that spot is not anything, really, because the spot a second from now will not be "made of" the same photons that it is made of right now. The most important thing is that there is no way that this spot could be made to carry information from one point on its path to another. And you are right, it has no mass, but even massless light cannot travel faster than the speed of light.

QUESTION:
As a chemistry teacher, I often get questions from students that are best asked of a physicist. Is there a "short" answer to explain the nature of charge? Why are there only two charges? We understand that the assignment of the negative to the electron could be completely arbitrary, but what exactly is a/the "negative charge"?

ANSWER:
For every force field in nature there is a corresponding source of that field: gravitational mass is the source of gravitational fields, electric charge is the source of electric fields, electric currents are the source of magnetic fields, quarks are the source of nuclear fields, etc. Further, if there are two such sources in proximity of each other, each experiences a force due to the field of the other. We first are aware of gravitational fields and conclude after some experimentation that the property something must have to be a source of that field is mass. Inspired by our success in understanding the gravitational field, we start looking around for other forces. Combing our hair one morning, we notice a new kind of force which is obviously not gravity. After some experimentation we discover that some objects in nature cause electric fields if they have a property which we call electric charge. So, putting two electric charges near each other, we find, not unexpectedly, that both experience a force due to the other. But now there is something different. In gravity, the force between two masses is always attractive, never repulsive. So mass is a relatively simple thing because there is only one kind of it. But with electric charges, sometimes the force is attractive and sometimes repulsive, so we conclude that there must be two kinds of electric charge which are most easily distinguished from each other by assigning a sign, + or -. Of course, further experimentation reveals that opposite charges attract and like charges repel. Why are there two? Well, just because that is the way nature is. You might just as well ask why there is only one kind of gravitational mass. The way that quarks interact with each other is more complex than just attractive or repulsive and three kinds of the quark property called "color charge" are required. So the answer to "what exactly is charge?" is that it is that property which something has which allows it to both cause and feel an electric field.